the geometry of whales and ants non-euclidean geometry
At the same time that a revolution was going on in algebra, similar events were taking place in geometry. Two millennia earlier, Euclid had written down a short set of axioms from which, supposedly, all of geometry could be derived. These axioms were intended to be self-evident truths that did not require any proof.
For centuries Euclid’s Geometry was considered the ne plus ultra of deductive reasoning. The eighteenth-century philosopher Immanuel Kant built up a theory of knowledge, in which he cited Euclid’s geometry as an example of “synthetic a priori” truth—in other words, infallible knowledge about the universe that is derived from pure reason rather than observation.
However, one axiom had always appeared a little bit clumsier than the others. The axiom in question is the “Parallel Postulate,” which Euclid does not use until late in his first book: “If a straight line falling on two straight lines makes the interior angles on the same side less than two right angles, the two straight lines, if produced indefinitely, meet on that side on which are the angles less than two right angles.” This assumption is used, for example, to prove that the sum of the angles of a triangle equals 180 degrees.
Many mathematicians felt the Parallel Postulate was true but far from self-evident, and thus a flaw in Euclid’s otherwise sterling system of axioms. They took up the challenge of proving it from the other axioms that Euclid had provided. This mathematical grail quest lured the famous and obscure alike. Legendre (whom we have met already) believed that he had proved it. So, at one time or another, did less-famous mathematicians like John Wallis, John Playfair, Girolamo Saccheri, Johann Lambert, and Wolfgang Bolyai. In all cases, they made hidden assumptions that, under the harsh light of scrutiny by other mathematicians, were no better motivated than Euclid’s postulate.
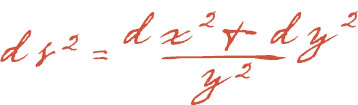
dx and dy represent the sides of an “infinitesimal” triangle, and ds represents their hypotenuse.
In the first half of the nineteenth century, three men separately and independently dared to think the unthinkable. Perhaps a valid geometry might exist in which the Parallel Postulate was actually false. This would be a non-Euclidean geometry—that is, a geometry in which one of the axioms laid down by Euclid, more than two millennia earlier, is expressly violated.
This idea was just as heretical as Hamilton’s idea of an algebra with no commutative law. However, denying the Parallel Postulate took perhaps even more courage, because it had the great weight of Euclid, Kant, and two thousand years of tradition behind it.
The first of the three revolutionaries was Karl Friedrich Gauss, the most famous mathematician of his era. Gauss, a friend of Bolyai from their student years, dabbled at proving the Parallel Postulate in the early 1800s. But gradually, around 1820, he seems to have become convinced that an alternative, non-Euclidean geometry could be constructed. However, he never published this idea, and only alluded to it somewhat vaguely in letters. The best evidence of his reasons comes from a letter he wrote in 1829 to his friend, Friedrich Bessel, in which he says that he feared the “howl from the Boeotians” (a pejorative term for stupid people) that would ensue if he published his work.
THE SECOND DISCOVERER of non-Euclidean geometry was Janos Bolyai, the son of Gauss’s old school chum. Wolfgang, who became a mathematics teacher in Hungary, tried to warn his son against trying to prove the Parallel Postulate: “For God’s sake, I beseech you, give it up. Fear it no less than sensual passions because it, too, may take all your time, and deprive you of your health, peace of mind, and happiness in life.” But his son ignored the advice, and he eventually wrote a 24-page treatise on what he called the “absolute science of space,” which his father generously published as an appendix to one of his textbooks in 1832.
The elder Bolyai naturally sent a copy to his old friend Gauss, who responded in unexpected fashion: “To praise [this work] would amount to praising myself. For the entire content of the work, the approach which your son has taken, and the results to which he is led, coincide almost exactly with my own meditations … It was my plan to put it all down on paper eventually, so that at least it would not perish with me. So I am greatly surprised to be spared this effort, and am overjoyed that it happens to be the son of my old friend who outstrips me in such a remarkable way.”
In spite of the compliment at the end, it was a crushing blow to the younger Bolyai. Gauss was saying that his discovery of non-Euclidean geometry was nothing new. Janos never published another mathematical paper in his life. Not only had Gauss lacked the courage to publish the discovery himself, he had now compounded his mistake by discouraging an aspiring young mathematician who might have made a great name for himself.
Because Gauss was too reticent, and Bolyai gave up too easily, the third discoverer of non-Euclidean geometry deserves the most credit for bringing it to the world’s attention. He was Nikolai Ivanovich Lobachevsky, a Russian mathematician who lived in Kazan, the ancient capital of the Tatars. He first published his version of non-Euclidean geometry in 1829 in a very obscure Russian journal, but unlike Bolyai he continued to write articles and books about it and finally succeeded in getting an article into Crelle’s Journal in 1837. Even so, he did not receive the kind of acclaim during his lifetime that one might expect. Today, however, Lobachevski is considered one of the first great Russian mathematicians, and in Russia his geometry is called Lobachevskian. Western mathematicians call it, more descriptively, hyperbolic geometry.
What exactly is hyperbolic, or Lobachevskian, geometry? I think that the best way to think about it is to forget all about the Parallel Postulate and about Euclid. You must especially forget about the prejudice that you have surely been brought up with, that Euclidean is the “natural” geometry of the real world. Hyperbolic geometry is no more artificial than Euclidean. Think of it as the geometry of the ocean. If whales had invented geometry, the geometry they would have invented would be hyperbolic.
Suppose, for a moment, that you are a whale. Light is not very useful in the deep ocean, because the water is dark. So you mostly communicate and experience the world through sound. The shortest distance between two points in your world would be the path taken by sound waves. To you, this would be the analogue of a straight line.
Now here’s the catch. Sound does not travel at a constant speed in the ocean. Below a certain depth, roughly 2000 feet (600 meters), it travels at a speed that is proportional to the depth below the surface. So the path that sound waves travel is not straight, but curved. A sound wave will get from whale A to whale B quicker if it goes downward, to exploit the greater sound speed at depth, and then comes back up. In fact, we can be more precise about the nature of these curves: they are arcs of circles centered at the ocean surface! Thus, to a whale, what humans call a “circle” is actually a “line” (the shortest distance between two points).
Below Demonstration of the curves along which sound travels in the ocean.
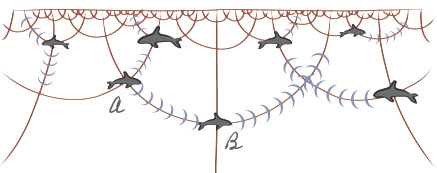
Whale Geometry is a geometry where some surprising (to us) things happen, but they would not be the least bit surprising to whales. The sum of the angles of a triangle is less than 180 degrees. Rectangles (four-sided figures with all right angles) do not exist; however, right-angled pentagons do. Most importantly, it is a geometry of negative curvature. This means that lines that start out parallel tend to move farther and farther apart.
AMAZINGLY, ANOTHER non-Euclidean geometry, besides hyperbolic geometry, had been known for centuries—only no one ever thought of it in those terms. It is the geometry of a sphere. On the surface of a sphere (such as Earth), the sum of the angles of a triangle is greater than 180 degrees. Rectangles do not exist, but right-angled triangles do. Keep in mind the curvature of the Earth! For example, a triangle can be drawn with three right angles: start at the North Pole, travel in a straight line down to the Equator, then travel due east or west a quarter of the way around the globe, and then go due north again. You will trace out a triangle with three 90-degree angles. Spherical geometry is a geometry of positive curvature. In other words, lines that start out parallel (such as meridians, near the Equator) tend to move closer and closer together, and they eventually converge at the poles.
The reason that no one ever thought of spherical geometry as an alternative to Euclidean geometry is simple: We can see a sphere as being imbedded in three-dimensional Euclidean space, so its “non-Euclideanness” is not immediately obvious. Suppose, however, that you were unable to perceive a third dimension beyond the surface of the sphere. For example, perhaps you are an ant, living on the surface of an asteroid with no oceans (so you can go anywhere you want to). You have no concept of space, no concept of underground; everything you know is the surface of your spherical world. The curvature of that world is positive and its geometry is non-Euclidean. We could call it Ant Geometry.
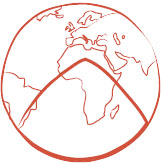
Left Spherical geometry and the curvature of the Earth.
Instead of one geometry of nature, we can now see there is a whole spectrum of geometries with different amounts of curvature, ranging from Ant Geometry (spherical) to Human Geometry (Euclidean) to Whale Geometry (hyperbolic). But that’s not all. These are only the geometries of constant curvature. We can also imagine geometries whose curvature varies from place to place. They can be two-dimensional, three-dimensional, or even higher. Gauss (perhaps influenced by his unpublished thoughts on hyperbolic geometry) was the first mathematician to understand the concept of varying curvature in a two-dimensional space, and his student Bernhard Riemann extended the concept to higher dimensions in 1854. Both of them thus anticipated one of the epochal discoveries of the twentieth century: Albert Einstein’s theory of general relativity, which postulates that our four-dimensional spacetime has curvature that varies from place to place. Without Lobachevski, Bolyai, Gauss, and Riemann, Einstein would never have been able to write down the equations for his theory.
Above An engraving displaying an “Allegory of Geometry,” by F. Floris, 16th century.