
Ethics Questionnaire
This questionnaire is part of an ongoing study of attitudes toward ethical issues that arise in business and personal life. Please read each question carefully and try to imagine yourself in the situation it describes. Then check the most appropriate response category for each question.
QUESTION #1.
In an effort to increase productivity, the owner of a small business has ordered ten personal computers for use by his staff. When the UPS shipment arrives, he notices that the invoice from the mail-order house bills for only nine PCs, even though all ten were included with the shipment.
The owner has two options. (1) He can inform the mail-order house of its error and ask to be billed for the correct amount; or (2) he can pay the amount shown on the invoice and take no further action.
If the owner pays the amount shown, the worst thing that can happen is that the mail-order house may later discover its error and bill him for the tenth computer. There is a high probability (.99, say) that the error will never be discovered.
What do you believe the chances are that the owner will inform the mail-order house of its mistake and ask to be billed for the correct amount? (Check one.)

QUESTION #2.
If YOU were the owner in the situation described in Question #1, what are the chances you would inform the mail-order house of its mistake and ask to be billed for the correct amount? (Check one.)

After attending a football game, you return home to discover that you have lost an envelope from your jacket pocket. The envelope contains $100 in cash and has your name and address written on the outside. A stranger has found the envelope.
What would you say the chances are that this person will return your $100 to you? (Check one.)

QUESTION #4.
If YOU found $100 in an envelope like the one described in Question #3, what are the chances that you would return the stranger’s cash? (Check one.)

For each of the following, please check the category that applies to you:
Sex: male __________ female __________
Class: freshman __________ sophomore __________
junior __________ senior __________ other __________
There will be a follow-up to this questionnaire in December. In order to match the follow-up questionnaire with this one, we need an identifying code for each of you, one that preserves your anonymity and that you will be able to recall easily in December. Past experience has taught that a code with these properties can be made from your middle name and your mother’s maiden name.
Your middle name _______________________________________________
Your mother’s maiden name __________________________________
Thank you very much for your cooperation.
1. This allocation procedure is described in a longer, unpublished version of the Carter and Irons paper (1990).
2. Because each subject responded twice, the 534 choices reported in figure 9.7 are not statistically independent, and so the most direct test of statistical significance, the chi-square test, is inappropriate for the sample as a whole. To overcome this problem, we performed a chi-square test on the number of subjects who made the same choice—cooperate or defect—in both of their games. There were 207 such subjects (78 percent of the sample). The pattern of results observed in this restricted sample is essentially the same as the one observed for the sample as a whole.
3. Because each subject played the game twice, the individual responses are not statistically independent. To get around this problem, we limited our sample to the 207 subjects who either cooperated with, or defected on, each of their two partners. The sixty subjects who cooperated with one partner and defected on the other were deleted from the sample. The dependent variable is the subject’s choice of strategy, coded as 0 for “cooperate” and 1 for “defect.” The independent variables are “econ,” which takes the value 1 for economics majors, 0 for all others; “unlimited,” which is 1 for subjects in the unlimited version of the experiment, 0 for all others; “intermediate,” which is 1 for subjects in the intermediate version, 0 for all others; “limited,” which is the reference category; “sex,” coded as 1 for males, 0 for females; and “class,” coded as 1 for freshmen, 2 for sophomores, 3 for juniors, and 4 for seniors.
The Effect of Education Level on Defection Rates
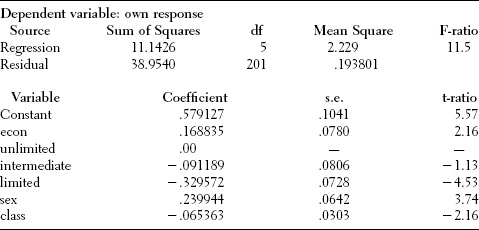
R2 = 22.2% R2(adjusted) = 20.3%
s = 0.4402 with 207 − 6 = 201 degrees of freedom
Because the conventional assumptions regarding the distribution of the error term are not satisfied in the case of linear models with dichotomous dependent variables, the standard OLS significance tests are not valid. So we also ran models based on the probit and logit transformations. The statistical significance patterns shown by the coefficients from these transformed models were the same as for the OLS model. Because the coefficients of the OLS model are more easily interpreted, we report the remainder of our results in that format only.
4. As did the whole-sample regression reported earlier, the regression reported below employs only those subjects who either cooperated in both games or defected in both games.

R2 = 16.4% R2(adjusted) = 12.8%
s = 0.4673 with 124 − 6 = 118 degrees of freedom
5. For microeconomics A, the average September responses to questions 1–4 were 30.9, 50, 30.4, and 70.1, respectively; for microeconomics B, the corresponding responses were 29.9, 47.4, 34.4, and 68.2.