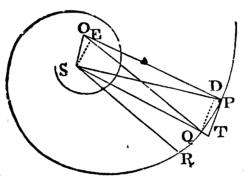
LEMMA III.
Let PQR be a spiral cutting all the radii SP, SQ, SR, &c., in equal angles. Draw the right line PT touching the spiral in any point P, and cutting the radius SQ in T; draw PO, QO perpendicular to the spiral, and meeting in O, and join SO. I say, that if the points P and Q approach and coincide, the angle PSO will become a right angle, and the ultimate ratio of the rectangle TQ 2PS to PQ² will be the ratio of equality.

For from the right angles OPQ, OQR, subduct the equal angles SPQ, SQR, and there will remain the equal angles OPS, OQS. Therefore a circle which passes through the points OSP will pass also through the point Q. Let the points P and Q coincide, and this circle will touch the spiral in the place of coincidence PQ, and will therefore cut the right line OP perpendicularly. Therefore OP will become a diameter of this circle, and the angle OSP, being in a semi-circle, becomes a right one. Q.E.D.
Draw QD, SE perpendicular to OP, and the ultimate ratios of the lines will be as follows: TQ to PD as TS or PS to PE, or 2PO to 2PS; and PD to PQ as PQ to 2PO; and, ex aequo perturbatè, to TQ to PQ as PQ to 2PS. Whence PQ² becomes equal to TQ 2PS. Q.E.D.
PROPOSITION XV. THEOREM XII.
If the density of a medium in each place thereof be reciprocally as the distance of the places from an immovable centre, and the centripetal force be in the duplicate ratio of the density; I say, that a body may revolve in a spiral which cuts all the radii drawn from that centre in a given angle.
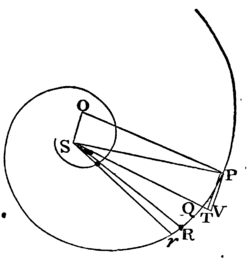
Suppose every thing to be as in the foregoing Lemma, and produce SQ to V so that SV may be equal to SP. In any time let a body, in a resisting medium, describe the least arc PQ, and in double the time the least arc PR; and the decrements of those arcs arising from the resistance, or their differences from the arcs which would be described in a non-resisting medium in the same times, will be to each other as the squares of the times in which they are generated; therefore the decrement of the arc PQ is the fourth part of the decrement of the arc PR. Whence also if the area QSr be taken equal to the area PSQ, the decrement of the arc PQ will be equal to half the lineola Rr; and therefore the force of resistance and the centripetal force are to each other as the lineola ½Rr and TQ which they generate in the same time. Because the centripetal force with which the body is urged in P is reciprocally as SP², and (by Lem. X, Book I) the lineola TQ, which is generated by that force, is in a ratio compounded of the ratio of this force and the duplicate ratio of the time in which the arc PQ is described (for in this case I neglect the resistance, as being infinitely less than the centripetal force), it follows that TQ SP², that is (by the last Lemma), ½PQ² SP, will be in a duplicate ratio of the time, and therefore the time is as PQ; and the velocity of the body, with which the arc PQ is described in that time, as or, that is, in the subduplicate ratio of SP reciprocally. And, by a like reasoning, the velocity with which the arc QR is described, is in the subduplicate ratio of SQ reciprocally. Now those arcs PQ and QR are as the describing velocities to each other; that is, in the subduplicate ratio of SQ to SP, or as SQ to; and, because of the equal angles SPQ, SQr, and the equal areas PSQ, QSr, the arc PQ is to the arc Qr as SQ to SP. Take the differences of the proportional consequents, and the arc PQ will be to the arc Rr as SQ to SP -, or ½VQ. For the points P and Q coinciding, the ultimate ratio of SP - to ½VQ is the ratio of equality. Because the decrement of the arc PQ arising from the resistance, or its double Rr, is as the resistance and the square of the time conjunctly, the resistance will be as. But PQ was to Rr as SQ to ½VQ, and thence becomes as, or as. For the points P and Q coinciding, SP and SQ coincide also, and the angle PVQ becomes a right one; and, because of the similar triangles PVQ, PSO, PQ becomes to ½VQ as OP to ½OS. Therefore is as the resistance, that is, in the ratio of the density of the medium in P and the duplicate ratio of the velocity conjunctly. Subduct the duplicate ratio of the velocity, namely, the ratio, and there will remain the density of the medium in P, as. Let the spiral be given, and, because of the given ratio of OS to OP, the density of the medium in P will be as. Therefore in a medium whose density is reciprocally as SP the distance from the centre, a body will revolve in this spiral. Q.E.D.
Cor. 1. The velocity in any place P, is always the same wherewith a body in a non-resisting medium with the same centripetal force would revolve in a circle, at the same distance SP from the centre.
Cor. 2. The density of the medium, if the distance SP be given, is as, but if that distance is not given, as. And thence a spiral may be fitted to any density of the medium.
Cor. 3. The force of the resistance in any place P is to the centripetal force in the same place as ½OS to OP. For those forces are to each other as ½Rr and TQ, or as and, that is, as ½VQ and PQ, or ½OS and OP. The spiral therefore being given, there is given the proportion of the resistance to the centripetal force; and, vice versa, from that proportion given the spiral is given.
Cor. 4. Therefore the body cannot revolve in this spiral, except where the force of resistance is less than half the centripetal force. Let the resistance be made equal to half the centripetal force, and the spiral will coincide with the right line PS, and in that right line the body will descend to the centre with a velocity that is to the velocity, with which it was proved before, in the case of the parabola (Theor. X, Book I), the descent would be made in a non-resisting medium, in the subduplicate ratio of unity to the number two. And the times of the descent will be here reciprocally as the velocities, and therefore given.

Cor. 5. And because at equal distances from the centre the velocity is the same in the spiral PQR as it is in the right line SP, and the length of the spiral is to the length of the right line PS in a given ratio, namely, in the ratio of OP to OS; the time of the descent in the spiral will be to the time of the descent in the right line SP in the same given ratio, and therefore given.
Cor. 6. If from the centre S, with any two given intervals, two circles are described; and these circles remaining, the angle which the spiral makes with the radius PS be any how changed; the number of revolutions which the body can complete in the space between the circumferences of those circles, going round in the spiral from one circumference to another, will be as, or as the tangent of the angle which the spiral makes with the radius PS; and the time of the same revolutions will be as, that is, as the secant of the same angle, or reciprocally as the density of the medium.
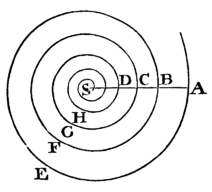
Cor. 7. If a body, in a medium whose density is reciprocally as the distances of places from the centre, revolves in any curve AEB about that centre, and cuts the first radius AS in the same angle in B as it did before in A, and that with a velocity that shall be to its first velocity in A reciprocally in a subduplicate ratio of the distances from the centre (that is, as AS to a mean proportional between AS and BS) that body will continue to describe innumerable similar revolutions BFC, CGD, &c., and by its intersections will distinguish the radius AS into parts AS, BS, CS, DS, &c., that are continually proportional. But the times of the revolutions will be as the perimeters of the orbits AEB, BFC, CGD, &c., directly, and the velocities at the beginnings A, B, C of those orbits inversely; that is as, ,. And the whole time in which the body will arrive at the centre, will be to the time of the first revolution as the sum of all the continued proportionals, , , going on ad infinitum, to the first term; that is, as the first term to the difference of the two first, or as ⅔AS to AB very nearly. Whence the whole time may be easily found.
Cor. 8. From hence also may be deduced, near enough, the motions of bodies in mediums whose density is either uniform, or observes any other assigned law. From the centre S, with intervals SA, SB, SC, &c., continually proportional, describe as many circles; and suppose the time of the revolutions between the perimeters of any two of those circles, in the medium whereof we treated, to be to the time of the revolutions between the same in the medium proposed as the mean density of the proposed medium between those circles to the mean density of the medium whereof we treated, between the same circles, nearly: and that the secant of the angle in which the spiral above determined, in the medium whereof we treated, cuts the radius AS, is in the same ratio to the secant of the angle in which the new spiral, in the proposed medium, cuts the same radius: and also that the number of all the revolutions between the same two circles is nearly as the tangents of those angles. If this be done every where between every two circles, the motion will be continued through all the circles. And by this means one may without difficulty conceive at what rate and in what time bodies ought to revolve in any regular medium.
Cor. 9. And although these motions becoming eccentrical should be performed in spirals approaching to an oval figure, yet, conceiving the several revolutions of those spirals to be at the same distances from each other, and to approach to the centre by the same degrees as the spiral above described, we may also understand how the motions of bodies may be performed in spirals of that kind.
PROPOSITION XVI. THEOREM XIII.
If the density of the medium in each of the places be reciprocally as the distance of the places from the immoveable centre, and the centripetal force be reciprocally as any power of the same distance, I say, that the body may revolve in a spiral intersecting all the radii drawn from that centre in a given angle.

This is demonstrated in the same manner as the foregoing Proposition. For if the centripetal force in P be reciprocally as any power SPn+1 of the distance SP whose index is n + 1; it will be collected, as above, that the time in which the body describes any arc PQ, will be as PQ, PS½n; and the resistance in P as, or as, and therefore as, that is (because is a given quantity), reciprocally as SPn+1. And therefore, since the velocity is reciprocally as SP½n, the density in P will be reciprocally as SP.
Cor. 1. The resistance is to the centripetal force as to OP.
Cor. 2. If the centripetal force be reciprocally as SP³, 1 - ½n will be = 0; and therefore the resistance and density of the medium will be nothing, as in Prop. IX, Book I.
Cor. 3. If the centripetal force be reciprocally as any power of the radius SP, whose index is greater than the number 3, the affirmative resistance will be changed into a negative.
SCHOLIUM.
This Proposition and the former, which relate to mediums of unequal density, are to be understood of the motion of bodies that are so small, that the greater density of the medium on one side of the body above that on the other is not to be considered. I suppose also the resistance, caeteris paribus, to be proportional to its density. Whence, in mediums whose force of resistance is not as the density, the density must be so much augmented or diminished, that either the excess of the resistance may be taken away, or the defect supplied.
PROPOSITION XVII. PROBLEM IV.
To find the centripetal force and the resisting force of the medium, by which a body, the law of the velocity being given, shall revolve in a given spiral.
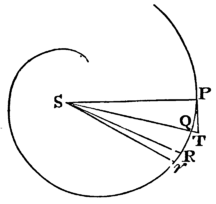
Let that spiral be PQR. From the velocity, with which the body goes over the very small arc PQ, the time will be given; and from the altitude TQ, which is as the centripetal force, and the square of the time, that force will be given. Then from the difference RSr of the areas PSQ and QSR described in equal particles of time, the retardation of the body will be given; and from the retardation will be found the resisting force and density of the medium.
PROPOSITION XVIII. PROBLEM V.
The law of centripetal force being given, to find the density of the medium in each of the places thereof, by which a body may describe a given spiral.
From the centripetal force the velocity in each place must be found; then from the retardation of the velocity the density of the medium is found, as in the foregoing Proposition.
But I have explained the method of managing these Problems in the tenth Proposition and second Lemma of this Book; and will no longer detain the reader in these perplexed disquisitions. I shall now add some things relating to the forces of progressive bodies, and to the density and resistance of those mediums in which the motions hitherto treated of, and those akin to them, are performed.