CHAPTER 9
A Few Notes about Notes
We draw the emotional content of music from melodies and harmonies that are, of course, sequences and combinations of notes. And to continue our discussion of music, we now need to know a little more about notes themselves. This short chapter will explain the basics of what a note is and—most important—the whys and wherefores of the octave. Once we’ve covered this ground, we can move on to discuss the ins and outs of how our brain responds to melodies and harmonies and why we have a natural aversion to dissonance.
First of all, what is a note?
Your eardrums are like tiny, highly sensitive trampolines that react quickly to changes in air pressure. Unless you are in a completely silent environment, the air near your ears is continuously flickering up and down in pressure hundreds or thousands of times every second. As long as these rippling pressure changes happen more frequently than twenty times a second and less frequently than twenty thousand times a second, we experience them as sound.* Whenever the pressure rises, your eardrum is pushed inwards, and when the pressure drops, the eardrum is flexed outwards a little.
When you hear non-musical sounds, like the scrunching up of a paper bag, there is no pattern to the pressure ripples involved. The movement of the paper while being scrunched produces a very complex selection of pressure waves that flow across the room to your ear like ripples across the surface of a pond. When these pressure ripples reach your ear, they move the eardrum in an equally complex way. Eventually your brain compares this noise to its library of often heard noises and comes to the conclusion “Ah, something made of paper is undergoing a thorough scrunching somewhere nearby.”
When you hear a musical note, however, there is a repeating pattern to the pushes and pulls on your eardrum. If you hit a piano key, twang a guitar string, or blow into a saxophone, you set up a trembling vibration in the instrument and the air around it. (You can feel this tremble if you touch any instrument when a note is playing.) The regular to-and-fro vibration of the instrument produces a pattern of repeating ripples of pressure in the air that push and pull on your eardrum. The pitch of the note you hear will be determined by how frequently the cycle of vibration takes place. For example, the lowest note on a piano will push your eardrum in and out fifty-five times every two seconds, and the highest note on a piano will do so at a rate of over four thousand times a second.
We get the frequency of a note from how frequently it pushes your eardrum in and out. If a note flexes your eardrum in and out in a repeating cycle 440 times a second, we say it has a frequency of 440 cycles per second. Generally the phrase “cycles per second” is replaced by the word “hertz” in honor of the German scientist Heinrich Hertz. And “hertz” is usually written as the abbreviation “Hz.” So the note that pushes your eardrum in and out 440 times a second has a frequency of 440 Hz.
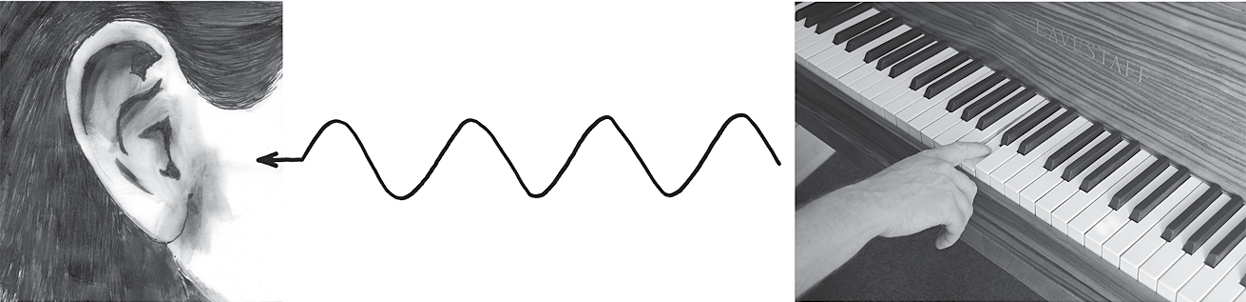
Ripples of pressure travel through the air from a vibrating musical instrument. When the ripples reach your ear, they push and pull on your eardrum in a rapid repeating pattern and you hear a musical note.
In the previous image I’ve drawn a very smooth repeating pattern of up-and-down pressure, but the ripples from a real instrument are usually a lot lumpier than this. For example, here are typical repeating pressure patterns from a flute, an oboe, and a violin, all playing the same note.* These traces are taken from recordings of the three instruments, and the up-and-down fluctuations of the pressure they produce are described by the rising and falling of the traced line.
| flute | 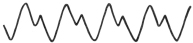 |
| oboe | 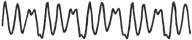 |
| violin |  |
A musical note can have almost any pressure ripple pattern as long as that pattern repeats itself over and over again. As you can see, the repeating pattern for the flute is a fairly smooth double bump followed by a valley, whereas the oboe pattern fluctuates a lot more and involves two bumps followed by a double bump followed by a valley. This increase in complexity in the repeating pressure pattern is reflected in the different timbres of the two instruments. A flute note sounds pure and simple, and the oboe has a richer, more complicated timbre. All the notes I’ve drawn here have the same pitch because each pattern takes the same amount of time to repeat itself. (In each case I’ve drawn about four and a half cycles of the pattern.) For the note involved here, your eardrum would be flexing in and out to these complex but repeating cycles of movement 440 times a second.
While we’re on the subject of timbre, it’s worth bearing in mind that the timbre of musical instruments is not the same over their whole range. For example, a clarinet sounds rich and warm for low notes and much edgier for high notes. So the pressure patterns for any instrument are different shapes for different notes.
From now on I’ll just use drawings of the simple up-and-down repeating pattern we saw first. But please bear in mind that real notes from actual instruments have far more complicated patterns.
When we listen to an unaccompanied melody, we hear notes of different pitch in a sequence. Higher-pitched notes have more pressure cycles per second.

I’ll be discussing melodies in the next chapter, but for the moment I want to talk about what happens when you play notes simultaneously. To make things as simple as possible, we’ll just look at the situation in which only two notes are involved.
Obviously, if you play two different notes at the same time, one of them will have a quicker up-down pattern than the other, so the ear is kept busy trying to respond to them both simultaneously. Depending on the notes involved, the resulting sound can be calm and relaxing or rather tense and anxious. Within any piece of music the composer or songwriter might choose an anxious combination of notes before moving on to a calm, relaxing combination, which is followed by more anxiety. This cycle of tension and release in the harmony is one of the tools musicians use to manipulate our emotions.
If you listen carefully to two notes played at the same time, you can generally distinguish the two individual notes involved. You could, for example, hum the lower one and then the higher one.
But—and it’s a big but—something odd happens when we play any note together with the note an octave above it.
“And what,” you might ask, “is an octave?”
At the heart of this question is a phenomenon that has been discovered by every musical society in the world and is the cornerstone of all musical systems throughout history. It’s possibly the most important single piece of musical information there is.
It’s called octave equivalence.
Octave equivalence
Musicians are told early in their training that notes an octave apart sound very similar, even though there is a big distance between them in pitch. (The first two notes of “Somewhere Over the Rainbow” are an octave apart.) But if, when you are learning an instrument, you ask why two such distant notes have such a strong resemblance, the response will generally be a single sentence along the lines of “They sound similar because the upper note has twice the frequency of the lower one.”
Unless you are familiar with the concept of frequency, this answer is only marginally more informative than “That’s just the way it is—OK?”
Octave equivalence is the reason why there are eight A’s on a piano—and eight B’s, and so on. If you look at a piano keyboard, you can see that there is a repeating pattern of keys from left to right, and the notes are named in a repeating pattern as well. The note names go from A to G sharp (often written G#), and then the letter names repeat. (We’ll see why in a minute.)
To show which A or D or F we’re talking about, each note is given a little number after the letter, going from zero for the lowest note to 8 for the highest. So, for example, A4 has a higher pitch than C3.
If you take any note as your starting point, the next note up (to the right on a piano keyboard) that has the same letter name is an octave above it: A3 is an octave above A2, and D2 is an octave above D1, and so on. (Carrying on in the same vein, E4 is three octaves above E1, and B6 is four octaves above B2, etc.)

If you play two notes an octave apart one after the other, they sound oddly similar even though they are a long way apart in pitch. Also, if you play the lower of the two notes first and then add the higher “octave above” note to it, the two notes merge into each other seamlessly. If you try combining two notes that aren’t an octave apart in the same way, they don’t consume each other like this; they retain their own identities.
For those of you with memories as bad as mine, here is that drawing of the pressure ripples from a smoothly timbred musical instrument traveling into a listener’s ear again. (I don’t know about you but I find it irritating when authors ask you to flick back a few pages to find an illustration.)
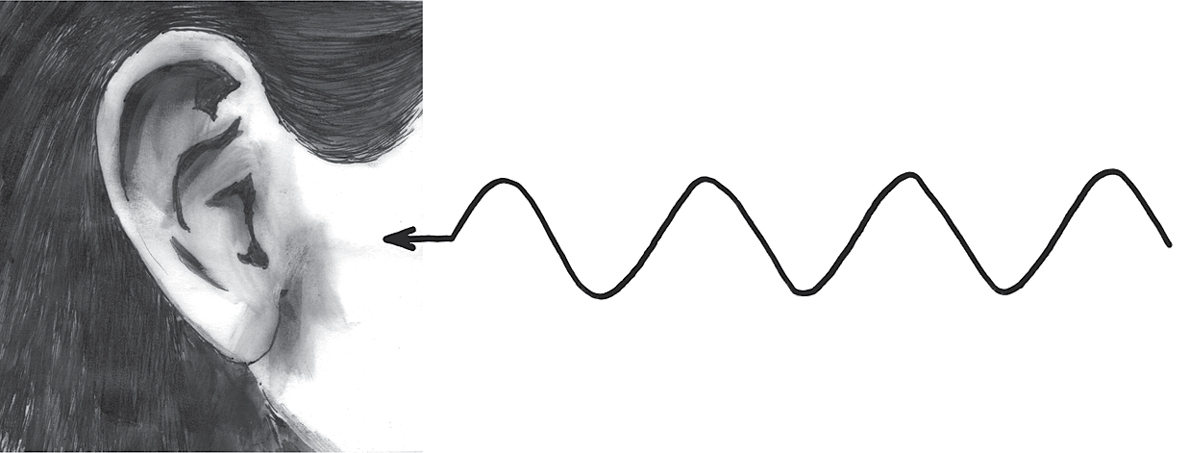
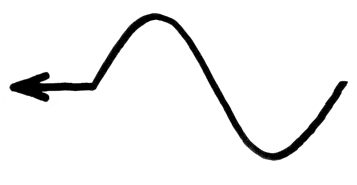
For our discussion of octave equivalence we are going to simplify things and look only at what happens during a single cycle of up-and-down pressure. Like this:
In real life you’d never experience this, but for our discussion a single cycle is useful because a real note is made up of a continuous stream of these cycles, so we need to discuss only one of them.
It takes a certain amount of time for our cycle to do its job on the eardrum. Let’s assume that you are in a quiet room and this cycle is the first part of a note that is just about to arrive at your ear. At this point your eardrum is in its relaxed, flat state and the air pressure outside the ear is “normal.” Now the pressure cycle arrives. Initially the pressure rises, so your eardrum is pushed inwards. After a short time the maximum pressure is reached and your eardrum starts to relax again as the pressure falls. But then the pressure continues to fall until it’s below normal, so your eardrum flexes outwards. After the minimum pressure is reached, the pressure starts to rise to a normal level again and your eardrum returns to its flat state. This cycle repeats and repeats and you experience a musical note.
Let’s introduce a bit of simple jargon here. The time needed for a complete single cycle of any note is called its cycle time. From a musical point of view, the most important thing about a note is its pitch, and the pitch of any note is directly related to its cycle time.
If the note is, for example, A2 (the note you get from the second-thickest string of a guitar), the cycle time will be nine one thousandths of a second,* or nine milliseconds. For higher-pitched notes the cycle time will be shorter, while lower-pitched notes have longer cycle times.
If we now play the note an octave above this (A3), we will find it has a cycle time of half that length: four and a half milliseconds.* It’s this halving of the cycle time that gives two notes an octave apart such a special relationship.
If we play one note and then add another that has half the cycle time of the first one, the timbre of what you hear will change—but not the cycle time of the experience. Two cycles of the higher note will fit nicely into one cycle of the lower one, so we still have an aural pattern that repeats at the cycle time of the slower cycle. And because the cycle time hasn’t changed, the pitch hasn’t changed either. Your ear doesn’t need to worry about coping with two notes with different up-down agendas. They’ve become fused together into one.
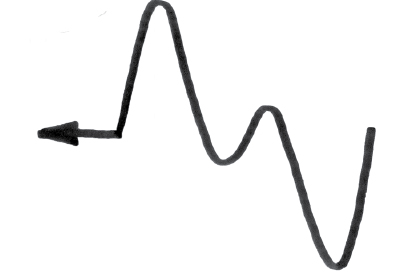
One cycle of our original note:
plus two cycles of our “octave above” note:

have combined to create one cycle of a slightly more complicated new sound with the same cycle time, and therefore the same pitch, as the original note.
Let’s look at several cycles together so we see the repeating pattern more clearly:

As far as our hearing system is concerned, the original note has swallowed the “octave above” note and merely become a more complicated personality in the process.
This phenomenon occurs with any two notes that are an octave apart. I happened to use A2 and A3 as my example, but I could have used D6 and D7 or F#4 and F#5, etc. Also, obviously, if it’s true for A2 and A3, it’s also true for A1 and A2, or A3 and A4, and so on. And this means that any A has a close family link with all the other A’s, which is why we let them all share the same letter name. The link between notes that are several octaves apart (e.g., A2 and A6) is weaker than it is for a single octave, in the same way that your great-grandmother is less connected to you than your mother. But the family connection is still strong; the A’s are a team, as are the B’s, and so on.
The way notes an octave apart fit perfectly into each other is also the reason why they sound oddly similar if they are played one after the other. Imagine your ear has just heard a note with this sort of pressure pattern:

If the note an octave above is played next, your brain has to work out if this new note has half the cycle time or whether it has the same cycle time but with a double bump in its pressure cycle:
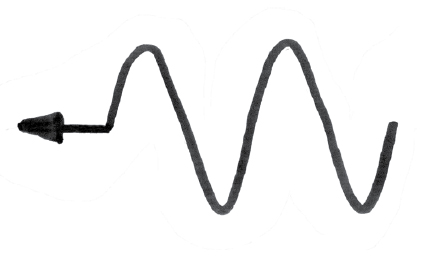
Your brain therefore has to make the choice as to whether this new note has a higher frequency or just a different timbre. This sort of confusion is possible only with notes an octave apart, and it’s the reason why such notes sound weirdly similar.
OK, so notes an octave apart are a closely related group—but why do we use the word “equivalence”?
Let’s say you are accompanying a singer on the piano. Your piano is in tune but some of the strings are broken, so one or two of the notes aren’t working. The songwriter has written down that the first chord of the song should be G major and, as is usual, he has chosen exactly which notes he wants for the chord: G4, B4, and D5.
But G4 is one of your silent notes. “Quelle horreur!” you might pretentiously whisper to yourself as your fingers hover over the keyboard. Well, get a grip—all is not lost. G3 or G2 or G5 will provide excellent replacements for your G4. The chord will sound a little different, but the choice of notes required for that chord depends more on the letters than the numbers involved. So notes an octave apart are said to be equivalent as far as harmonies are concerned.
This equivalence, however, doesn’t usually work for tunes in the way it does for chords. The replacement of a melody note with the note an octave above or below works on some occasions, but large hops like this tend to spoil the contour of the melody, and if you introduce several octave replacements in a tune, it rapidly becomes unrecognizable. Frequent octave hopping in the melody should generally be avoided unless you’re a jazz soloist who’s deliberately obscuring the tune in order to reveal it later (or as Kim would put it, “Unless you’re a jazz soloist who’s deliberately trying to make everyone’s life a misery”).
You may be wondering why, apart from the fact that notes an octave apart merge into each other and can sometimes replace each other, the octave is so important to our understanding of music.
Well, all musical systems around the world use notes that have different pitches. But how do we choose the sizes of the intervals, the jumps in pitch between the different notes? Wouldn’t it be convenient if there were a naturally occurring interval that gave us a definite structure to build our system on?
You guessed it: the octave is a naturally occurring interval. By “naturally occurring” I mean that it can be produced easily from inanimate objects. If you take a bottle, hold it to your lips, and blow across its neck, you will get a pleasant low whistling sound—a musical note. Blow a bit harder and you will produce the note an octave above. If you are a prehistoric hunter twanging on the string of your bow, you will find that when you touch the vibrating string at its center point, it gives you the note an octave above its usual twang.
The octave is the basic building block of all musical systems, but it’s a big interval; an untrained singing voice has a range of only about two octaves. So musical societies throughout history have devised ways of dividing the interval up into several smaller intervals. In the case of Western music, we eventually decided to divide the octave up into twelve equal steps and choose to use a selection, or key, of about seven of them at any one time (although we can change from one selection to another during the course of a piece of music). Classical Chinese music has opted for slightly different steps, with only five notes in any key.
But whatever the system involved, it’s going to be based on the octave.