FIDDLY DETAILS E

Scales and Keys

I’ll begin this section by taking another look at how we choose the notes for a major scale using the “Just” system. As I explained in chapter twelve, we begin with only two notes: the keynote and the note an octave above it.

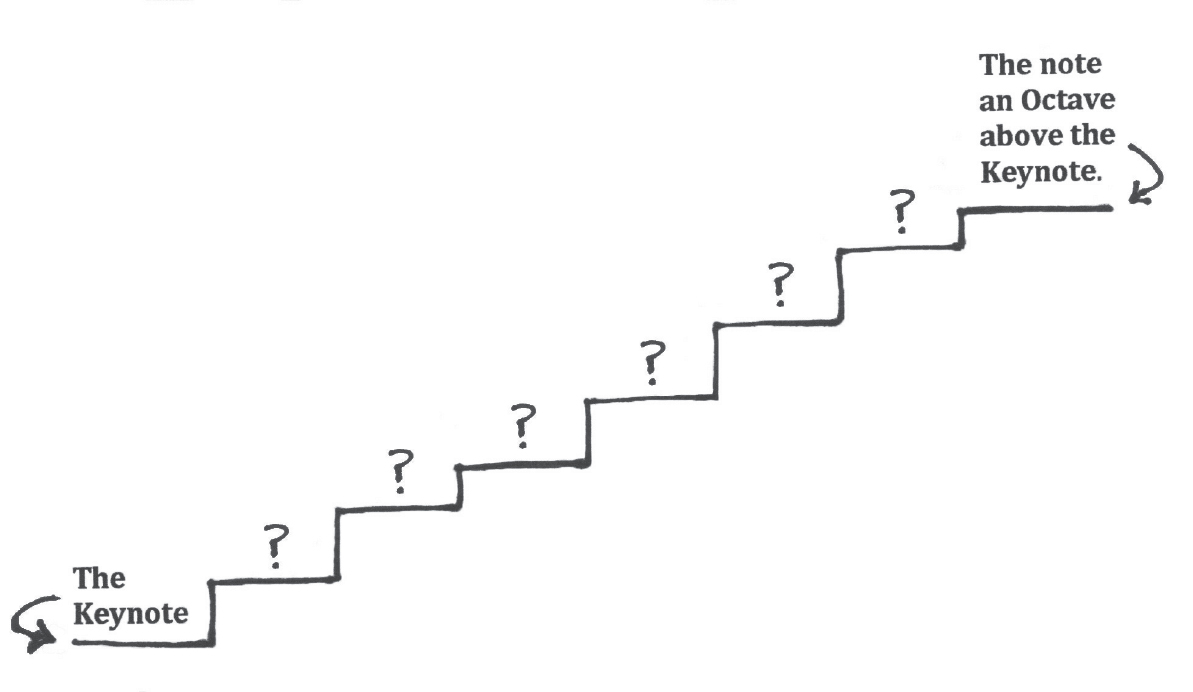
These two notes are in tune with each other because two cycles of the “octave above” note fit exactly into one cycle of the keynote. Another way of saying this is that these two notes are in tune because two halves equal one.

Continuing this line of thinking, it doesn’t take long to realize that three thirds are also equal to one. Surely a note that has one third of the keynote’s cycle time would also be nicely in tune with the keynote. Wouldn’t three of its cycles fit perfectly into the keynote cycle to give us a match that was almost as good as an octave?

This is all very true, but now we have a problem. This new note is even higher in pitch than our “octave above” note. For our scale we need notes that fit inside our octave, and this one is too high.

Happily, octave equivalence comes to the rescue. We know that any two notes an octave apart are linked closely enough to make the note an octave below this “too high” note work pretty well. And so it does. The cycle time of this note is twice one third—that is, two thirds (⅔)—of the keynote cycle time.

Although one cycle of our keynote doesn’t match one, two, or three cycles of our new note, we get the next-best thing: two cycles of the keynote match three cycles of the new note, producing a nicely repeating pattern. Because the repeat is spread over two cycles of the keynote, it’s not quite as strong a link as the octave coupling I discussed earlier, but we still hear it as a very pleasant combination.

Using the same logic, if we look at the note that has ¾ of the keynote cycle time, we can see that four of its cycles fit exactly into three of the keynote cycles. And sure enough, this does make another nice-sounding pairing.

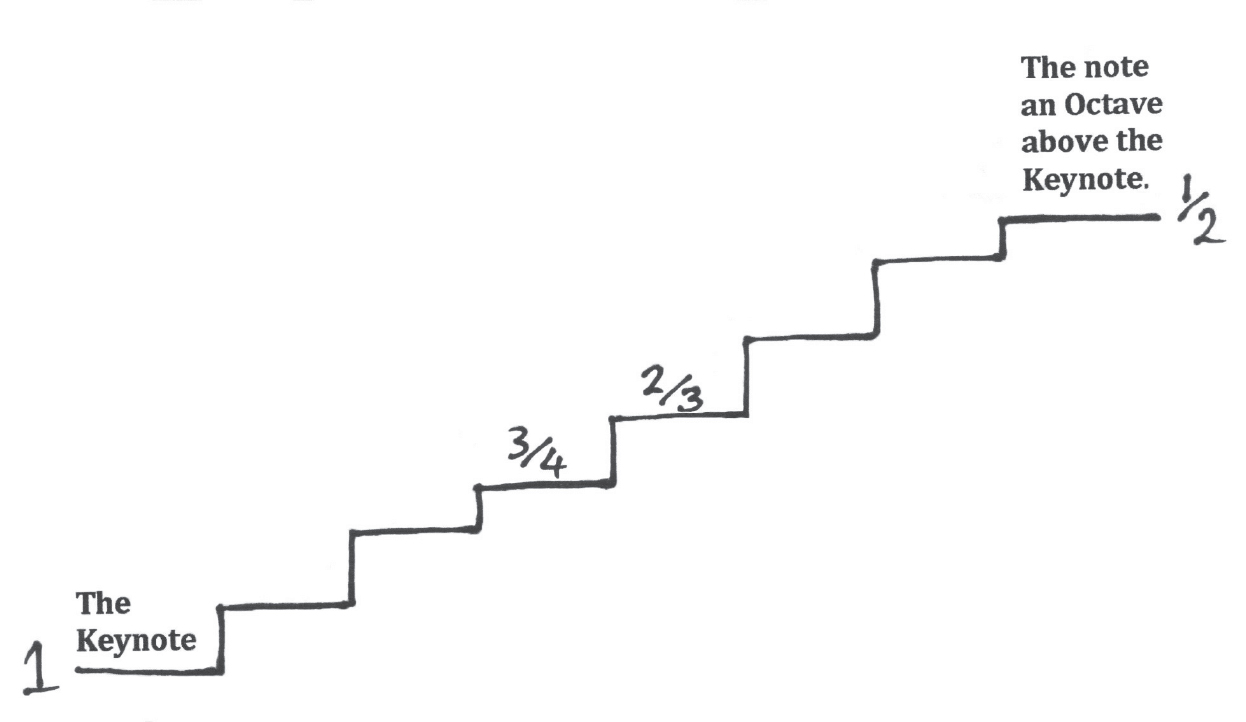
We now have four notes in our octave and they have the following cycle times:

• the keynote cycle time (the keynote)

• ¾ of the keynote cycle time

• ⅔ of the keynote cycle time

• ½ the keynote cycle time (an octave above the keynote)

So it’s becoming clear that simple fractions of the keynote cycle time might be the answer to finding notes that are in tune with the keynote. In fact we can now come up with a definition of the term “in tune”:

If a few cycle times of one note fit exactly into a few cycle times of another note, the two notes are in tune with each other.

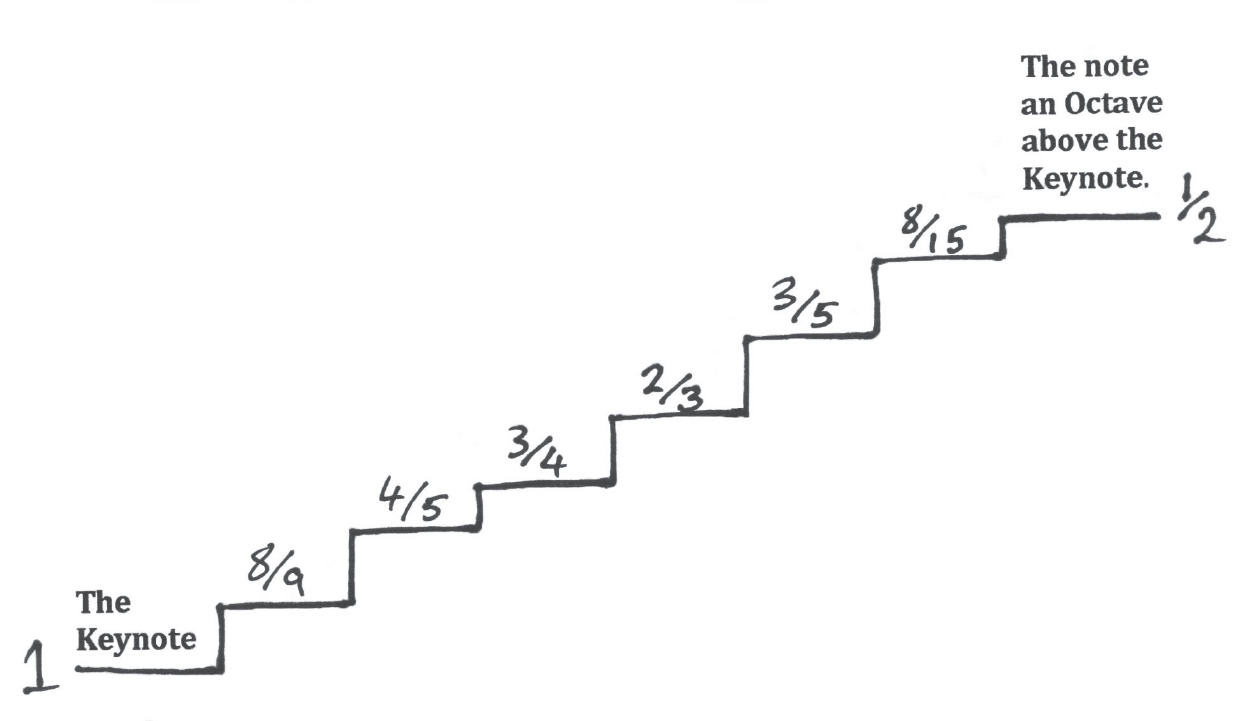
And that’s how we find all the other notes in our scale. By using simple fractions, we can create a major scale of eight notes that are all as in tune with the keynote as they can be. Here are the fractions of the keynote cycle time for them all, starting with the keynote:

Note 1, the keynote, obviously has the cycle time of the keynote.

Note 2 has a cycle time that is of the keynote cycle time.

Note 3 has a cycle time that is of the keynote cycle time.

Note 4 has a cycle time that is ¾ of the keynote cycle time.

Note 5 has a cycle time that is ⅔ of the keynote cycle time.

Note 6 has a cycle time that is 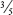of the keynote cycle time.

Note 7 has a cycle time that is 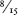of the keynote cycle time.

Note 8 has a cycle time that is ½ the keynote cycle time (the “octave above” note).

If you play any of these notes at the same time as the keynote, they set up a repeating pattern like the ones I drew earlier. The number above the line in each fraction is the number of keynote cycles it takes before the cycle repeats in each case.

This system of choosing notes from simple fractions creates what is called a Just scale. (It’s called “Just” because in the past the word “just” meant “correct” or “true.”)

Clearly some of these fractions are simpler than others. When we listen to any of these notes played at the same time as the keynote, we find that the smoothest combinations come from the fractions with the lowest numbers above the fraction line. Our hearing system finds combinations that repeat in less than four or five keynote cycles pleasant, and the lower the number, the smoother the sound. Played at the same time as the keynote, the ½ note sounds smoother than ⅔, which sounds smoother than ¾.

But two of the notes in our scale don’t sound smooth at all when they are played at the same time as the keynote. As you might expect, these are the ones with the biggest numbers above the line in their fractions: and 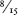. In each case we have to wait eight cycles of the keynote before the pattern repeats. Our hearing system struggles to make sense of this, and we hear the combination of the two sounds as rough rather than smooth.

Even though two of the notes in our scale sound rough if they are played at the same time as our keynote, they are in tune with it, because a few of their cycles fit exactly into a few cycles of the keynote.

You might wonder why we even bother having a couple of notes in the group that, although they are in tune with the keynote, sound rough when they are played alongside it. The reason is that without these notes there would be a big gap in our scale on either side of the keynote. In order to have small, easily singable steps in our scale, we need to fill in these gaps, so we use the most “in tune” notes we can get to do so.

Problems with the Just scale

On the face of it, the Just scale is an excellent way of dividing up the octave to provide us with a usable number of notes. We have a keynote, the note an octave above, and six notes between them that are in tune with the keynote. Hurrah!

Ah, if only life (and music) were that simple…

Notes 1 (the keynote) and 5 sound great together because the cycle time of note 5 is exactly two thirds of the cycle time of note 1.

Notes 2 and 6 should share the same relationship—but they don’t. You might suspect something is different from the fractions involved: the relationship between 1 and ⅔ (notes 1 and 5) is very simple, but the relationship between and 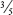(notes 2 and 6) is obviously more complicated.

Three cycles of note 6 last for a slightly longer time than two cycles of note 2. The difference isn’t much, but it’s enough to mean that, instead of two cycles of the lower note fitting into three cycles of the upper one, we need twenty-seven cycles of the lower note before we get an exact fit.

In this case forty cycles of the higher note fit precisely into twenty-seven cycles of the lower one, but the combined pattern takes so long to repeat that our hearing system assumes that the notes involved don’t fit together well enough to pass the “in tune” test. You may remember that to be “in tune,” a few cycle times of one note need to fit exactly into a few cycle times of another note. Well, twenty-seven is too high a number to be described as “a few,” so this combination sounds out of tune.

This is the problem with Just scales and keys: the simple fractions ensure that the notes are all in tune with the keynote, but some of the other notes can be out of tune with one another.*

The Just scale has other intrinsic problems if you use it to tune a piano and you’d like to be able to change key occasionally (which most Western music does). You can’t tune a piano (or any other keyboard instrument) to the Just system without some of the chords being out of tune. This is the reason why we use a different tuning system, called equal temperament, for tuning fixed-note instruments.

Equal temperament

Equal temperament (ET) doesn’t use fractions to decide on the cycle times or frequencies of the notes in the scale; it uses a mathematical formula to make all the semitone jumps exactly the same size.

The ET system was worked out independently by the Chinese scholar Chu Tsai-Yü in 1580 and by Galileo’s father, Vincenzo Galilei, in 1581. The system wasn’t commonly used until the late 1700s, though, and the British piano firm Broadwood didn’t change over to ET until 1846.

Galilei and Chu Tsai-Yü found that calculating the equal temperament system is pretty easy once you have presented the problem clearly and logically:

1. A note an octave above another must have twice the frequency of the lower one.

2. The octave must be divided up into twelve semitone steps.

3. All the twelve steps must be equal. (If you take any two notes one step apart, then the frequency ratio between them must always be the same.)

To achieve these aims our two wise men calculated that we needed an increase in frequency of 5.9463 percent between two notes which are a semitone apart.* This means that most of the notes in our scale are slightly higher or lower in pitch than they would be in the Just system.

Let’s have a look at the difference between a Just and an equal temperament A major chord (which is made up of the three notes A, C sharp, and E). Both the Just and the ET systems use the 440Hz A as their starting point, but the frequencies of the other notes are slightly different for the two systems. For the Just system, the frequency of the E above our A will be exactly 1½ times 440, which is 660 Hz. In the ET system, however, this has been adjusted a bit to 659.2 Hz. The C sharp has been adjusted a larger amount, from 550 Hz up to 554.4.

These differences mean that a Just A major chord sounds very smooth but an ET major chord sounds only moderately smooth. There is some compensation for this, however, because some of the other chords in the Just system sound definitely out of tune, whereas all the chords in the ET system have the same level of moderate smoothness.

Equal temperament has been a source of heated arguments among musicians for over two hundred years. There is an excellent book on the subject by musicologist Ross W. Duffin called How Equal Temperament Ruined Harmony (and Why You Should Care), so I think we all know which side of the fence he’s on. Yet a lot of the music you’ve ever heard, particularly solo keyboard music, has been performed using equal temperament tuning, so it’s clearly a very successful compromise.

Equal temperament has been by far the most popular tuning system for fixed-note Western musical instruments since about 1850, although there are some enthusiasts who still prefer to use various methods of adjusting the Just system to make it work more evenly. If you’re interested, have a look at “musical temperament” on YouTube, where you’ll find quite a few videos of people comparing various different tuning systems.

But, as I discussed at the end of chapter twelve, although equal temperament is the tuning system we use for fixed-note instruments like keyboards, musical groups that involve only variable-pitch instruments (singers, violinists, etc.) often use Just tuning—particularly for slow, harmonized pieces. Skilled performers on variable-pitch instruments can alter the pitch of the notes they play to compensate for the inherent problems of the Just system and produce purer harmonies than the ones produced by equal temperament.