In this chapter, you learn about ratios and proportions.
Ratio Concepts
A ratio is a comparison by division of two quantities having the same units. Suppose the lengths of the radii of two circles are in the ratio of 3 to 5. If the radius of the first circle is 3 in, then the radius of the second circle is 5 in. That is, the radius of the first circle is 
as long as the radius of the second circle. Ratios can be expressed in several different forms: 3 to 5, 3 : 5, 3 5, , 0.6, or 60%. The context of the discussion at the time usually dictates the form preferred. A ratio is a pure number—it has no units. The units “cancel” out as in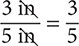 
. Fractions, percents, and decimals are ratios. If the items compared have units that cannot be “canceled” such as 
, then the comparison is called a rate or a scale.
Proportion Concepts
The mathematical statement that two ratios (or rates or scales) are equal is a proportion. The statement 
is a proportion and is commonly read as “2 is to 3 as 4 is to 6.” This can also be written as 2 : 3 = 4 : 6, although for computations the fractional form is necessary. The fundamental property of proportions is that 
if and only if ad = bc. The products ad and bc are the cross products.
Proportions and proportional thinking pervade such diverse topics as medicine dosage, application of fertilizer to a lawn, and oil additives in gasoline for outboard motors. Consequently, you should master the basics presented here to prepare for any real-world applications you might encounter.
Solving Proportions
If the values of three of the four terms of a proportion are known, then the value of the fourth term can be determined by using the fundamental property of proportions.
Problem
Set up a proportion and solve for the unknown term.
a. A number n compared to 45 is the same as 7 compared to 15. Find n.
b. 38 is to 640 as 152 is to the number n. Find n.
Solution
a. A number n compared to 45 is the same as 7 compared to 15. Find n.
Step 1. Write a proportion.
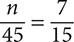
Step 2. Solve the proportion.
Find a cross product you can calculate.
7 × 45
Divide by the numerical term you didn’t use.
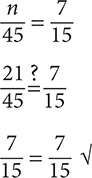
b. 38 is to 640 as 152 is to the number n. Find n.
Step 1. Write a proportion.
Step 2. Solve the proportion.
Find a cross product you can calculate.
640 × 152
Divide by the numerical term you didn’t use.
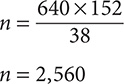
Step 3. Check.
Note: The fraction on the left initially could have been reduced.
Solving Application Problems Involving Proportions
When you have an application problem involving proportions, look for a sentence or phrase in the problem that provides the information you need for the left portion of the proportion, and then look for another sentence or phrase that gives you the information you need for the right portion of the proportion.
On a map, the distance between two cities is 13.5 in. On the map scale 0.5 in represents 20 mi. How far is it, in miles, between the two cities?
Solution
This problem is a proportion problem involving a map scale. Information for the left portion of the proportion is in the first sentence of the problem, and information for the right portion of the proportion is in the second sentence.
Step 1. Use the first sentence to write the left portion of the proportion.
Let d be the unknown distance. Then d corresponds to 13.5 in:
Step 2. Use the second sentence to write the right portion of the proportion. 0.5 in corresponds to 20 mi: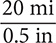 
Step 3. Write the proportion by setting the left portion equal to the right portion.
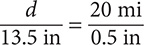
Notice that the units in the left portion match up with the units in the right portion.
Step 4. Solve the proportion.
Find a cross product you can calculate.
13.5in × 20mi
Divide by the numerical term you didn’t use.
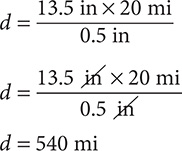
Notice that the units work out to be miles, which is what you should expect because d is a distance.
Problem
If 25 ft of wire weighs 3 lb, what is the weight of 8 ft of this wire?
Solution
This problem is a proportion problem involving lengths and weights. Information for the left portion of the proportion is in the first part of the problem question, and information for the right portion of the proportion is in the second part of the problem question.
Step 1. Use the first part of the problem question to write the left portion of the proportion.
25 ft of wire corresponds to 3 lb: 
Step 2. Use the second part of the problem question to write the right portion of the proportion.
Let w be the unknown weight. Then 8 ft corresponds to w: 
.
Step 3. Write the proportion by setting the left portion equal to the right portion.
Notice that the units in the left portion match up with the units in the right portion.
Step 4. Solve the proportion.
Find a cross product you can calculate.
(3lb)(8 ft)
Divide by the numerical term you didn’t use.
Notice that the units work out to be pounds, which is what you should expect because w is a weight.
Step 5. Check.
Problem
The ratio of a propeller rate to that of the engine is 2 : 3. If the engine is turning at a rate of 4,800 revolutions per minute (rpm), what is the propeller rate?
Solution
This is a proportion problem involving two ratios. The first ratio is in the first sentence, and the second ratio is in the question. Let R be the unknown propeller rate and compare the ratios.
Step 1. Write a proportion.
Step 2. Solve the proportion
Find a cross product you can calculate.
(2)(4,800 rpm)
Divide by the numerical term you didn’t use.
Step 3. Check.
Using Proportions to Solve Percent Problems
Percent problems can be solved using a “percent proportion,” which has the following form:
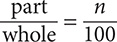
The relationship among the three elements n, part, and whole can be explained in a percent statement like this:
The part is n% of the whole.
The secret to solving percent problems is being able to identify the three elements correctly. Start with n and the whole because they are usually easier to find. The part will be the other amount in the problem. The value of two of the elements will be given in the problem, and you will be solving for the third element. After you identify the three elements, substitute the two you know into the percent proportion and solve for the one that you don’t know.
Problem
Use a percent proportion to solve the given percent problem.
a. You inherited 15% of $9,000. How much money did you inherit?
b. A student scored 60 out of 80 questions. What is the student’s percent grade?
c. A toy is on sale for $13. This sale price is 80% of the regular price of the toy. What is the regular price?
d. What is 30% of 140?
e. Twenty-five is 40% of what number?
f. Twenty-seven out of 40 is what percent?
Solution
a. You inherited 15% of $9,000. How much money did you inherit?
Step 1. Write the percent proportion.
Step 2. Set up the percent proportion using $9,000 as the whole and 15 as n. Let p be the part inherited.
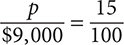
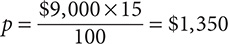
Step 4. Check.
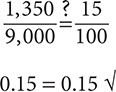
Step 5. Answer the question.
$1,350 is the amount inherited.
b. A student scored 60 out of 80 questions. What is the student’s percent grade?
Step 1. Write the percent proportion.
Step 2. Set up the percent proportion using 80 as the whole and 60 as the part. Let n be the percent number.
Step 3. Solve the proportion.
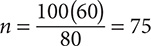
Thus, n% = 75%
Step 4. Check.
0.75 = 0.75 V
Step 5. Answer the question.
The percent score is 75%.
c. A toy is on sale for $13. This sale price is 80% of the regular price of the toy. What is the regular price?
Step 1. Write the percent proportion.
Step 2. Set up the percent proportion using the regular price as the whole and $13 as the part. Let w be the regular price.
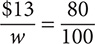
Step 3. Solve the proportion.
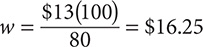
Step 4. Check.
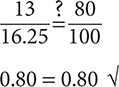
Step 5. Answer the question.
The regular price is $16.25.
d. What is 30% of 140?
Step 1. Write the percent proportion.
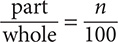
Step 2. Set up the percent proportion using 140 as the whole and 
as 30%. Let p be the part.
Step 3. Solve the proportion.
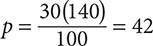
Step 5. Answer the question.
30% of 140 is 42.
e. Twenty-five is 40% of what number?
Step 1. Write the percent proportion.
Step 2. Set up the percent proportion using w as the whole, 
as 40%, and 25 as the part.
Step 3. Solve the proportion.
Step 4. Check.
Step 5. Answer the question.
25 is 40% of 62.5.
f. Twenty-seven out of 40 is what percent?
Step 1. Write the percent proportion.
Step 2. Set up the percent proportion using 40 as the whole and 27 as the part. Let n be the percent number.
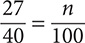
Step 3. Solve the proportion.
Step 4. Check.
Step 5. Answer the question.
27 out of 40 is 67.5%.
Exercise 9
1. If 14 oz of salt is mixed with 5 oz of pepper, what is the ratio of salt to pepper?
2. Solve the proportion 
for n.
3. In a paint mixture that uses 2 parts of white paint to 5 parts of blue paint, how many quarts of white paint are needed to mix with 20 quarts of blue paint?
4. A stake 10 ft high casts a shadow 8 ft long at the same time that a tree casts a shadow 60 ft long. What is the height of the tree?
5. If 7 g of iron combines with 4 g of sulfur to form iron sulfide, how much sulfur will combine with 56 g of iron?
6. The tax on a property valued at $12,000 is $800. Assuming the tax rate is the same, what is the value of a property taxed at $1,100?
7. Ninety-two is 80% of what number?
8. What is 35% of 80?
9. You buy a $5,000 savings certificate that pays 4% simple annual interest. How much interest will you earn in 6 months?
10. Forty-five out of 120 is what percent?
11. If a jet plane can travel 4,500 km in 5 hr, how many kilometers can it travel in 25 min?
12. A map has a scale of 1 in = 15 mi. How much distance is represented by 18 in?
13. What percent of 7,580 is 454.8?
14. Calcium and chlorine combine in the weight ratio of 36:64. How much chlorine will combine with 5 g of calcium?