
Fig. 1 – The opening of ‘An Act for Providing a Publick Reward for such Person or Persons as shall Discover the Longitude at Sea’ (the Longitude Act, 1714)
{Parliamentary Archives, London}
in the Judgment of Able Mathematicians and Navigators, several Methods have already been Discovered, true in Theory, though very Difficult in Practice ...
‘An Act for Providing a Publick Reward for such Person or Persons as shall Discover the Longitude at Sea’ (1714)1
Longitude, as a definable problem that could be separated out from the myriad risks and uncertainties of maritime travel, was of interest to theorists as well as practical navigators. It was, in fact, an issue that advocates of natural and experimental philosophy – what we today call science – latched onto as one for which their approach might be particularly successful. It was clear that finding a solution would be a propaganda coup for the new scientific institutions. It would be irrefutable proof that experimental, observational and mathematical methods, overseen by gentleman philosophers, could be applied to practical issues of importance to national interests.
Within Britain, maritime matters had been a significant focus for the Royal Society of London. That these included tackling longitude was a matter for satire, as in this anonymous poem, ‘In praise of the Choyce company of Philosophers and witts who meet on Wednesdays weekley, at Gresham Colledge’, written in 1661:
The Colledge will the whole world measure,
Which most impossible conclude,
And Navigation make a pleasure
By finding out the longitude.
Every Tarpalling shall then with ease
Sayle any ships to th’ Antipodes.2
Fellows of the Royal Society were to play a significant role in the passing of the Longitude Act by the British Parliament in 1714, which was itself to transform the relationship between scientific expertise and the state. It was, however, just the latest in a long line of initiatives to reward anyone able to arrive at workable solutions to the problem.
Longitude rewards
Rulers and states with maritime ambitions who were convinced that the longitude problem could be solved looked for ways to hasten solutions. Their conviction was, it seems, more often the result of lobbying by those likely to gain from financial incentives than a response to calls for assistance from practical seamen. The first such incentive scheme was established in Spain, the leading maritime power of the sixteenth century, by Philip II in 1567. This was followed in 1598 by Philip III’s offer of a reward of 6000 ducats, plus 2000 a year for life – some sixty times the annual income of a labourer – and 1000 towards expenses. The large reward was never paid out but several promising inventions were recognized by the repayment of expenses.
The life-changing size of the rewards on offer, and the fact that workable and complete solutions to the problem were clearly not appearing, led to the whole enterprise being satirized. Cervantes, author of Don Quixote, was one among many over the centuries to mock those who were mad enough to attempt such an impossible task, or who drove themselves mad in its pursuit. He wrote in 1613 of a mathematician who found fixing the longitude like chasing a will-o’-the-wisp:
Two and twenty years I have been employed in finding out the longitude ... and imagining oftentimes, that I have found it, and that it cannot possibly escape me, when I do not in the least suspect it, I find myself as far to seek as ever, which fills me with surprise and astonishment: it is the same with the square of the circle, which I have come so nigh finding out, that I know not, nor can imagine why I have it not at this time in my pocket ...3
Finding the longitude – like squaring the circle, creating perpetual motion or predicting the future – was often presented as a fool’s errand. Yet the solutions were, in theory, well understood. What they needed were technical responses to the various challenges thrown up by imperfect astronomical tables and conditions at sea. The answer might, therefore, have seemed tantalizingly close. To nudge them forward, the States General and States of Holland and, less officially, a number of other governments and individuals followed the Spanish example by offering rewards for meeting these challenges. Serious and important ideas were submitted, trials were made and some money paid out.
The Dutch scheme began in 1600 and established a range of rewards, with large one-off sums, annuities and smaller sums for those with promising ideas ready for trial. The top rewards increased over time, with the States of Holland offering 3000 guilders in 1601 and 50,000 in 1738. These sums were sufficiently alluring to attract a steady flow of ideas. There were forty-six submissions between 1600 and 1775, judged by ad hoc committees of theoretical and practical experts, including surveyors, teachers of navigation and university professors. The Dutch East India Company took an interest in the process, reflecting the particular risks and rewards of their trade routes. As with the Spanish reward, a whole range of ideas and solutions was offered, and a number underwent sea trial.
England and France came relatively late to longitude research but their arrival coincided with a period of significant advances in astronomy and instrument-making. Government and royal interest in finding longitude solutions led in both nations to the patronage of individuals with plausible methods, to the theme being taken up by the learned academies – the Royal Society of London and French Académie des Sciences, founded in 1660 and 1666 respectively – and to the establishment of observatories in Paris in 1667 and at Greenwich in 1675.
In terms of both the legislation and the work it was meant to encourage, the passing of the British Longitude Act of 1714 was more a case of continuity than of change. It did, however, come at a time when key successes and initiatives relating to navigation and longitude determination were bearing fruit: several areas of research, with long and sometimes distinguished track records, were earmarked as most likely to lead to success. Given advances in these areas over the preceding decades, there was probably some confidence that at least partial success could be achieved in the not-too-distant future.
The Longitude Act of 1714
If legislators believed that success was imminent, they were encouraged in that view by lobbyists and scientific experts. Their call for a British scheme to echo the Spanish and Dutch was answered on 9 July 1714, when Parliament passed ‘An Act for Providing a Publick Reward for such Person or Persons as shall Discover the Longitude at Sea’. It provided
That the First Author or Authors, Discoverer or Discoverers of any such Method ... shall be Entitled to ... a Reward, or Sum of ten thousand Pounds, if it Determines the said Longitude to One Degree of a great Circle, or Sixty Geographical Miles; to fifteen thousand Pounds, if it Determines the same to Two Thirds of that Distance; and to Twenty thousand Pounds, if it Determines the same to One half of the same Distance ...4
The value of this reward, in today’s terms, depends on the yardstick. £20,000 in 1710 can be calculated as worth over £1.5 million early in the twenty-first century, or as a sum that would have paid the annual wages of more than 600 craftsmen in the building trade or bought nearly 5000 cows.5 By any measure it was a large amount of money but the Act asked for a great deal: the successful method was to be ‘Tried and found Practicable and Useful at Sea’.
The emphasis on practical utility was underlined by the provision that half of the reward could be given to a method that ensured ‘the Security of Ships within Eighty Geographical Miles of the Shores, which are the Places of the greatest Danger’. However, the full reward was dependent on a successful long-distance sea trial:

Fig. 1 – The opening of ‘An Act for Providing a Publick Reward for such Person or Persons as shall Discover the Longitude at Sea’ (the Longitude Act, 1714)
{Parliamentary Archives, London}

Fig. 2 – Isaac Newton, by Charles Jervas, 1717
{The Royal Society}
when a Ship by the Appointment of the said Commissioners, or the major part of them, shall thereby actually Sail over the Ocean, from Great Britain to any such Port in the West-Indies, as those Commissioners, or the major part of them, shall Choose or Nominate for the Experiment, without Losing their Longitude beyond the Limits before mentioned.
The Act also allowed for the advancement of sums up to £2000 ‘to make Experiment’ of promising schemes. This appears to have meant costs associated with the trial but was later interpreted more broadly by the Commissioners. It was also possible to give rewards to schemes that failed to achieve the desired accuracy, but were nevertheless thought to be ‘of considerable Use to the Publick’.
Perhaps most importantly, the Act appointed the Commissioners of Longitude. They were the Lord High Admiral, or First Commissioner of the Admiralty; the Speaker of the House of Commons; the First Commissioner of the Navy; the First Commissioner of Trade; the Admirals of the Red, White and Blue Squadrons of the Navy; the Master of Trinity House; the President of the Royal Society; the Astronomer Royal; the Savilian, Lucasian and Plumian Professors of Mathematics (the first at Oxford University, the other two at Cambridge); and ten named Members of Parliament. They were not yet a standing body or a ‘Board’, but the Act was significant in creating a group, mostly selected because they held another relevant office, that combined political, navigational and scientific interests. While the President of the Royal Society and university professors had previously acted as advisors, this Act brought philosophers and mathematicians directly into a process of allocating government funds.
When action was being considered in June 1714, it was one of the future Commissioners, Isaac Newton, who presented evidence to a Committee of the House of Commons. He was then President of the Royal Society and, as such, an unofficial advisor to politicians on matters scientific (Fig. 2). He was also working directly on the mathematical and astronomical theories that had potential to help solve the problem. Newton’s written statement noted, in a phrase echoed in the legislation, that ‘there have been several Projects, true in theory but difficult to execute’. He provided a succinct summary of the methods and the problems still surrounding them:
One is by a Watch to keep Time exactly. But, by reason of the motion of a ship, the variation of heat & cold, & the difference of gravity in different Latitudes, such a Watch hath not yet been made.
Another is by the Eclipses of Jupiter’s Satellites. But by reason of the length of Telescopes requisite to observe them & the motion of a ship at sea, those Eclipses cannot yet be there observed.
A third is by the place of the Moon. But her Theory is not yet exact enough for this purpose. It is exact enough to determine her Longitude within two or three degrees, but not within a degree.
A Fourth is Mr Ditton’s project. And this is rather for keeping an account of the Longitude at sea than for finding it if at any time it should be lost, as it may easily be in cloudy weather ...6
The first three methods on this list were, to Newton, the most promising. All were a means of carrying or finding a reference time against which to compare observations of local time on board ship. If practicable, they would allow difference in longitude to be established anywhere in the world. The last method, ‘Mr Ditton’s project’, as Newton said, targeted particular circumstances rather than being a universal solution to the problem. It is, however, of particular interest to the story, being the cause of parliamentary interest in the issue of longitude in 1713.
‘Mr Ditton’s project’
The Mr Ditton to whom Newton referred was Humphry Ditton (1675–1715), Master of the Royal Mathematical School at Christ’s Hospital. In reality, it was Mr Ditton’s and Mr Whiston’s project, but the latter was an individual with whom Newton, once close, had now broken ties. Perhaps Newton could not quite bring himself to write the name, despite the presence of William Whiston (1667–1752, Fig. 3) at the parliamentary committee. Whiston had been Newton’s chosen successor as Lucasian Professor of Mathematics at Cambridge in 1702, but was expelled from the university in 1710 for his unorthodox theological views. Those views were largely shared by Newton but he was anxious to avoid a public accusation of heresy. Whiston blamed their rupture on Newton’s ‘fearful, cautious, and suspicious Temper’.7
After 1710, Whiston made his living as a scientific and theological lecturer, author and, he hoped, longitude projector – that is, someone who sought backing for a scheme, or project, intended to solve the longitude problem. He had lectured with Ditton from at least 1712 and they were promoting their longitude scheme the following year, through newspaper advertisements and letters drumming up English support by asking questions about foreign longitude rewards. In 1714, two petitions to Parliament appeared: one in April from Whiston and Ditton, and another in May recorded as being from ‘several Captains of her Majesty’s Ships, Merchants of London, and Commanders of Merchant-men’. The latter petition suggested that public ‘Encouragement’ would aid the search for a longitude solution.8 It was this, in which Whiston may also have had a hand, that instigated the parliamentary committee at which Newton presented his evidence. Prominent Whig politicians, whose patronage Whiston enjoyed, were to steer the new legislation.
Claiming inspiration from the extraordinary display of fireworks on the Thames that celebrated the end of the War of the Spanish Succession in 1713, Whiston and Ditton proposed that vessels moored at known locations could fire shells vertically to 6440 feet at set times. Navigators would keep a look out for the lights and gauge their bearing and distance relative to the moored vessel by compass and by timing the difference between the flash and sound of the shell, or by measuring its elevation. Whiston and Ditton thought large shells might be visible for a hundred miles and that, where deep seas meant hulks could not be moored, ships might run down the latitude until they neared the next signal post. Newton seemed disinclined to comment more than necessary: ‘How far this is practicable & with what charge, they that are skilled in sea affairs are best able to judge’.9

Fig. 3 – William Whiston, by an unknown artist, c.1690
{The Master, Fellows and Scholars of Clare College, Cambridge}
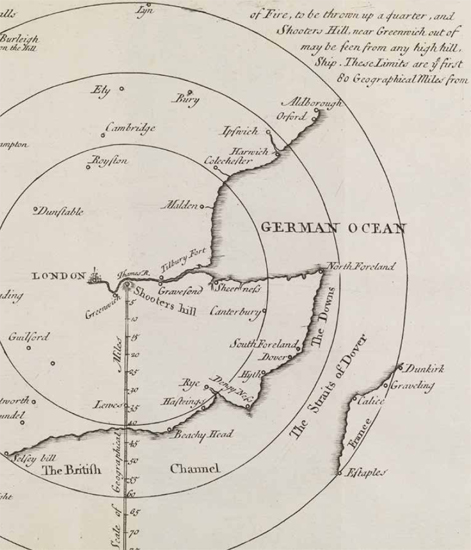
Fig. 4 – The limits of viewing the flash from a mortar fired at Shooter’s Hill, near Greenwich, from William Whiston’s The Longitude Discovered (London, 1738) (detail)
{National Maritime Museum, Greenwich, London}

Fig. 5 – A terrella (or ‘little earth’), a spherical lodestone used to model the Earth’s magnetic field, c.1600
{National Maritime Museum, Greenwich, London}
Whiston and Ditton presented their scheme more fully in a pamphlet addressed to the newly appointed Commissioners of Longitude. They offered it as a practical idea that, without universally solving the problem, would make a material difference. It was ‘easy to be understood and practis’d by Ordinary seamen, without the Necessity of any puzzling Calculations in Astronomy’ but would ‘prevent the Loss of abundance of Ships and Lives of Men’. The signals could provide both latitude and longitude, might be used to give exceptional warnings of bad weather, and would have most success in the areas of greatest danger – that is, near coasts. Here they invoked the maritime tragedy that occurred off Scilly in 1707, claiming their scheme ‘would certainly have sav’d all Sir Cloudsly Shovel’s Fleet, had it been then put in Practice’.
To put the idea into effect, Whiston, who continued the project after Ditton’s untimely death in 1715, relied on the skills of London’s firework makers and gunners as he began trials on Hampstead Heath and Blackheath (Fig. 4). There was merit in the idea, and explosives were later occasionally used to measure distances in survey work, but there were serious practical problems. Not least was the difficulty of mooring vessels in deep water, despite a claim that anchors might be secured by reaching down to supposedly still layers of water far below the surface.
It was all too easy for the firework scheme to be ridiculed, particularly by Tory satirists, who connected it to Whiston’s fiery and suspect theology, and cast the whole concept of longitude rewards as a Whig folly. Nevertheless, projecting, publicizing and finding patronage for longitude schemes remained one of Whiston’s major activities and sources of income. He explored all the accepted avenues of research, including one not mentioned by Newton: the Earth’s magnetism.
Magnetic variation and inclination
The idea that patterns in the Earth’s magnetic field might be a means of fixing position at sea had a long history and continued to be investigated in the eighteenth century and even into the nineteenth. It is interesting that Newton did not mention it in his evidence to the parliamentary committee, especially since he was joined there by Edmond Halley, an experienced astronomer, mathematician and navigator who had investigated these phenomena himself. As with Whiston’s signals, this method was about finding position relative to known locations rather than finding longitude itself. Presumably Newton and Halley therefore considered it discounted as a universal solution.
Most of the incoming proposals to the Spanish and Dutch longitude reward schemes were based on patterns in the Earth’s magnetic field, and many would be put to the British Commissioners of Longitude. There were two patterns that were investigated, with the hope that they were regular enough to be mapped and used. One was magnetic variation (also known as magnetic declination), which is the angular difference between magnetic north, shown on the compass, and true north, determined by the Sun or stars. A positive variation shows that magnetic north is east of true north, a negative one that it is to the west. The other was magnetic inclination, or magnetic dip, which is measured by the compass needle’s vertical rather than horizontal deviation. This is caused by the needle aligning itself with the Earth’s curving lines of magnetic force.
These were phenomena that had long been observed and investigated: variation had to be understood by navigators to correct steering directions, if not for position finding. In trying to make sense of the patterns of observational data, natural philosophers attempted to describe the Earth as, or containing, a giant magnet. One of the most famous of these accounts was De Magnete, published by William Gilbert, a London physician, in 1600. He undertook much of his research with spherical lodestones: known as terrellae, meaning ‘little earths’, these magnetic rocks were used to model patterns of geomagnetism (Fig. 5).
Magnetic inclination was also explored as a means of finding latitude, which, given that the Earth’s lines of magnetic force run north–south, had some plausibility. However, experiments had shown that the variations were too irregular and the observations too difficult to make at sea. In any case, astronomical observations were becoming much more effective for determining latitude. Thus, while schemes relating to magnetic inclination for latitude or longitude did not disappear, and even occasionally recurred in navigational textbooks, it was magnetic variation that had more impact. It involved significantly easier on-board observations and had a more plausible theoretical underpinning. This too was challenged, however, when it was demonstrated that the patterns change over time as well as place.

Fig. 6 – An amplitude compass, used for measuring magnetic variation from the apparent bearing of the Sun’s rising or setting; made by Ferreira, Lisbon, 1780
{National Maritime Museum, Greenwich, London}

Fig. 7 – Edmond Halley, by Thomas Murray, c.1690
{The Royal Society}
Nevertheless, navigation by magnetic variation was actually achieved, albeit in restricted locations or on familiar routes. The necessary tools were an amplitude compass (Fig. 6), to measure variation from observations of the sun and a chart recording previously observed lines of equal variation, against which to plot the ship’s position. This could be effective in specific circumstances, where the charting was detailed, and the lines ran nearly north–south and were reasonably close together. Some Portuguese navigators, for example, and those on Dutch East Indiamen, put the method into practice at various times during the seventeenth and eighteenth centuries, many apparently satisfied with the results.
Research into magnetism was a serious interest at the Royal Society, with demonstrations by their curator of experiments, Robert Hooke (1635–1703), who developed his own magnetic theory. Between 1668 and 1716, annual predictions by Henry Bond, a teacher of mathematics and navigation, were published in the Society’s journal, with the aim of encouraging magnetic observations against which his theory might be tested. Bond’s claims were investigated by a Royal Commission in 1674 and, although there was some doubt, he was paid £50 and given licence to publish his book The Longitude Found. On the basis that the six Commissioners were all Fellows, the book claimed the Royal Society’s approval, to which its President, Viscount Brouncker, objected strongly.
The Society’s interest nevertheless continued and was instrumental in persuading the government and the Navy to fund and equip a scientific voyage that would, among other things, chart magnetic variation as widely as possible. Edmond Halley (Fig. 7) was, very unusually for a civilian, given command of a specially built naval vessel, the Paramore, and set sail on two voyages in 1698 and 1699. He published charts of magnetic variation in 1701 and 1702 (Fig. 8), noting that they might be useful both for rectifying courses where compass readings might be unreliable, and for estimating longitude in places where the lines of similar variation were almost parallel to a meridian, provided that such charts were kept up to date to reflect change of variation over time.
While Halley did not produce updated charts, others did and they were put to use. However, the fact that this inexact, localized, practice-based and changeable method was not mentioned at the 1714 parliamentary committee underlines Newton’s view at the time that Parliament should be looking for a more complete solution. While Whiston and Ditton’s scheme had to be mentioned – and, by Whiston’s account, Newton’s initial ignoring of it risked the complete rejection of the proposed legislation – it perhaps served as a contrast to the great aim of finding a method that could be applied confidently at any location. It was, Newton suggested, only the astronomical and timekeeper solutions that held out that promise.
‘the Eclipses of Jupiter’s Satellites’
While rocket signals and magnetic schemes were about finding a means of fixing position relative to a known location (a moored hulk or charted magnetic feature), the other methods focused on the long-understood relationship between time and longitude. These were the only universal solutions to finding longitude at sea and, as Newton explained more than once to the Admiralty, only astronomical methods could be used to find longitude if it had been lost. The downside of astronomy was that observations could usually only be done at night, and sometimes irregularly if the target object was not in the right position, which meant that dead reckoning and other techniques were still required. Observations could also be also hampered by clouds, although this was equally true for calculating latitude and local time, without which neither astronomical nor timekeeper methods were effective.
The use of lunar and solar eclipses was the earliest of several potential astronomical methods for finding longitude. One key branch of research was to find ways of using the Moon’s position on a more regular basis, while the discovery of the moons (satellites) of Jupiter, with much more frequent eclipses, opened up new opportunities. The satellites were discovered in 1610 by Galileo Galilei (1564–1642, Fig. 9) with the use of a new instrument – the telescope. It revealed that Jupiter was orbited by four satellites that would disappear and reappear with useful regularity as they passed in front of or behind the planet (Fig. 10). They provided, in essence, a celestial timekeeper, visible at the same time from different points on Earth.
Galileo quickly realized that this was a potential means of finding longitude and, having drawn up provisional tables to predict the satellites’ motions, he attempted in 1616 to interest the Spanish in a proposal to make 100 telescopes and teach navigators the method. Having failed to convince them, he began protracted negotiations with the Dutch government in 1636, which only ended with his death six years later. The scepticism of the Spanish longitude committee was undoubtedly related to the practicalities of the method. Observing objects as small as Jupiter’s satellites with a telescope from a moving ship was clearly going to be very difficult. Even a century later, when the production of telescopes and lenses had vastly improved, Newton noted that ‘by reason of the length of Telescopes requisite to observe them & the motion of a ship at sea, those Eclipses cannot yet be there observed’.6
Galileo recognized this problem and looked for a means of steadying the observer. He designed a helmet, the celatone, which supported a telescope that could be adjusted continually to counteract the ship’s movement. At least one was made and tried on board a ship in the harbour at Livorno. It impressed a member of the powerful Medici family, who apparently ‘judged this invention more important than the discovery of the telescope itself’.10 Another idea was a hemispherical vessel in which the observer could sit and which would, in theory, be kept level by floating in a bath of oil (see Chapter 3, Fig. 29). Given the small number of telescopes at this time that could show Jupiter’s satellites at all, let alone a sharp image, such adaptations for use at sea were perhaps premature. Nevertheless, chairs, platforms and other devices that would ease shipboard observations continued to be explored.
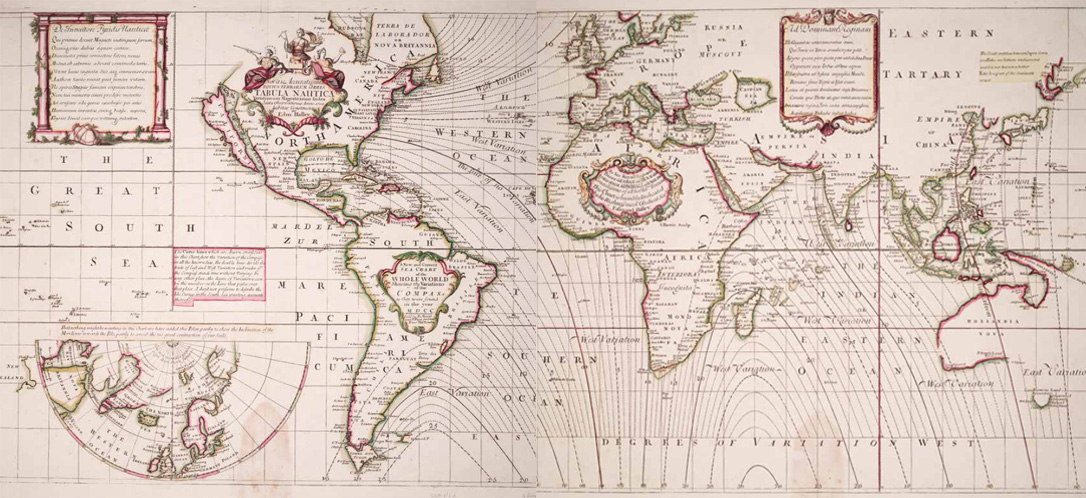
Fig. 8 – Edmond Halley’s world sea chart on two sheets, showing lines of equal magnetic variation, 1702
{National Maritime Museum, Greenwich, London}

Fig. 9 – Galileo Galilei, attributed to Francesco Apollodoro, c.1602–07
{National Maritime Museum, Greenwich, London}

Fig. 10 – Galileo’s journal of the observations of Jupiter and its satellites, 1610
{Biblioteca Nazionale Centrale di Firenze}
Ongoing attempts to make the method workable at sea were encouraged by the successful use of Jupiter’s satellites to establish longitude on land. This method began to flourish with the availability of improved telescopes and the publication of more accurate tables by Giovanni Cassini (1625–1712) in 1668. As director of the newly established observatory in Paris (Fig. 11), Cassini promoted the use of his tables on expeditions and in the mapping of France. In 1693, the Académie des Sciences published a map that compared the position of France’s coastlines on the new maps with the old (Fig. 12). Although Louis XIV, it is said, complained that the astronomers had taken more territory from him than his enemies, he and the Académie continued to finance the method and ambitious expeditions to map the nation and her empire.
Cassini’s method and tables were taken up elsewhere, including Britain. There, the new observatory at Greenwich focused on longitude, and its first director, John Flamsteed, produced his own tables of Jupiter’s satellites, published by the Royal Society in 1683. He doubted their use could be made practical at sea but encouraged sailors to learn the method for use on coastal surveys (or, rather, berated them for having not already begun to do so). By the early eighteenth century, it was clear that the use of simultaneous observations of Jupiter’s satellites to establish longitude on land could, with the best equipment and careful observers, be extremely effective. The main focus of research into astronomical methods for use at sea, meanwhile, moved elsewhere.

Fig. 11 – Paris Observatory, 1729. An astronomical quadrant with a telescopic sight and a large telescope with a mast and pulley for raising it are shown
{National Maritime Museum, Greenwich, London}

Fig. 12 – Map of France that compares the position of its coastlines on maps using new astronomical data with older maps, from Recueil d’Observations (Paris, 1693)
{National Maritime Museum, Greenwich, London}
‘the Place of the Moon’
While the leading astronomers at Paris and Greenwich had championed the use of Jupiter’s satellites, the founding of both observatories had been prompted by interest in what was known as the lunar-distance method. In France it was raised by a physician and Professor of Mathematics at the Collège Royal, Jean-Baptiste Morin, in 1634, and in London in 1674 by Le Sieur de St Pierre, another Frenchman, about whom almost nothing is known beyond his title. The method had advantages over Jupiter’s satellites in terms of what navigators would be required to observe at sea but it also had significant disadvantages with regard to the complexity of the Moon’s motions.
The method was already more than a century old, the first description having been published by Johann Werner of Nuremburg in 1514 and clarified in Peter Apian’s Cosmographica (1524) and Introductio Geographica (1533, Fig. 13), influential works that went through several editions. It made use of the well-known cross-staff (Fig. 14) to measure the Moon’s position as it moved against the background of stars. The crucial measurement – the lunar distance or ‘lunar’ – was the angle formed between the Moon and a star. With that, plus their altitudes, an accurate reference for the positions of bright stars distributed around the night sky and an almanac predicting positions of the Moon, a navigator could find the time at the place on which the tables’ data was based and subtract this from observed local time. This worked in theory but neither tables nor instruments were yet sufficiently accurate in practice, and the calculations required were actually more complex than those mentioned by Werner.

Fig. 13 – Title page of Introductio Geographica, Peter Apian (Ingolstadt, 1533)
{National Maritime Museum, Greenwich, London}

Fig. 14 – Decorated ivory cross-staff, by Thomas Tuttell, c.1700
{National Maritime Museum, Greenwich, London}
There were some surprisingly early attempts to determine longitude by versions of this method. In July 1612, the English Arctic explorer William Baffin observed the Moon’s transit, or passing, across his local meridian to determine longitude while in Greenland, but found it ‘somewhat difficult and troublesome’.11 He took the observation from land, recognizing that it would be impossible from a moving ship. Three years later, on an expedition in search of the North-West Passage, he made observations from the ship to determine longitude from the angular distance of the Moon from the Sun. The range of observations Baffin made on these voyages suggests that he was quite unusual among English mariners. For most, these forays into the complexities of astronomical navigation would have been entirely unfamiliar.
Even in the seventeenth century, the method was not well known beyond mathematical circles. Thus, when Charles II heard of St Pierre’s claims to have solved the problem of longitude by using this method, a commission was appointed to examine their validity. The commissioners foreshadowed those appointed forty years later by the 1714 Act. They included the President of the Royal Society, the King’s Master of Mechanics, professors of astronomy and mathematics, including Christopher Wren (1632–1723) and Robert Hooke, and other Fellows of the Royal Society. Most of them had also been responsible for judging Henry Bond’s magnetic scheme the year before and it is likely that St Pierre hoped for a reward, given the commissioners’ recommendation that the King ‘grant some present support for Mr Bonde’.12
However, as happened in France, it became clear that the method was unworkable without a vastly improved catalogue of stars and theory of the Moon’s motion. In both cases, the resulting recommendation was to found an observatory and appoint astronomers. Already co-opted to the Commission was John Flamsteed, a young astronomer who had impressed several Fellows of the Royal Society and had an influential patron in Jonas Moore, Surveyor-General of the Ordnance. On 4 March 1675, Charles II signed a royal warrant that appointed Flamsteed his ‘astronomical observator’ and charged him ‘to apply himself with the most exact Care and Diligence to the rectifying the Tables of the Motions of the Heavens, and the places of the fixed Stars, so as to find out the so much desired Longitude of Places for perfecting the art of Navigation’.13 An observatory, designed by Wren and Hooke, was built at Greenwich (Fig. 15) and Flamsteed began his long series of observations there on 16 September 1676.
It was in the second of Flamsteed’s tasks that he had greatest success, although it was over a lifetime of observation and was the cause of some tribulation. His great legacy was a much larger and more accurate catalogue of ‘fixed stars’ than previously existed. On the way to producing this master-work, Flamsteed published tables of Jupiter’s satellites and other observations, calculations and commentaries. He also shared data with astronomers and mathematicians across Europe as part of a reciprocal, scholarly correspondence. His relationship with one of the most important figures of the period, Isaac Newton, broke down when he felt this scholarly etiquette was ignored. Newton, desperate to have access to as many observations as he could for the improvement of his theoretical work, demanded, in Flamsteed’s view, too much and gave too little in return.

Fig. 15 – Royal Observatory from Crooms Hill, British School, c.1696
{National Maritime Museum, Greenwich, London}

Fig. 16 – The effects of the Sun on the Moon’s motion, from Isaac Newton’s Philosophiae Naturalis Principia Mathematica (Cambridge, 1713) (detail)
{National Maritime Museum, Greenwich, London}
A serious quarrel took place over Flamsteed’s catalogue. Newton, as President of the Royal Society, urged its publication and Prince George of Denmark, Queen Anne’s consort and Lord High Admiral, agreed to pay. Newton, Halley and other Royal Society ‘referees’ for the publication gained the upper hand by persuading Queen Anne to appoint a Royal Society committee as a Board of Visitors to the Royal Observatory, with power to tell the Astronomer Royal what to observe and publish. In 1712, an edition of Flamsteed’s valuable catalogue was published prematurely, without his knowledge and to his lasting fury. The process of assembling his own edition took the rest of his life and, thanks to the dedication of his wife and two assistants, appeared posthumously in 1725.
Newton’s interest in Flamsteed’s observations had been particularly intense when he was struggling with the second edition of his Principia. The first edition, with its mathematically expressed laws, including the inverse square law of universal gravitation, provided a new and essential framework for predicting the motions of the planets. However, the complexity of the Moon’s motion, caused by the interplay of the gravitational influences of Earth, Sun and Moon, was great enough to defeat Newton. He struggled again with lunar theory and the so-called three-body problem for the book’s 1713 edition (Fig. 16), building on observational data from Flamsteed and others and recalling later that ‘his head never ached but with his studies on the moon’.14
Newton wished to devise a theory, based on both mathematics and empirical observations, that was accurate to two minutes of arc (that is, to one-thirtieth of a degree). He felt that this high level of accuracy was necessary in the theory in order to achieve accuracy to within one degree in practical navigation observations. However, in this he failed. Despite his best efforts, his evidence to the parliamentary committee of 1714 had to state that the Moon’s ‘Theory is not yet exact enough’ to determine longitude at sea within one degree. Nevertheless, he gave the impression that this improvement would be forthcoming and that it was here that the long-awaited solution would lie.
‘a Watch to keep Time exactly’
The accuracy of Flamsteed’s observations depended on a recent revolution in timekeeping. One of the coordinates that indicates the position of stars is expressed as time: the moment at which a heavenly body passes, or transits, the observer’s local meridian (line of longitude). In order to record this accurately and precisely good clocks are required. Clocks had become capable of acting as scientific instruments, known as astronomical regulators, once they incorporated pendulums, the timekeeping properties of which had been observed by Galileo. It was left to the Dutch mathematician and astronomer Christiaan Huygens (1629–95) to apply this to a clock in 1657.
The Octagon Room of the Royal Observatory at Greenwich was designed around the pendulum clocks installed there (Fig. 17). Made by Thomas Tompion, London’s leading clockmaker, they were an experimental design with thirteen-foot pendulums suspended behind the room’s panelling and above the dials. They were accurate enough to help Flamsteed in his first task as Astronomer Royal: to demonstrate that the Earth itself is a regular timekeeper, a prerequisite of the positional astronomy he was appointed to improve.
The timekeeper method of finding longitude at sea – a shipboard clock that would keep the time at a known location throughout a sea voyage for comparison with observations of local time – was, as Whiston and Ditton said, ‘the easiest to understand and practice’. The huge strides made in the accuracy of pendulum clocks were encouraging but the technical challenges facing their application at sea were huge. Watches, less influenced by the motion of the ship, were much too inexact. As Whiston and Ditton’s 1714 pamphlet put it:

Fig. 17 – The Octagon Room at the Royal Observatory, Greenwich, by Francis Place, c.1676
{National Maritime Museum, Greenwich, London}
Watches are so influenc’d by heat and cold, moisture and drought; and their small Springs, Wheels, and Pevets are so incapable of that degree of exactness, which is here requir’d, that we believe all wise Men give up their Hopes from them in this Matter. Clocks, govern’d by long Pendulum’s, go much truer: But then the difference of Gravity in different Latitudes, the lengthening of the Pendulum-rod by heat, and shortening it by cold; together with the different moisture of the Air, and the tossings of the Ship, all put together, are circumstances so unpromising, that we believe Wise Men are almost out of hope of Success from this Method also.15
It had ‘been so long in vain attempted at Sea, that we see little Hopes of its great usefulness there’.16 Dependence on a single clock was also potentially dangerous if there were no means to check its performance.

Fig. 18 – Marine timekeeper, by Severyn Oosterwijck, c.1662 based on the designs of Alexander Bruce and Christaan Huygens
{National Maritime Museum, Greenwich, London, Private Collection}

Fig. 19 – Frontispiece to Thomas Sprat’s History of the Royal-Society of London (London, 1667); note the triangular maritime timekeeper at the top left and the two navigational instruments hanging on the column behind it
{National Maritime Museum, Greenwich, London}
One of those who had attempted to make functional sea-clocks was the same Christiaan Huygens who had had such success with pendulums. As with others in this story, his attempt to apply this work to the problem of longitude was immediate. Huygens had a deep theoretical understanding of the physical principles behind this practical work, making use of clockmakers and others to construct, test and develop his designs. These collaborations were fruitful but not always easy.
One individual with whom he became involved was Alexander Bruce, Earl of Kincardine, who tested a timekeeper on a voyage between Scotland and The Hague in early 1662. Its performance convinced Huygens to collaborate, and he joined Bruce in making and testing two further clocks on the same model, with the assistance of Severyn Oosterwijck, a clockmaker from The Hague (Fig. 18). Further trial results were sufficiently encouraging for work to be continued, now also in collaboration with Hooke. The clocks were approved at the Royal Society, especially after a very favourable (and probably untrue) report of them was given by Captain Robert Holmes after a voyage to Lisbon. Huygens was made a Fellow of the Royal Society when he visited London in 1663, patents were discussed and the Duke of York expressed interest in the clocks. One of these triangular clocks has been identified on the left of the iconic frontispiece of Thomas Sprat’s 1667 History of the Royal-Society of London (Fig. 19). Despite all this, the clocks were little used.
Huygens continued to develop his ideas. Much of this work was done in relative secrecy and, even once revealed, seems to have made little impact. However, by 1666 he was working within the Académie des Sciences for the French Crown and further sea trials were undertaken, some achieving good results. He published another design in Horologium Oscillatorium in 1673; this timekeeper was spring-driven, set on gimbals (devices designed to keep objects level in unstable conditions), and had a triangular pendulum that was forced to move in only one plane. A later version (Fig. 20) was tried, inconclusively, in the 1680s, this time in collaboration with the Dutch East India Company. Huygens was also one of those who, in the 1670s, were involved with the invention of the balance spring, crucial to the development of accurate watches. There are hints that Huygens saw this as a potential alternative for a timekeeper solution to the longitude problem but, because springs were too greatly affected by changes in temperature, it was not one he pursued.
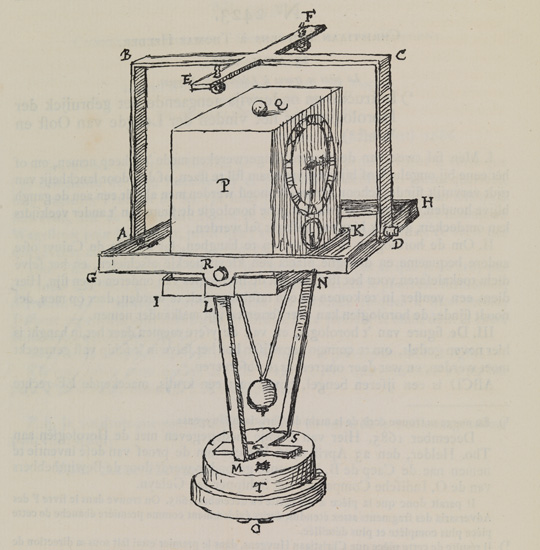
Fig. 20 – Christiaan Huygens’ design for a marine timekeeper, originally drawn c.1685–86
{National Maritime Museum, Greenwich, London, Courtesy of Jonathan Betts}

Fig. 21 – Longitude timekeeper, designed by Lothar and Conrad Zumbach de Koesfelt, made by Franciscus le Dieu, 1749
{Museum Boerhaave, Leiden}

Fig. 22 – Plate from Henry Sully’s Description Abrégée d’une Horlorge d’une Nouvelle Invention (Paris, 1724)
{National Maritime Museum, Greenwich, London}
Huygens died in 1695, having made some huge practical and theoretical advances but without a clock having yet been taken up as a usable tool at sea. His influence on those who followed, through his publications, manuscripts or collaborators, was enormous. One follower was Lothar Zumbach de Koesfelt, a Dutch physician, mathematician and musician, who described a sea-clock in 1714. It was later improved by his son Conrad, who in 1749 also designed a clock that used a glass container to control its temperature (Fig. 21). Another was Henry Sully, who, trained in England and working on the Continent, experimented with a marine clock and watch (Fig. 22). Sully made ample use of both French and English networks, not least the Royal Society’s chief authority on instruments and timekeepers, George Graham (1673–1751). While Sully’s clock had mechanisms that made it portable and minimized the effects of temperature and gravity, Graham’s report and trials were to show that a ship’s motion in open sea would fatally influence its pendulum.
In 1714, then, there were several potential solutions to the problem of finding longitude at sea. Most of them were theoretically viable and had already been researched by mathematicians, astronomers, artisans and mariners. In many ways the ground had been prepared: the next, crucial step may not have seemed all that distant. However, all of the methods presented practical problems, most particularly due to the conditions under which observers or instruments had to perform at sea. It was a practical and technical set of problems, and the government hoped that these could be met so that the methods ‘true in theory’ might be made ‘Practicable and Useful at Sea’.
While much previous work had taken place in Spain, Holland, Venice and elsewhere, the momentum had decisively shifted towards Britain. Ideas and skills were available, particularly in London with its flourishing instrument trade, maritime interests, places of open discussion, such as coffee-houses, and widespread access to print. These opportunities were seized by many of those with ideas, mechanisms and projects relating to longitude. The Royal Society, Astronomer Royal and Commissioners of Longitude were marked out as the key figures from whom to seek interest, approval and, perhaps, a route towards one of the 1714 Act’s enormous rewards.