You line up against the wall. Someone puts his hand on top of your head and marks its position with a short, horizontal line. You step away and look at the wall. This is how tall you are.
In Roman Ondák's ‘Measuring the Universe’, first performed in 2007 at the Munich Pinakothek der Moderne, visitors relive a common scene from childhood. Substituting for the parent, museum attendants ask visitors whether they want to be measured and mark their height with black felt-tipped pen on the exhibition room's wall, annotated with their name and the date of their visit. As in the common household tradition, the marks indicate a passing of time. Not as a document of a child's growth, however, but as a reminder of the succession of visitors. Over the course of an exhibition, ‘Measuring the Universe’ grows from a single black mark on the wall into a hazy wall drawing that eventually encircles the space. As more and more people are measured, the marks accumulate, and a dense, almost entirely black area appears – a dark horizon that indicates the average height of the exhibition's visitors. While Ondák argues that ‘the performance has the same status with one mark on the wall as with thousands of them’ (Ondák 2009: 78), the relation between the black horizon and the space points to a specific proportion between body and space. So, if the title of the work refers to ‘humankind's age-old desire to gauge the scale of the world’ (MoMA 2009), as the curators of MoMA's ‘Performance Exhibition Series’ claim, how exactly does measuring the body function in determining that sense of scale?
Before Ondák was even born, the act of measurement had already been referred to or implemented by a number of artists. In 1963, for example, Robert Morris presented a work entitled ‘Three Rulers’, an oblique reference to a much older work: Marcel Duchamp's ‘Three Standard Stoppages’ (1913–14). Most examples, however, date from around the time of Ondák's birth in 1966. Most importantly, it is then that two dedicated artists started to build an entire oeuvre around the premise of measurement. By placing Ondák's ‘Measuring the Universe’ in relation to the works of these two artists, this chapter considers the different ways in which measurement is employed as an artistic strategy and the implications thereof on defining a notion of scale.
Sometime during the late 1960s or early 1970s, the illusive Dutch artist Stanley Brouwn took on the task of meticulously measuring and recording distances and dimensions. In order to do so, however, he did not count on the metric system alone. Rather, one of the first things he did was to formulate a personal system of measurement, based entirely on the dimensions of his own body. Thus, Brouwn's earliest measurement pieces revolve primarily around the definition of a list of standard measures: the sb (or Stanley Brouwn) -foot, -ell, and -step. Most often, the standards were defined in relation to the metric system and transferred onto paper in a variety of ways. ‘1 Step’ (1973), for example, is a filing cabinet holding 847 index cards in which each card represents 1 mm. As the title suggests, the total of 847 cards refers to the length of 1 sb-step. Another filing cabinet holds 1,000 of the same cards (1 m) and includes a bookmark to indicate the length of 1 sb-step. The same confrontation between a sb-step and a metre appears in the artist's book from 1976, entitled Distance = Length. Each page of the book is marked with a series of 10 cm lines, but whereas a left-hand page shows ten lines (1 m), the facing right-hand page only shows seven full lines and part of an eighth line (1 sb-step).1 In later works, which he called ‘portraits’, Brouwn also measured other people – representing them in the form of a single aluminium bar and a note indicating their height.
It was around the same time, in the late 1960s, that the American artist Mel Bochner became interested in measurements. Rather than measure his body, however, Bochner took a ruler around his studio and measured more or less everything he could find, from cans of spray adhesive to the distance between the light switch and a shelf (Field 1995a: 34). Later, he started to tack cardboard pieces to the walls to measure the space between them, or he simply measured the width of a piece of cardboard and inscribed it directly onto the panel itself. His 1969 ‘8” Measurement’, a sheet of graph paper with the simple notation of its width, is just one of the many examples of Bochner's self-referential measurement pieces.
Because both Mel Bochner and Stanley Brouwn are usually considered conceptual artists, and Roman Ondák is referred to as a neo-conceptual artist, it seems obvious to consider their respective interests in measurement from that vantage point. Given its seeming simplicity and the systematic way in which it can be applied, ‘measuring things’ must have appeared to the conceptual artist as a perfect way to make art. Just like counting, stacking and ordering, the systematic act of measuring implies but a simple premise that can easily be repeated: to compare an object's (or person's) dimensions to a standard measure such as the inch or the centimetre. By simply applying that premise, one can easily circumvent the need for personal, aesthetic and stylistic decisions, and produce an artwork that is instead based solely on a predetermined description and its systematic execution. As Sol LeWitt so elegantly put it in his 1967 ‘Paragraphs on Conceptual Art’: ‘The idea becomes a machine that makes the art’ (LeWitt 1999: 12).
If we are to believe popular narratives of conceptual art, artists even aimed for an art in which the idea that initially led to the production of an artwork would simply replace it, altogether substituting ‘the object of spatial and perceptual experience by linguistic definition alone (the work as analytic proposition)’ (Buchloh 1999: 515). Accordingly, measurement pieces such as those by Brouwn, Bochner and even Ondák seem to replace the objects of spatial and perceptual experience by their straightforward geometric definition – geometry being, just like language, a sign-system and model of thought. We get measurements instead of objects; instead of people, even. Indeed, with their simple notations in black felt-tipped pen, their artist's books and their filing cabinets, the works under discussion are easily associated with what Buchloh called the ‘aesthetic of administration’.2
The human body enters into the total continuum of sizes and establishes itself as a constant on that scale.
(Morris 1995: 11)
In a work from 1968, Mel Bochner obliquely addresses issues of size and scale. ‘Actual Size’ consists of two photographs printed on a 1:1 scale. Each photograph depicts a part of the artist's body as it is lined up along a black line annotated ‘12″’. In both photographs, the background is a simple white wall; all we see is Bochner's head (‘Actual Size (Face)’) or arm (‘Actual Size (Hand)’) next to the line of black tape. The juxtaposition of body and measurement, as well as Bochner's insistence on printing the photographs in ‘actual size’, raises the question as to how we determine an object's size when it is depicted in a medium that is essentially scaleless; for photographs are not usually printed in actual size, nor are we used to them featuring measurements. We might have seen rulers appear in detailed photographs of crime scenes or archaeological finds, but most of the time, there is no absolute way of telling how big a depicted object is in reality.
So how do we know the size of an object in a photograph (Field 1995a: 34); or in a painting, drawing or sculpture for that matter, as the issue is hardly exclusive to photography? When it comes to representation, most artworks have a very specific illusionistic scale, inherent to the work itself. We do not take pictures literally; we size up the depicted elements in relation to each other rather than in absolute terms. Yet, in order to get a sense of scale, we depend on a certain frame of reference. The depiction of bodies, among other things, constitutes such a frame of reference. A background, too, can provide a frame of reference, but there's nothing we know quite as well as (the size of) the human body. Claes Oldenburg, for example, made excellent use of this quality in his sketches of proposed colossal monuments. By adding tiny marks suggestive of human bodies or traces of a cityscape to his drawings, Oldenburg catapulted everyday objects such as popsicles (‘Proposed Colossal Monument for Park Avenue: Good Humor Bar’, 1965) and vacuum cleaners (‘Proposed Colossal Monument for the Battery, NYC: Vacuum Cleaner’, 1965) from the modest realm of their everyday use into that of giant buildings (Davidts 2008). When determining the illusionistic scale of a picture, bodies thus serve as excellent references of size.
‘Actual Size’, however, is about more than just illusionistic or imaginary scale. Here, the photographs are ‘life size’, creating a conflation between their illusionistic scale and the actual scale of the situation in which they are presented. Rather than being a point of reference between the realm of the photograph and that of the viewer, the depicted body becomes a point of confrontation, of collision, ‘returning the photograph to a literal relationship with the world’ (Field 1995a: 34). As such, ‘Actual Size’ does not obviate the issue of scale, as Richard S. Field suggests, but rather pulls it in a different direction – that of minimal art's (or, better yet, Robert Morris's) phenomenological notion of scale. Because minimal art renounced representation, there was no place for an imaginary scale. Instead of looking for relations within a work, minimal art called attention to the relations between a work and its surroundings. ‘Sculpture no longer stands apart, on a pedestal or as pure art’, Hal Foster observed in his seminal essay ‘The Crux of Minimalism’, ‘but is repositioned among objects and redefined in terms of place’ (Foster 1996: 38). Scale, then, revolves around a physical and phenomenological confrontation between a spectator and a work of art within a certain space. But Morris did not want just any relationship between these different aspects; he pleaded for a very specific one. Minimal art had what appears to be a very clear project: to make the viewer aware of how his or her own presence and position within a certain situation influenced perception. In order to do so, however, viewers could not be distracted by formal details or skewed relations between an object and its surroundings. In order to reveal the disjunctions in the perceptual process, artists thus opted for ‘extraordinarily clear elementary polyhedrons, executed in specific materials at a precise scale in relation to the human body’ (Mitchell 1995: 254). Scale, in other words, becomes a very specific dimensional rapport between the artwork, the spectator and the space in which they are confronted.
The question in this chapter, however, is what kind of relation between artwork, spectator and space is pronounced when the artwork seems to exist only as a simple notation of measurement. And what if, as Stanley Brouwn's insistence on the term ‘portrait’ implies, the body can equally be reduced to a mere dimension? What does this imply for a definition of scale in conceptual art?
In a series of works started in the late 1980s, Stanley Brouwn used aluminium bars and wooden cubes based on his own bodily dimensions to measure (exhibition) spaces. The resulting works describe spaces in terms of a very basic equation, measuring x by y by z sb-feet, for example. Sometimes, the dimensions are inscribed on a plaque hung in the space itself, but in most cases they are simply printed in artist's books and referred to as ‘portraits’ of spaces. In pronouncing the specific dimensional rapport between an object, a body and a space, these works are directly related to minimal art's notion of scale. Moreover, by defining that rapport in terms of the body, they underscore and even literalise the body's role as a reference of size. Only, instead of doing so through the spectator's own, messy experience of such a relation, it is immediately reduced or ‘purified’ to a literal recording of it. Following a reductivist notion of conceptual art in which ideas can replace reality: if scale is a dimensional relation to begin with, why not pronounce it as a simple geometric equation, a basic proportion?
By literalising the body's role as a reference of size, however, Brouwn also seems to undermine that role. For, while everything is considered in terms of the artist's bodily dimensions, the actual body is inexorably absent. Throughout his career, Brouwn has made it a point to remain anonymous and to leave both himself and his body out of the picture. By presenting nothing but descriptive titles or collections of lines, the notion of a body as a reference of size is extracted from the realms of vision and experience, and reduced to a purely abstract, notational level. In the end, the body has become pure dimension, pure idea, much in the same way as the empirical system has lost all reference to our actual bodies and become mere convention. Although Brouwn's system of measurements may have been based on the dimensions of an actual body, to the viewer it is nothing but an abstract convention – a reference of measurement that is, quite literally, disembodied.
The notion of a disembodied measurement, however, is problematic. In the late 1960s, Mel Bochner had measured a distance of 25 inches between two pieces of paper, which he had subsequently removed – not unlike Brouwn, who ‘removed’ the actual reference of his measurements, namely his body. What remained was an isolated notation of 25 inches on the wall, nothing but a line and some numbers. According to the curator Brenda Richardson, the isolated measurement raised a large number of questions regarding the qualities of measurement. ‘What is inside and what is outside a given measure of length or width or height? By what criterion can any unit of measurement be determined? How verifiable is a measurement?’ (Bochner 2008c: 98). Bochner addressed these questions in a range of experiments. In a cardboard installation entitled ‘Measurement: 36” + 36”’ (1969), for example, he dealt with issues of visibility and accumulation by defining 36 inches as the width of one cardboard piece as well as that of all the visible parts of six other pieces put together. In a slightly more poetic work, ‘Measurement: Plant’ (1969), he measured not the plant itself but rather the projection of its shadow on the wall and the change therein. The work points to a problematic aspect of the act of measuring, namely that three-dimensional objects don't always allow for easy measurement. In order to measure something, one often depends on a one-or two-dimensional projection of the object. In other words, one often measures but a projection and not the object itself, suggesting that the act of measurement is hardly as straightforward as it may appear in popular narratives of conceptual art.
Bochner's oft-cited conclusion to all these experiments states that
measurement reveals an essential nothing-ness. The yardstick does not say that the thing we are measuring is one yard long. Something must be added to the yardstick in order that it assert anything about the length of an object. This something is a purely mental act … an ‘assumption’. If we subtract this assumption (avoiding any connotations of irony), what is left?
(Bochner 2008c: 98)
In a 2005 essay entitled ‘From Box to Street and Back Again’, art historian Mark Godfrey calls upon this citation in a discussion of ‘Actual Size’. He notes that
if the photographs unusually doubled the scale of the situation, the measurement itself remained quite abstract. They did not show you how tall Bochner stood, but simply the length of his appendages. Remove the body, and the annotation would tell you nothing – for what is 12 inches alone on a wall?
(Godfrey 2005: 33)
In reality, the 12-inch measurements did not even tell you the length of Bochner's appendages, as they acted as general references of size rather than specific measurements, but the point is that the measurement alone is essentially meaningless. It is the depiction of Bochner's body that provides us with a sense of scale, not the 12-inch measurement. Likewise, the abstracted dimensions of Brouwn's body can assert little about scale. They may tell you how many times Stanley Brouwn's foot fits into the height of a room, but they do not show ‘how tall the artist stood’. Just like the terms of the empirical system – once based on bodily dimensions – they have lost all relation to actuality. They have become mere convention, or, in the words of Mel Bochner, ‘signifiers with nothing to signify’ (Godfrey 2005: 33), implying that the scalar equation too has collapsed and become meaningless.
This is exactly what differentiates ‘Measuring the Universe’ from Stanley Brouwn's works. For, while both Brouwn and Ondák refer to a certain proportion between a body and a space without that body being present, Ondák does show how tall someone stood. The terms of ‘Measuring the Universe’ are, in fact, quite different from those of Brouwn's spatial portraits, and so are the relations between object, body and space pronounced by the work. ‘Measuring the Universe’ unfolds only as a confrontation between a spectator and a specific space – the spectator's actual presence in that space, even if only for a little while, is essential. Ondák does not present the viewer with an estab I i shed, though meaningless, ‘fact’ – ‘that space measures x by y by z sb-feet’ – but rather calls upon him to ascertain for himself how the height of the space relates to that of his own body.
A crucial aspect here is that Ondák never goes on to actually measure people. Unlike parents marking their children's height, no one ever puts a ruler to the marks made by the museum attendants. In ‘Measuring the Universe’, there are no numerical indications of people's heights whatsoever. Thus, contrary to Stanley Brouwn, Ondák does not reduce bodily dimensions to an abstract system of meaning that, in its turn, can be applied to a number of situations. Rather, he limits himself to projecting people's heights onto the wall, thus leaving the work grounded in the actual situation at hand. While the material component of ‘Measuring the Universe’ consists, just as in Brouwn's works, of little more than linguistic and numerical signs, these markings are far from absolute. Following the traditions of minimal art, their meaning is wholly dependent upon their position on the wall and in space. Here, there is no measurement to ‘pick up’ and apply to other things; there is only a mark on the wall that indicates the height of a body that was once there. As such, the work retains a close relation to minimal art's notion of scale and the role of the body as a reference of size. By confronting the spectator with a projection of other bodies onto space, he becomes aware of the relation of his own height to that of not only the space, but of other people as well. Unlike the projection of the length of one's stride onto the height of the room, which bears no relation to how one actually experiences that height, the marks in ‘Measuring the Universe’ are inherently related to how we ourselves are positioned in space. The spectator can thus relate to the markings on the wall in an actual, experiential sense rather than as abstracted measurements. Ondák does not attempt to capture or indicate the size of the body in a seemingly absolute and widely applicable abstract system, nor does he present the notion of scale as a simple equation between two dimensions; he aims to show the (past) presence of specific bodies in relation to a specific space.
Ondák's projection of people's lengths onto the wall might imply some level of abstraction; his insistence on a spectator's actual presence within the space keeps the work grounded in experience. One could say his work exists in the space of events, whereas Brouwn's exists primarily in the abstract space of statements. The terms ‘space of statements’ and ‘space of events’ are derived from a 1995 installation of Mel Bochner's ‘Measurement: Room’ (1969), which received the subtitle ‘(From the Space of Statements to the Space of Events)’, and refer to categories of thought on the one hand and ‘raw’ material on the other. Instead of accepting the mental and the experiential as two distinct categories, Mel Bochner dedicated a large part of his career to examining the friction between abstract systems of knowledge and our experience of the world. Therefore, the artist has always insisted on coupling abstract measurements with the specific experience of the situation at hand – a coupling that, in light of this chapter, is most interestingly realised in ‘Measurement: Room’.
Contrary to the works of Ondák and Brouwn, ‘Measurement: Room’ does not measure the body. Instead, it measures the specific architectural elements of an exhibition room and inscribes their dimensions directly onto the surface of its walls, right next to the element under consideration, resulting in what looks like a three-dimensional blueprint of the space. The abstracted space, in the form of its measurements, is thus inscribed directly onto the actual space. Yet, as Yves-Alain Bois has pointed out, the abstracted and the actual space do not coincide. ‘The small intervals between the actual architectural elements (arris and apertures) and the taped mensuration were not insignificant. On the contrary, they reinforced the impression that the room had been duplicated’ (Bois 1995: 168). Or, in the words of Bochner himself, ‘[t]he measurement projects a mental construct of the space onto the space itself’ (Bois 1995: 168).
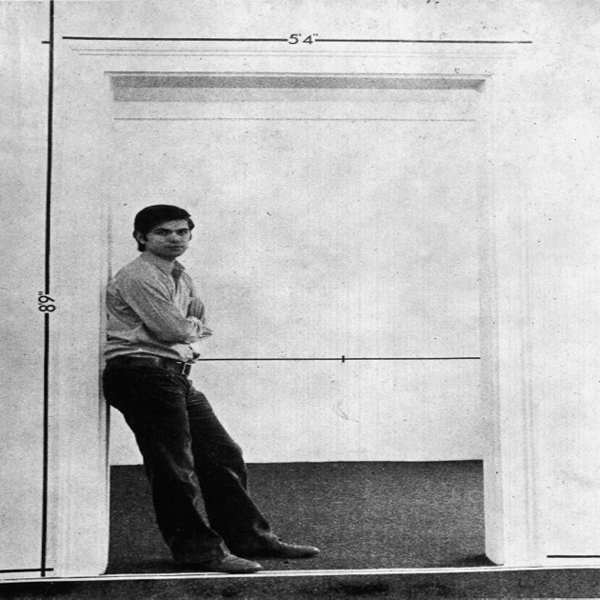
Despite the fact that ‘Measurement: Room’ entails the measuring of a space instead of that of a body, the work does propose a specific relation between measurement, body and space, if only because, just like in Ondák's ‘Measuring the Universe’, one has to actually enter the space to ‘see’ the work. More interestingly, though, it is exactly the fact that it is not a body that is being measured that makes the work such a valuable case study. In order to ascertain the work's and the measurements’ relation to the body, consider the invitation card to Bochner's 1969 solo show at the Konrad Fischer Gallery in Dusseldorf.
Figure 1 Invitation card to the Mel Bochner exhibition ‘Measured Room Series: 48” Longitudinal Projection’ at Konrad Fischer Gallery, Dusseldorf, 1969.
The photograph shows the artist inside his initial ‘Measurement: Room’ at the Heiner Friedrich Gallery in Munich, leaning against the door frame that is so often pictured to illustrate the work. In relation to the body, the measurements of the room function in much the same way as the 12-inch measurements in ‘Actual Size’: they are references of size, ‘standard’ measures to which one can compare the body but not actually measure it. Unlike in the photographs of ‘Actual Size’, however, Bochner does not even try to ‘line up’ to these measurements anymore. Instead of the controlled, static pose of ‘Actual Size’, the artist is nonchalantly slouched against the door frame. This is not the passive object/body that serves as a mere reference of size, as in Brouwn's spatial portraits and, to a less abstracted degree, ‘Actual Size’; it is a specific subject that occupies the space. Thus, what the opposition between Bochner's casual pose and the geometric rendering of space points to is not only that the body does not allow for easy measurement or that there exists no unilateral relation between the measurements of a body and those of a space, but primarily that one's height is not the only point of reference in a relation between body and space. It suggests that the question of scale revolves not just around ‘how one measures up’, but more elaborately around how one occupies, uses and experiences space.
Instead of merely presenting the space or its relation to a body in the most reductive and abstract of terms, Bochner deliberately posits the subjectivity of the viewer's experience as an essential counterpart to the supposed objectivity of geometry. He highlights the tension between our experience of the world and the terms in which we try to grasp it. This confrontation between a space of statements and a space of events illustrates that abstraction is a one-way street and that, while our experience may be influenced by dimensional notations, the latter can never replace that experience. No matter how accurate a geometric description, ‘our only means of verification’, as Bochner once explained in an interview with Lisa Haller, ‘is our own experience’ (Bochner 2008a: 108). While at significantly different levels of bodily and spatial engagement, the works discussed in this chapter eventually all underline the impossibility of reducing body and space to mere dimension, to pure references of size. For, while the measurement might be disembodied, the spectator never is, and the work never appears in a spatial vacuum.
Each artwork asks a spectator to relate to it, and it is this relation that constitutes a work's scale, not the object–body–space relations within the work. Thus, if Ondák asserts that ‘Measuring the Universe’ has the same status with one as with a thousand marks, he does so because what matters is each spectator's personal experience of his relation to the space, not the average proportion of space to spectator. Likewise, the scale of Stanley Brouwn's works is not the proportional relation between the artist's body and the space ‘portrayed’, but rather that between the spectator and the ‘portrait’. As ‘spectators’ of these abstracted measurements, our relation to the work is dependent on a specific frame of reference, just as in representation. Only, as we cannot grasp Brouwn's works in their purely notational form, the work itself does not provide us with such a frame. The measurements themselves mean little to us, and we inevitably project them back onto our own situation, considering that, if Brouwn's feet are more or less the same size as ours, the space represented in his portrait must be slightly higher than the one we are in, for example. Maybe we even walk through the space, retracing Brouwn's steps, even though they were taken at an entirely different place. Our confrontation with even a simple dimensional notation, even if it refers to a wholly different situation, inevitably triggers a certain experiential relation to our own situation. Thus, the use of measurements does not reduce the matter of scale to a purely dimensional rapport, nor does it collapse the relation into abstract meaninglessness. Rather, the measurement pieces show that a scalar relation between body and space considers more than just one's height or size; it also considers how one uses, occupies and experiences space in terms of size, movement and time. If scale is considered as a relation between the spectator and a work of art, works of conceptual art underline the origin of such relations in subjective experience.
1 Contrary to the sb-foot and the sb-ell, measuring 27 cm and 47 cm respectively, the sb-step is not considered an absolute dimension. Rather, the length of Stanley Brouwn's stride was re-ascertained with every application of it, explaining the different definitions of an sb-step.
2 The term refers to how ‘Conceptual art came to displace even that image of the mass-produced object and its aestheticized forms in Pop art, replacing an aesthetic of industrial production and consumption with an aesthetic of administrative and legal organization and institutional validation’ (Buchloh 1999).