Chapter 8

Acids and Bases

Two acid–base theories are used in organic chemistry today: the Brnsted theory and the Lewis theory.¹ These theories are quite compatible and are used for different purposes.² However, the Lewis-based idea of electron-donating species (bases) and electron- accepting species (acids) is often the more useful for organic chemistry. Remember also that most organic reactions are not done in an aqueous medium, and focus on electron transfer rather than proton transfer is far more useful.

8.A. Brønsted Theory

According to this theory, an acid is defined as a proton donor3 and a base as a proton acceptor. However, a base must have a pair of electrons available to share with the proton; this is usually present as an unshared pair, but sometimes is in a π orbital. By this definition, an acid–base reaction is the transfer of a proton from an acid to a base. However, protons do not exist free in solution, but must be attached to an electron pair. In fact, the acid does not “give up” a proton, but rather the base donates electrons to the proton, “pulling it away” to form the conjugate acid. After removal of the proton, the species remaining (the conjugate base) still retains the electron pair to which the proton was formerly attached. The conjugate base, in theory at least, can reacquire a proton and is therefore a base. All acids will generate a conjugate base upon reaction with a suitable base, and all bases will generate a conjugate acid by reaction with a suitable acid. All acid–base reactions fit the equation

No charges are shown in this equation, but an acid always has a charge one positive unit higher than that of its conjugate base.

8.A.i Brnsted Acids

According to the Brnsted definition, acid strength may be defined as the tendency to give up a proton and base strength as the tendency to accept a proton. All acid–base reactions are reversible, and both an acid and a conjugate acid are present in the equilibrium mixture. In one sense, acid–base reactions occur because the acid and the conjugate acid are not of equal strength (i.e., the equilibrium can be shifted to one side or the other). If an acid, say HCl, is placed in contact with the conjugate base of a weaker acid, say acetate ion, the conjugate acid in this reaction would be acetic acid. Since HCl is a stronger acid than acetic acid (see Table 8.1), the equilibrium lies well to the right. As the reaction is written, if the equilibrium lies to the right (higher concentration of acetic acid and a lower concentration of HCl), HCl is the stronger acid. Likewise, acetate is taken to be a stronger base than the chloride ion. If this is a correct statement, treatment of acetic acid with chloride ion should give essentially no reaction, since the weaker acid already has the proton. This is found to be correct.

Table 8.1 The pK_a Values for Many Types of Acids^a

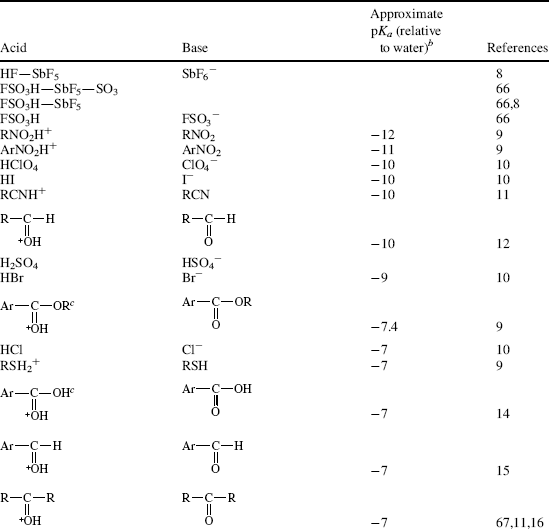
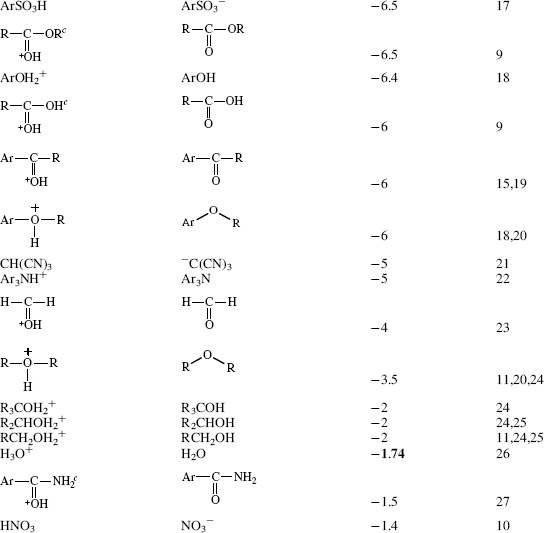
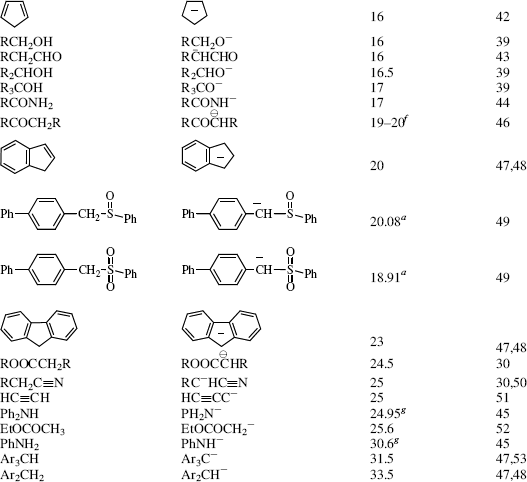
For a comparison of two different acids, the position of the equilibrium in reaction with a common base allows the relative strengths of acids to be determined.⁴ Likewise, the strength of two different bases will be determined by comparing the equilibrium established when they react with a common acid. By definition, the acid and base are always drawn on the left side of the equation, and the conjugate acid and conjugate base are assumed to be on the right side of the equation.

Of course, if the two acids involved are close to each other in strength, a measurable reaction will occur from both sides. This finding really means that the concentration of acid and base at equilibrium will be close to that of the concentration of the conjugate acid and conjugate base. However, the position of equilibrium will still be over to the side of the weaker acid (unless the acidities are equal within experimental limits). If the concentration of acid and base is higher, the reaction of conjugate acid and conjugate base is more facile, and the compound labeled as the acid is considered to be a weaker acid. If the concentration of the conjugate acid and conjugate base is higher, the reaction of the acid and base is more facile, and the compound labeled as the acid is a stronger acid.

Using these protocols as the definition of acid strength, it is possible to construct a table in which acids are listed in order of acid strength⁵ (Table 8.1).⁶ The conjugate base is shown next to each acid in Table 8.1. Using the axiom that a strong acid generates a weak conjugate base and a weak acid will generate a strong conjugate base, it is clear that if the acids in such a table are listed in decreasing order of acid strength, the bases must be listed in increasing order of base strength. The pK_a values⁷ in Table 8.1 are most accurate in the middle of the table.⁸–⁶⁷

The pK_a values are much harder to measure⁶⁸ for very strong and very weak acids, and these values must be regarded as approximate. If one did not have the pK_a values available, it can be determined experimentally that HClO₄ is a stronger acid than H₂SO₄. A mixture of HClO₄ and H₂SO₄ in 4-methyl-2-pentanone can be titrated to an HClO₄ end point without interference by H₂SO₄.⁶⁹ Similarly, HClO₄ can be shown to be stronger than HNO₃ or HCl. However, this is not quantitative, and the value of −10 in the table is not much more than an educated guess. The values for RNO₂H⁺, ArNO₂H⁺, HI, RCNH⁺ and RSH₂⁺ must also be regarded as highly speculative.⁷⁰ A wide variety of pK_a values have been reported for the conjugate acids of even such simple bases as acetone⁶⁷ (−0.24 to −7.2), diethyl ether (−0.30 to −6.2), ethanol (−0.33 to −4.8), methanol (−0.34 to −4.9), and 2-propanol (−0.35 to −5.2), depending on the method used to measure them.⁷¹ Very accurate values can be obtained only for acids weaker than hydronium ion and stronger than water.

A crystallographic scale of acidity has been developed, including the acidity of C–H compounds. Measuring the mean C–HO distances in crystal structures correlated well with conventional pK_a(DMSO) values,⁷² where DMSO is dimethyl sulfoxide. An ab initio study was able to correlate ring strain in strained hydrocarbons with hydrogen-bond acidity.⁷³ The kinetic acidity of aliphatic hydrocarbons has been determined.⁷⁴

The bottom portion of Table 8.1 consists of very weak acids (pK_a above that of water ≈15.8).⁷⁵ In most of these acids, the proton is lost from a carbon atom, and such acids are known as carbon acids. The pK_a values for such weak acids are often difficult to measure and are known only approximately. The methods used to determine the relative positions of these acids are discussed in Chapter 5.⁷⁶ The acidity of carbon acids is proportional to the stability of the carbanions that are their conjugate bases (see Sec. 5.B.i).

The extremely strong acids at the top of the table are known as superacids (see Sec. 5.A.ii).⁷⁷ The actual species present in the FSO₃H–SbF₅ mixture are probably H[SbF₅(SO₃F)] and H[SbF₂(SO₃F)₄.⁶⁶ The addition of SO₃ causes formation of the still stronger H[SbF₄(SO₃F)₂], H[SbF₃(SO₃F)₃], and H[(SbF₅)₂(SO₃F)].⁶⁶ There is a study of electrophilic intermediates that are generated in superacids⁷⁸ (also see Chapter 10).

By the use of tables (e.g., Table 8.1), it is possible to determine whether a given acid will react with a given base to give reasonable concentrations of the conjugate acid and base. For tables in which acids are listed in order of decreasing strength, the rule is that any acid will react with any base in the table that is below it but not with any above it.⁷⁹ The greater the separation in the table, the better the reaction. It must be emphasized that the order of acid strength in Table 8.1 separation applies when a given acid and base react without a solvent or, when possible, in water. In other solvents, the order may be greatly different (see Sec. 8.G). In the gas phase, where solvation effects are completely or almost completely absent, acidity orders may also differ greatly.⁸⁰ For example, in the gas phase, toluene is a stronger acid than water and tert-butoxide ion is a weaker base than methoxide ion⁸¹ (see also Sec. 8.G). It is also possible for the acidity order to change with temperature. For example, >50 °C the order of base strength is BuOH > H₂O > Bu₂O; from 1 to 50 °C the order is BuOH > Bu₂O > H₂O; while <1 °C the order becomes Bu₂O > BuOH > H₂O.⁸²

8.A.ii. Brnsted Bases

Basicity may be measured by a parameter known as proton affinity of an anion. The dissociation of a hydrogen ion for a molecule in the gas phase is called the proton affinity of the conjugate base.⁸³ A hydrogen-bond basicity scale has been developed that can be used to determine the relative basicity of molecules. Table 8.2 gives the pK_HB values for several common heteroatom-containing molecules.⁸⁴ This is obtained from the protonated form (conjugated acid) of the base in question. The larger the number, the more basic is that compound. The basicity of aliphatic amines has been calculated,⁸⁵ the ion-pair basicity of amines in THF⁸⁶ and in water⁸⁷ has been determined, and the basicity of pyridine was examined.⁸⁸ There are secondary deuterium isotope effects for measuring the basicity of secondary amines, and deuteration was found to increase the basicity.⁸⁹ Weaker bases have also been examined, and the basicity of carbonyl compounds in carbon tetrachloride has been determined.⁹⁷ Alkenes are weak bases⁹⁸ that react with strong acids (e.g., HCl or HBr, Reaction 15-02). Note that extremely twisted amides (Sec. 4.Q.ii) exhibit high basicity.⁹⁹

Table 8.2 The pK_HB Values for Many Types of Bases.

Base	Approximate pKHB	Reference
N-Methyl-2-piperidone	2.60	90
Et2NCONEt2	2.43	90
N-Methyl-2-pyrrolidinone	2.38	90
PhCONMe2	2.23	90
HCONMe2	2.10	90
PhCONHMe	2.03	90
18-crown-6	1.98	91
HCONHMe	1.96	90
Aniline	4.60	92
N-methylaniline	4.85	92
PhNHNH2	5.27	92
Ph(Me)NNH2	4.99	92
15-crown-5	1.82	91
12-crown-4	1.73	91
PhOCONMe2	1.70	90
Et2N–CN	1.63	93
Me2N–CN	1.56	93
δ-Valerolactone	1.43	94
Oxetane	1.36	91
γ-Butyrolactone	1.32	94
THF	1.28	91
Cyclopentanone	1.27	95
t-BuOMe	1.19	91
Acetone	1.18	95
MeCOOEt	1.07	95
1,4-Dioxane	1.03	91
Et2O	1.01	91
1,3-Dioxane	0.93	91
1-Methyloxirane	0.97	91
PhCOOMe	0.89	94
MeOCOOMe	0.82	94
PhCHO	0.78	95
Bu2O	0.75	91
HCOOEt	0.66	94
MeCHO	0.65	95
Me2NO2	0.41	96
MeNO2	0.27	96
PhNO2	0.30	96
Furan	−0.40	91

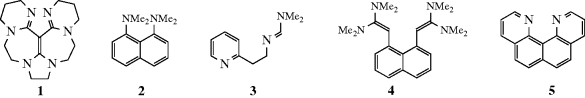
A class of organic compounds termed superbases has been developed.¹⁰⁰ Vinamidine type or Schwesinger proton sponges (see Sec. 8.F), 1,¹⁰¹ are dubbed superbases and are probably the most powerful organic neutral bases known. The pK_a (pK_BH⁺) in MeCN was measure as 31.94. It has been shown that the pK_a values of strong neutral organic (super)bases in acetonitrile are well described by the density functional theory.¹⁰² The fundamental type of proton sponge is 1,8-bis(dimethylamino)naphthalene (2, see Sec. 8.F), with a pK_BH⁺ of 18.18.¹⁰³ Other superbase-type compounds include amidinazines [e.g., N¹,N¹-dimethyl-N²-β-(2-pyridylethyl)-formamidine (3)], pK_BH⁺ in DMSO = 25.1,¹⁰⁴ 1,8-bis(tetramethylguanidino)naphthalene, (4),¹⁰⁵ and quinolino[7,8-h]quinolines (e.g., 5) with a pK_BH⁺ = 12.8.¹⁰⁶

It is important to note that organometallic compounds, such as Grignard reagents (RMgX) and organolithium reagents (RLi),¹⁰⁷ are powerful bases. The conjugate bases of both of these bases are alkanes, (R–H), which are very weak acids indeed (see Table 8.1).

8.B. The Mechanism of Proton-Transfer Reactions

Proton transfers between a base and an oxygen or nitrogen acid are usually extremely fast.108 Such reactions are generally diffusion controlled in the thermodynamically favored direction.¹⁰⁹ In fact, a normal acid is defined¹¹⁰ as one whose proton-transfer reactions are completely diffusion controlled, except when the conjugate acid of the base to which the proton is transferred has a pK value very close (differs by less than ~ 2 pK units) to that of the acid. The normal acid–base reaction mechanism consists of three steps:

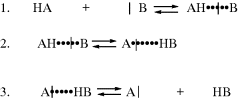
The actual proton transfer takes place in the second step the first step is formation of a hydrogen-bonded complex. The product of the second step is another hydrogen-bonded complex, which dissociates in the third step.

However, not all such proton transfers are diffusion controlled. For example, if an internal hydrogen bond exists in a molecule, reaction with an external acid or base is often much slower.¹¹¹ In a case such as 3-hydroxypropanoic acid, the ⁻OH ion can form a hydrogen bond with the acidic hydrogen only if the internal hydrogen bond breaks. Therefore only some of the collisions between ⁻OH ions and 3-hydroxypropanoic acid molecules result in proton transfer. In many collisions, the ⁻OH ions will come away “empty-handed”, resulting in a lower reaction rate. Note that this affects only the rate, not the equilibrium. Other systems are capable of hydrogen bonding (e.g., 1,2-diols). In the case of cyclohexane-1,2-diols, hydrogen bonding, ion–dipole interactions, polarizability, and stereochemistry all play a role in determining the acidity.¹¹² The presence of halogen atoms (e.g., chlorine) can lead to hydrogen-bonding effects.¹¹³ Another factor that can create lower rates is a molecular structure in which the acidic proton is protected within a molecular cavity (e.g., the in–in and out–in isomers shown in Sec. 4.L). See also the proton sponges mentioned in Section 8.F. Proton transfers between an acidic and a basic group within the same molecule can also be slow, if the two groups are too far apart for hydrogen bonding. In such cases, participation of solvent molecules may be necessary.

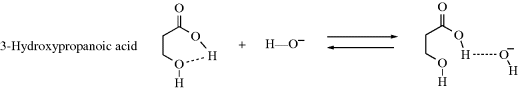
Proton transfers to or from a carbon atom¹¹⁴ in most cases are much slower than those strictly between oxygen or nitrogen atoms. At least three factors can be responsible for this,¹¹⁵ not all of them applying in every case.

1. Hydrogen bonding is very weak or altogether absent for carbon (Chap 3).

2. Loss of a proton from many carbon acids leads to carbanions that are stabilized by resonance. Calculations show that carbon acidity is influenced by coordination based on electrophile coordination geometry.¹¹⁶ Structural reorganization (movement of atoms to different positions within the molecule) may accompany this process. Chloroform, HCN, and 1-alkynes do not form resonance-stabilized carbanions, and these¹¹⁷ behave kinetically as normal acids.¹¹⁸ It has been reported that carborane acids [e.g., H(CHB₁₁H₅Cl₆)] are the strongest isolable (Lewis-free) Brnsted acids known.¹¹⁹

3. There may be considerable reorganization of solvent molecules around the ion as compared to the neutral molecule.¹²⁰

In connection with factors 2 and 3, it has been proposed¹¹⁵ that any factor that stabilizes the product (e.g., by resonance or solvation) lowers the rate constant if it develops late on the reaction coordinate, but increases the rate constant if it develops early. This is called the Principle of Imperfect Synchronization.

Mechanisms of proton transfer have been studied for many compounds, including the reactions of acids with lactams,¹²¹ amides with various bases,¹²² and amines with alkoxide bases.¹²³

8.C. Measurements of Solvent Acidity124

When a solute is added to an acidic solvent it may become protonated by the solvent. This effect can lead to an enhancement of acidity, as in the effect of using formic acid rather than methanol.¹²⁵ An acidity scale has been reported for ionic liquids¹²⁶ (see Sec. 9.D.iii for a discussion of ionic liquids), and the Lewis acidity of ionic liquids has been established using IR.¹²⁷ If the solvent is water and the concentration of solute is not very great, then the pH of the solution is a good measure of the proton-donating ability of the solvent. Unfortunately, this is no longer true in concentrated solutions because activity coefficients are no longer unity. A measurement of solvent acidity is needed that works in concentrated solutions and applies to mixed solvents as well. The Hammett acidity function¹²⁸ is a measurement that is used for acidic solvents of high dielectric constant.¹²⁹ For any solvent, including mixtures of solvents (but the proportions of the mixture must be specified), a value H₀ is defined as

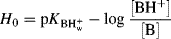
H₀ is measured by using “indicators” that are weak bases (B) and so are partly converted, in these acidic solvents, to the conjugate acids BH⁺. Typical indicators are o-nitroanilinium ion, with a pK in water of −0.29, and 2,4-dinitroanilinium ion, with a pK in water of −4.53. For a given solvent, [BH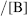⁺][B] is measured for one indicator, usually by spectrophotometric means and with the known pK in water (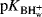) for that indicator, H₀ can be calculated for that solvent system. In practice, several indicators are used, so that an average H₀ is taken. Once H₀ is known for a given solvent system, pK_a values in it can be calculated for any other acid–base pair.

The symbol H₀ is defined as

where 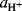is the activity of the proton and f_I and 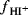are the activity coefficients of the indicator and conjugate acid of the indicator,¹³⁰ respectively. The parameter H₀ is related to H₀ by

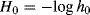
so that H₀ is analogous to pH and H₀ to [H⁺], and indeed in dilute aq solution H₀ = pH.

The parameter H₀ reflects the ability of the solvent system to donate protons, but it can be applied only to acidic solutions of high dielectric constant, mostly mixtures of water with acids (nitric, sulfuric, perchloric, etc.). It is apparent that the H₀ treatment is valid only when is independent of the nature of the base (the indicator). Since this is so only when the bases are structurally similar, the treatment is limited. Even when similar bases are compared, many deviations are found.¹³¹ Other acidity scales¹³² have been set up, including a scale for C–H acids,¹³³ among them H₋ for bases with a charge of −1, H_R for aryl carbinols,¹³⁴H_C for bases that protonate on carbon,¹³⁵ and H_A for unsubstituted amides.¹³⁶ It is now clear that there is no single acidity scale that can be applied to a series of solvent mixtures, irrespective of the bases employed.¹³⁷

Although most acidity functions have been applied only to acidic solutions, some work has also been done with strongly basic solutions.¹³⁸ The H₋ function, which is used for highly acidic solutions when the base has a charge of −1, can also be used for strongly basic solvents, in which case it measures the ability of these solvents to abstract a proton from a neutral acid (BH).¹³⁹ When a solvent becomes protonated, its conjugate acid is known as a lyonium ion.

Another approach to the acidity function problem was proposed by Bunnett et al.,¹⁴⁰ who derived the equation

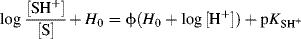
where S is a base that is protonated by an acidic solvent. Thus the slope of a plot of log ([SH⁺]/[S]) + H₀ against H₀ + log [H⁺] is the parameter ϕ, while the intercept is the pK_a of the lyonium ion (SH⁺, referred to infinite dilution in water). The value of ϕ expresses the response of the equilibrium

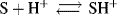
to changing acid concentration. A negative ϕ indicates that the log of the ionization ratio [SH⁺]/[S] increases, as the acid concentration increases, more rapidly than −H₀. A positive ϕ value indicates the reverse. The Bunnett–Olsen equation given above is a linear free–energy relationship (see Sec. 9.C) that pertains to acid–base equilibria. A corresponding equation that applies to kinetic data is

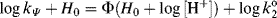
where k_ψ is the pseudo-first-order rate constant for a reaction of a weakly basic substrate taking place in an acidic solution and 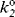is the second-order rate constant at infinite dilution in water. In this case, ϕ characterizes the response of the reaction rate to changing acid concentration of the solvent. The Bunnett–Olsen treatment has also been applied to basic media, where, in a group of nine reactions in concentrated NaOMe solutions, no correlation was found between reaction rates and either H₋ or stoichiometric base concentration, but where the rates were successfully correlated by a linear free energy equation similar to those given above.¹⁴¹

A treatment partially based on the Bunnett–Olsen treatment is that of Bagno et al.,¹⁴² which formulates medium effects (changes in acidity of solvent) on acid–base equilibria. An appropriate equilibrium is chosen as reference, and the acidity dependence of other reactions compared with it, by use of the linear free energy equation

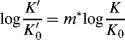
where the K values are the equilibrium constants for the following: K for the reaction under study in any particular medium; K′ for the reference reaction in the same medium; K₀ for the reaction under study in a reference solvent; 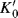for the reference reaction in the same reference solvent; and m^∗ is the slope of the relationship [corresponding to (1 − ϕ) of the Bunnett–Olsen treatment]. This equation has been shown to apply to many acid–base reactions.

Another type of classification system was devised by Bunnett¹⁴³ for reactions occurring in moderately concentrated acid solutions. Log k_ψ + H₀ is plotted against log , where K_ψ is the pseudo-first-order rate constant for the protonated species and 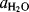is the activity of water. Most such plots are linear or nearly so. According to Bunnett, the slope of this plot w tells something about the mechanism. Where w is between −2.5 and 0, water is not involved in the rate-determining step; where w is between 1.2 and 3.3, water is a nucleophile in the rate-determining step; where w is between 3.3 and 7, water is a proton-transfer agent. These rules hold for acids in which the proton is attached to oxygen or nitrogen.

A new acidity scale has been developed based on calorimetric measurement of N-methylimidazole and N-methylpyrrole in bulk solvents.¹⁴⁴ A revised version of this method was shown to give better results in some cases.¹⁴⁵ Another scale of solvent acidities was developed based on the hydrogen-bond donor acidities in aq DMSO.¹⁴⁶ Note that bond energies, acidities, and electron affinities are related in a thermodynamic cycle, and Fattahi and Kass¹⁴⁷ show that by measuring two of these quantities the third can be found.

8.D. Acid and Base Catalysis148

Many reactions are catalyzed by acids or bases. Some are catalyzed by both acids and bases. In such cases, the catalyst is involved in a fundamental way in the mechanism. The first step of such a reaction is nearly always a proton transfer between the catalyst and the substrate.

Reactions can be catalyzed by acid or base in two different ways, called general and specific catalysis. If the rate of an acid-catalyzed reaction run in a solvent (S) is proportional to its conjugate acid [SH⁺], the reaction is said to be subject to specific acid catalysis, the acid being the lyonium ion (SH⁺). The acid that is put into the solvent may be stronger or weaker than SH⁺, but the rate is proportional only to the [SH⁺] that is actually present in the solution derived from the equilbrium

The identity of HA is important only to the extent that it determines the position of equilibrium, and hence the [SH⁺]. Most measurements have been made in water, where SH⁺ is H₃O⁺.

In general acid catalysis, the rate is increased not only by an increase in [SH⁺], but also by an increase in the concentration of other acids (e.g., in water by phenols or carboxylic acids). These other acids increase the rate even when [SH⁺] is held constant. In this type of catalysis the strongest acids catalyze best, so that, in the example given, an increase in the phenol concentration catalyzes the reaction much less than a similar increase in [H₃O⁺]. This relationship between acid strength of the catalyst and its catalytic ability can be expressed by the Brnsted catalysis equation¹⁴⁹

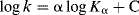
where k is the rate constant for a reaction catalyzed by an acid of ionization constant K_α. According to this equation, when log k is plotted against log K_α for catalysis of a given reaction by a series of acids, a straight line should be obtained with slope and intercept C. Straight lines are obtained in many cases, but not always. The relationship usually fails when acids of different types are compared. For example, it is much more likely to hold for a group of substituted phenols than for a collection of acids that contains both phenols¹⁵⁰ and carboxylic acids. The Brnsted equation is another linear free energy relationship (see Sec. 9.C).

Analogously, there are general and specific (S⁻ from an acidic solvent SH) base-catalyzed reactions. The Brnsted law for bases is

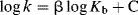
The Brnsted equations relate a rate constant k to an equilibrium constant K_a. In Chapter 6, the Marcus equation was seen to relate a rate term (in that case ΔG^‡) to an equilibrium term (ΔG°). When the Marcus treatment is applied to proton transfers¹⁵¹ between a carbon and an oxygen (or a nitrogen), the simplified¹⁵² equation (Sec. 6.I)

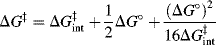
where

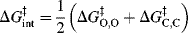
can be further simplified: Because proton transfers between oxygen and oxygen (or nitrogen and nitrogen) are much faster than those between carbon and carbon, is much smaller than 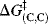and one can write¹⁵³

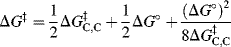
Thus, if the carbon part of the reaction is kept constant and only the A of HA is changed (where A is an oxygen or nitrogen moiety), then ΔG^‡ is dependent only on ΔG°. Differentiation of this equation yields the Brnsted α:

The Brnsted law is therefore a special case of the Marcus equation.

A knowledge of whether a reaction is subject to general or specific acid catalysis supplies information about the mechanism. For any acid-catalyzed reaction we can write

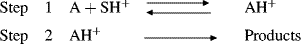
If the reaction is catalyzed only by the specific acid SH⁺, it means that step 1 is rapid and step 2 is rate controlling. This means that an equilibrium has been rapidly established between A and the strongest acid present in the solution, namely, SH⁺ (since this is the strongest acid that can be present in S). On the other hand, if step 2 is faster, there is no time to establish equilibrium and the rate-determining step must be step 1. This step is affected by all the acids that may be present, and the rate reflects the sum of the effects of each acid (general acid catalysis). General acid catalysis is also observed if the slow step is the reaction of a hydrogen-bond complex (AHB), since each complex reacts with a base at a different rate. A comparable discussion can be used for general and specific base catalysis.¹⁵⁴ Further information can be obtained from the values α and β in the Brnsted catalysis equations, since these are approximate measures of the extent of proton transfer in the transition state. In most cases, values of α and β are between 1 and 0. A value of α or β near 0 is generally taken to mean that the transition state resembles the reactants; that is, the proton has been transferred very little when the transition state has been reached. A value of α or β near 1 is taken to mean the opposite; that is, in the transition state the proton has been almost completely transferred. However, cases are known in which these generalizations are not followed,¹⁵⁵ and their theoretical basis has been challenged.¹⁵⁶ In general, the proton in the transition state lies closer to the weaker base.

8.E. Lewis Acids and Bases

At about the same time that Brnsted proposed his acid–base theory, Lewis put forth a broader theory. A base in the Lewis theory is the same as in the Brnsted one, namely, a compound with an available pair of electrons, either unshared or in a π orbital. However, a Lewis base donates electrons to an atom other than H or C.157 A Lewis acid is any species with a vacant orbital.¹⁵⁸ In a Lewis acid–base reaction, the unshared pair of the base forms a covalent bond with the vacant orbital of the acid, as represented by the general equation

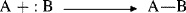
in which charges are not shown, since they may differ. A specific example is

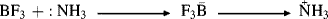
In the Brnsted picture, the acid is a proton donor, but in the Lewis picture the proton itself is the acid since it has a vacant orbital. A Brnsted acid becomes, in the Lewis picture, the compound that gives up the actual acid. The advantage of the Lewis theory is that it correlates the behavior of many more processes. For example, AlCl₃ and BF₃ are Lewis acids because they have only six electrons in the outer shell and have room for eight. Lewis acids SnCl₄ and SO₃ have eight, but their central elements, not being in the first row of the periodic table, have room for 10 or 12. Other Lewis acids are simple cations, like Ag⁺. The simple reaction is not very common in organic chemistry, but the scope of the Lewis picture is much larger because reactions of the types shown here, which are very common in organic chemistry, are also Lewis acid–base reactions. In fact, all reactions in which a covalent bond is formed through one species contributing a filled and the other a vacant orbital may be regarded as Lewis acid–base reactions. An ab initio analysis of the factors that determine Lewis versus Lowry–Brnsted acidity–basicity is available.¹⁵⁹

When a Lewis acid combines with a base to give a negative ion in which the central atom has a higher than normal valence, the resulting salt is called an ate complex.¹⁶⁰ Examples are

Ate complexes are analogous to the onium salts formed when a Lewis base expands its valence, for example,

Far fewer quantitative measurements have been made of Lewis acid strength compared to that of Brnsted acids.¹⁶¹ A simple table of Lewis acidities based on some quantitative measurement (e.g., that given for Brnsted acids in Table 8.1) is not feasible because Lewis acidity depends on the nature of the base and any solvent that can function as a base. For example, lithium perchlorate functions as a weak Lewis acid in ether.¹⁶² Qualitatively, the following approximate sequence of acidity of Lewis acids of the type MX_n has been suggested, where X is a halogen atom or an inorganic radical: BX₃ > AlX₃ > FeX₃ > GaX₃ > SbX₅ > SnX₄ > AsX₅ > ZnX₂ > HgX₂.

8.E.i Hard–Soft Acids–Bases

The facility with which an acid–base reaction takes place depends, of course, on the strengths of the acid and the base. But it also depends on quite another quality, called the hardness¹⁶³ or softness of the acid or base.¹⁶⁴ Hard and soft acids and bases have these characteristics:

Soft Bases. The donor atoms are of low electronegativity and high polarizability, and are easy to oxidize. They hold their valence electrons loosely.

Hard Bases. The donor atoms are of high electronegativity and low polarizability, and are hard to oxidize. They hold their valence electrons tightly.

Soft Acids. The acceptor atoms are large, have low positive charge, and contain unshared pairs of electrons (p or d) in their valence shells. They have high polarizability and low electronegativity.

Hard Acids. The acceptor atoms are small, have high positive charge, and do not contain unshared pairs in their valence shells. They have low polarizability and high electronegativity.

A qualitative listing of the hardness of some acids and bases is given in Table 8.3.¹⁶⁵ The treatment has also been made quantitative,¹⁶⁶ with the following operational definition:

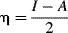
In this equation, η, the absolute hardness, is half the difference between I, the ionization potential, and A, the electron affinity.¹⁶⁷ The softness (σ), is the reciprocal of η. Values of η for some molecules and ions are given in Table 8.4.¹⁶⁸ Note that the proton, which is involved in all Brnsted acid–base reactions, is the hardest acid listed, with η = ∞ (it has no ionization potential). The above equation cannot be applied to anions, because electron affinities cannot be measured for them. Instead, the assumption is made that η for an anion X⁻ is the same as that for the radical X^•.¹⁶⁹ Other methods are also needed to apply the treatment to polyatomic cations.¹⁶⁹

Table 8.3 Hard and Soft Acids and Bases^a

a. See Ref. 165.

Once acids and bases have been classified as hard or soft, a simple rule can be given: hard acids prefer to bond to hard bases, and soft acids prefer to bond to soft bases [the HSAB (hard–soft acid–base) principle].¹⁷⁰ The rule has nothing to do with acid or base strength but merely says that the product A–B will have extra stability if both A and B are hard or if both are soft. Another rule is that a soft Lewis acid and a soft Lewis base tend to form a covalent bond, while a hard acid and a hard base tend to form ionic bonds.

One application of the first rule given above is found in complexes between alkenes or aromatic compounds and metal ions (see above). Alkenes and aromatic rings are soft bases and should prefer to complex with soft acids. Thus, Ag⁺, Pt²⁺, and Hg²⁺ complexes are common, but complexes of Na⁺, Mg²⁺, or Al³⁺ are rare. Chromium complexes are also common, but in such complexes the chromium is in a low or zero oxidation state (which softens it) or attached to other soft ligands. Another application is the reaction:

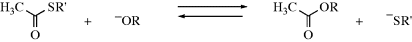
The HSAB principle predicts that the equilibrium should lie to the right, because the hard acid CH₃CO⁺ should have a greater affinity for the hard base (RO⁻) than for the soft base (RS⁻). Indeed, thiol esters are easily cleaved by RO⁻ or hydrolyzed by dilute base (⁻OH is also a hard base).¹⁷¹ Another application of the rule is discussed in Section 10.G.ii.¹⁷² The HSAB principles have been applied to analyze the reactivity of ketone and ester enolate anions,¹⁷³ and in analyzing catalyst selectivity in synthesis.¹⁷⁴

8.F. The Effects of Structure on the Strengths of Acids and Bases175

The structure of a molecule can affect its acidity or basicity in a number of ways. Unfortunately, in most molecules two or more of these effects (as well as solvent effects) are operating, and it is usually very difficult or impossible to say how much each effect contributes to the acid or base strength.¹⁷⁶ Small differences in acidity or basicity between similar molecules are particularly difficult to interpret. It is well to be cautious when attributing them to any particular effect.

1. Field Effects. These effects were discussed in Section 1.I. In general, changes in substituents can have an effect on acidity. As an example of the influence of field effects on acidity, compare the acidity of acetic acid and 2-nitroacetic acid:

The only difference in the structure of these molecules is the substitution of NO₂ for H. Since NO₂ is a strongly electron-withdrawing group, it withdraws electron density from the negatively charged COO⁻ group in the anion of 2-nitroacetic acid (compared with the anion of acetic acid). As the pK_a values indicate, 2-nitroacetic acid is ~1000 times stronger than acetic acid.¹⁷⁷ Any effect that results in electron withdrawal from a negatively charged center (−I effect) is a stabilizing effect because it spreads the charge. Thus, −I groups increase the acidity of uncharged acids (e.g., acetic) because they spread the negative charge of the anion. However, −I groups also increase the acidity of any acid, no matter what the charge. For example, if the acid has a charge of +1 (and its conjugate base is therefore uncharged), a −I group destabilizes the positive center (by increasing and concentrating the positive charge) of the acid, a destabilization that will be relieved when the proton is lost. In general, groups that withdraw electrons by the field effect increase acidity and decrease basicity, while electron-donating groups act in the opposite direction. Another example is the molecule (C₆F₅)₃CH, which has three strongly electron-withdrawing C₆F₅ groups and a pK_a of 16,¹⁷⁸ compared with Ph₃CH, with a pK_a of 31.5 (Table 8.1), an acidity enhancement of ~ 10¹⁵. Table 8.5 shows pK_a values for some acids. An approximate idea of field effects can be obtained from this table. In the case of the chlorobutyric acids, the effect decreases with distance. It must be remembered, however, that field effects are not the sole cause of the acidity differences noted and that in fact solvation effects may be more important in many cases (see Sec. 8.G).¹⁷⁹ The influence of various substituents on the acidity of acetic acid has been calculated,¹⁸⁰ Substituent effects for weak acids (e.g., phenols and benzyl alcohols) have been discussed.¹⁸¹

Field effects are important in benzoic acid derivatives, and the pK_a of the acid will vary with the nature and placement of the “X” group in 6.¹⁸² The pK_a of 3-OMe (6) is 5.55, but 4-OMe (6) is 6.02 in 50% aq methanol,¹⁸³ compared with a pK_a of 5.67 when X = H. When X = 4-NO₂, the pK_a is 4.76 and 4-Br is 5.36.¹⁷² The pK_a of 2,6-diphenylbenzoic acid is 6.39.¹⁸⁴

2. Resonance Effects. Resonance that stabilizes a base, but not its conjugate acid, results in the acid having a higher acidity than otherwise expected and vice versa. An example is found in the higher acidity of carboxylic acids¹⁸⁵ compared with primary alcohols.

The RCOO⁻ ion is stabilized by resonance not available to the RCH₂O⁻ ion (or to RCOOH).¹⁸⁶ Note that the RCOO⁻ is stabilized not only by the fact that there are 2 equiv canonical forms, but also by the fact that the negative charge is spread over both oxygen atoms, and is therefore less concentrated than in RCH₂O⁻. The same effect is found in other compounds containing a C=O or CN group. Thus amides (RCONH₂) are more acidic than amines (RCH₂NH₂); esters (RCH₂COOR′) more than ethers (RCH₂CH₂OR′); and ketones (RCH₂COR′) more than alkanes (RCH₂CH₂R′) (Table 8.1). The effect is enhanced when two carbonyl groups are attached to the same carbon (because of additional resonance and spreading of charge); for example, β-keto esters (see 7) are more acidic than simple ketones or carboxylic esters (Table 8.1). Compounds such as (7) are generically referred to as active methylene compounds (X–CH₂–X), where X is an electron-withdrawing groups (a carbonyl, cyano, sulfonyl, etc.).¹⁸⁷ The influence of substituents in the α-position of substituted ethyl acetate derivatives has been studied.¹⁸⁸ Extreme examples of this effect are found in the molecules tricyanomethane [(NC)₃CH], with a pK_a of −5 (Table 8.1), and 2-(dicyanomethylene)-1,1,3,3-tetracyanopropene (NC)₂C=C[CH(CN)₂]₂, whose first pK_a is below −8.5 and whose second pK_a is −2.5.

Resonance effects are also important in aromatic amines. m-Nitroaniline is a weaker base than aniline, a fact that can be accounted for by the −I effect of the nitro group. But p-nitroaniline is weaker still, though the −I effect should be less because of the greater distance. This result is obtained by taking the canonical form A into account. Because A contributes to the resonance hybrid,¹⁸⁹ the electron density of the unshared pair is lower in p-nitroaniline than in m-nitroaniline, where a canonical form (e.g, A) is impossible. Note that the pK_a values reported are those of the conjugate acid, the ammonium ion.¹⁹⁰ The basicity is lower in the para compound for two reasons, both caused by the same effect: (1) the unshared pair is less available for attack by a proton, and (2) when the conjugate acid is formed, the resonance stabilization afforded by A is no longer available because the previously unshared pair is now being shared by the proton. The acidity of phenols is affected by substituents in a similar manner.¹⁹¹

In general, resonance effects lead to the same result as field effects. That is, here too, electron-withdrawing groups increase acidity and decrease basicity, and electron-donating groups act in the opposite manner. As a result of both resonance and field effects, charge dispersal leads to greater stability.

3. Periodic Table Correlations. When comparing Brnsted acids and bases that differ in the position of an element in the periodic table:

a. Acidity increases and basicity decreases in going from left to right across a row of the periodic table. Thus acidity increases in the order CH₄ < NH₃ < H₂O < HF, and basicity decreases in the order ⁻CH₃ > ⁻NH₂ > ⁻OH > F⁻. This behavior can be explained by the increase in electronegativity upon going from left to right across the table. It is this effect that is responsible for the great differences in acidity between carboxylic acids, amides, and ketones: RCOOH RCONH₂ RCOCH₃.

b. Acidity increases and basicity decreases in going down a column of the periodic table, despite the decrease in electronegativity. Thus acidity increases in the order HF < HCl < HBr < HI and H₂O < H₂S, and basicity decreases in the order NH₃ > PH₃ > AsH₃. This behavior is related to the size of the species involved. Thus, for example, F⁻, which is much smaller than I⁻, attracts a proton much more readily because its negative charge occupies a smaller volume, and is therefore more concentrated (note that F⁻ is also much harder than I⁻ and is thus more attracted to the hard proton; see Sec. 8.E). This rule does not always hold for positively charged acids. Thus, although the order of acidity for the group 16 hydrides is H₂O < H₂S < H₂Se, the acidity order for the positively charged ions is H₃O⁺ > H₃S⁺ > H₃Se⁺.¹⁹²

Lewis acidity is also affected by periodic table considerations. In comparing acid strengths of Lewis acids of the form MX_n¹⁶¹:

c. Acids that require only one electron pair to complete an outer shell are stronger than those that require two. Thus GaCl₃ is stronger than ZnCl₂. This results from the relatively smaller energy gain in adding an electron pair that does not complete an outer shell and from the buildup of negative charge if two pairs come in.

d. Other things being equal, the acidity of MX_n decreases in going down the periodic table because as the size of the molecule increases, the attraction between the positive nucleus and the incoming electron pair is weaker. Thus BCl₃ is a stronger acid than AlCl₃.¹⁹³

4. Statistical Effects. In a symmetrical diprotic acid, the first dissociation constant is twice as large as expected since there are 2 equiv ionizable hydrogens, while the second constant is only one-half as large as expected because the conjugate base can accept a proton at 2 equiv sites. So K₁/K₂ should be 4, and approximately this value is found for dicarboxylic acids where the two groups are sufficiently far apart in the molecule that they do not influence each other. A similar argument holds for molecules with 2 equiv basic groups.¹⁹⁴

5. Hydrogen Bonding. Internal hydrogen bonding can greatly influence acid or base strength. For example, the pK for o-hydroxybenzoic acid is 2.98, while the value for the para isomer is 4.58. Internal hydrogen bonding between the ⁻OH and COO⁻ groups of the conjugate base of the ortho isomer stabilizes it and results in an increased acidity.

6. Steric Effects. The proton itself is so small that direct steric hindrance is seldom encountered in proton transfers. Steric effects are much more common in Lewis acid–base reactions in which larger acids are used. Spectacular changes in the order of base strength have been demonstrated when the size of the acid was changed. Table 8.6 shows the order of base strength of simple amines when compared against acids of various size.¹⁹⁵ It can be seen that the usual order of basicity of amines (when the proton is the reference acid) can be completely inverted by using a large enough acid. The strain caused by formation of a covalent bond when the two atoms involved each have three large groups is called face strain or F strain.

Steric effects can indirectly affect acidity or basicity by affecting the resonance (Sec. 2.F). For example, o-tert-butylbenzoic acid is ~ 10 times as strong as the para isomer, because the carboxyl group is forced out of the plane by the tert-butyl group. Indeed, virtually all ortho benzoic acids are stronger than the corresponding para isomers, regardless of whether the group on the ring is electron donating or electron withdrawing.

Steric effects can also be caused by other types of strain. 1,8-Bis(diethylamino)-2,7-dimethoxynaphthalene (8) is an extremely strong base for a tertiary amine (pK_a of the conjugate acid = 16.3; cf. N,N-dimethylaniline, pK_a = 5.1), but proton transfers to and from the nitrogen are exceptionally slow; slow enough to be followed by a UV spectrophotometer.¹⁹⁶ Compound 8 is severely strained because the two nitrogen lone pairs are forced to be near each other.¹⁹⁷ Protonation relieves the strain: one lone pair is now connected to a hydrogen, which forms a hydrogen

bond to the other lone pair (shown in 9). The same effects are found in 4,5-bis(dimethylamino)fluorene (10)¹⁹⁸ and 4,5-bis(dimethylamino)phenanthrene (11).¹⁹⁹ Compounds (e.g., 8, 10, and 11), are known as proton sponges.²⁰⁰ The basicity of a proton sponge has been calculated as the sum of the proton affinity¹⁵² of an appropriate reference monoamine, the strain released on protonation, and the energy of the intramolecular hydrogen bond formed on protonation.²⁰¹ Another type of proton sponge is quino[7,8-h]quinoline (12).²⁰² Protonation of this compound also gives a stable mono protonated ion similar to 9, but the steric hindrance found in 8, 10, and 11 is absent. Therefore, 12 is a much stronger base than quinoline (13) (pK_a values of the conjugate acids are 12.8 for 12 and 4.9 for 13), but proton transfers are not abnormally slow. A cyclam-like macrocyclic tetramine (15) was prepared by a coupling reaction of bispidine, and was shown to be a new class of proton sponge.²⁰³

Chiral Lewis acids are known. Indeed, an air stable and storable chiral Lewis acid catalyst has been prepared, a chiral zirconium catalyst combined with molecular sieves powder.²⁰⁴ Association of a bulky silicon group with the bis(trifluoromethanesulfonyl)imide (known as triflimide) anion leads to enhancement of the electrophilic character of R₃SiNTf₂. The presence of a chiral substituent derived from (−)-myrtenal on the silicon atom led to a chiral silicon Lewis acid.²⁰⁵

Another type of steric effect is the result of an entropy effect. The compound 2,6-di-tert-butylpyridine is a weaker base than either pyridine or 2,6-dimethylpyridine.²⁰⁶ The reason is that the conjugate acid 14 is less stable than the conjugate acids of nonsterically hindered pyridines. In all cases, the conjugate acids are hydrogen bonded to a water molecule, but in the case of 14 the bulky tert-butyl groups restrict rotations in the water molecule, lowering the entropy.²⁰⁷

The conformation of a molecule can also affect its acidity. The following pK_a values were determined for compounds 16–19.²⁰⁸

Since ketones are stronger acids than carboxylic esters (Table 8.1), it is not surprising that 16 is a stronger acid than 18.²⁰⁹ A comparison of 16 with cyclic diketone 17 shows an increase in acidity of only 2.1 pK units, while a comparison of 18 with cyclic diester 19 shows an increase of 8.6 units. Indeed, 19 (called Meldrum's acid) is an unusually strong acid for a 1,3-diester. In order to account for this very large effect of a ring, MO calculations were carried out for two conformations of methyl acetate and of its enolate ion.²¹⁰ Loss of a proton is easier by ~5 kcal mol⁻¹ (21 kJ mol⁻¹) for the syn than for the anti conformer of the ester. In an acyclic molecule like 18, the preferred conformations are anti, but in Meldrum's acid (19) the conformation on both sides is constrained to be syn.

Facial differences in proton reactivity can lead to enantioselective deprotonation. Enantioselective deprotonation is also achieved by using a chiral base and/or a chiral complexing agent. Enantioselective deprotonation in cyclic ketones,²¹¹ and with heterodimer bases has been studied.²¹² When a Lewis acid coordinates to a base, the resulting complex can have conformational properties that influence reactivity. Coordination of SnCl₄ with aldehydes and esters, for example, leads to a complex where the conformation is determined by interactions of the C=O SnCl₄ unit with substituents attached to the carbonyl.²¹³

7. Hybridization. An s orbital has a lower energy than a p orbital. Therefore, the more s character a hybrid orbital contains, the lower the energy of that orbital. It follows that a carbanion at an sp carbon is more stable than a corresponding carbanion at an sp² carbon. Thus HCC⁻, which has more s character in its unshared pair than CH₂=CH⁻ or CH₃CH₂⁻ (sp vs sp² vs sp³, respectively), is a much weaker base. This explains the relatively high acidity of acetylenes and HCN. Another example is that alcohol and ether oxygen atoms, where the unshared pair is sp³, are more strongly basic than carbonyl oxygen atoms, where the unshared pair is sp² (Table 8.1).

An understanding of the reactivity of bases arises from the study of their structures in solution and in the crystalline state. Due to the importance of dialkylamide bases, there is a significant body of work, led by independent work by Williard and by Collum, that has attempted to understand the structures of these reactive molecules. It is clear that the dialkylamide bases are aggregates. Note that the simplest member of the amide base family, lithium amide (LiNH₂), was shown to be monomeric and unsolvated, as determined using a combination of gas-phase synthesis and millimeter/submillimeter-wave spectroscopy.²¹⁴ Both monomeric LiNH₂ and LiNMe₂ are planar.²¹⁵ Lithium diisopropylamide (LiNiPr₂, LDA) was isolated from a THF solution and X-ray crystallography revealed a dimeric structure (20; R = iPr, S = THF) in the solid state.²¹⁶ Lithium diisopropylamide was also shown to be a dimer in solutions of THF²¹⁷ and/or HMPA (see 20, R = iPr and S = THF, HMPA).²¹⁸ In the presence of HMPA, many derivatives of 20 tend to be mixed aggregates.²¹⁹ Extremely hindered LiNR₂ (R = 2-adamantyl) are monomeric under all conditions.²²⁰ In hydrocarbon solvents, lithium tetramethylpiperidide [LTMP, RR′NLi, where RR′ = –CMe₂(CH₂)₃C(Me₂)–] forms cyclic trimers and tetramers, with the tetrameric species predominating.²²¹ In THF, lithium hexamethyldisilazide [LHMDS, (Me₃Si)₂NLi] forms a five-coordinate tetrasolvate [(Me₃Si)₂NLi(thf)₄],²²² but in ether there is an equilibrium mixture of monomer and dimer.²²³ A review is available that discusses the solution structures of amide bases LiNR₂.²²⁴ Chiral lithium amide bases are known and they show similar behavior in solution.²²⁵ Chelation effects are common in enantioenriched amide bases, which also form aggregates.²²⁶ The aggregation state of lithium phenylacetonitrile has been studied.²²⁷ Dianion aggregates can be generated, and in the case of the lithiation reaction of N-silyl allylamine, X-ray structure determination showed the presence of three uniquely different aggregates.²²⁸ A mixed aggregate is formed when the lithium enolate of a ketone is mixed with a lithium amide.²²⁹

Similar information is available for other bases. Lithium phenoxide (LiOPh) is a tetramer in THF.²³⁰ Lithium 3,5-dimethylphenoxide is a tetramer in ether, but addition of HMPA leads to dissociation to a monomer.²³¹

Enolate anions are nucleophiles in reactions with alkyl halides (Reaction 10-68), with aldehydes and ketones (Reactions 16-34 and 16-36) and with acid derivatives (Reaction 16-85). Enolate anions are also bases, reacting with water, alcohols and other protic solvents, and even the carbonyl precursor to the enolate anion. Enolate anions exist as aggregates, and the effect of solvent on aggregation and reactivity of lithium enolate anions has been studied.²³² Alkyl substitution has a significant influence on the energetics of enolate anions.²³³

Table 8.4 Some Absolute Hardness Values in Electron Volts^a

Table 8.5 The pK Values for Some Acids^a

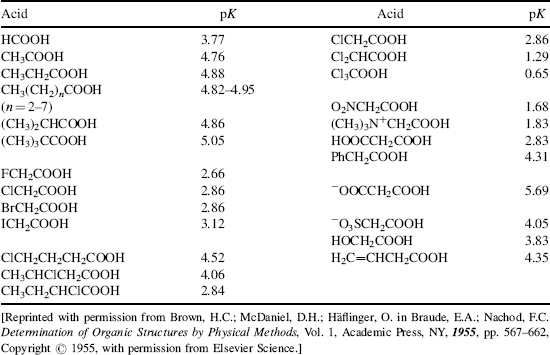
a. See Ref. 47.

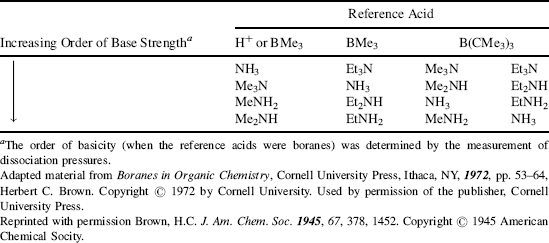
Table 8.6 Bases Listed in Increasing Order of Base Strength when Compared with Certain Reference Acids.

8.G. The Effects of the Medium on Acid and Base Strength

Structural features are not the only factors that affect acidity or basicity. The same compound can have its acidity or basicity changed when the reaction conditions are changed. The effect of temperature (Sec. 8.A) has already been mentioned. More important is the effect of the solvent, which can exert considerable influence on acid and base strengths by differential solvation.234 If a base is more solvated than its conjugate acid, its stability is increased relative to the conjugate acid. For example, Table 8.6 shows that in reactions with a proton, where steric effects are absent, methylamine is a stronger base than ammonia and dimethylamine is stronger still.²³⁵ These results are easily explainable if one assumes that methyl groups are electron donating. However, trimethylamine, which should be even stronger, is a weaker base than dimethylamine or methylamine. This apparently anomalous behavior can be explained by differential hydration.²³⁶ Thus, NH₄⁺ is much better hydrated (by hydrogen bonding to the water solvent) than NH₃ because of its positive charge.²³⁷ It has been estimated that this effect contributes ~11 pK units to the base strength of ammonia.²³⁸ When methyl groups replace hydrogen, this difference in hydration decreases²³⁹ until, for trimethylamine, it contributes only ~6 pK units to the base strength.¹⁹⁵ Thus two effects act in opposite directions, the field effect increasing the basicity as the number of methyl groups increases and the hydration effect decreasing it. Taken together, the strongest base is dimethylamine and the weakest is ammonia in solution. If alkyl groups are electron donating, one would expect that in the gas phase,²⁴⁰ where the solvation effect does not exist, the basicity order of amines toward the proton should be R₃N > R₂NH > RNH₂ > NH₃, and this has indeed been confirmed, for R = Me, as well as R = Et and Pr.²⁴¹ Aniline too, in the gas phase, is a stronger base than NH₃,²⁴² so its much lower basicity in aqueous solution (pK_a of PhNH₃⁺ 4.60 compared with 9.24 for aq NH₄⁺) is caused by similar solvation effects and not by resonance and field electron-withdrawing effects of a phenyl group. Similarly, pyridine²⁴³ and pyrrole²⁴⁴ are both much less basic than NH₃ in aqueous solution (pyrrole²⁴⁵ is neutral in aqueous solution), but more basic in the gas phase. Care must be taken in attributing relative acidities or basicities to any particular effect. Solvent has a significant influence on the Hammett reaction constant (Sec. 11.D), which influences the acidity of substituted benzoic acids.²⁴⁶

In the case of Lewis acids, protic solvents (e.g., water or alcohol) can strongly influence their reactivity, cause it to react via an alternative path to the one desired, or even cause decomposition. Rare earth metal triflates have been used to develop water tolerant Lewis acids that can be used in many organic reactions.²⁴⁷

For simple alcohols, the order of gas-phase acidity is completely reversed from that in aqueous solution. In solution, the acidity is in the order H₂O > MeCH₂OH > Me₂CHOH > Me₃COH, but in the gas phase the order is precisely the opposite.²⁴⁸ Once again solvation effects can be invoked to explain the differences. Comparing the two extremes, H₂O and Me₃COH, we see that the OH⁻ ion is very well solvated by water while the bulky Me₃CO⁻ is much more poorly solvated because the water molecules cannot get as close to the oxygen. Thus in solution H₂O gives up its proton more readily. When solvent effects are absent, however, the intrinsic acidity is revealed and Me₃COH is a stronger acid than H₂O. This result demonstrates that simple alkyl groups cannot be simply regarded as electron donating. If methyl is an electron-donating group, then Me₃COH should be an intrinsically weaker acid than H₂O, yet it is stronger. A similar pattern is found with carboxylic acids, where simple aliphatic acids (e.g., propanoic) are stronger than acetic acid in the gas phase,²⁴⁹ although weaker in aqueous solution (Table 8.5). The evidence in these and other cases²⁵⁰ is that alkyl groups can be electron donating when connected to unsaturated systems, but may have either no effect or may actually be electron withdrawing in other systems. It appears that the intrinsic gas-phase acidity order of alcohols as well as the basicity order of amines is due to the effect of alkyl groups, because of their polarizability, which can spread both positive and negative charges.²⁵¹ It has been calculated that even in the case of alcohols the field effects of the alkyl groups are still operating normally, but are swamped by the greater polarizability effects.²⁵² Polarizability effects on anionic centers are a major factor in gas-phase acid–base reactions.²⁵³ It has been shown (by running reactions on ions that are solvated in the gas phase) that solvation by even one molecule of solvent can substantially affect the order of basicities.²⁵⁴

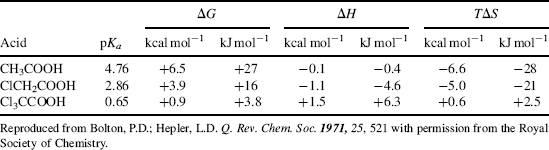
The effect on the orientation of solvent molecules when an acid or base is converted to its conjugate is an important aspect of solvent effects. For example, consider an acid (RCOOH) converted to RCOO⁻ in aqueous solution. The solvent molecules, by hydrogen bonding, arrange themselves around the COO⁻ group in a much more orderly fashion than they had been arranged around the COOH group (because they are more strongly attracted to the negative charge). This leads to a considerable loss of freedom and a decrease in entropy. Thermodynamic measurements show that for simple aliphatic and halogenated aliphatic acids in aqueous solution at room temperature, the entropy (TΔS) usually contributes much more to the total free energy change ΔG than does the enthalpy ΔH.²⁵⁵ Two examples are shown in Table 8.7.²⁵⁶ Resonance and field effects of functional groups therefore affect the acidity of RCOOH in two distinct ways. They affect the enthalpy (electron-withdrawing groups increase acidity by stabilizing RCOO⁻ by charge dispersal), but they also affect the entropy (by lowering the charge on the COO⁻ group and by changing the electron-density distribution in the COOH group, electron-withdrawing groups alter the solvent orientation patterns around both the acid and the ion, and consequently change ΔS).

Table 8.7 Thermodynamic Values for the Ionizations of Acetic and Chloroacetic Acids in H₂O at 25°C^a

a. See Ref. 256.

A change from a protic to an aprotic solvent can also affect the acidity or basicity, since there is a difference in solvation of anions by a protic solvent (which can form hydrogen bonds) and an aprotic one.²⁵⁷ The effect can be extreme: In DMF, picric acid is stronger than HBr,²⁵⁸ though in water HBr is far stronger. This particular result can be attributed to size. That is, the large ion (O₂N)₃C₆H₂O⁻ is better solvated by DMF than the smaller ion Br⁻.²⁵⁹ The ionic strength of the solvent also influences acidity or basicity, since it has an influence on activity coefficients.

In summary, solvation can have powerful effects on acidity and basicity. In the gas phase, the effects discussed in Section 8.F, especially resonance and field effects, operate unhindered by solvent molecules. Electron-withdrawing groups generally increase acidity (and decrease basicity); electron-donating groups act in the opposite way. In solution, especially aqueous solution, these effects still largely persist (which is why pK values in Table 8.5 do largely correlate with resonance and field effects), but in general are much weakened, and occasionally reversed.¹⁷⁹

Notes

1. For monographs on acids and bases, see Stewart, R. The Proton: Applications to Organic Chemistry, Academic Press, NY, 1985; Bell, R.P. The Proton in Chemistry, 2nd ed., Cornell University Press, Ithaca, NY, 1973; Finston, H.L.; Rychtman, A.C. A New View of Current Acid–Base Theories, Wiley, NY, 1982.

2. For discussion of the historical development of acid–base theory, see Bell, R.P. Q. Rev. Chem. Soc. 1947, 1, 113; Bell, R.P. The Proton in Chemistry, 1st ed., Cornell University Press, Ithaca, NY, 1959, pp. 7–17.

3. According to IUPAC terminology (Bunnett, J.F.; Jones, R.A.Y. Pure Appl. Chem. 1988, 60, 1115), an acid is a hydron donor. The IUPAC recommends that the term proton be restricted to the nucleus of the hydrogen isotope of mass 1, while the nucleus of the naturally occurring element, which contains ~0.015% deuterium, be called the hydron (the nucleus of mass 2 has always been known as the deuteron). This accords with the naturally occurring negative ion, which has long been called the hydride ion. In this book, however, we will continue to use proton for the naturally occurring form, because most of the literature uses this term.

4. Although equilibrium is reached in most acid–base reactions extremely rapidly (see Sec. 8.B), some are slow (especially those in which the proton is lost from a carbon) and in these cases time must be allowed for the system to come to equilibrium.

5. For a review of stronger Bronsted acids, see Akiyama, T. Chem. Rev. 2007, 107, 5744.

6. Table 8.1 is a thermodynamic acidity scale and applies only to positions of equilibria. For the distinction between thermodynamic and kinetic acidity (see Sec. 8.B).

7. For a first principles calculation of pK values in nonaqueous solution, see Ding, F.; Smith, J.M.; Wang, H. J. Org. Chem. 2009, 74, 2679.

8. Gold,V.; Laali, K.; Morris, K.P.; Zdunek, L.Z. J. Chem. Soc. Chem. Commun. 1981, 769; Sommer, J.; Canivet, P.; Schwartz, S.; Rimmelin, P. Nouv. J. Chim. 1981, 5, 45.

9. Arnett, E.M. Prog. Phys. Org. Chem. 1963, 1, 223, pp. 324–325.

10. Bell, R.P. The Proton in Chemistry, 2nd ed., Cornell University Press, Ithaca, NY, 1973.

11. Deno, N.C.; Gaugler, R.W.; Wisotsky, M.J. J. Org. Chem. 1966, 31, 1967.

12. Levy, G.C.; Cargioli, J.D.; Racela, W. J. Am. Chem. Soc. 1970, 92, 6238. See, however, Brouwer, D.M.; van Doorn, J.A. Recl. Trav. Chim. Pays-Bas 1971, 90, 1010.

13. Carboxylic acids, esters, and amides are shown in this table to be protonated on the carbonyl oxygen. See Smith, C.R.; Yates, K. Can. J. Chem. 1972, 50, 771; Benedetti, E.; Di Blasio, B.; Baine, P. J. Chem. Soc. Perkin Trans. 2 1980, 500; Homer, R.B.; Johnson, C.D. in Zabicky, J. The Chemistry of Amides, Wiley, NY, 1970, pp. 188–197. It has been shown that some amides protonate at nitrogen: see Perrin, C.L. Acc. Chem. Res. 1989, 22, 268. For a review of alternative proton sites, see Liler, M. Adv. Phys. Org. Chem. 1975, 11, 267.

14. Stewart, R.; Granger, M.R. Can. J. Chem. 1961, 39, 2508.

15. Yates, K.; Stewart, R. Can. J. Chem. 1959, 37, 664; Stewart, R.; Yates, K. J. Am. Chem. Soc. 1958, 80, 6355.

16. Lee, D.G. Can. J. Chem. 1970, 48, 1919.

17. Cerfontain, H.; Koeberg-Telder, A.; Kruk, C. Tetrahedron Lett. 1975, 3639.

18. Arnett, E.M.; Wu, C.Y. J. Am. Chem. Soc. 1960, 82, 5660; Koeberg-Telder, A.; Lambrechts, H.J.A.; Cerfontain, H. Recl. Trav. Chim. Pays-Bas 1983, 102, 293.

19. Fischer, A.; Grigor, B.A.; Packer, J.; Vaughan, J. J. Am. Chem. Soc. 1961, 83, 4208.

20. Arnett, E.M.; Wu, C.Y. J. Am. Chem. Soc. 1960, 82, 4999.

21. Boyd, R.H. J. Phys. Chem. 1963, 67, 737.

22. Arnett, E.M.; Quirk, R.P.; Burke, J.J. J. Am. Chem. Soc. 1970, 92, 1260.

23. McTigue. P.T.; Sime, J.M. Aust. J. Chem. 1963, 16, 592.

24. Deno, N.C.; Turner, J.O. J. Org. Chem. 1966, 31, 1969.

25. Chandler, W.D.; Lee, D.G. Can. J. Chem. 1990, 68, 1757.

26. For a discussion, see Campbell, M.L.; Waite, B.A. J. Chem. Educ. 1990, 67, 386.

27. Grant, H.M.; McTigue, P.; Ward, D.G. Aust. J. Chem. 1983, 36, 2211.

28. Bruckenstein, S.; Kolthoff, I.M. in Kolthoff, I.M.; Elving, P.J. Treatise on Analytical Chemistry, Vol. 1, pt. 1, Wiley, NY, 1959, pp. 432–433.

29. Brown, H.C.; McDaniel, D.H.; Häflinger, O. in Braude, E.A.; Nachod, F.C. Determination of Organic Structures by Physical Methods, Vol. 1, Academic Press, NY, 1955, pp. 567–662.

30. Pearson, R.G.; Dillon, R.L. J. Am. Chem. Soc. 1953, 75, 2439.

31. This value includes the CO₂ usually present. The value for H₂CO₃ alone is 3.9 in Bell, R.P. The Proton in Chemistry, 2nd ed., Cornell University Press, Ithaca, NY, 1973.

32. Crampton, M.R. in Patai, S. The Chemistry of the Thiol Group, pt. 1, Wiley, NY, 1974, pp. 396–410.

33. See Bunting, J.W.; Kanter, J.P. J. Am. Chem. Soc. 1993, 115, 11705.

34. Perrin, D.D. Ionisation Constants of Inorganic Acids and Bases in Aqueous Solution, 2nd ed., Pergamon, Elmsford, NY, 1982.

35. Rochester, C.H. in Patai, S. The Chemistry of the Hydroxyl Group, pt. 1, Wiley, NY, 1971, p. 374.

36. Cram, D.J. Chem. Eng. News 1963, 41 (No. 33, Aug. 19), 94.

37. Bowden, K.; Stewart, R. Tetrahedron 1965, 21, 261.

38. Hine, J.; Philips, J.C.; Maxwel, J.I. J. Org. Chem. 1970, 35, 3943. See also, Ang, K.P.; Lee, T.W.S. Aust. J. Chem. 1977, 30, 521.

39. Reeve, W.; Erikson, C.M.; Aluotto, P.F. Can. J. Chem. 1979, 57, 2747.

40. See also, Olmstead, W.N.; Margolin, Z.; Bordwell, F.G. J. Org. Chem. 1980, 45, 3295.

41. Harned, H.S.; Robinson, R.A. Trans. Faraday Soc. 1940, 36, 973.

42. Streitwieser, Jr., A.; Nebenzahl, L. J. Am. Chem. Soc. 1976, 98, 2188.

43. Guthrie, J.P.; Cossar, J. Can. J. Chem. 1986, 64, 2470.

44. Homer, R.B.; Johnson, C.D. in Zabicky, J. The Chemistry of Amides, Wiley, NY, 1970, pp. 238–240.

45. The pK_a of acetone in DMSO is reported to be 26.5. See Bordwell, F.G.; Zhang, X.-M. Accts. Chem. Res. 1997, 26, 510.

46. Guthrie, J.P.; Cossar, J.; Klym, A. J. Am. Chem. Soc. 1984, 106, 1351; Chiang, Y.; Kresge, A.J.; Tang, Y.S.; Wirz, J. J. Am. Chem. Soc. 1984, 106, 460.

47. Streitwieser, Jr., A.; Ciuffarin, E.; Hammons, J.H. J. Am. Chem. Soc. 1967, 89, 63.

48. Streitwieser, Jr., A.; Hollyhead, W.B.; Pudjaatmaka, H.; Owens, P.H.; Kruger, T.L.; Rubenstein, P.A.; MacQuarrie, R.A.; Brokaw, M.L.; Chu, W.K.C.; Niemeyer, H.M. J. Am. Chem. Soc. 1971, 93, 5088.

49. Streitwieser, A.; Wang, G.P.; Bors, D.A. Tetrahedron 1997, 53, 10103.

50. For a review of the acidity of cyano compounds, see Hibbert, F. in Patai, S.; Rappoport, Z. The Chemistry of Triple-bonded Functional Groups, pt. 1; Wiley, NY, 1983, pp. 699–736.

51. Cram, D.J. Fundamentals of Carbanion Chemistry, Academic Press, NY, 1965, p. 19. See also, Dessy, R.E.; Kitching, W.; Psarras, T.; Salinger, R.; Chen, A.; Chivers, T. J. Am. Chem. Soc. 1966, 88, 460.

52. Amyes, T.L.; Richard, J.P. J. Am. Chem. Soc. 1996, 118, 3129.

53. Streitwieser, Jr., A.; Hollyhead, W.B.; Sonnichsen, G.; Pudjaatmaka, H.; Chang, C.J.; Kruger, T.L. J. Am. Chem. Soc. 1971, 93, 5096.

54. Buncel, E.; Menon, B. J. Am. Chem. Soc. 1977, 99, 4457.

55. Buncel, E.; Menon, B. J. Organomet. Chem. 1977, 141, 1.

56. Albrech, H.; Schneider, G. Tetrahedron 1986, 42, 4729.

57. Boerth, D.W.; Streitwieser, Jr., A. J. Am. Chem. Soc. 1981, 103, 6443.

58. Streitwieser, Jr., A.; Scannon, P.J.; Niemeyer, H.M. J. Am. Chem. Soc. 1972, 94, 7936.

59. Streitwieser, Jr., A.; Boerth, D.W. J. Am. Chem. Soc. 1978, 100, 755.

60. This value is calculated from results given in Streitwieser, Jr., A.; Caldwell, R.A.; Young, W.R. J. Am. Chem. Soc. 1969, 91, 529. For a review of acidity and basicity of cyclopropanes, see Battiste, M.A.; Coxon, J.M. in Rappoport, Z. The Chemistry of the Cyclopropyl Group, pt. 1, Wiley, NY, 1987, pp. 255–305.

61. See Daasbjerg, K. Acta Chem. Scand. B 1995, 49, 878 for pK_a values of various hydrocarbons in DMF.

62. This value is calculated from results given in Streitwieser, Jr., A.; Taylor, D.R. J. Chem. Soc. D 1970, 1248.

63. These values are based on those given in Cram, D.J. Chem. Eng. News 1963, 41 (No. 33, Aug. 19), 94, but are corrected to the newer scale of Streitwieser, A.; Streitwieser, Jr., A.; Scannon, P.J.; Niemeyer, H.M. J. Am. Chem. Soc. 1972, 94, 7936; Streitwieser, Jr., A.; Boerth, D.W. J. Am. Chem. Soc. 1978, 100, 755.

64. Breslow, R. and co-workers report a value of 71 (Breslow, R.; Grant, J.L. J. Am. Chem. Soc. 1977, 99, 7745), but this was obtained by a different method, and is not comparable to the other values in Table 8.1. A more comparable value is 53. See also, Juan, B.; Schwarz, J.; Breslow, R. J. Am. Chem. Soc. 1980, 102, 5741.

65. This table gives average values for functional groups. See Brown, H.C.; McDaniel, D.H.; Häflinger, O. in Braude, E.A.; Nachod, F.C. Determination of Organic Structures by Physical Methods, Vol. 1, Academic Press, NY, 1955; Serjeant, E.P.; Dempsey, B. Ionisation Constants of Organic Acids in Aqueous Solution, Pergamon, Elmsford NY, 1979; Kortüm, G.; Vogel, W.; Andrussow, K. Dissociation Constants of Organic Acids in Aqueous Solution, Butterworth, London, 1961. The index in the 1979 volume covers both volumes. Kortüm, G.; Vogel, W.; Andrussow, K. Pure Appl. Chem. 1960, 1, 190; Arnett, E.M. Prog. Phys. Org. Chem. 1963, 1, 223; Perrin, D.D. Dissociation Constants of Organic Bases in Aqueous Solution, Butterworth, London, 1965, and Supplement, 1972; Collumeau, A. Bull. Soc. Chim. Fr. 1968, 5087; Bordwell, F.G. Acc. Chem. Res. 1988, 21, 456; Perrin, D.D. Ionisation Constants of Inorganic Acids and Bases in Aqueous Solution, 2nd ed., Pergamon, Elmsford NY, 1982; Pure Appl. Chem. 1969, 20, 133.

66. Gillespie, R.J. Acc. Chem. Res. 1968, 1, 202.

67. For discussions of pK_a determinations for the conjugate acids of ketones, see Bagno, A.; Lucchini,V.; Scorrano, G. Bull. Soc. Chim. Fr. 1987, 563; Toullec, J. Tetrahedron Lett. 1988, 29, 5541.

68. For a review of methods of determining pK_a values, see Cookson, R.F. Chem. Rev. 1974, 74, 5.

69. Kolthoff, I.M.; Bruckenstein, S. in Kolthoff, I.M.; Elving, P.J. Treatise on Analytical Chemistry, Vol. 1, pt. 1, Wiley, NY, 1959, pp. 475–542, p. 479.

70. For reviews of organic compounds protonated at O, N, or S, see Olah, G.A.; White, A.M.; O'Brien, D.H. Chem. Rev. 1970, 70, 561; Olah, G.A.; White, A.M.; O'Brien, D.H. in Olah, G.A.; Schleyer, P.v.R. Carbonium Ions, Vol. 4, Wiley, NY, 1973, pp. 1697–1781.

71. Rochester, C.H. Acidity Functions, Academic Press, NY, 1970. For discussion of the basicity of such compounds, see Liler, M. Reaction Mechanisms in Sulfuric Acid, Academic Press, NY, 1971, pp. 118–139.

72. Pedireddi, V.R.; Desiraju, G.R. J. Chem. Soc. Chem. Commun. 1992, 988.

73. Alkorta, I.; Campillo, N.; Rozas, I.; Elguero, J. J. Org. Chem. 1998, 63, 7759.

74. Streitwieser, A.; Keevil, T.A.; Taylor, D.R.; Dart, E.C. J. Am. Chem. Soc. 2005, 127, 9290.

75. See Reutov, O.A.; Beletskaya, I.P.; Butin, K.P. CH-Acids, Pergamon, NY, 1978; Cram, D.J. Fundamentals of Carbanion Chemistry, Academic Press, NY, 1965, pp. 1–45; Streitwieser, Jr., A.; Hammons, J.H. Prog. Phys. Org. Chem. 1965, 3, 41; Wiberg, K.B. J. Org. Chem. 2002, 67, 1613.

76. See Jones, J.R. Q. Rev. Chem. Soc. 1971, 25, 365; Fischer, H.; Rewicki, D. Prog. Org. Chem. 1968, 7, 116; Reutov, O.A.; Beletskaya, I.P.; Butin, K.P. CH-Acids, Chapter 1, Pergamon, NY, 1978 (an earlier version of this chapter appeared in Russ. Chem. Rev. 1974, 43, 17); Gau, G.; Assadourian, L.; Veracini, S. Prog. Phys. Org. Chem. 1987, 16, 237; in Buncel, E.; Durst, T. Comprehensive Carbanion Chemistry, pt. A, Elsevier, NY, 1980, the reviews by Pellerite, M.J.; Brauman, J.I. pp. 55–96 (gas-phase acidities); and Streitwieser, Jr., A.; Juaristi, E.; Nebenzahl, L. pp. 323–381.

77. See Olah, G.A.; Prakash, G.K.S.; Sommer, J. Superacids, Wiley, NY, 1985; Gillespie, R.J.; Peel, T.E. Adv. Phys. Org. Chem. 1971, 9, 1; Arata, K. Adv. Catal. 1990, 37, 165. For a review of methods of measuring superacidity, see Jost, R.; Sommer, J. Rev. Chem. Intermed. 1988, 9, 171.

78. Prakash, G.K.S. J. Org. Chem. 2006, 71, 3661.

79. These reactions are equilibria. What the rule actually says is that the position of equilibrium will be such that the weaker acid predominates. However, this needs to be taken into account only when the acid and base are close to each other in the table (within ~2 pK units).

80. See Gal, J.; Maria, P. Prog. Phys. Org. Chem. 1990, 17, 159.

81. Bohme, D.K.; Lee-Ruff, E.; Young, L.B. J. Am. Chem. Soc. 1972, 94, 4608, 5153.

82. Gerrard, W.; Macklen, E.D. Chem. Rev. 1959, 59, 1105. For other examples, see Calder, G.V.; Barton, T.J. J. Chem. Educ. 1971, 48, 338; Hambly, A.N. Rev. Pure Appl. Chem. 1965, 15, 87, p. 88.

83. Tal'Rose, V.L.; Frankevitch, E.L. J. Am. Chem. Soc. 1958, 80, 2344; DeKock, R.L. J. Am. Chem. Soc. 1975, 97, 5592; McDaniel, D.H.; Coffman, N.B.; Strong, J.M. J. Am. Chem. Soc. 1970, 92, 6697. For a computational study of the proton affinities of ketones, vicinal diketones and α-keto esters, see Taskinen, A.; Nieminen, V.; Toukoniitty, E.; Murzin, D.Yu.; Hotokka, M. Tetrahedron 2005, 61, 8109.

84. For measnurement of amine basicity via ion pair statibity in ionic liquids (Sec. 9.D.iii), see D'Anna, F.; Renato Noto, R. Tetrahedron 2007, 63, 11681.

85. Caskey, D.C.; Damrauer, R.; McGoff, D. J. Org. Chem. 2002, 67, 5098.

86. Streitwieser, A.; Kim, H.-J. J. Am. Chem. Soc. 2000, 122, 11783; Garrido, G.; Koort, E.; Ràfols, C.; Bosch, E.; Rodima, T.; Leito, I.; Rosés, M. J. Org. Chem. 2006, 71, 9062.

87. Canle, L.M.; Demirtas, I.; Freire, A.; Maskill, H.; Mishima, M. Eur. J. Org. Chem. 2004, 5031.

88. Chmurzynski, L. J. Heterocyclic Chem. 2000, 37, 71.

89. Perrin, C.L.; Ohta, B.K.; Kuperman, J.; Liberman, J.; Erdélyi, M. J. Am. Chem. Soc. 2005, 127, 9641.

97. Carrasco, N.; González-Nilo, F.; Rezende, M.C. Tetrahedron 2002, 58, 5141.

98. A new scale of π-basicity is proposed. See Stoyanov, E.S.; Stoyanova, I.V.; Reed, C.A. Chemistry: European J. 2008, 14, 7880.

99. Ly, T.; Krout, M.; Pham, D.K.; Tani, K.; Stoltz, B.M.; Julian, R.R. J. Am. Chem. Soc. 2007, 129, 1864.

90. Le Questel, J.-Y.; Laurence, C.; Lachkar, A.; Helbert, M.; Berthelot, M. J. Chem. Soc. Perkin Trans. 2 1992, 2091.

91. Berthelot, M.; Besseau, F.; Laurence, C. Eur. J. Org. Chem. 1998, 925.

92. Korzhenevskaya, N.G.; Rybachenko, V.I.; Kovalenko, V.V.; Lyashchuk, S.N.; Red'ko, A.N. Russ. J. Org. Chem. 2007, 43, 1475.

93. Berthelot, M.; Helbert, M.; Laurence, C.; LeQuestel, J.-Y.; Anvia, F.; Taft, R.W. J. Chem. Soc. Perkin Trans. 2 1993, 625.

94. Besseau, F.; Laurence, C.; Berthelot, M. J. Chem. Soc. Perkin Trans. 2 1994, 485.

95. Besseau, F.; Luçon, M.; Laurence, C.; Berthelot, M. J. Chem. Soc. Perkin Trans. 2 1998, 101.

96. Laurence, C.; Berthelot, M.; Luçon, M.; Morris, D.G. J. Chem. Soc. Perkin Trans. 2 1994, 491.

100. For calculated basicities of super bases see Glasovac, Z.; Eckert-Maksi, M.; Maksi, Z.B. New J. Chem., 2009, 33, 588.

101. Schwesinger, R.; Mißfeldt, M.; Peters, K.; von Schnering, H.G. Angew. Chem. Int. Ed. 1987, 26, 1165; Schwesinger, R.; Schlemper, H.; Hasenfratz, Ch.; Willaredt, J.; Dimbacher, T.; Breuer, Th.; Ottaway, C.; Fletschinger, M.; Boele, J.; Fritz, H.; Putzas, D.; Rotter, H.W.; Bordwell, F.G.; Satish, A.V.; Ji, G.Z.; Peters, E.-M.; Peters, K.; von Schnering, H.G. Liebigs Ann. 1996, 1055.

102. Kovaevi, B.; Maksi, Z.B. Org. Lett. 2001, 3, 1523.

103. Alder, R.W.; Bowman, P.S.; Steele, W.R.S.; Winterman, D.R. Chem. Commun. 1968, 723; Alder, R.W. Chem. Rev. 1989, 89, 1215.

104. Raczynska, E.D.; Darowska, M.; Dabkowska, I.; Decouzon, M.; Gal, J.-F.; Maria, P.-C.; Poliart, C.D. J. Org. Chem. 2004, 69, 4023.

105. Raab, V.; Kipke, J.; Gschwind, R.M.; Sundermeyer, J. Chem. Eur. J. 2002, 8, 1682.

106. Krieger, C.; Newsom, I.; Zirnstein, M.A.; Staab, H.A. Angew. Chem. Int. Ed. 1989, 28, 84.

107. Gorecka-Kobylinska, J.; Schlosser, M. J. Org. Chem. 2009, 74, 222.

108. For reviews of such proton transfers, see Hibbert, F. Adv. Phys. Org. Chem. 1986, 22, 113; Crooks, J.E. in Bamford, C.H.; Tipper, C.F.H. Chemical Kinetics, Vol. 8; Elsevier, NY, 1977, pp. 197–250. See Bernasconi, C.F.; Fairchild, D.E.; Montañez, R.L.; Aleshi, P.; Zheng, H.; Lorance, E. J. Org. Chem. 2005, 70, 7721.

109. See Eigen, M. Angew. Chem. Int. Ed. 1964, 3, 1.

110. See, for example, Hojatti, M.; Kresge, A.J.; Wang, W. J. Am. Chem. Soc. 1987, 109, 4023.

111. See Ritchie, C.D.; Lu, S. J. Am. Chem. Soc. 1989, 111, 8542.

112. Chen, X.; Walthall, D.A.; Brauman, J.I. J. Am. Chem. Soc. 2004, 126, 12614.

113. Abraham, M. H.; Enomoto, K.; Clarke, E. D.; Sexton, G. J. Org. Chem. 2002, 67, 4782.

114. See Hibbert, F. in Bamford, C.H.; Tipper, C.F.H. Chemical Kinetics, Vol. 8, Elsevier, NY, 1977, pp. 97–196; Kreevoy, M.M. Isot. Org. Chem. 1976, 2, 1; Leffek, K.T. Isot. Org. Chem. 1976, 2, 89.

115. See Bernasconi, C.F. Tetrahedron 1985, 41, 3219.

116. Houk, R.J.T.; Anslyn, E.V.; Stanton, J.F. Org. Lett. 2006, 8, 3461.

117. Kresge, A.J.; Powell, M.F. J. Org. Chem. 1986, 51, 822; Formosinho, S.J.; Gal, V.M.S. J. Chem. Soc. Perkin Trans. 2 1987, 1655.

118. Not all 1-alkynes behave as normal acids; see Aroella, T.; Arrowsmith, C.H.; Hojatti, M.; Kresge, A.J.; Powell, M.F.; Tang, Y.S.; Wang, W. J. Am. Chem. Soc. 1987, 109, 7198.

119. Juhasz, M.; Hoffmann, S.; Stoyanov, E.; Kim, K.-C.; Reed, C.A. Angew. Chem. Int. Ed. 2004, 43, 5352.

120. See Kurz, J.L. J. Am. Chem. Soc. 1989, 111, 8631.

121. Wang, W.; Cheng, P.; Huang, C.; Jong, Y. Bull. Chem. Soc. Jpn. 1992, 65, 562.

122. Wang, W.-h.; Cheng, C.-c. Bull. Chem. Soc. Jpn. 1994, 67, 1054.

123. Lambert, C.; Hampel, F.; Schleyer, P.v.R. Angew. Chem. Int. Ed. 1992, 31, 1209.

124. For fuller treatments, see Hammett, L.P. Physical Organic Chemistry, 2nd ed., McGraw-Hill, NY, 1970, pp. 263–313; Jones, R.A.Y. Physical and Mechanistic Organic Chemistry, 2nd ed., Cambridge University Press, Cambridge, 1984, pp. 83–93; Arnett, E.M.; Scorrano, G. Adv. Phys. Org. Chem. 1976, 13, 83.

125. Holt, J.; Karty, J.M. J. Am. Chem. Soc. 2003, 125, 2797.

126. Thomazeau, C.; Olivier-Bourbigou, H.; Magna, L.; Luts, S.; Gilbert, B. J. Am. Chem. Soc. 2003, 125, 5264.

127. Yang, Y.-l.; Kou, Y. Chem. Commun. 2004, 226.

128. Hammett, L.P.; Deyrup, A.J. J. Am. Chem. Soc. 1932, 54, 2721.

129. See Rochester, C.H. Acidity Functions, Academic Press, NY, 1970; Cox, R.A.; Yates, K. Can. J. Chem. 1983, 61, 2225; Boyd R.H. in Coetzee, J.F.; Ritchie, C.D. Solute–Solvent Interactions, Marcel Dekker, NY, 1969, pp. 97–218.

130. See Yates, K.; McClelland, R.A. Prog. Phys. Org. Chem. 1974, 11, 323.

131. See Kreevoy, M.M.; Baughman, E.H. J. Am. Chem. Soc. 1973, 95, 8178; García, B.; Leal, J.M.; Herrero, L.A.; Palacios, J.C. J. Chem. Soc. Perkin Trans. 2 1988, 1759; Arnett, E.M.; Quirk, R.P.; Burke, J.J. J. Am. Chem. Soc. 1970, 92, 1260.

132. For lengthy tables of many acidity scales, with references, see Cox, R.A.; Yates, K. Can. J. Chem. 1983, 61, 2225. For an equation that is said to combine the vast majority of acidity functions, see Zalewski, R.I.; Sarkice, A.Y.; Geltz, Z. J. Chem. Soc. Perkin Trans. 2 1983, 1059.

133. See Vianello, R.; Maksi, Z.B. Eur. J. Org. Chem. 2004, 5003.

134. Deno, N.C.; Berkheimer, H.E.; Evans, W.L.; Peterson, H.J. J. Am. Chem. Soc. 1959, 81, 2344.

135. Reagan, M.T. J. Am. Chem. Soc. 1969, 91, 5506.

136. Edward, J.T.; Wong, S.C. Can. J. Chem. 1977, 55, 2492; Liler, M.; Markovi, D. J. Chem. Soc. Perkin Trans. 2 1982, 551.

137. Hammett, L.P. Physical Organic Chemistry, 2nd ed., McGraw-Hill, NY, 1970, p. 278; Rochester, C.H. Acidity Functions, Academic Press, NY, 1970, p. 21.

138. For another approach to solvent basicity scales, see Catalán, J.; Gómez, J.; Couto, A.; Laynez, J. J. Am. Chem. Soc. 1990, 112, 1678.

139. See Rochester, C.H. Q. Rev. Chem. Soc. 1966, 20, 511; Rochester, C.H. Acidity Functions, Academic Press, NY, 1970, pp. 234–264; Bowden, K. Chem. Rev. 1966, 66, 119.

140. Bunnett, J.F.; McDonald, R.L.; Olsen, F.P. J. Am. Chem. Soc. 1974, 96, 2855.

141. More O'Ferrall, R.A. J. Chem. Soc. Perkin Trans. 2 1972, 976.

142. Bagno, A.; Scorrano, G.; More O'Ferrall, R.A. Rev. Chem. Intermed. 1987, 7, 313. See also, Cox, R.A. Acc. Chem. Res. 1987, 20, 27.

143. Bunnett, J.F. J. Am. Chem. Soc. 1961, 83, 4956, 4968, 4973, 4978.

144. Catalán, J.; Couto, A.; Gomez, J.; Saiz, J.L.; Laynez, J. J. Chem. Soc. Perkin Trans. 2 1992, 1181.

145. Abraham, M.H.; Taft, R.W. J. Chem. Soc. Perkin Trans. 2 1993, 305.

146. Liu, P.C.; Hoz, S.; Buncel, E. Gazz. Chim. Ital. 1996, 126, 31. See also, Abraham, M.H.; Zhao, Y.J. J. Org. Chem. 2004, 69, 4677.

147. Fattahi, A.; Kass, S.R. J. Org. Chem. 2004, 69, 9176.

148. See Stewart, R. The Proton: Applications to Organic Chemistry, Academic Press, NY, 1985, pp. 251–305; Willi, A.V. in Bamford, C.H.; Tipper, C.F.H. Chemical Kinetics, Vol. 8, Elsevier, NY, 1977, pp. 1–95; Jones, R.A.Y. Physical and Mechanistic Organic Chemistry, 2nd ed., Cambridge University Press, Cambridge, 1984, pp. 72–82; Bender, M.L. Mechanisms of Homogeneous Catalysis from Protons to Proteins, Wiley, NY, 1971, pp. 19–144.

149. See Klumpp, G.W. Reactivity in Organic Chemistry, Wiley, NY, 1982, pp. 167–179; Bell, R.P. in Chapman, N.B.; Shorter, J. Correlation Analysis in Chemistry: Recent Advances, Plenum Press, 1978, pp. 55–84; Kresge, A.J. Chem. Soc. Rev. 1973, 2, 475.

150. See Silva, P.J. J. Org. Chem. 2009, 74, 914.

151. See Marcus, R.A. J. Phys. Chem. 1968, 72, 891; Kresge, A.J. Chem. Soc. Rev. 1973, 2, 475.

152. Omitting the work terms.

153. Albery, W.J. Annu. Rev. Phys. Chem. 1980, 31, 227, p. 244.

154. See Jencks, W.P. Acc. Chem. Res. 1976, 9, 425; Stewart, R.; Srinivasan, R. Acc. Chem. Res. 1978, 11, 271; Guthrie, J.P. J. Am. Chem. Soc. 1980, 102, 5286.

155. See Agmon, N. J. Am. Chem. Soc. 1980, 102, 2164; Murray, C.J.; Jencks, W.P. J. Am. Chem. Soc. 1988, 110, 7561.

156. Pross, A.; Shaik, S.S. New J. Chem. 1989, 13, 427; Lewis, E.S. J. Phys. Org. Chem. 1990, 3, 1.

157. Lewis bases are useful catalysts in organic synthesis. See Denmark, S.E.; Beutner, G.L. Angew. Chem. Int. Ed. 2008, 47, 1560.

158. For a monograph on Lewis acid–base theory, see Jensen, W.B. The Lewis Acid–Base Concept, Wiley, NY, 1980. For a discussion of the definitions of Lewis acid and base, see Jensen, W.B. Chem. Rev. 1978, 78, 1.

159. Rauk, A.; Hunt, I.R.; Keay, B.A. J. Org. Chem. 1994, 59, 6808.

160. For a review of ate complexes, see Wittig, G. Q. Rev. Chem. Soc. 1966, 20, 191.

161. See Satchell, D.P.N.; Satchell, R.S. Q. Rev. Chem. Soc. 1971, 25, 171; Chem. Rev. 1969, 69, 251. See also, Sandström, M.; Persson, I.; Persson, P. Acta Chem. Scand. 1990, 44, 653; Laszlo, P.; Teston-Henry, M. Tetrahedron Lett. 1991, 32, 3837.

162. Springer, G.; Elam, C.; Edwards, A.; Bowe, C.; Boyles, D.; Bartmess, J.; Chandler, M.; West, K.; Williams, J.; Green, J.; Pagni, R.M.; Kabalka, G.W. J. Org. Chem. 1999, 64, 2202.

163. See Ayers, P.W.; Parr, R.G. J. Am. Chem. Soc. 2000, 122, 2010.

164. Pearson, R.G.; Songstad, J. J. Am. Chem. Soc. 1967, 89, 1827. For a monograph on the concept, see Ho, T. Hard and Soft Acids and Bases Principle in Organic Chemistry, Academic Press, NY, 1977; Pearson, R.G. J. Chem. Educ. 1987, 64, 561; Ho, T. Tetrahedron 1985, 41, 1; Pearson, R.G. in Chapman, N.B.; Shorter, J. Advances in Linear Free-Energy Relationships, Plenum Press, NY, 1972, pp. 281–319. For a collection of papers, see Pearson, R.G. Hard and Soft Acids and Bases, Dowden, Hutchinson, and Ross, Stroudsberg, PA, 1973.

165. Taken from Pearson, R.G. J. Chem. Ed. 1968, 45, 581, 643.

166. Pearson, R.G. Inorg. Chem. 1988, 27, 734; J. Org. Chem. 1989, 54, 1423. See also, Orsky, A.R.; Whitehead M.A. Can. J. Chem. 1987, 65, 1970.

167. See Sauers, R.R. Tetrahedron 1999, 55, 10013.

168. Parr, R.G.; Pearson, R.G. J. Am. Chem. Soc. 1983, 105, 7512. Note that there is not always a strict correlation between the values in Table 8.4 and the categories of Table 8.3.

169. Pearson, R.G. J. Am. Chem. Soc. 1988, 110, 7684.

170. For proofs of this principle, see Chattaraj, P.K.; Lee, H.; Parr, R.G. J. Am. Chem. Soc. 1991, 113, 1855.

171. Wolman, Y. in Patai, S. The Chemistry of the Thiol Group, pt. 2, Wiley, NY, 1974, p. 677; Maskill, H. The Physical Basis of Organic Chemistry, Oxford University Press, Oxford 1985, p. 159.

172. See also, Bochkov, A.F. J. Org. Chem. USSR 1986, 22, 1830, 1837.

173. Méndez, F.; Gázguez, J.L. J. Am. Chem. Soc. 1994, 116, 9298.

174. Woodward, S. Tetrahedron 2002, 58, 1017.

175. See Hine, J. Structural Effects on Equilibria in Organic Chemistry, Wiley, NY, 1975; Taft, R.W. Prog. Phys. Org. Chem. 1983, 14, 247; Petrov, E.S. Russ. Chem. Rev. 1983, 52, 1144 (NH acids); Bell, R.P. The Proton in Chemistry, 2nd ed., Cornell Univ. Press, Ithaca, NY, 1973, pp. 86–110. For a monograph on methods of estimating pK values by analogy, extrapolation, and so on, see Perrin, D.D.; Dempsey, B.; Serjeant, E.P. pK_a Prediction for Organic Acids and Bases, Chapman and Hall, NY, 1981.

176. The varying degrees by which the different factors that affect gas-phase acidities of 25 acids has been calculated: Taft, R.W.; Koppel, I.A.; Topsom, R.D.; Anvia, F. J. Am. Chem. Soc. 1990, 112, 2047.

177. For a review of the enhancement of acidity by NO₂, see Lewis, E.S. in Patai, S. The Chemistry of Functional Groups, Supplement F, pt. 2, Wiley, NY, 1982, pp. 715–729.

178. Filler, R.; Wang, C. Chem. Commun. 1968, 287.

179. See Edward, J.T. J. Chem. Educ. 1982, 59, 354; Schwartz, L.M. J. Chem. Educ. 1981, 58, 778.

180. Headley, A.D.; McMurry, M.E.; Starnes, S.D. J. Org. Chem. 1994, 59, 1863.

181. Wiberg, K.B. J. Org. Chem. 2003, 68, 875.

182. For calculated gas-phase acidities of substituted benzoic acids see Wiberg, K.B. J. Org. Chem. 2002, 67, 4787. Also see Gupta, K.; Giri, S.; Chattaraj, P.K. New J. Chem. 2008, 32, 1945.

183. DeMaria, P.; Fontana, A.; Spinelli, D.; Dell'Erba, C.; Novi, M.; Petrillo, G.; Sancassan, F. J. Chem. Soc. Perkin Trans. 2 1993, 649.

184. Chen, C.-T.; Siegel, J.S. J. Am. Chem. Soc. 1994, 116, 5959. See also, Sotomatsu, T.; Shigemura, M.; Murata, Y.; Fujita, T. Bull. Chem. Soc. Jpn. 1992, 65, 3157.

185. See Exner, O.; ársky, P. J. Am. Chem. Soc. 2001, 123, 9564. See also, Liptak, M.D.; Shields, G.C. J. Am. Chem. Soc. 2001, 123, 7314.

186. It has been contended that resonance delocalization plays only a minor role in the increased strength of carboxylic acids compared to alcohols, and the “...higher acidity of acids arises principally because the electrostatic potential of the acidic hydrogens is more positive in the neutral acid molecule...”: Siggel, M.R.; Streitwieser, Jr., A.; Thomas, T.D. J. Am. Chem. Soc. 1988, 110, 8022; Thomas, T.D.; Carroll, T.X.; Siggel, M.R. J. Org. Chem. 1988, 53, 1812. For contrary views, see Exner, O. J. Org. Chem. 1988, 53, 1810; Perrin, D.D. J. Am. Chem. Soc. 1991, 113, 2865. See also, Godfrey, M. Tetrahedron Lett. 1990, 31, 5181.

187. Copper complexes of active methylene compounds show large pK_a shifts. See Zhong, Z.; Postnikova, B.J.; Hanes, R.E.; Lynch, V.M.; Anslyn, E.V. Chemistry: European J. 2005, 11, 2385.

188. Goumont, R.; Magnier, E.; Kizilian, E.; Terrier, F. J. Org. Chem. 2003, 68, 6566.

189. See, however, Krygowski, T.M.; Maurin, J. J. Chem. Soc. Perkin Trans. 2 1989, 695.

190. Smith, J.W. in Patai, S. The Chemistry of the Amino Group; Wiley, NY, 1968, pp. 161–204.

191. Liptak, M.D.; Gross, K.C.; Seybold, P.G.; Feldus, S.; Shields, G.C. J. Am. Chem. Soc. 2002, 124, 6421.

192. Taft, R.W. Prog. Phys. Org. Chem. 1983, 14, 247, see Sec. 5.B.i.

193. Note that Lewis acidity decreases, whereas Brnsted acidity increases, going down the table. There is no contradiction here when we remember that in the Lewis picture the actual acid in all Brnsted acids is the same, namely, the proton. In comparing, say, HI and HF, we are not comparing different Lewis acids but only how easily F^– and I^– give up the proton.

194. The effect discussed here is an example of a symmetry factor. For an extended discussion, see Eberson, L. in Patai, S. The Chemistry of Carboxylic Acids and Esters, Wiley, NY, 1969, pp. 211–293.

195. Brown, H.C. J. Am. Chem. Soc. 1945, 67, 378, 1452, Boranes in Organic Chemistry, Cornell University Press, Ithaca, NY, 1972, pp. 53–64. See also, Brown, H.C.; Krishnamurthy, S.; Hubbard, J.L. J. Am. Chem. Soc. 1978, 100, 3343.

196. Hibbert, F.; Simpson, G.R. J. Chem. Soc. Perkin Trans. 2 1987, 243, 613.

197. For a review of the effect of strain on amine basicities, see Alder, R.W. Chem. Rev. 1989, 89, 1215.

198. Staab, H.A.; Saupe, T.; Krieger, C. Angew. Chem. Int. Ed. 1983, 22, 731.

199. Saupe, T.; Krieger, C.; Staab, H.A. Angew. Chem. Int. Ed. 1986, 25, 451.

200. For a review, see Staab, H.A.; Saupe, T. Angew. Chem. Int. Ed. 1988, 27, 865.

201. Howard, S.T. J. Am. Chem. Soc. 2000, 122, 8238.

202. Krieger, C.; Newsom, I.; Zirnstein, M.A.; Staab, H.A. Angew. Chem. Int. Ed. 1989, 28, 84. See also, Staab, H.A.; Zirnstein, M.A.; Krieger, C. Angew. Chem. Int. Ed. 1989, 28, 86.

203. Miyahara, Y.; Goto, K.; Inazu, T. Tetrahedron Lett. 2001, 42, 3097.

204. Ueno, M.; Ishitani, H.; Kobayashi, S. Org. Lett. 2002, 4, 3395.

205. Mathieu, B.; de Fays, L.; Ghosez, L. Tetrahedron Lett 2000, 41, 9651

206. Brown, H.C.; Kanner, B. J. Am. Chem. Soc. 1953, 75, 3865; 1966, 88, 986.

207. Meot-Ner, M.; Smith, S.C. J. Am. Chem. Soc. 1991, 113, 862, and references cited therein. See also, Benoit, R.L.; Fréchette, M.; Lefebvre, D. Can. J. Chem. 1988, 66, 1159.

208. Arnett, E.M.; Harrelson, Jr., J.A. J. Am. Chem. Soc. 1987, 109, 809.

209. For a discussion of why esters and amides are weaker acids than ketones, see Fersner, A.; Karty, J.M.; Mo, Y. J. Org. Chem. 2009, 74, 7245.

210. Wang, X.; Houk, K.N. J. Am. Chem. Soc. 1988, 110, 1870; Wiberg, K.B.; Laidig, K.E. J. Am. Chem. Soc. 1988, 110, 1872.

211. Majewski, M.; Wang, F. Tetrahedron 2002, 58, 4567.

212. Amedjkouh, M. Tetrahedron Asymm. 2004, 15, 577.

213. Gung, B.W.; Yanik, M.M. J. Org. Chem. 1996, 61, 947.

214. Grotjahn, D.B.; Sheridan, P.M.; Al Jihad, I.; Ziurys, L.M. J. Am. Chem. Soc. 2001, 123, 5489.

215. Fressigné, C.; Maddaluno, J.; Giessner-Prettre, C.; Silvi, B. J. Org. Chem. 2001, 66, 6476.

216. Williard, P.G.; Salvino, J.M. J. Org. Chem. 1993, 58, 1. For a study of the oligomer structure of LDA at low ligand concentrations, see Rutherford, J.L.; Collum, D.B. J. Am. Chem. Soc. 2001, 123, 199.

217. Ito, H.; Nakamura, T.; Taguchi, T.; Hanzawa, Y. Tetrahedron Lett. 1992, 33, 3769.

218. Aubrecht, K.B.; Collum, D.B. J. Org. Chem. 1996, 61, 8674.

219. Romesberg, F.E.; Collum, D.B. J. Am.Chem. Soc. 1994, 116, 9198, 9187. For a study of other mixed aggregates, see Thomas, R.D.; Huang, J. J. Am. Chem. Soc. 1999, 121, 11239.

220. Sakuma, K.; Gilchrist, J.H.; Romesberg, F.E.; Cajthami, C.E.; Collum, D.B. Tetrahedron Lett. 1993, 34, 5213.

221. Lucht, B.L.; Collum, D.B. J. Am. Chem. Soc. 1994, 116, 7949.

222. Lucht, B.L.; Collum, D.B. J. Am. Chem. Soc. 1995, 117, 9863. See also, Lucht, B.L.; Collum, D.B. J. Am. Chem. Soc. 1996, 118, 2217, 3529. See Romesberg, F.E.; Bernstein, M.P.; Gilchrist, J.H.; Harrison, A.T.; Fuller, D.J.; Collum, D.B. J. Am. Chem. Soc. 1993, 115, 3475 for the structure in HMPA.

223. Lucht, B.L.; Collum, D.B. J. Am. Chem. Soc. 1994, 116, 6009.

224. Collum, D.B. Acc. Chem. Res. 1993, 26, 227. For NMR studies of LiNEt₂ and ring laddering see Rutherford, J.L.; Collum, D.B. J. Am. Chem. Soc. 1999, 121, 10198.

225. Hilmersson, G.; Davidsson, Ö. J. Org. Chem. 1995, 60, 7660. See O'Brien, P. J. Chem. Soc. Perkin Trans. 1 1998, 1439; Sott, R.; Grandander, J.; Dinér, P.; Hilmersson, G. Tetrahedron Asymm. 2004, 15, 267.

226. Arvidsson, P.I.; Hilmersson, G.; Ahlberg, P. J. Am. Chem. Soc. 1999, 121, 183.

227. Carlier, P.R.; Madura, J.D. J. Org. Chem. 2002, 67, 3832.

228. Williard, P. G.; Jacobson, M. A. Org. Lett. 2000, 2, 2753. For the structure and bonding of dilithiodiamines see Pratt, L.M.; Mu, R. J. Org. Chem. 2004, 69, 7519.

229. Sun, C.; Williard, P.G. J. Am. Chem. Soc. 2000, 122, 7829. See also, Pratt, L.M.; Streitwieser, A. J. Org. Chem. 2003, 68, 2830.

230. Jackman, L.M.; Çizmeciyan, D.; Williard, P.G.; Nichols, M.A. J. Am. Chem. Soc. 1993, 115, 6262.

231. Jackman, L.M.; Chen, X. J. Am. Chem. Soc. 1992, 114, 403.

232. Streitwieser, A.; Juaristi, E.; Kim, Y.-J.; Pugh, J.K. Org. Lett. 2000, 2, 3839.

233. Alconcel, L.S.; Deyerl, H.-J.; Continetti, R.E. J. Am. Chem. Soc. 2001, 123, 12675.

234. See Epshtein, L.M.; Iogansen, A.V. Russ. Chem. Rev. 1990, 59, 134; Dyumaev, K.M.; Korolev, B.A. Russ. Chem. Rev. 1980, 49, 1021; Taft, R.W.; Bordwell, F.G. Acc. Chem. Res. 1988, 21, 463; Heemstra, J.M.; Moore, J.S. Tetrahedron 2004, 60, 7287.

235. See Smith, J.W. in Patai, S. The Chemistry of the Amino Group, Wiley, NY, 1968, pp. 161–204.

236. Aue, D.H.; Webb, H.M.; Bowers, M.T. J. Am. Chem. Soc. 1972, 94, 4726; 1976, 98, 311, 318; Mucci, A.; Domain, R.; Benoit, R.L. Can. J. Chem. 1980, 58, 953. See also, Drago, R.S.; Cundari, T.R.; Ferris, D.C. J. Org. Chem. 1989, 54, 1042.

237. For discussions of the solvation of ammonia and amines, see Jones, III, F.M.; Arnett, E.M. Prog. Phys. Org. Chem. 1974, 11, 263; Grunwald, E.; Ralph, E.K. Acc. Chem. Res. 1971, 4, 107.

238. Condon, F.E. J. Am. Chem. Soc. 1965, 87, 4481, 4485.

239. For two reasons: (1) the alkyl groups are poorly solvated by the water molecules, and (2) the strength of the hydrogen bonds of the BH⁺ ions decreases as the basicity of B increases: Lau, Y.K.; Kebarle, P. Can. J. Chem. 1981, 59, 151.

240. See Liebman, J.F. Mol. Struct. Energ. 1987, 4, 49; Dixon, D.A.; Lias, S.G. Mol. Struct. Energ. 1987, 2, 269; Bohme, D.K. in Patai, S. The Chemistry of Functional Groups, Supplement F, pt. 2, Wiley, NY, 1982, pp. 731–762; Arnett, E.M. Acc. Chem. Res. 1973, 6, 404. See Lias, S.G.; Liebman, J.F.; Levin, R.D. J. Phys. Chem. Ref. Data, 1984, 13, 695. See also, the tables of gas-phase acidities and basicities in the following articles, and their cited references: Meot-Ner, M.; Kafafi, S.A. J. Am. Chem. Soc. 1988, 110, 6297; Headley, A.D. J. Am. Chem. Soc. 1987, 109, 2347; Fujio, M.; McIver, Jr., R.T.; Taft, R.W. J. Am. Chem. Soc. 1981, 103, 4017; Lau, Y.K.; Nishizawa, K.; Tse, A.; Brown, R.S.; Kebarle, P. J. Am. Chem. Soc. 1981, 103, 6291.

241. Briggs, J.P.; Yamdagni, R.; Kebarle, P. J. Am. Chem. Soc. 1972, 94, 5128; Aue, D.H.; Webb H.M.; Bowers, M.T. J. Am. Chem. Soc. 1972, 94, 4726; 1976, 98, 311, 318.

242. Ikuta, S.; Kebarle, P. Can. J. Chem. 1983, 61, 97.

243. Taft, R.W.; Taagepera, M.; Summerhays, K.D.; Mitsky, J. J. Am. Chem. Soc. 1973, 95, 3811.

244. Yamdagni, R.; Kebarle, P. J. Am. Chem. Soc. 1973, 95, 3504.

245. See Catalan, J.; Abboud, J.L.M.; Elguero, J. Adv. Heterocycl. Chem. 1987, 41, 187.

246. Bartnicka, H.; Bojanowska, I.; Kalinowski, M.K. Aust. J. Chem. 1993, 46, 31.

247. Kobayashi, S. Synlett, 1994, 689.

248. Arnett, E.M.; Small, L.E.; McIver, Jr., R.T.; Miller, J.S. J. Am. Chem. Soc. 1974, 96, 5638; Blair, L.K.; Isolani, P.C.; Riveros, J.M. J. Am. Chem. Soc. 1973, 95, 1057; McIver, Jr., R.T.; Scott, J.A.; Riveros, J.M. J. Am. Chem. Soc. 1973, 95, 2706. Also see Bartmess, J.E.; McIver, Jr., R.T. J. Am. Chem. Soc. 1977, 99, 4163.

249. See Caldwell, G.; Renneboog, R.; Kebarle, P. Can. J. Chem. 1989, 67, 611.

250. Brauman, J.I.; Blair, L.K. J. Am. Chem. Soc. 1971, 93, 4315; Laurie, V.W.; Muenter, J.S. J. Am. Chem. Soc. 1966, 88, 2883.

251. Brauman, J.I.; Riveros, J.M.; Blair, L.K. J. Am. Chem. Soc. 1971, 93, 3914; Huheey, J.E. J. Org. Chem. 1971, 36, 204; Radom, L. Aust. J. Chem. 1975, 28, 1; Aitken, E.J.; Bahl, M.K.; Bomben, K.D.; Gimzewski, J.K.; Nolan, G.S.; Thomas, T.D. J. Am. Chem. Soc. 1980, 102, 4873.

252. Taft, R.W.; Taagepera, M.; Abboud, J.M.; Wolf, J.F.; DeFrees, D.J.; Hehre, W.J.; Bartmess, J.E.; McIver, Jr., R.T. J. Am. Chem. Soc. 1978, 100, 7765. For a scale of polarizability parameters, see Hehre, W.J.; Pau, C.; Headley, A.D.; Taft, R.W.; Topsom, R.D. J. Am. Chem. Soc. 1986, 108, 1711.

253. Bartmess, J.E.; Scott, J.A.; McIver, Jr., R.T. J. Am. Chem. Soc. 1979, 101, 6056.

254. Bohme, D.K.; Rakshit, A.B.; Mackay, G.I. J. Am. Chem. Soc. 1982, 104, 1100.

255. Bolton, P.D.; Hepler, L.G. Q. Rev. Chem. Soc. 1971, 25, 521; Gerrard, W.; Macklen, E.D. Chem. Rev. 1959, 59, 1105. See also, Wilson, B.; Georgiadis, R.; Bartmess, J.E. J. Am. Chem. Soc. 1991, 113, 1762.

256. Bolton, P.D.; Hepler, L.G. Q. Rev. Chem. Soc. 1971, 25, 521; p. 529.

257. For a review, see Parker, A.J. Q. Rev. Chem. Soc. 1962, 16, 163.

258. Sears, P.G.; Wolford, R.K.; Dawson, L.R. J. Electrochem. Soc. 1956, 103, 633.

259. Miller, J.; Parker, A.J. J. Am. Chem. Soc. 1961, 83, 117.