 10
10 
 10
10 
For most recipes, scaling up or down is just arithmetic. If a recipe serves four and you have six dinner guests, multiply each ingredient by 6/4 and you will generally get good results.
However, not all recipes are this easy, and scaling a recipe for four people up to a banquet of 200 people by multiplying by 50 probably won’t work out so well. Why that is the case gets us into some interesting science. And the science can get us safely back to scaling the recipe.
One thing that causes problems in recipe scaling is that as you increase the volume of the ingredients, the surface area of the mixture does not increase as quickly. As an example, suppose you have a recipe that calls for boiling a quart of chicken stock in a four-quart saucepan. When you double the recipe, the bottom surface of the saucepan, where the heat is, remains the same. The top surface, where the evaporation happens, remains the same. The depth of the liquid is the only thing that has doubled along with the volume.
If your saucepan is eight inches in diameter, a quart of liquid will fill it a little over an inch (call it 1.14889975 inches if you like). Two quarts raises the level to almost 21/3 inches (2.29779949 inches). You can do all the arithmetic easily using Google: just search for “2 quarts / (pi * 4 inches * 4 inches) in inches.”
Calculating the surface area can be done the same way:
For one quart, you have 129 square inches of surface area. For two quarts, you have 158 square inches. The volume has doubled, but the surface area has increased by only 158/129, or about 1¼ times.
In a saucepan, this will mean several things. Since the heat applied to the bottom is the same, and the evaporation from the top is the same, it should take about twice as long to bring it to a boil, and about twice as long to reduce the stock to half its volume.
This is not a big problem for reducing a pot of chicken stock, but consider what happens if you are baking a loaf of bread and you double the volume. If you make the loaf twice as long, you don’t have much of a problem. Likewise, you can make two loaves and not have much trouble. But if you try to keep the shape of the loaf by scaling up the length, width, and height by the same amount, you get into trouble.
Suppose you have a loaf that is 5 inches wide, 4 inches high, and about 8½ inches long. It has a volume of 3 quarts. Doubling each dimension to 10 inches wide, 8 inches high, and 17 inches long gives us a volume of almost 24 quarts. That’s eight times the volume.
Should you cook it twice as long, or eight times as long?
In an oven, the heat comes from all sides of a loaf pan. The heat has to travel twice as far to reach the center of a large loaf. But there is eight times as much loaf to heat, and you have only twice the surface area through which the heat can reach the dough.
Being a foam, bread dough is not a particularly good conductor of heat. The outside of the loaf will dry out and then brown before the inside has reached the temperature needed to make the starches gel and the proteins denature.
The rate at which something heats up is proportional to the difference between the temperature inside and the temperature outside. This is known as Isaac Newton’s Law of Cooling, although it works the same for both heating and cooling. As a loaf of bread heats up, the difference between the inside and outside temperatures gets smaller, and so the rate at which it warms gets slower.
Small things heat up quickly. Large things heat up more slowly. So as a recipe is doubled, the rate at which the bread cooks goes down. But because bread is not a good conductor of heat, the outside cooks faster than the center. This gets worse the larger the loaf. You can reduce this effect by cooking at a lower temperature, taking advantage of Newton’s Law of Cooling.
What makes the volume grow faster than the surface area is the number of dimensions involved. As the radius of a sphere doubles, the surface area goes up four times, because while the radius is a one-dimensional line, the surface area is two dimensional. You multiply the radius by two, but you multiply the surface by two because the width doubled, and then by two again because the length doubled. The volume is three dimensional, so you multiply by two a third time (that’s a factor of 8 because 2 × 2 × 2 = 8) to account for the depth doubling. This is why surfaces are measured in square inches, and volumes in cubic inches.
If we want to keep the surface-to-volume ratio constant, you can eliminate that third doubling by keeping the depth constant. This almost works perfectly. A 2-inch cube has a surface area of 24 square inches, and a volume of 8 cubic inches, for a ratio of 3/1. Doubling the width and length gives a volume of 32 cubic inches and a surface area of 64 square inches, for a ratio of 2/1. Not perfect, but much better than doubling all the dimensions, where the ratio would go from 3/1 down to 2/3.
To make up for the rest of the difference, you can reduce the temperature and cook for a longer time.
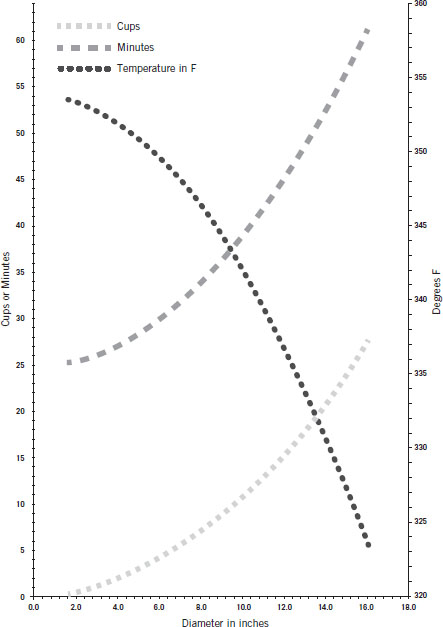
Consider baking a wedding cake, for which you want to use the same batter for all the layers, but you want the top layer to be 6 inches in diameter and the bottom layer to be 16 inches in diameter, with other layers in between of successively increasing diameter from top to bottom. Each layer will be 4 inches in height, composed of two layers, each 2 inches high, cemented together with frosting or jelly.
You do some testing and find that a 6-inch cake is best when baked at 350°F (177°C) for 30 minutes, and a 16-inch cake is best when baked at 325°F (163°C) for an hour. Instead of testing each intermediate layer, you remember some algebra for finding the formula of a line given two points, and use that to draw a graph.
Now you can just look up the diameter of the layer on the bottom axis and read off the temperature and cooking time according to their lines. And, as a bonus, you can find out how many cups of batter each layer will require. As the diameter of the layers grows, the temperature gradually decreases, and the time needed to bake it gradually increases.
The surface-to-volume ratio affects how long it takes something to dry. Water evaporates at the surface, so the surface area determines the rate at which something dries. But the amount of water in something depends on the volume.
If you want something to dry quickly, spread it out over a large area. If you want something to stay moist, form it into a sphere (the shape with the smallest surface-to-volume ratio), or put it into a tall cylinder (assuming you can’t just cover it up!).
Sea salt is evaporated in very shallow ponds that have square miles of surface area. Sun-dried apricots and prunes are spread out to dry, as are cacao beans and coffee beans.
The smaller an object is, the higher the surface-to-volume ratio. So finely ground bread crumbs will dry faster than a slice of bread, and a thin slice of bread will dry faster than a thick slice.
In making dried apples, slicing the apple into very thin slices and drying them in a 250°F (121°C) oven for an hour will make crisp chips. Trying to dry the whole apple will be much less rewarding, and take much longer.
You saw how adjusting the timing when baking different sizes of cake allowed you to scale up the recipe, and how you can speed up drying by increasing the surface-to-volume ratio. Other things are also affected by or affect timing.
Deep-frying a large potato will not get the same result as deep-frying thin slices of potato. But deep-frying a big bucket of french fries will not produce the same result as dividing the bucket into quarters and frying four batches. Dumping a large amount of cold food into hot oil cools the oil too quickly. Newton’s Law of Cooling works both ways. It has the same effect in baking. Putting several loaves in the oven at once cools the oven, and each loaf shades the other from one of the hot oven walls.
You can compensate in several ways. You can raise the temperature of the oven. You can blow the hot air around inside the oven, as in a convection oven, so that the food cooks more from the hot air than from the heat radiated from the oven walls. You can cook for a longer time. You can also put big slabs of stone in the oven, called thermal mass, that preheat along with the oven walls and take longer to cool. The extra thermal mass counters the mass of the cold food.
Doubling a recipe that involves foam creates another problem besides the low thermal conductivity of the foam: the weight of the top half of the food presses down on the bottom half, and the bubbles get progressively smaller the closer you get to the bottom. This makes the bottom denser than the top, so they cook at different rates.
You may not always have a pot or pan that is exactly the right size. For a soup, this may not matter much, as long as the bottom of the pot is evenly heated. Making a soup in a skillet is possible, but it may require more stirring to prevent the food from sticking to an unevenly heated bottom, and it will lose water faster, since there is more surface for evaporation.
If the stove is capable of delivering heat effectively to the larger surface, the soup may actually cook faster. This may not be desirable if the flavors need time to blend, or it may be just the thing for reheating yesterday’s stew.
In baking, you can use what you’ve learned earlier to adjust a recipe to the size of pan you have available. Pay attention to the surface-to-volume ratio, and adjust the temperature and the cooking time if you can’t keep the ratio the same as the recipe calls for. If your pan is too small, consider making the dish in two batches. If it is too large, you might scale up the recipe and freeze what you don’t eat today.
Different pots and pans conduct heat differently. A cast iron skillet does not conduct heat as well as copper or aluminum, and it may develop hot spots where the heat is applied. You can test a frying pan for heat conductivity and evenness of heating by sprinkling a fine layer of flour in the pan and heating it. If the flour browns evenly all over the bottom of the pan, the combination of stove and pan are effective at spreading the heat. If some areas brown first, you can see where the hot spots are. That pan will need more careful stirring when used for anything but boiling water.
The cast iron pan is much heavier than an aluminum pan or a thin stainless steel pan with a copper bottom. Once it gets hot, it will retain the heat longer due to this extra thermal mass. If you want to present your fajitas at the table still sizzling in the pan, the cast iron pan is the one you want.