6

How puzzling all these changes are! I’m never sure what I’m going to be, from one minute to another.

—Lewis Carroll, Alice’s Adventures in Wonderland

I MUST ADMIT that I am having real trouble with the counterintuitive nature of the quantum world. Apparently, this is a good sign. The quantum physicist Niels Bohr once declared, “If quantum physics hasn’t profoundly shocked you, you haven’t understood it yet.”

Richard Feynman went even further, declaring, “no one understands quantum physics.” In a keynote address he gave in his sixties he admitted, “Might I say immediately that we have always had (secret, secret, close the doors!) a great deal of difficulty in understanding the worldview that quantum mechanics represents. I still get nervous with it.”

The mathematician in me hankers after some mechanism that will tell me when my pot of uranium is going to spit out its next particle. Newton’s equations held out the exciting possibility that if I know the momentum and position of a particle, then the equations of motion will tell me the complete behavior of that particle into the future. And if I repeat the same experiment with another particle located at the same point with the same momentum, it will repeat the path of the first particle.

The hope that we could ever really know the future was fundamentally crushed by the discoveries made by Heisenberg in 1927. He revealed that it doesn’t actually make sense to say, “I know the momentum and the position of a particle at the same time.” There seems to be an elastic relationship between knowing the location of a particle and knowing its momentum. If I measure the position of a particle with increasing precision, it seems to lead to its momentum having a whole range of different possible values. This is the content of Heisenberg’s famous uncertainty principle. And as we shall discover, Heisenberg’s uncertainty principle accounts for why the uranium sitting on my desk is randomly chucking out particles.

Heisenberg himself expressed well how important it is to be ready to reset your view of the world in light of new revelations: “Whenever we proceed from the known into the unknown we may hope to understand, but we may have to learn at the same time a new meaning of the word ‘understanding.’” Quantum physics isn’t about figuring out answers to old questions, but about challenging the questions we are allowed to ask.

Suppose I take one of the particles inside my uranium. If I know that the particle is at rest, it turns out I can’t know where it is located. Indeed, there is a chance that when I look, I could find it anywhere across the universe. But if I try to pin down exactly where this particle is, I suddenly lose my handle on how it is moving. What appeared to be a particle at rest can suddenly find itself moving in any direction.

This seems totally crazy. If I throw my die through the air, I don’t expect that watching it fall will suddenly cause it to fly off in a totally new direction. This intuition, as it turns out, applies only to things with a large mass. When the mass is as small as an electron, anything can happen. If I pin down the location of the electron to within the radius of an atom, its speed could change by as much as a thousand kilometers per second in any direction.

It’s like trying to install a strange quantum carpet: every time I pin down one end of the carpet, the other end pops out.

To get a feel for this elastic relationship between position and momentum, let’s return to my screen with the slits. I’ve been exploring the strange behavior of a particle fired at a screen with two slits in it. The tension between its position and momentum has actually already revealed itself in the behavior of the particle as it passes through a single slit. I get some diffusion of the particles as they pass through the single slit. But why should just one electron passing through the slit be deflected at all? Why doesn’t it sail straight through? The trade-off between knowledge of position versus knowledge of momentum explains the diffusion I see.

If I can arrange for the electron to be fired from a distant source, which ensures that it passes through the slit, then I will know that there was no movement in the direction perpendicular to the slit. This means that as the particle enters the slit, I know the up–down momentum is zero.

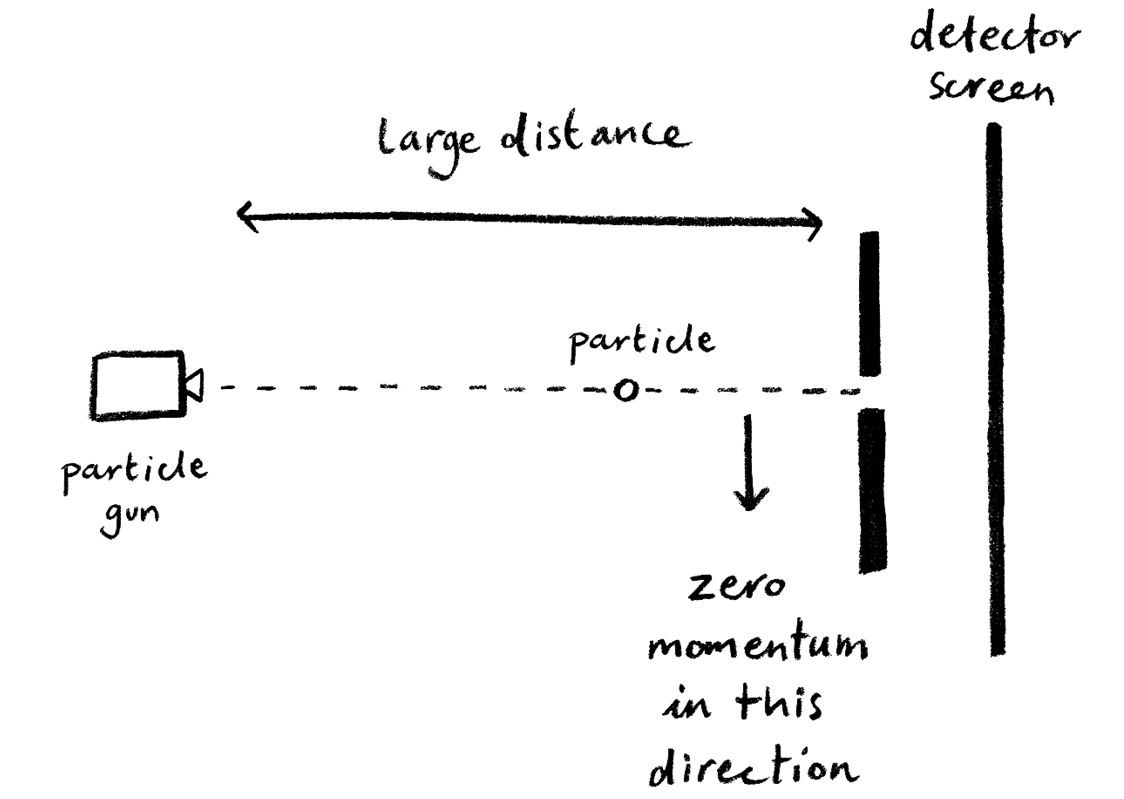
If I think of the electron as a point particle, then it either passes cleanly through the slit or it doesn’t pass through it at all. If it passes through the slit, I have very precise knowledge of its position and should be able to predict precisely where it is going to hit the screen. The momentum was zero in the direction perpendicular to the slit before the electron entered the slit, so it should hit a region on the detector screen whose width is precisely the width of the slit. So why, as I fire more and more electrons through the slit, do I get the same diffusion pattern that I see for waves hitting the detector plate? Why aren’t they all arriving in a region that is the same width as the slit?

Heisenberg’s uncertainty principle asserts that any measurement that involves determining the precise position of the electron results in a new indeterminacy in the value of the momentum. So if the electron has passed through the slit, I can know the location of the electron within a margin of error given by the width of the slit. As the width of the slit is reduced, that margin of error decreases. But this causes the diffusion pattern to get wider and wider. Why? Because the value of the momentum is affected. While it was zero, once the electron emerges with its position narrowed down, the momentum becomes indeterminate. I’ve pulled the quantum carpet down in the position end and caused the momentum end to pop out.

This is a very strange situation. To top it off, the precise impact on the momentum is something I can’t calculate in advance. I can measure it only at a later date. What I can know is a range of possible values in which I can expect to find the momentum once observed. Not only that, it now seems that if I repeat the experiment, I have only a probabilistic mechanism to determine what the momentum might be.

QUANTIFYING UNCERTAINTY

Heisenberg’s uncertainty principle isn’t some wishy-washy statement. It actually quantifies the loss of knowledge. Once I know the position of the electron with high precision, the momentum of the electron as it emerges can vary statistically around the average value of 0. I can’t know what value I’ll get when I measure the momentum, since that is still undetermined, but I know that statistically the possibilities for the momentum will be distributed on either side of the average value of 0. I can measure the spread of this distribution with something called the standard deviation, denoted as ∆p, a statistical measure of the spread of possibilities. The greater the spread, the larger ∆p, and the more uncertain I am of the value of the momentum.

Following Heisenberg’s original paper detailing this strange inverse relationship between knowledge of position and knowledge of momentum, Earle Kennard and later Howard Robertson mathematically deduced the trade-off in knowledge. If the spread of possibilities of the position has a standard deviation of ∆x, and the standard deviation for the spread of momentum is ∆p, then these two values satisfy the following inequality,

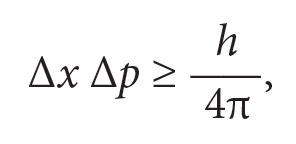
Where h is Planck’s constant, which we came across when considering the energy of a photon of light. It is a mathematical consequence of quantum physics that the more knowledge you gain of the possible locations of a particle, the more this results in the possibility of the momentum being spread over a larger range of values. This is exactly what happens in the case of the electron passing through the single slit.

The order in which I do my measurements matters—the act of measuring position and momentum are described mathematically by two operations that give different answers if you do them in a different order. This idea can be illustrated using my casino die. Suppose I place my die on the table with the 1 on top, as illustrated on the next page. Now I am going to rotate it by a quarter turn around the vertical axis running through the top face and will follow that by a quarter turn through a horizontal axis running through one of the side faces. The top face now has a 5 showing. If I return the die to its original position and repeat the moves but in reverse order, horizontal axis spin followed by vertical axis spin, I get a different outcome. Now there is a 4 showing on the top face.

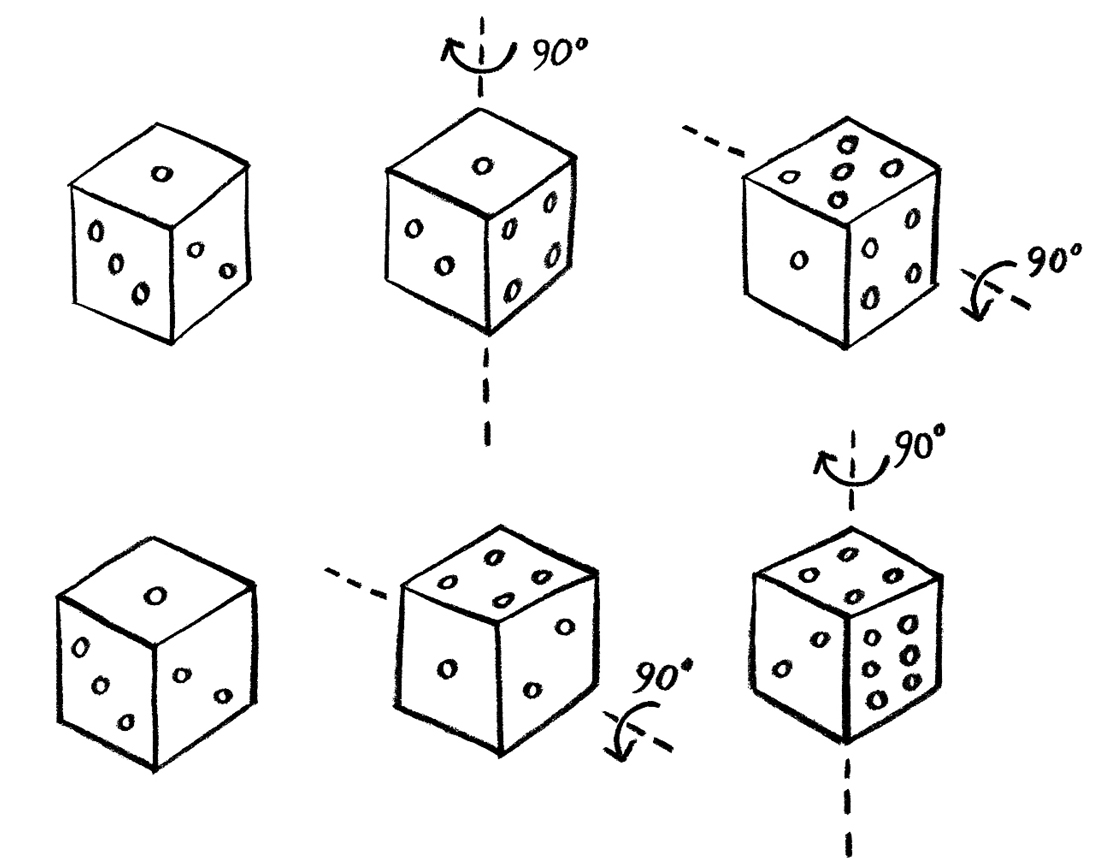
Any measurements that have this property—such that when you translate them into mathematical operations it matters what order you do them in—will give rise to an uncertainty principle. This is simply a mathematical consequence of a property called non-commutativity.

The math underlying quantum physics accounts for much of its counterintuitive nature. As I’ve buried myself in books and papers explaining quantum physics, I sometimes feel like I’ve entered a labyrinth. I thought I knew where I was before I started my journey. I’ve worked my way logically through the twists and turns of the labyrinth, relying on my math skills to lead me, as the walls are too high for me to intuit anything about the world beyond. The trouble is, once I make it out, where I’ve ended up doesn’t look anything like where I started.

Quantum physics is a rabbit hole. Once you fall through it you have to reset your vision and formulate a new language to navigate this looking-glass world. For better or worse, that language is mathematics.

But can we trust the math? In this case, yes. The behavior predicted by Heisenberg’s uncertainty principle has been confirmed. In a paper published in 1969, American physicist Clifford Shull described the results of firing neutrons at slits of decreasing width. As the slit narrowed, the increased knowledge of the location of the neutrons resulted, as theory predicted, in a greater spread of possible values for the momentum. When the neutrons arrived at the detector plate, they were spread in a distribution whose standard deviation corresponded exactly to that predicted by the equation of Heisenberg’s uncertainty principle.

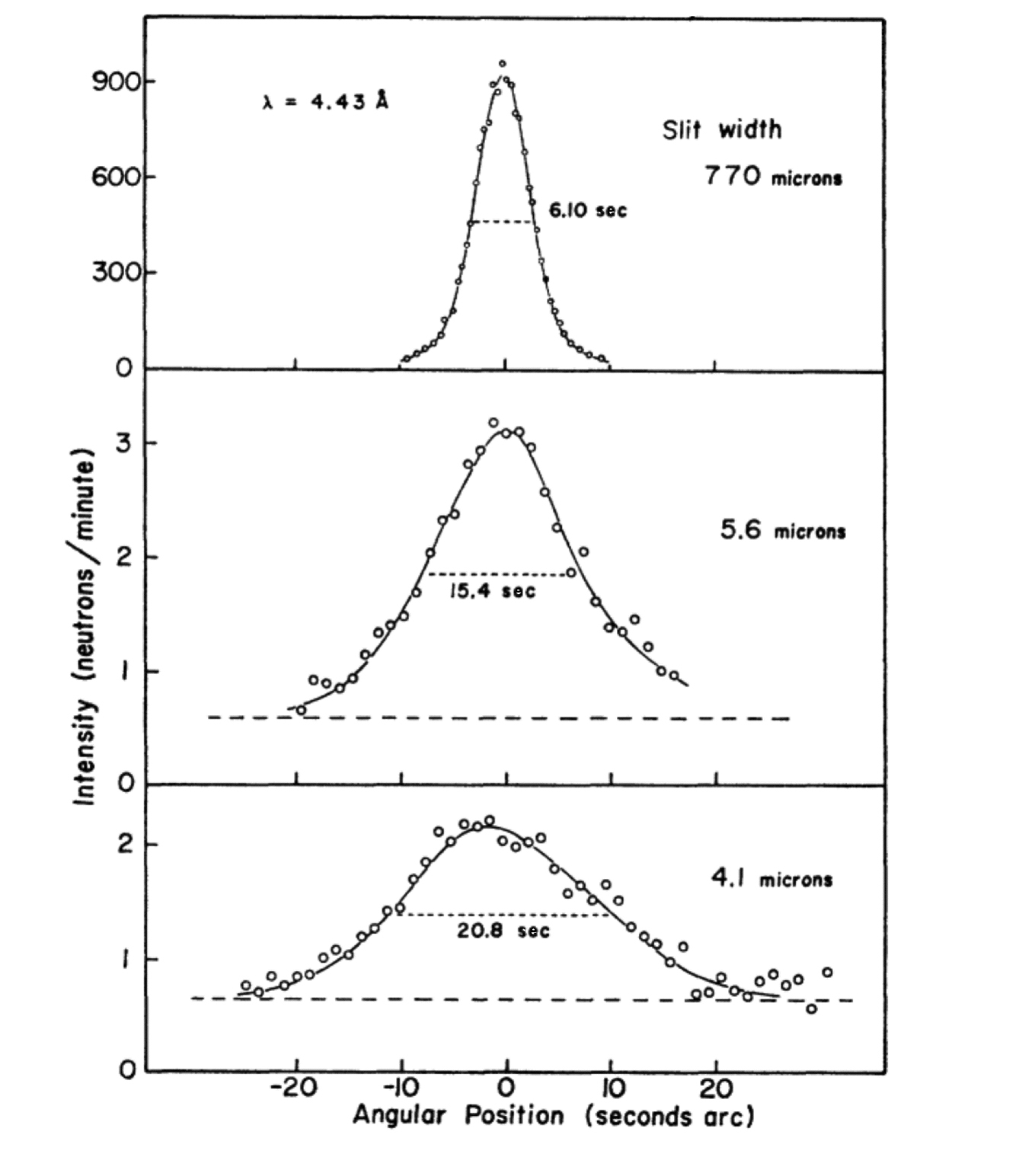
Clifford Shull’s experiment confirmed that as the size of the slit is decreased, the statistical spread of the locations of the neutrons increases.

Visit bit.ly/2m8g2vg for a larger version of this diagram.

An increase in the known must always be traded off against a corresponding increase in the unknown. But this indeterminacy can have some unexpected consequences. If I trap an electron in a very tiny box, its position is known to a high degree of accuracy. That results in the possible values of the momentum being spread over a huge range. As soon as I have measured the momentum, you might say that I now know both the position and the momentum. But it actually results in the position becoming indeterminate, so much so that I get an effect called quantum tunneling, whereby the particle that I thought was trapped inside a box can suddenly appear outside the box. This phenomenon is responsible for the radiation of the uranium that is sitting on my desk.

An alpha particle, consisting of two protons and two neutrons, can be found inside the nucleus of my uranium. In general, alpha particles don’t have enough energy to escape the confines of the nucleus. But if we follow Heisenberg’s uncertainty principle and think of the nucleus as a box, we will find that the position of these particles is not so clearly defined. In fact, there is a chance that they might be located outside the nucleus. This uncertainty about position is responsible for the possibility of radiation.

OBSERVATION IS CREATION

There have been many attempts to explain the uncertainty principle as a consequence of the act of observation. If you want to see where a particle is, you have to fire a photon at it, and this will kick the particle, giving it an unknown momentum. Beware of such explanations! They sound attractive, but they are misleading. In the description I gave above of the electron passing through a single slit, I did not need to fire any photons at the electron to alter its momentum. It was purely the act of the electron passing through the screen that gave me new knowledge of its location. There was no direct interaction with the particle that kicked it in one direction or the other. Apparently this misleading description of photons of light kicking the particle goes back to Heisenberg’s original paper. He needed to include it in order to persuade his skeptical editors to publish the paper.

What does Heisenberg’s uncertainty principle really tell us? Is it that we can never know the precise location and momentum of an electron? Or is it that such things don’t exist? Or that observation is creation? His own formulation in this instance is that reality is created by our observations: “I believe that one can formulate the emergence of the classical ‘path’ of a particle as follows: the ‘path’ comes into being only because we observe it.”

For some time I’ve found it very hard to believe that fundamental properties like position and momentum come into existence only once they are measured. The momentum of an electron might change after it passes through the tiny slit, but surely it must have some precise value even before I measure it? I can accept that I might not know what that value is until I determine the momentum, but there are lots of things I don’t know before I measure them. Quantum physics, however, is trying to tell me that this belief in the existence of a precise value before measurement is a mistake. Can it really be my act of measurement that produces the reality of this particle?

It turns out I am in good company. Einstein (among others) tried to challenge the idea that things like momentum and position are indeterminate until they are observed. Surely, he argued, these things really do have explicit values as the particle flies through the vacuum. We might not know what they are, or have the machinery or knowledge to work them out, but they still exist. The argument is that we shouldn’t confuse epistemology with ontology. I may not be able to know both momentum and position (epistemology), but that doesn’t mean they don’t exist (ontology).

I finally, reluctantly, had to give up on this intuitive belief when I read about Bell’s theorem. In 1964, Northern Irish physicist John Bell definitively proved that it was impossible for certain properties of a particle to exist prior to a measurement being taken. Try to make them exist prior to measurement and everything ends in a contradiction. Bell showed that it was impossible to assign a value to account for every possible measurement without creating contradictory results. It’s like trying to do a Sudoku puzzle with a mistake in it. However hard I try to assign values to all the squares, I will always get a row or column with the same number twice.

Given that Bell’s theorem is as mathematically robust as they come, I’ve had to concede that the act of measuring truly seems to determine the properties of my particle. But I’m still deeply suspicious that the outcome is as random as current theory would have us believe.

I must admit to a sneaky feeling that quantum physics is a stopgap on the way to a more complete understanding of the behavior of fundamental particles. Surely there must be some mechanism deciding when my lump of radioactive uranium is going to spit out an alpha particle, or where the electron will hit after passing through the double slits.

Einstein certainly thought so, leading to his famous quote: “Quantum mechanics is very impressive. But an inner voice tells me that it is not yet the real thing. The theory produces a good deal but hardly brings us closer to the secret of the Old One. I am at all events convinced that He does not play dice.”

Einstein believed that there must be something behind the veil that we have yet to penetrate. Even if we can’t access it, he believed there must be some internal mechanism at work that determines the outcome. I am happy to concede that this mechanism may follow a model of randomness, just as the throw of my die does. But there must be something that determines the outcome. Perhaps the particles inside my pot of uranium have some little internal ticking clock, so that if the second hand is between zero and thirty when I measure it, the uranium will emit radiation; but if it’s between thirty and sixty, then no radiation is detected.

The trouble is that a scenario first dreamed up by Einstein and his colleagues Boris Podolsky and Nathan Rosen revealed that if there is such a mechanism, then part of it could be on the other side of the universe. The scenario that Einstein, Podolsky, and Rosen cooked up involves the idea of quantum entanglement. It is possible to create two particles whose properties are entangled such that if I measure the properties of one particle, it will force the result to be mirrored by the other particle. It is a bit like having two casino dice, and whenever one lands on a 6, the other must too. It would be pretty difficult to rig up such an entangled pair of dice, but in quantum physics it is entirely possible to create such entangled and dependent particles. These entangled particles in turn reveal the very strange properties of any hidden mechanism that could determine how they will behave when measured.

To demonstrate the weird nonlocal nature of such a mechanism—if it exists—the experiment sends the two quantum dice in their entangled state off to opposite sides of the universe. If I then measure the first quantum die, it instantaneously determines which face is showing on the other at the opposite side of the universe. Some people, Einstein included, had a real problem with this “spooky” action at a distance. He felt that there might be a way for the roll of the dice to be preset before the particles head off to opposite ends of the universe. But that was before Bell proved his theorem, which tells me that it is impossible to preset the properties of a quantum particle in advance of measurement. Remember—measurement is creation.

The real challenge is to understand how creation at one end of the universe can instantaneously create a new state for the second particle at the other end. Because if there is some internal mechanism at work determining the outcome of the second particle, that mechanism has just been altered by something that has happened on the other side of the universe. It cannot be localized.

Einstein already expressed his concerns at this “spooky action at a distance” with the double-slit experiment. How does the photographic plate know not to record an electron in one location if it is to be detected at a second location? There seems to be an instantaneous collapse of the wave function with no cascade effect from the point of observation across the result of the plate.

In this new case I have two particles, but because they are entangled in some sense, they are described by one wave function, which makes them not dissimilar to the particle being detected in the double-slit experiment. The two particles must be considered as one holistic unit. Bell’s theorem means that the properties of the particles can’t be preset before they travel to the ends of the universe, which in turn means that any mechanism determining their properties must span the entire universe.

So if there is a mechanism deciding when my radioactive uranium emits radiation (as my deterministic soul yearns for there to be), it will have to span the universe. It can’t just be an internal mechanism in the heart of the blob of uranium on my desk.

Many scientists are not as keen as I am to eliminate the possibility that the behavior of my uranium is random, fueled by the hope that this small chink in the knowable will allow something many people cherish to enter the scientific picture: free will. Some have argued that if there is genuine randomness in quantum physics it is evidence of free will at work in the universe. Humans may not be totally free, but these microparticles seem able to do what they want . . . within reason.

Some religious thinkers contend that quantum physics allows room for an external agent to act in the world and influence its course. Provided that the results over the long term are in line with what we’d expect from randomness, there seems to be room for an agent to determine individual outcomes. This would take advantage of our present inability to explain how the macroscopic world of measurement interacts with the quantum world. So is the unknown of quantum physics home for a theistic God? If I wanted to get anywhere in my attempt to understand whether God could hide in the equations of quantum physics, I would need to talk to someone who was as much at home in a laboratory as in a cathedral. So I made a trip to Cambridge.

THE VEGETARIAN BUTCHER

John Polkinghorne learned his physics at the feet of Paul Dirac in Cambridge and then with Richard Feynman and Murray Gell-Mann at Caltech. You can’t ask for better teachers than that. His research has, among other things, helped to confirm the existence of quarks. Polkinghorne is back at his alma mater, so I arranged to meet him at his home in Cambridge. Having done a five-year research stint at Cambridge, I always enjoy a chance to visit, even if my heart is with Oxford. Polkinghorne’s decision to become an ordained priest, after a quarter of a century pushing the limits of quantum physics, makes him the perfect person to explore the theology in the quantum unknowable. To many it seemed like a dramatic career change. As he explained the decision, “I didn’t leave science because I was disillusioned, but felt I’d done my bit for it after about 25 years. I was very much on the mathematical side, where you probably do your best work before you’re 45.”

Yikes. I hate it when people say that. I’ve always clung to the hope that it is a myth that mathematics is only for the under-forties. But then I guess, being on the wrong side of that divide, I would say that. So long as there are still unanswered questions to struggle with, that is what drives me on. And I’ve still got plenty of those unanswered questions on my desk. But I can certainly understand the desire to set yourself new challenges . . . like my current attempts to understand quantum physics. For Polkinghorne, the new challenge was getting ordained, and he often jokes about the seemingly contradictory natures of the two professions he has dedicated his life to. “People sometimes think that it is odd, or even disingenuous, for a person to be both a physicist and a priest. It induces in them the same sort of quizzical surprise that would greet the claim to be a vegetarian butcher.” He himself thinks of the two roles as a harmonious combination. “The basic reason is simply that science and theology are both concerned with the search for truth.”

I wondered whether there were any questions that he thought were beyond the reach of either discipline.

“There are two sorts of questions that science cannot answer,” he offered. “Some of them arise out of science itself. The first is something we’ve learned from quantum physics, which is that although the world is orderly, it’s also cloudy and fitful in its character and we don’t have access to that clear, unquestionable post-Newtonian world that seems to be sitting there.

“But there are also questions that by their very nature don’t lie within science’s purview to answer. I think science has been tremendously successful and I have enormous respect for it, but it’s achieved its success by limiting its ambition. Essentially, science is asking a single question about how things happen: What is the process of the world? And it deliberately brackets out by its nature questions of meaning and value and purpose.”

That’s not the first time I’ve come across this supposed dividing line. Science does the “how” and religion does the “why.” It’s an attractive sound bite, but I think it’s a fundamentally flawed take on science.

Science tackles a lot of “why” questions. Why is my pot of uranium radiating alpha particles? Why do the planets orbit the sun on the same two-dimensional plane rather than at arbitrary angles to each other? Why do bees make their hives in hexagons? Why does the population of lemmings plummet every four years? Why is the sky blue? Why can’t things travel faster than light?

Polkinghorne tried to tease out for me the difference he sees in the two approaches.

“My favorite homely example is that you come into my kitchen and you see the kettle boiling. If I put on my scientific hat, then I explain that it is boiling because the burning gas heats the water, and so on. But I can take off my scientific hat and say the kettle is boiling because I wanted a cup of tea and would you like to have one?”

I decided to take him up on his offer of tea. As it brewed, Polkinghorne continued.

“I don’t have to choose between those two answers, and if I am to fully understand the phenomenon of the boiling kettle, I have to answer both questions: how it’s happening and why it’s happening.”

I agree with Polkinghorne to some extent that science has limited its ambitions. Fermat’s Last Theorem is frankly easier to grasp than the logic of my cat’s behavior or the next move my son is going to make. But that doesn’t mean that science can’t hope ultimately to understand the complexities of a cat or the vagaries of human desire.

In my view, the science-versus-religion debate has fallen prey to our terrible desire to compartmentalize everything, the silo mentality that says, “This is science and this is theology and this is art and this is psychology.” The exciting thing is that we have developed a multitude of discourses to navigate our environment. The evolution of everything in the universe might be reducible to the solutions of Schrödinger’s wave equation—and that includes Polkinghorne’s decision to boil his kettle—but while math is a great language for describing the behavior of my pot of uranium, it isn’t the right language to explain the migration of a flock of birds, the thrill of listening to Mozart, or the immorality of torture.

Polkinghorne concurred that there were real dangers of a too-reductionist take on reality: “Sometimes when I’m having arguments with firmly reductionist friends who say that physics is everything, I say, first of all, ‘What about mathematics?’ and secondly, ‘What about music?’ Of course, music is just vibrations of the ear, but when you’ve said that you’ve said all that science can say about music, but you certainly haven’t said all that can be said about music. It does seem to me very important that one doesn’t just take a reductionist ax and chop everything down.” I pushed him on his first example of a question science can’t answer. Does he really believe that quantum physics means that I can’t know when my pot of uranium is going to spit out its next particle? Is it really just chance?

“It’s very unsatisfactory that there is this sort of lottery going on. A casino, in effect. Most quantum physicists who are busy doing the numbers have just got used to that, but I think it is unsatisfactory. The question is whether it is epistemic or ontological.

“Epistemological problems have an answer, but you don’t happen to know it. But ontological things are situations where you could not know it. And that’s the traditional interpretation of quantum theory—you cannot know.

“In the casino, we know it’s essentially epistemic. There are tiny effects that influence things. My feeling is that if the problems of quantum theory are epistemic, then you need to have some notion of how that epistemological frustration arises, what stops you from finding an answer. I think it’s sensible to try to push the issue ontologically as far as you can. We haven’t got there yet.”

Most quantum physicists believe that before you observe a particle, it is in a superposition of states described by the wave function, and that observation by macroscopic apparatus causes a jump in the behavior. The particle now has one state, and the wave function encodes the probability that you will find the particle in one state rather than another. There is no attempt to explain the jump. This is called the Copenhagen interpretation, after the home of its principal proponent, the Danish physicist Niels Bohr. Basically it’s the “Shut Up and Calculate” school of quantum physics.

“Although I sign up to the Copenhagen interpretation of quantum theory, I don’t think it’s intellectually satisfying,” Polkinghorne confessed. “At the end of the day all these things come down to someone saying ‘and then it happens.’ It’s somehow produced by the intervention of macroscopic measuring apparatus. End of discussion. But that’s just winning by definition. It is a problem. There are still puzzles.”

Given Polkinghorne’s belief that there is a God acting in the world, I wondered whether he thought the unknown of this collapsing wave function was a window for his God to act.

“I don’t think that God is on hand to decide whether the nucleus in your uranium decays. There is some sort of mechanism . . . no, ‘mechanism’ isn’t quite the right word . . . some sort of influence that sorts this thing out. One of the paradoxes of quantum theory is that here we are eighty years later and we still don’t understand it.”

I wondered why, as I discovered in the First Edge, Polkinghorne had chosen chaos theory, rather than his home territory of quantum physics, as the unknown through which his God might act.

“There was a period of about ten years when the science and theology communities were wrestling with these forms of agency. Of course they didn’t solve the problem, because that would have been a very ambitious project. There were a lot of people, especially on the West Coast of America, who put their money on quantum theory explaining everything. That appeared to me just a little too slick. To counterbalance that, I lurched a bit too far in the other direction. I don’t think chaos theory is the whole solution. It’s really just the suggestion that the physical universe is orderly but looser in its order than Newton would have thought.”

But he certainly isn’t dismissive of the implications of quantum physics.

“The discovery of the intrinsic unpredictability in quantum theory shows us that the world certainly isn’t mechanical and therefore we certainly aren’t automata in some trivial and unbelievable sense.”

It’s intriguing to consider that an agent trying to dictate the course of the future using the unknown of quantum physics would have the opportunity to act only when measurement is made. Until the measurement causes a phase change, the equations of quantum physics are totally deterministic, rolling along in a linear, nonchaotic fashion, with no room for agency. This is one reason why religious physicists like Polkinghorne are not particularly enamored of the God of quantum physics.

As I drive back from Cambridge, I find myself lingering on this question of epistemology versus ontology, as it seems central to navigating what quantum physics tells me about the limits of human knowledge. Is it like my casino die? Although we can’t know the precise starting point for the throw, we don’t question whether the dice exist. But quantum physics questions whether I can talk about my pot of uranium as having a well-defined initial state. As Heisenberg put it, “The atoms or elementary particles themselves are not real; they form a world of potentialities or possibilities rather than one of things or facts.”

Although Heisenberg’s uncertainty principle seems to create an unknown or gap through which God can slip back in, it may actually fill another gap that is the spark for most people’s belief in a creator. One of the big unknowns is this: Why is there something rather than nothing? My pot of uranium arrived in the post, sent via Amazon from Images Scientific Instruments in Staten Island. But if I keep reaching back, trying to find the ultimate origin of my uranium, I am eventually going to hit an unknown. The need for some explanation of this unknown is at the heart of many cultures’ concepts of God. But what sort of answer is that?

I think most scientists who talk of God have in mind something that answers the seemingly unanswerable question of where all this stuff came from. Once the universe is up and running, they are happy to engage their scientific brains to understand how the stuff we have behaves. They are not looking for God to intervene in the world. This is what is often called deism rather than theism. This sort of God is very much one that can be equated with “things we cannot know.”

Of course, if we try to describe what this answer actually looks like, we encounter the problem of infinite regress. If you think that something is responsible for creating the universe, then you quickly hit the question of who created that something. Of course, that “who” is part of the problem, because we have a terrible urge to personify this concept.

So this is why many talk about transcendent definitions, things that can’t be articulated; to avoid the problem of infinite regress, they avoid even an attempt to articulate what the answer might look like. It’s just something that is unknown and transcends our attempts to know it. This is the God the presenter of the Sunday morning program on BBC Northern Ireland whom I tangled with asked me about.

But if God is defined as something that can’t be articulated, does it have any potency at all to act? If it can’t interfere, or influence, if it can’t be articulated and described, why do we need it? This is why mythmakers have had to mold their gods into forms that can be articulated, recognized, often personified. A God that is too transcendental loses its potency. For many early religions, this was precisely what happened to the idea of the High God or Sky God. As religious commentator Karen Armstrong writes in her book The Case for God, “He became Deus otiosus, a ‘useless’ or ‘superfluous’ deity, and gradually faded from the consciousness of his people.”

As the theologian Herbert McCabe declared, “To assert the existence of God is to claim that there is an unanswered question about the universe.” But he also warned that the fault of religion was always to make this God into a thing rather than a philosophical idea. The problem, he believed, is that religion far too often commits idolatry by trying to engage too personally with this concept of God.

The trouble is that an undefined, unknowable, transcendent concept is too abstract for many to engage with. It can’t offer the sort of consolation that many seek. So perhaps it is inevitable that God’s potency depends on becoming a little less transcendent, and more tangible.

ZERO EQUALS ONE MINUS ONE

The question of why there is something rather than nothing may not be as unanswerable as we think. As soon as you have a bit of empty space, quantum physics is going to start filling it with stuff. The version of Heisenberg’s uncertainty principle that I have explored so far looks at the relationship between position and momentum. But there are other physical concepts that are similarly entangled.

For one thing, Heisenberg’s uncertainty principle also connects the measurement of energy and time. If I look at what is happening in an apparently empty bit of space, then decreasing the time period in which I examine the space increases the uncertainty of the energy content—which means that empty space can never be truly empty. Over very short periods of time there is the chance of energy fluctuations. Since energy can change into mass, this results in particles spontaneously appearing from the vacuum. Most of the time, they annihilate each other and disappear back into the void, but sometimes things survive. And this gives us a mechanism for getting something out of nothing.

But where does this energy come from? Doesn’t its sudden appearance contradict the concept of conservation of energy that physics holds dear? Some propose that the total energy content of the universe is actually zero, so no one is cheating the system. The key here is that gravity provides a negative energy content. So the universe can emerge from zero energy—from nothing—because what emerges is a combination of positive and negative energy. We are just seeing the equation 0 = 1 – 1 at work.

It might seem a bit bizarre to call gravity negative energy, but think about putting a large mass like an asteroid next to the Earth. As the asteroid falls toward Earth it gains kinetic energy, but the gravitational pull is also going up because gravity increases the closer two masses are to each other. So to maintain a constant energy, this gravitational potential energy is negative and balances the increase in kinetic energy.

According to Heisenberg’s uncertainty principle, it follows from the fact that space exists that you will get particles appearing from nothing. You don’t have any need for a creator. Quantum fluctuations mean that we are seeing something appearing from nothing all the time. As we shall see in the Fifth Edge, this is how Hawking explained why black holes radiate particles. Nothing becomes a particle and an antiparticle—one gets trapped in the black hole and the other radiates away. So quantum physics already provides a partial answer to the something-from-nothing question.

However, you do at least need a stage on which to play this quantum game. Some equate empty space with nothing. But that is a mistake. Three-dimensional empty space, a vacuum, is still something. It is an arena in which geometry, mathematics, and physics can play out. After all, the fact that you have a three-dimensional rather than a four-dimensional empty space already hints at the evidence of something. Nothing does not have a dimension. The ultimate challenge, therefore, is to explain how quantum fluctuations might produce space and time out of genuine nothingness.