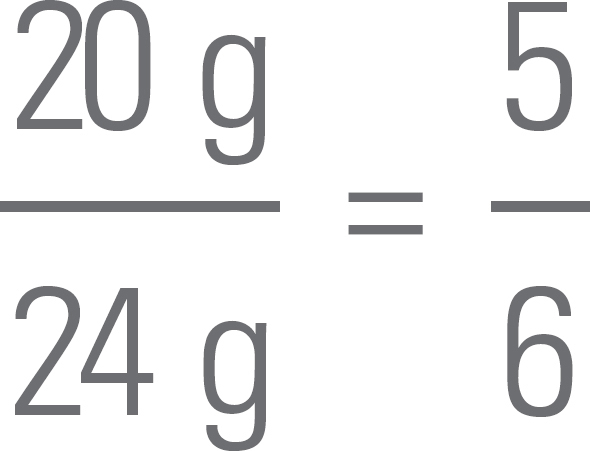Chapter 5 Chemical Reactions and Stoichiometry

Chemistry is a science largely concerned with reactions, or how molecules of one or more substances are converted to molecules of a different substance(s). This chapter discusses formula (or molecular) weight, empirical formulas, and percent composition; moles and molar mass; balancing chemical reactions and stoichiometry; and thermodynamics, which is the role of energy in a reaction: how much energy is given off or absorbed by a reaction and what this can tell us about the reaction.

THE WORLD OF MOLECULES

As we mentioned in Chapter 4, an atom is the smallest particle of an element. Atoms of one element often attract each other or atoms of a different element. If this attraction is strong enough, a chemical bond can result. Chemical bonds join two or more atoms into units called molecules. (We’ll talk more about bonding in Chapter 7.)

Everybody knows the formula of water—it’s H₂O. This formula tells us that, in a molecule of water, 2 hydrogen atoms and 1 oxygen atom are bonded together into a unit. An individual water molecule is the smallest unit of water that exists.

Diatomic Molecules

When a molecule consists of just two atoms (whether they are of identical or different elements), it’s called a diatomic molecule. Some elements exist as diatomic molecules at room temperature and atmospheric pressure (1 atm). For example, oxygen in air exists in the form of O₂. There are seven important elements that exist as diatomic molecules: oxygen (O₂), iodine (I₂), hydrogen (H₂), nitrogen (N₂), chlorine (Cl₂), fluorine (F₂), and bromine (Br₂).

Formula Weights, Empirical Formulas, and Percent Composition

For the SAT Subject Test in Chemistry, you’ll need to know some simple atom-molecule math.

1. Formula Weight: For any molecule, we calculate the formula weight by adding up the atomic weights of all the atoms in the molecule. It’s easy. Take hydrogen peroxide (H₂O₂) as an example.

• The molecule has 2 hydrogen atoms and 2 oxygen atoms. Let’s start with hydrogen.

• The atomic weight of hydrogen is 1 amu. Since there are 2 atoms, we have a total of 2 × 1, or 2 amu.

• Now let’s talk about the oxygen in the molecule. The atomic weight of oxygen is 16, and since there are 2 atoms, the total is 16 × 2, or 32 amu.

• The total is 2 + 32 = 34. The formula weight for H₂O₂ is 34 amu.

Here’s another example. Let’s figure out the weight for sulfuric acid: H₂SO₄.

• The molecule has 2 hydrogen atoms, 1 atom of sulfur, and 4 oxygen atoms. Let’s start with hydrogen.

• The atomic weight of hydrogen is 1 amu. We have 2 hydrogens, so there are a total of 2 amu.

• The atomic weight of sulfur is 32 amu. So we have 32 amu of sulfur.

• Now let’s talk about the oxygen. We know the atomic weight of oxygen is 16 and there are 4 atoms. So we have a total of 16 × 4, or 64 amu.

• 2 + 32 + 64 = 98. The formula weight for H₂SO₄ is 98 amu.

2. Empirical Formula: An empirical formula shows the ratio of atoms within a molecule. To find an empirical formula from a molecular formula, first find the largest whole number by which all of the subscripts in the molecular formula are divisible. Then divide each subscript by that number. Let’s look at a few examples.

• We talked about the molecular formula of hydrogen peroxide, which is H₂O₂. The largest number that can divide into 2 is 2. If we factor a 2 out of both subscripts, we’re left with HO. So the empirical formula for hydrogen peroxide is HO.

• Ethane has the molecular formula C₂H₆. Let’s find the empirical formula. What’s the largest number that goes evenly into 2 and 6? It’s 2. If we divide both subscripts by 2, we get an empirical formula of CH₃.

• How about water? The largest whole number that will divide evenly into 2 and 1 is 1. So the empirical formula for water is the same as its molecular formula: H₂O.

3. Percent Composition: This refers to the percent of a substance contained within a molecule. The test writers may ask you to determine this percent. Here is one example of a question you might see on the test.

What is the percent of oxygen by mass in hydrogen peroxide, H₂O₂?

In order to determine the percent composition of oxygen, you need to find the mass in amu of all of the oxygen atoms in the molecule and compare it with the total formula weight. As shown earlier, each of the two oxygen atoms in H₂O₂ weighs 16 amu. This means that the mass due to oxygen is 2(16) or 32 amu. The formula weight of H₂O₂ is 34 amu. So the percent of oxygen by mass in H₂O₂ is 32by34 × 100%, or roughly 94%. You can then calculate the percent of hydrogen by mass in H₂O₂, which is 100% – 94%, or about 6%.

THE MOLE

What’s a mole? It’s a number, like a dozen or a gross. Dozen, as you know, means 12. Gross means 144. Mole means 6.02 × 10²³. The number 6.02 × 10²³ is known as Avogadro’s number.

We said earlier that atomic mass is measured in atomic mass units (amu); 1 amu is 1/12 the mass of a carbon-12 atom, or a relative mass of 1 gram (g). Each 1 g of a substance has 6.02 × 10²³ amu. Think about what that means. If, for any element, we take a sample whose mass in grams is numerically equal to its atomic weight in amu, the sample has 1 mole of atoms in it. If we take a substance whose mass in grams is numerically equal to twice its atomic weight in amu, we have 2 moles of atoms. If we take a substance whose mass in grams is numerically equal to three times its atomic weight in amu, we have 3 moles of atoms. It’s as simple as that.

For example, helium’s atomic weight is 4 amu, so 4 g of helium contain 1 mole (6.02 × 10²³) of helium atoms, and 8 g of helium contain 2 moles of helium atoms. Carbon’s atomic weight is 12 amu, so in 12 g of carbon there is 1 mole (6.02 × 10²³) of carbon atoms, and in 36 g of carbon there are 3 moles (18.06 × 10²³) of carbon atoms. Got it?

How many moles of oxygen molecules are in 64 g of oxygen gas? Remember that oxygen is diatomic. Each O₂ molecule has a mass of roughly 2(16), or 32 amu, so 1 mole of O₂ molecules would have a mass of 32 g. Thus, in 64 g of oxygen gas, there are 2 moles of oxygen molecules. Now how many moles of oxygen atoms would be present in this 64 g sample? Each O₂ molecule is made up of 2 oxygen atoms, so if the sample contains 2 moles of oxygen molecules, it contains 4 moles of oxygen atoms.

Note that the lower the molar mass is for a given element, the more moles there will be present in 1.0 g of it. Hydrogen has a molar mass of 1.0 g/mol, and helium has a molar mass of 4.0 g/mol. 1.0 g of hydrogen atoms would thus represent one mole of hydrogen, but 1.0 g of helium atoms is only 0.25 mol of helium! This is important—moles represents the amount of substance that reacts (or is produced) during a chemical reaction, and all calculations in chemistry revolve around moles!

Converting Mass Composition to Empirical Formula

Now that you know what an empirical formula is, we’ll tell you how to figure out an empirical formula from the percent composition of a molecule. For example, the test writers might tell you that some unknown substance is made up of approximately 75% mercury and 25% chlorine, and they might ask you to take this percent composition and figure out the substance’s empirical formula.

Here’s how you do it.

1. Imagine, first, that you have 100 g of the substance.

2. If you have 100 g of the substance, and it’s 75% mercury by mass, then you’ve got 75 g of mercury, right? Since the atomic weight of mercury is about 200 amu (which means that 1 mole of mercury atoms weighs 200 g), you’ve got 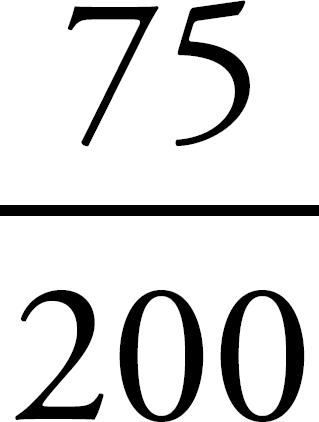75by200 mole = 0.375 moles of mercury atoms in a 100 g sample.

3. If you have 100 g of the substance, and it’s 25% chlorine by mass, then you’ve got 25 g of chlorine. Since the atomic weight of chlorine is about 35 amu (which means that 1 mole of chlorine atoms weighs 35 g), you’ve got 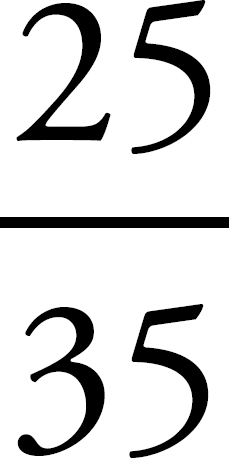25by35 = 0.700 moles of chlorine atoms in the 100 g sample.

4. If you’ve got 0.375 moles of mercury atoms and 0.700 moles of chlorine atoms, then the ratio of chlorine to mercury atoms is 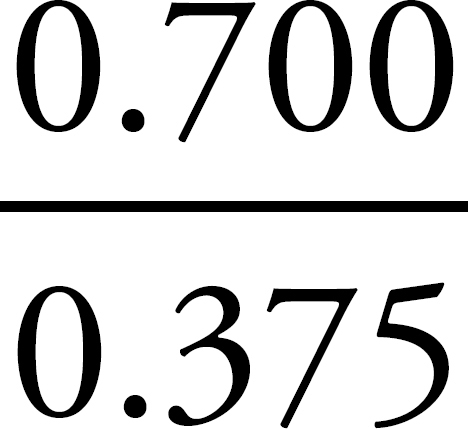93-1 (which is close to 2:1), which means the empirical formula is HgCl₂.

Now review everything we’ve told you about molecules and moles, and answer the following questions. The answers can be found in Part IV.

DRILL 1

Question Type A

Questions 1–4 refer to the following.

(A) N₂O

(B) C₆H₁₂O₆

(D) NO

(E) N₂O₅

1. Is a diatomic molecule

2. Has a formula weight of approximately 108 amu

3. Has an empirical formula that is different from its molecular formula

4. Composition is approximately 60% oxygen by mass

Question Type B

I		II
101. Chlorine is an element	BECAUSE	chlorine exists as unbonded atoms at room temperature and atmospheric pressure.

102. One mole of HBr has greater mass than one mole of NO₂	BECAUSE	the mass of a molecule of HBr is greater than the mass of a molecule of NO₂.

Question Type C

24. The formula for calcium nitrate is Ca(NO₃)₂. What is its approximate formula weight?

(A) 64 amu

(B) 164 amu

(D) 310 amu

(E) 380 amu

25. An unknown substance is found to have a composition of 9% magnesium and 91% iodine by weight. The empirical formula for the substance is

(A) MgI

(B) Mg₂I ₂

(D) MgI₂

(E) Mg₃I₂

CHEMICAL REACTIONS—HOW MOLECULES ARE FORMED, BROKEN DOWN, AND REFORMED

In the course of a chemical reaction, the bonds that hold together the atoms that make up the reactants break. The free atoms then form new bonds with one another to form new molecules—the products of the reaction. Take a look at the chemical reaction below:

C₃H₈(g) + 5O₂(g) → 4H₂O(l) + 3CO₂(g)

What does this equation tell us? Well, two things. On a molecular level, this equation says that 1 molecule of propane (C₃H₈) and 5 molecules of oxygen react to form 4 molecules of water and 3 molecules of carbon dioxide. It also tells us that 1 mole of C₃H₈ reacts with 5 moles of O₂ to form 4 moles of H₂O and 3 moles of CO₂. This equation also indicates the state of each reactant and product: (s) means solid, (l) means liquid, and (g) means gas.

Note that when a substance is dissolved in water to create a solution, it is in the aqueous phase, represented with (aq). This is not the same thing as the liquid phase. If you were to take table salt (NaCl (s)) and heat it until it melted, it would become NaCl (l). However, if you took that table salt and dissolved it into a glass of water, it would create NaCl (aq). Much of chemistry is solution-based, and compounds will frequently be dissolved in water to form aqueous solutions before any reactions take place.

Chemical Equations Must Be Balanced

Look again at the chemical equation we just presented:

C₃H₈(g) + 5O₂(g) → 4H₂O(l) + 3CO₂(g)

This is a balanced equation. How can you tell? For each element on the left side of the equation, multiply the molecular coefficient by the element’s subscript. (Any number that doesn’t appear is assumed to be 1.) For oxygen, in 5O₂, there are 5 × 2 = 10 oxygen atoms. Now do the same for the right side of the equation. In 4H₂O, there are 4 × 1 = 4 oxygen atoms. In 3CO₂, there are 3 × 2 = 6 oxygen atoms. So there are 4 + 6 = 10 oxygen atoms on the right side, and since there are also 10 oxygen atoms on the left side, oxygen is balanced. Now check to see that carbon and hydrogen are also balanced. They are. There are 3 carbons on the left and 3 carbons on the right. There are 8 hydrogens on the left and 8 hydrogens on the right. For each element in a balanced equation, the total number of atoms on the left must equal the total number of atoms on the right.

Balancing Equation Steps:

1. First, start with the smallest answer choice, and plug it in front of what you want to solve for.

2. Next, turn to the other side of the equation and fill in coefficients based on what you started with.

3. If you can’t find whole number coefficients so all the atoms on either side of the equation add up, turn to the next smallest answer choice and repeat.

4. If you can balance the equation, check to make sure there is no common factor for all of the coefficients. For example, 4, 6, and 10 have a common factor of 2. If there is a common factor, divide the coefficients, and you have your answer. If there isn’t, you already have your answer.

On the SAT Subject Test in Chemistry, you may see up to five questions that will show you unbalanced equations and ask you to balance them. Here’s what those will look like.

29….C₂H₄(g) +…O₂(g) →…CO₂(g) +…H₂O(l)

When the equation above is balanced and all coefficients are reduced to lowest whole-number terms, which of the following would be the coefficient for CO₂ ?

(A) 1

(B) 2

(D) 5

(E) 6

Fortunately, these questions are easy to answer if you use the “plug-in” balancing strategy. Start with (A), and put the number 1 in front of CO₂. (Don’t be afraid to write in your test booklet as much as you want. Your test booklet belongs to you, and nobody cares what you write in it; only your answer sheet is scored.) By adding a 1 in front of CO₂, we end up with 1 carbon on the right. Yet, we have at least 2 carbons on the left, so we know that 1 is not the answer.

Let’s try (B). Put a 2 in front of CO₂, and see what happens. We have 2 carbons on the right and 2 on the left. Good. We have 4 hydrogens on the left, so let’s try putting a 2 in front of the H₂O. That gives us a total of 6 oxygens on the right and 2 on the left. Let’s put a 3 in front of O₂ on the left, so we have a total of 6 oxygens on the left.

Now we have 2 carbons on the right and the left, 4 hydrogens on the right and the left, and 6 oxygens on the right and the left. The equation is balanced, and (B) is correct. So, every time a question asks you to balance an equation, use the plugging-in strategy. It can’t fail.

STOICHIOMETRY

Sometimes SAT Chemistry questions will ask you to determine how much product is formed or reactant is consumed in the course of a chemical reaction. These are stoichiometry questions. When you begin a stoichiometry problem, always remember to work from a balanced equation. The coefficients in front of each species indicate the molar ratio between the species. Consider the reaction between ammonia gas and oxygen, which yields nitrogen monoxide and water.

4NH₃(g) + 5O₂(g) → 4NO(g) + 6H₂O(l)

This equation tells us that

• For every 4 moles of ammonia consumed, 5 moles of oxygen are also consumed.

• For every 5 moles of oxygen consumed, 6 moles of water are produced.

• For every 4 moles of ammonia consumed, 4 moles of nitrogen monoxide are produced. In other words, the molar ratio of ammonia consumption to nitrogen monoxide production is 1:1.

How do you put these molar ratios to use? Take a look: If 2 moles of ammonia are consumed, how many moles of water are produced?

The best way to figure this problem out is to use unit conversions. The number of moles in our conversion comes from the coefficients of the balanced equation.

2 moles NH₃ × 97-1 = 3 mol H₂O

We know the moles of NH₃ cancel because they appear in both the numerator and denominator, leaving us with moles of H₂O.

It can get a bit more complicated; we can only use the coefficients in the balanced equation to determine the mole ratio, not the mass ratio. So, if we start with mass, we have to convert to moles first. After we get our moles of product, we can then convert those to the mass of product. Consider this: if 34 g of NH₃ gas reacts, how many grams of NO(g) are created?

This time we have three necessary conversions:

Note that when converting from grams to moles, we use the molar mass of each compound, which is always equivalent to the mass of one mole of that compound. The coefficients from the balanced equation only appear in the mole ratio step, and never in the moles to grams step.

The last thing that you need to know about stoichiometry (for now!) is that if you start with the amount of both reactants, inevitably, one of them will run out first. Once one of the reactants is gone, the reaction will stop. The reactant that runs out first is called the limiting reactant, and the reactant which does not run out is called the excess reactant.

You can mathematically determine which reactant is limiting by seeing which would produce LESS product. Whichever reactant is able to produce less product will always run out first and thus be limiting. When 3 mol of NH₃ is mixed with 3 mol of O₂, the limiting reactant can be determined as follows:

In this case, the oxygen would be the limiting reactant (and 2.4 moles of NO would be produced). We could have gone to moles of H₂O instead of NO if we wanted to; it doesn’t matter which product we choose as long as we’re consistent. It’s important to realize that it’s not always the reactant which is present in lower amounts that will limit. The limiting reactant depends on a number of variables, including the molar mass of each reactant and the mole ratio between the reactants and the products.

THERMODYNAMICS

Entropy

One of the most important things to remember about thermodynamics is that low-energy states are more stable than high-energy states. That’s such a fundamental principle that we’ll ask you to repeat it. Fill in the blank lines:

_________energy states are more stable than_________energy states.

Fundamentally, the universe prefers low-energy states, and also fundamentally, it tends toward disorder. When we talk about disorder, we use the term entropy, which is symbolized by S. Everything tends toward maximum entropy. When we talk about a chemical reaction and the difference between entropy of the products and entropy of the reactants, we use the symbol ∆S. If ∆S is negative, the reaction has lost entropy; the products are more “orderly” than the reactants. If ∆S is positive, the reaction has gained entropy; the products are less “orderly” than the reactants.

The State of Things

Entropy is a measure of randomness, or disorder. To get an idea of what this means, consider the two boxes below. Which one has greater entropy, or disorder? It’s the one on the right.

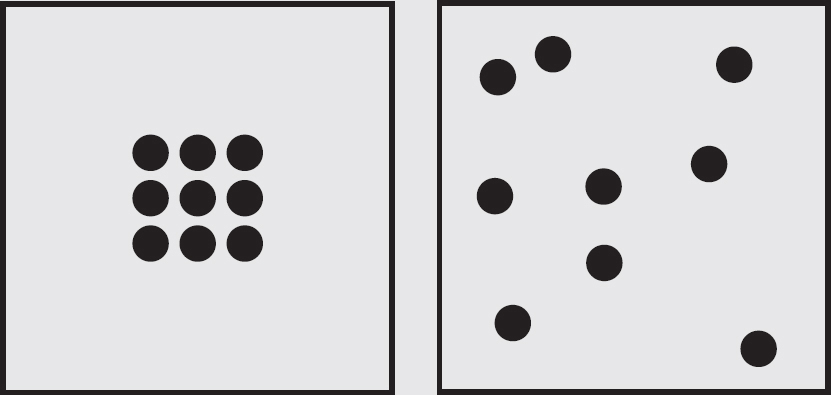
The universe, it turns out, likes disorder. Systems move toward increasing disorder. They also tend to move toward states of lower energy. For the SAT Subject Test in Chemistry, when you see the word entropy, think “disorder,” and realize that, because the universe is lazy, it tends toward maximum entropy. All things in the universe are more stable when they’re in (1) states of low energy, and (2) states of high entropy.

Higher Entropy + Lower Energy → More Stability

Lower Entropy + Higher Energy → Less Stability

Enthalpy

Because the universe tends toward lower energy, chemical reactions that release energy—reactions that set energy free—are favored in the universe. When we talk about the energy states of reactants or products, we use the term enthalpy, which is symbolized by H. High enthalpy means high-energy state, and low enthalpy means low-energy state. So, the universe likes reactions in which the enthalpy decreases—reactions in which ∆H (the change in enthalpy that occurs in the course of a reaction) is negative. These reactions are said to be exothermic, and they result in the release of energy in the form of heat. If, however, the enthalpy of the products is greater than the enthalpy of the reactants, then ∆H is positive and the reaction is said to be endothermic. Endothermic reactions require the input of energy in order to take place.

Energetic Bonds

When we say that the products are at a higher or lower energy than the reactants, we mean the bonds in the products are at a higher or lower energy. If energy is released in a reaction, that energy comes from the potential energy stored in the bonds of the reactants. If the bonds of the products have more potential energy than the bonds of the reactants, we have to supply the difference, and energy is absorbed in the reaction.

Exothermic reaction → energy is released → ΔH < 0 → enthalpy decreases

Endothermic reaction → energy is absorbed → ΔH > 0 → enthalpy increases

Enthalpy is often represented graphically by a diagram often called a reaction coordinate. The reaction coordinate for an exothermic reaction looks like this:

And an endothermic reaction would look like this:

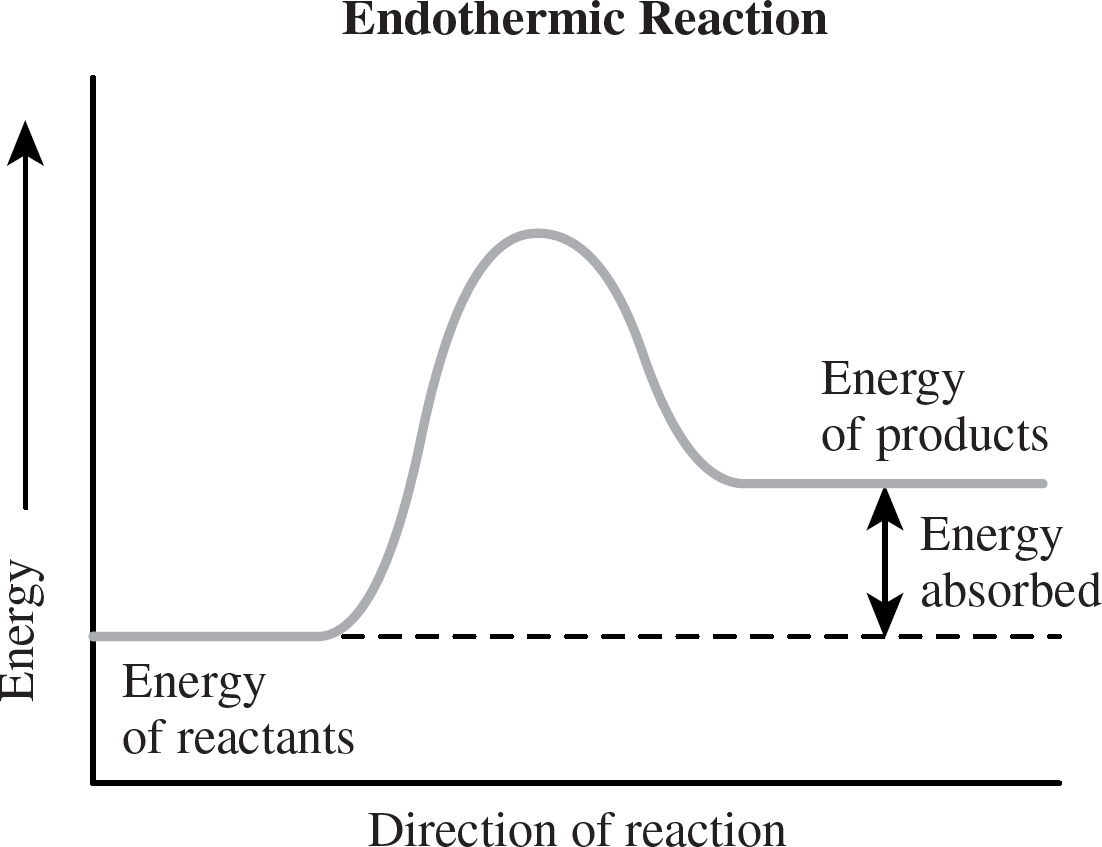
Note that all that matters here is the energy difference between the reactants and the products. The “hump” in between them is related to the reaction’s speed, something that we’ll take a look at in a later chapter.

Heat of Formation

Another term you should be familiar with for the test is heat of formation—that is, the amount of heat that’s released or absorbed when 1 mole of a compound is formed from its elements. When we talk about heat of formation, we use the same symbol we use for enthalpy change, but we put a subscript “f” on it: ∆H_f. Let’s consider the heat of formation of gaseous carbon dioxide, CO₂.

C(s) + O₂(g) → CO₂(g); ∆H_f = –393 kJ/mol

The fact that the heat of formation is negative means that heat is released during this reaction; this is an exothermic reaction. When 1 mole of CO₂ (g) is formed from its elements (C(s) and O₂(g)), 393 kJ of energy are released.

C, Ni, Cl₂, O₂, H₂, and N₂—or all other elemental atoms or molecules—have a heat of formation of zero. Remember this for the exam.

For all elements, the heat of formation is zero.

For this test, you’ll also need to keep in mind that for any reaction, the heats of formation of all the products minus the heats of formation of all the reactants is equal to ∆H_f for the whole reaction. The test writers might show you a reaction and give you heats of formation for all of the reactants and products. Then they’ll ask you to figure out ∆H_f for the whole reaction.

That’s simple to do; you just add up the heats of formation for all of the products and then all of the reactants, multiplying each by its coefficient from the balanced equation, and you’ve got ∆H_f for the reaction. Remember that ∆H_f (reaction) = ∆H_f (products) – ∆H_f (reactants) and that the heats of formation of all elements are zero. Look at this reaction:

C₆H₁₂O₆(s) + 6O₂(g) → 6CO₂(g) + 6H₂O(l)

Suppose you’re told that the heat of formation for

• C₆H₁₂O₆(s) is –1,273 kJ/mol

• H₂O(l) is –286 kJ/mol

• CO₂(g) is –393 kJ/mol

(∆H_f for O₂(g), of course, is 0.)

∆H_f for the whole reaction is equal to

∆H_f(products) – ∆H_f(reactants)

∆H_f(products) = 6 mol(–393 kJ/mol) + 6 mol(–286 kJ/mol) = – 4,074 kJ

∆H_f(reactants) = –1,273 kJ + 0 kJ = –1,273 kJ

So, ∆H for the whole reaction = (– 4,074) – (–1,273) = –2,801 kJ. ∆H for the whole reaction is negative, which means the reaction is exothermic.

We can also combine stoichiometry with thermochemistry in order to figure out how much heat is given off or released when a certain amount of reactants are used up. Let’s use the reaction we just looked at to show you how.

If 18.0 g of C₆H₁₂O₆ were to be reacted, how much energy would be released?

Note that the –2,801 kJ goes with 1 mole of C₆H₁₂O₆ because that is the coefficient on the C₆H₁₂O₆ in the balanced reaction. If you were to instead start with 32.0 g of oxygen, the math would look like this:

Remember, without a calculator, any math you would be asked to do should be fairly straightforward, as it is above.

Hess’s Law

If you have to manipulate any reaction, then there are rules in place for how the enthalpy of that reaction changes as well. There’s three rules you should know:

1. If you multiply (or divide) all of the species in that reaction by a coefficient, the enthalpy change must be multiplied (or divided) by the same coefficient.

2. If you flip a reaction, you must also flip the sign on the enthalpy.

3. If a reaction is the sum of several component reactions, the enthalpy for that reaction will be the sum of the enthalpies of the various components.

These rules combined are often called Hess’s Law. What Hess’s Law essentially tells us is that ΔH for a reaction is independent of the number of steps or the pathway the reaction follows.

Take a look at the following example.

Given:

C(s) + O₂(g) → CO₂(g)	ΔH = x
H₂O(l) → H₂(g) + ½ O₂(g)	ΔH = y
C₃H₈(g) → 3C(s) + 4 H₂(g)	ΔH = z

Find the enthalpy change for C₃H₈(g) + 5 O₂(g)→ 3 CO₂(g) + 4 H₂O(l) in terms of the defined variables.

To solve this, we need to manipulate the given reactions so that when they are added together, we get our target reaction. The first step in doing this is to flip the middle reaction—the H₂O (l) has to be on the right side when we finish. While we’re at it, we’ll also multiply it by four so the coefficient on the H₂O (l) is correct. That gives us:

4H₂(g) + 2O₂(g) → 4 H₂O(l)

ΔH = y × –4 = –4y

We also need to multiple the top reaction by 3, so the coefficient on the CO₂(g) is correct.

3C(s) + 3O₂(g) → 3CO₂(g)

ΔH = x × 3 = 3x

If we add all three equations together:

4H₂(g) + 2O₂(g) + 3C(s) + 3O₂(g) + C₃H₈(g) → 4H₂O (l) + 3CO₂(g) + 3C(s) + 4H₂(g)

Anything that appears on both sides of the arrow cancels out and any identical substances on the same side of the arrow combine, leaving us with our target equation:

To determine the enthalpy for that equation, all we have to do is add the enthalpies for all three reactions. So, the enthalpy in terms of the given variables would be 3x – 4y + z.

Spontaneity and Gibbs Free Energy

A spontaneous reaction is one that will occur at a given temperature without the input of energy. Strangely enough, however, sometimes endothermic reactions (which require the input of energy in order to take place) occur spontaneously. Why? Because, as we said, the universe likes entropy—disorder. If a particular reaction is endothermic (∆H is positive) but creates greater disorder (∆S is also positive), and the disorder the reaction creates exceeds the energy it requires, then the reaction may occur spontaneously although it’s endothermic. Similarly, if a reaction creates order instead of disorder, it may occur spontaneously as long as it’s exothermic and the negative enthalpy change exceeds the negative entropy change.

What determines whether a reaction will or won’t occur spontaneously? The combination of ∆H and ∆S. This combination of ∆H and ∆S is called Gibbs free energy, and is symbolized by ∆G. The actual formula for determining ∆G is ∆G = ∆H – T∆S (where T is temperature, measured in degrees Kelvin). Remember the following points about Gibbs free energy:

• If ∆G for the reaction is negative, then that reaction occurs spontaneously in the forward direction.

• If ∆G for the reaction is positive, then that reaction occurs spontaneously in the reverse direction.

• If ∆G for the reaction is zero, then the reaction is in equilibrium. (We’ll discuss equilibrium later.)

Important Facts About Gibbs Free Energy

ΔG = ΔH - TΔS

If ΔG < 0, then the reaction is spontaneous in the forward direction.

If ΔG > 0, then the reaction is spontaneous in the reverse direction.

Review everything we’ve talked about in this chapter, and then answer the following set of questions. The answers can be found in Part IV.

DRILL 2

Question Type A

Questions 5-7 refer to the following.

(A) Gibbs free energy

(B) Heat of formation

(D) Entropy

(E) Kinetic energy

5. Value that determines whether a reaction is spontaneous

6. Quantity that determines whether a reaction is exothermic or endothermic

7. Indicates the degree of disorder of a system

Question Type B

I		II
103. If a reaction is exothermic, it always proceeds spontaneously	BECAUSE	the universe favors a negative enthalpy change.

104. Ice melting is an endothermic process	BECAUSE	heat must be absorbed by ice if it is to melt.

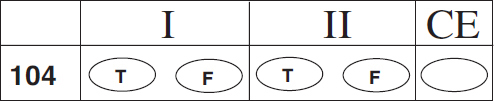
Question Type C

26. …C₂H₄(g) +…O₂(g) →…CO₂(g) +…H₂O(l)

If the equation for the reaction above is balanced using the smallest possible whole-number coefficients, then the coefficient for oxygen gas is

(A) 1

(B) 2

(D) 4

(E) 5

27. 2Na(s) + Cl₂(g) → 2NaCl(s) + 822 kJ

How much heat is released by the above reaction if 0.5 mole of sodium reacts completely with chlorine?

(A) 205 kJ

(B) 411 kJ

(D) 1,644 kJ

(E) 3,288 kJ

28. 2Al(s) + Fe₂O₃(s) → Al₂O₃(s) + 2Fe(s)

If 80 grams of Al and 80 grams of Fe₂O₃ are combined, what is the maximum number of moles of Fe that can be produced?

(A) 0.5

(B) 1

(D) 3

(E) 4

Summary

○ A molecule is a unit consisting of two or more atoms.

○ The elements that exist as diatomic molecules are: O₂, I₂, H₂, N₂, Cl₂, F₂, Br₂.

○ The formula weight of a molecule is the sum of the weights of the atoms making up that molecule.

○ The empirical formula is the smallest whole number ratio of the numbers of atoms of different elements within a molecule.

○ The percent composition of an element in a molecule is the mass of all the atoms of that element within the molecule, divided by the formula weight, times 100.

○ A mole is 6.02 × 10²³ molecules. 1 mole of atomic mass units is 1 gram (1/12 the mass of a carbon-12 atom), so the atomic weight of a molecule equals the mass of 1 mole of that molecule in grams.

○ To convert mass composition to empirical formula, first calculate the number of moles of each element in a 100-gram sample, dividing the percent composition of each element by that element’s atomic weight. The whole number ratio of moles of each element gives the empirical formula.

○ To balance chemical equations on the SAT Subject Test in Chemistry, plug in each answer choice as the coefficient of the molecule being asked about. If the equation can be balanced so that the numbers of each type of atom are the same on the right and left, and that the resulting coefficients don’t have any common factor, then you’ve found the right answer.

○ To solve limiting reactant problems, use stoichiometry to determine which reactant would create less of a given produce. The reactant which creates less product is limiting, and the other reactant is excess.

○ If we manipulate a chemical reaction by flipped it or multiplying it by a coefficient, the enthalpy of that reaction will also change in the manner outlined using Hess’s Law.

○ Entropy, S, is a measure of the randomness of a system. The higher the entropy and lower the energy, the more stable the system.

○ The energy given off or absorbed by a reaction is the enthalpy change, ΔH.

• Enthalpy change is the difference in potential energy between the bonds in the reactants and the bonds in the products.

• A reaction with a negative enthalpy is exothermic, or produces heat, while a reaction with a positive enthalpy is endothermic, or absorbs heat.

• Heat of formation is the energy released or absorbed when a molecule is created from its constituent elements.

• The enthalpy change for a reaction is the heat of formation of the products minus the heat of formation of the reactants.

○ Gibbs free energy is given by

ΔG = ΔH – TΔS

If the Gibbs free energy is positive, the reaction is non-spontaneous; if the Gibbs free energy is negative, the reaction is spontaneous.