27.The Art of Reductionism
Scientific reductionism is the idea of reducing complex interactions and entities to the sum of their constituent parts, in order to make them easier to study (explorer.com). It is based on the idea that science can be used to explain everything by a mere look at the individual constituent processes.
There are three types of reductionism, that is, ontological, methodological and theory reduction. In this section we shall emphasize theory reduction because we have a great deal of reducing known laws of physics from a somewhat simple rule. This was the case when Kepler’s laws of the motion of planets and Galileo’s theories of motion were reduced to the Newtonian theories of mechanics.
Newtonian Mechanics became a more general theory simply because all the explanatory power of Kepler’s and Galileo’s laws was contained in it. Therefore theoretical reduction is considered as the reduction of one explanation or theory to another.
The most interesting thing about this section is that, during the process of reduction we create a relationship between the known law to another law explaining the same thing but unknown to the entire physics community. For example in the reduction of the Weidman Franz- Lorenz law we create in a process a law for the thermal conductivity of gravito-electric phenomenon.
Therefore reductionism is deriving something complicated from something simple. For example in the derivation of the Weidman Franz law we set a formula that states that, the electric force

on an electron is proportional to the gravitational force at the schwarzichild’s radius

but inversely proportional to the gravitational coupling constant

as given below,
Where n, is the principle quantum number.
On squaring the above equation we obtain the square of the electric field as,
From the formular for the temperature of the black hole, the function
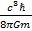
is related to temperature as kT, and then the law for thermal conductivity will be reduced as,
The left hand side represents the ratio of the thermal conductivity K to the electric conductivity δ, which is the Weidman Franz law. From the above reduction we have generated an important rule given by,
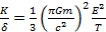
=
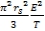
The above formula explains the thermal properties of black holes at the schwarzichild’s radius
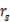
.
 on an electron is proportional to the gravitational force at the schwarzichild’s radius
on an electron is proportional to the gravitational force at the schwarzichild’s radius  but inversely proportional to the gravitational coupling constant
but inversely proportional to the gravitational coupling constant  as given below,
as given below, on an electron is proportional to the gravitational force at the schwarzichild’s radius
on an electron is proportional to the gravitational force at the schwarzichild’s radius  but inversely proportional to the gravitational coupling constant
but inversely proportional to the gravitational coupling constant  as given below,
as given below,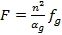
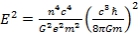
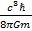 is related to temperature as kT, and then the law for thermal conductivity will be reduced as,
is related to temperature as kT, and then the law for thermal conductivity will be reduced as,
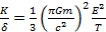 =
=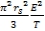
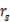 .
.