-
-
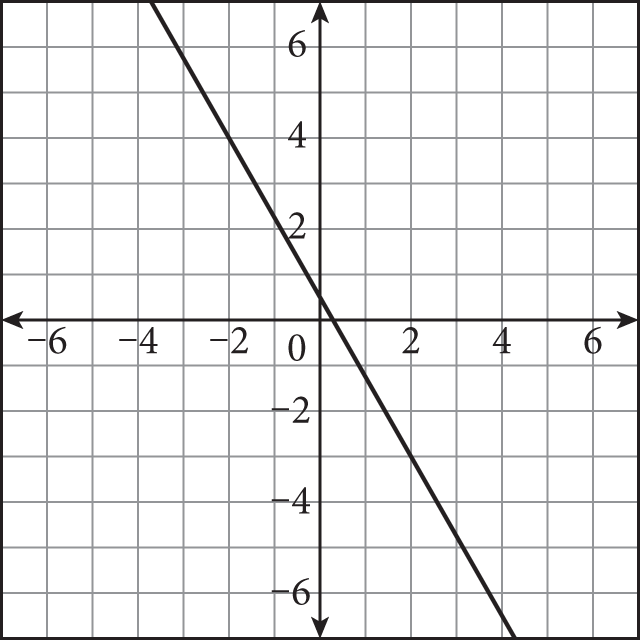
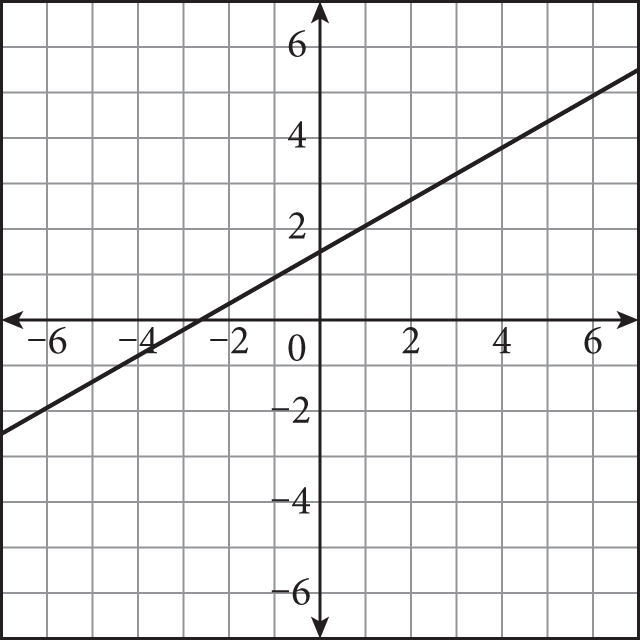
The line
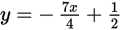 is shown in the graph. If the line is shifted down 2 units and then reflected over
the x-axis, which of the following graphs represents the new line?
is shown in the graph. If the line is shifted down 2 units and then reflected over
the x-axis, which of the following graphs represents the new line?
Now you’ll have a chance to try a few more test-like questions. Use the scaffolding as needed to guide you through the question and get the right answer.
Some guidance is provided, but you’ll need to fill in the missing parts of explanations or the step-by-step math to get to the correct answer. Don’t worry—after going through the examples at the beginning of this chapter, these questions should be completely doable. If you find yourself struggling, however, review the worked examples again.

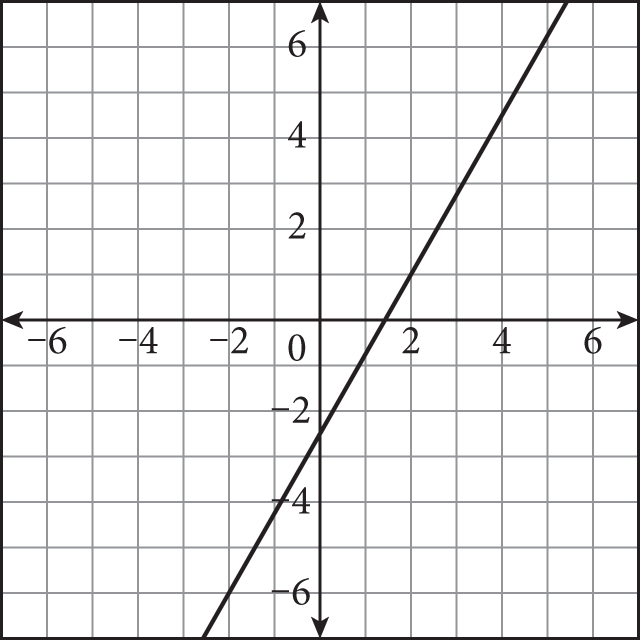
The line
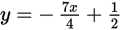 is shown in the graph. If the line is shifted down 2 units and then reflected over
the x-axis, which of the following graphs represents the new line?
is shown in the graph. If the line is shifted down 2 units and then reflected over
the x-axis, which of the following graphs represents the new line?

The following table can help you structure your thinking as you go about solving this problem. Kaplan's strategic thinking is provided, as are bits of structured scratchwork. If you’re not sure how to approach a question like this, start at the top and work your way down.
| Strategic Thinking | Math Scratchwork |
|---|---|
| Step 1: Read the question, identifying and organizing important information as you go | |
You’re asked for the graph that corresponds to the described changes made to
 . .
|
|
| Step 2: Choose the best strategy to answer the question | |
|
While you could apply the transformations to the entire line, picking a test point will be faster. For example, draw a dot at (–2, 4) on the original line and apply the changes to that point. Cross off any choices that don’t pass through the new point. You might need to pick more than one point if your initial choice doesn’t eliminate all of the incorrect answer choices. |
 |
| Step 3: Check that you answered the right question | |
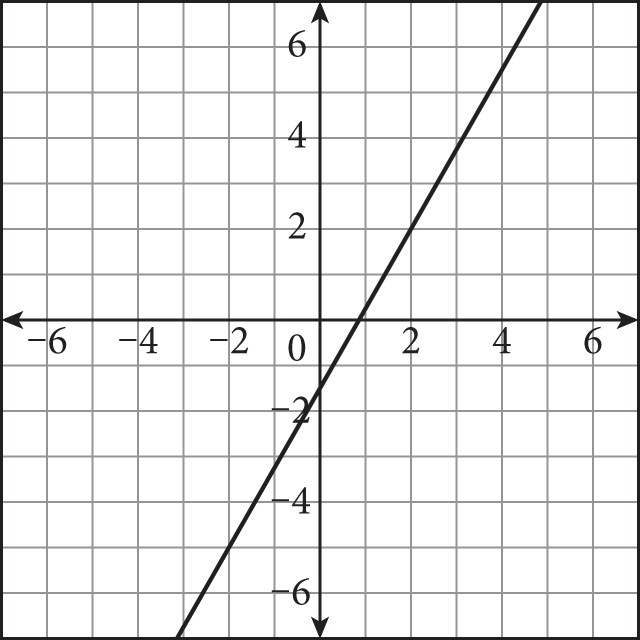
| Did you get (D)? If so, you’re correct! Beware of C—it results from a reflection over the wrong axis. |
Here’s another test-like example to try.
Three years ago, Madison High School started charging an admission fee for basketball games to raise money for new bleachers. The initial price was $2 per person; the school raised the price of admission to $2.50 this year. Assuming this trend continues, which of the following equations can be used to describe the cost of admission, c, y years after the school began charging for admission to games?
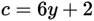
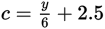
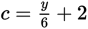

The following table can help you structure your thinking as you go about solving this problem. Kaplan's strategic thinking is provided, as are bits of structured scratchwork. If you’re not sure how to approach a question like this, start at the top and work your way down.
| Strategic Thinking | Math Scratchwork |
|---|---|
| Step 1: Read the question, identifying and organizing important information as you go | |
| You need to identify the equation that correctly relates cost to years after the admission charge implementation. | |
| Step 2: Choose the best strategy to answer the question | |
| Look carefully; you’re implicitly given two sets of coordinates. You can use these to find a key piece of a linear equation and eliminate two answer choices. |
(___, ___), (___, ___) m = = eliminate ___ and ___ |
| The school started charging admission at a certain point in time; the price at this point is the y-intercept. Use this to pick the correct answer. |
b = ___ |
| Step 3: Check that you answered the right question | |
| Did you come up with (C)? If so, great job! You’re correct. | ___ |