III.79 Riemann Surfaces
Alan F. Beardon
Let D be a region (that is, a connected open set) in the complex plane. If f is a complex-valued function defined on D, then we can define its derivative just as we would for real-valued functions defined on subsets of 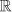 : the derivative of f at w is the limit as z tends to w of the “difference quotient” (f(z) - f(w)) / (z - w). Of course, this limit does not necessarily exist, but if it exists for every w in D, then f is said to be analytic, or holomorphic, on D. Analytic functions have amazing properties; for example, if a function is analytic in a region, then it automatically has a Taylor-series expansion at each point of the region, from which one can deduce that it is infinitely differentiable. This is in stark contrast to the theory of real functions of a real variable, where, for example, a function may be once differentiable but not twice differentiable at some point x, yet three-times differentiable at some other point y. Complex analysis is the study of analytic functions. Perhaps more than any other mathematical topic, it is both immensely useful in a practical sense and profound and beautiful in a theoretical sense. (Some of the basic results of complex analysis are described in [I.3 §5.6].)
: the derivative of f at w is the limit as z tends to w of the “difference quotient” (f(z) - f(w)) / (z - w). Of course, this limit does not necessarily exist, but if it exists for every w in D, then f is said to be analytic, or holomorphic, on D. Analytic functions have amazing properties; for example, if a function is analytic in a region, then it automatically has a Taylor-series expansion at each point of the region, from which one can deduce that it is infinitely differentiable. This is in stark contrast to the theory of real functions of a real variable, where, for example, a function may be once differentiable but not twice differentiable at some point x, yet three-times differentiable at some other point y. Complex analysis is the study of analytic functions. Perhaps more than any other mathematical topic, it is both immensely useful in a practical sense and profound and beautiful in a theoretical sense. (Some of the basic results of complex analysis are described in [I.3 §5.6].)
Just as group theorists do not generally distinguish between isomorphic groups, and topologists do not distinguish between homeomorphic topological spaces, complex analysts do not distinguish between two regions D and D′ if there is an analytic bijection between D and D′. When this is the case, we say that D and D′ are conformally equivalent. Conformal equivalence is, as its name suggests, an EQUIVALENCE RELATION [I.2 §2.3]: the proof depends on the surprising fact that if f is an analytic bijection from D to D′, then its inverse f-1 : D′ → D is also analytic. Again, this contrasts with real analysis. If D and D′ are conformally equivalent, then “interesting” properties of analytic functions on D are transferred automatically to corresponding properties of analytic functions defined on D′. Indeed, this statement can almost be taken as a definition of “interesting” properties (although admittedly this conflicts with the numerical side of complex analysis, because purely numerical statements do not usually transfer under such maps). Naturally, we would like to know which properties of analytic functions are “interesting” in this sense. One such property is that (except at certain isolated points) the angle between two intersecting curves in D is preserved under an analytic map: this is the origin of the term “conformal.” It is less well-known that if a bijection (which is not assumed to be differentiable) preserves the angles between curves (that is, both their magnitude and whether they are measured clockwise or counterclockwise), then it is analytic. Thus, loosely speaking, the preservation of angles implies the existence of a Taylor series!
The impact of complex analysis on other topics is so great that it is natural to try to find the most general type of surface on which we can study analytic functions. This leads to the definition of a Riemann surface (after BERNHARD RIEMANN [VI.49], who introduced the idea in his doctoral dissertation). In order to put a coordinate system on a surface S we try to map S bijectively onto a plane region D; if we succeed, then we can transfer the coordinates from D to S. For many surfaces (for example, a sphere) it is not possible to find such a map, and we have to be satisfied with local coordinates. This means that at each point w of S, we map a neighborhood N of w onto a plane region, and so obtain coordinates that are restricted to N. As there are usually infinitely many ways to do this, we are forced to consider the class of transition maps; that is, the maps from one coordinate system at w to another. The surface is a Riemann surface precisely when each such transition map is an analytic bijection. This definition resembles that of a two-dimensional MANIFOLD [I.3 §6.9], but the requirement that the transition maps should be analytic is much stronger, so by no means every 2-manifold is a Riemann surface.
It is not difficult to construct Riemann surfaces. Consider, for example, a sphere S resting on a horizontal table. If we imagine a light source at the highest point P of the sphere, then each point of S except P casts a “shadow” on the table: since the table has a simple coordinate system, we can use these “shadows” to define a coordinate system on all of S except the point P. Similarly, a light source at the point Q of tangency with the table casts a shadow onto the (horizontal) tangent plane at P, and this gives a coordinate system valid throughout S except at Q. It can be shown that if the second coordinate system is composed with a reflection, then the sphere does have the structure of a Riemann surface. This is an extremely important example, because it allows one to handle questions involving infinity in a satisfactory way; it is known as the Riemann sphere.
For another example, consider a cube C, and (for simplicity only) remove the eight vertices. Given a face F of C (without its bounding edges), we can find a Euclidean rigid motion that maps F into  , so we can easily define a coordinate system on F. If w is an interior point of an edge E of C, we can “open” the two faces that meet at E to make a planar region that contains E, and then map this region into
, so we can easily define a coordinate system on F. If w is an interior point of an edge E of C, we can “open” the two faces that meet at E to make a planar region that contains E, and then map this region into  by a Euclidean rigid motion. In this way we see that C (less its vertices) is a Riemann surface. The problem with the vertices can be solved by technical means, and this method can then be generalized to show that any polyhedron (even one with holes, such as a “square” torus) is a Riemann surface. These are known as compact surfaces. It is a deep but fascinating classical result that each such surface corresponds bijectively to an irreducible polynomial P(z, w) in two complex variables. To give an idea of how the correspondence works, let us consider an equation such as w3 + wz + z2 = 0. For each z this can be solved to give three values of w, say w1, w2, and w3; as we allow z to vary in
by a Euclidean rigid motion. In this way we see that C (less its vertices) is a Riemann surface. The problem with the vertices can be solved by technical means, and this method can then be generalized to show that any polyhedron (even one with holes, such as a “square” torus) is a Riemann surface. These are known as compact surfaces. It is a deep but fascinating classical result that each such surface corresponds bijectively to an irreducible polynomial P(z, w) in two complex variables. To give an idea of how the correspondence works, let us consider an equation such as w3 + wz + z2 = 0. For each z this can be solved to give three values of w, say w1, w2, and w3; as we allow z to vary in  , the values wj vary, and as they do so they create a Riemann surface W, which can be shown to be connected. This surface can be thought of as lying “above”
, the values wj vary, and as they do so they create a Riemann surface W, which can be shown to be connected. This surface can be thought of as lying “above”  , and for all but a finite set of z in
, and for all but a finite set of z in  there are exactly three points on W that are “above” z.
there are exactly three points on W that are “above” z.
As we have mentioned, Riemann surfaces are important because they are the most general surfaces on which one can study analytic functions, with all of their remarkable properties. It is easy to define what we mean by an analytic function f on a Riemann surface R. Given a coordinate system on part of R, we can think of f as a function of the coordinates, and we then regard f as analytic if and only if it depends analytically on the coordinates. Because the transition maps are analytic, f will be analytic with respect to one coordinate system if and only if it is analytic with respect to all the other coordinate systems defined at the point in question.
This simple property—that if something holds in one coordinate system, then it holds in all of them—is one of the crucial features of the theory. For example, suppose that we have two curves crossing on an (abstract) Riemann surface. If we transfer the two curves to plane regions using different local coordinate systems at the crossing point, and then measure the angle of intersection in each case, we must get the same result (since the transition from one coordinate system to another preserves angles). It follows that the angle between intersecting curves on an abstract Riemann surface is a well-defined concept.
It turns out that analysis on Riemann surfaces goes beyond analytic functions. Harmonic functions (solutions of LAPLACE’S EQUATION [I.3 §5.4]) are intimately connected to analytic functions, since the real part of an analytic function is harmonic and any harmonic function is (locally) the real part of an analytic function. Thus, on a Riemann surface, complex analysis merges almost imperceptibly with potential theory (which is the study of harmonic functions).
Perhaps the most profound theorem of all about Riemann surfaces is the UNIFORMIZATION THEOREM [V.34]. Roughly speaking, this says that every Riemann surface is obtained from either Euclidean, spherical, or hyperbolic geometry (see SOME FUNDAMENTAL MATHEMATICAL DEFINITIONS [I.3 §§6.2, 6.5, 6.6]) by taking a polygon in that geometry and gluing its sides together, in the same way that one obtains a torus by gluing opposite sides of a rectangle together. (See also FUCHSIAN GROUPS [III.28].) Remarkably, only very few Riemann surfaces come from the Euclidean or spherical geometries; essentially, every Riemann surface can be constructed in this way from (and only from) the hyperbolic plane. This means that virtually every region in the complex plane comes equipped with a natural and intrinsic geometry whose character is hyperbolic and not, as one might expect, Euclidean. The Euclidean character of a generic plane region comes from its embedding in  , and not from its own intrinsic hyperbolic geometry.
, and not from its own intrinsic hyperbolic geometry.
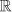 : the derivative of f at w is the limit as z tends to w of the “difference quotient” (f(z) - f(w)) / (z - w). Of course, this limit does not necessarily exist, but if it exists for every w in D, then f is said to be analytic, or holomorphic, on D. Analytic functions have amazing properties; for example, if a function is analytic in a region, then it automatically has a Taylor-series expansion at each point of the region, from which one can deduce that it is infinitely differentiable. This is in stark contrast to the theory of real functions of a real variable, where, for example, a function may be once differentiable but not twice differentiable at some point x, yet three-times differentiable at some other point y. Complex analysis is the study of analytic functions. Perhaps more than any other mathematical topic, it is both immensely useful in a practical sense and profound and beautiful in a theoretical sense. (Some of the basic results of complex analysis are described in [I.3 §5.6].)
: the derivative of f at w is the limit as z tends to w of the “difference quotient” (f(z) - f(w)) / (z - w). Of course, this limit does not necessarily exist, but if it exists for every w in D, then f is said to be analytic, or holomorphic, on D. Analytic functions have amazing properties; for example, if a function is analytic in a region, then it automatically has a Taylor-series expansion at each point of the region, from which one can deduce that it is infinitely differentiable. This is in stark contrast to the theory of real functions of a real variable, where, for example, a function may be once differentiable but not twice differentiable at some point x, yet three-times differentiable at some other point y. Complex analysis is the study of analytic functions. Perhaps more than any other mathematical topic, it is both immensely useful in a practical sense and profound and beautiful in a theoretical sense. (Some of the basic results of complex analysis are described in [I.3 §5.6].) , so we can easily define a coordinate system on F. If w is an interior point of an edge E of C, we can “open” the two faces that meet at E to make a planar region that contains E, and then map this region into
, so we can easily define a coordinate system on F. If w is an interior point of an edge E of C, we can “open” the two faces that meet at E to make a planar region that contains E, and then map this region into