b. Columbia, Missouri, 1894; d. Stockholm, 1964
Stochastic processes; applications to electrical engineering and physiology; harmonic analysis; cybernetics
Wiener was just eighteen years old when, in 1913, he was awarded a Ph.D. in logic while studying under Josiah Royce at Harvard University. Afterward, he studied with, among others, RUSSELL [VI.71] and HARDY [VI.73] in Cambridge and HILBERT [VI.63] in Göttingen. After doing work on ballistics for the military during World War II, he was appointed instructor of mathematics at the fledgling Massachusetts Institute of Technology in Cambridge, MA, where he remained for the rest of his career.
Wiener was in many respects a nonconformist, certainly scientifically and mathematically, but also socially, culturally, politically, and philosophically. He was a precocious child and his home education by his father (a noted linguist and Harvard professor), along with his Jewish background in a society still stricken by anti-Semitism, made his nonconformism almost inevitable. Garrett Birkhoff, the son of GEORGE BIRKHOFF [VI.78], said the following in 1977:
Wiener was notable as one of the few Americans of his time who was outstanding in both pure mathematics and its applications. How much of this can be attributed to his varied and cosmopolitan early background, and how much to his continuing contacts with non-mathematicians… it is hard to say.
During a period in which American mathematics was largely self-sufficient and was still in a phase in which interdisciplinary approaches were generally ignored, Wiener was reaching out to European mathematics and collaborating with engineers such as Vannevar Bush.
This attitude also affected his choices of research topics, even within pure mathematics: he worked on whatever took his fancy. In a talk in 1938, George Birkhoff described Wiener’s work on Tauberian theorems as an example of “exercising talent for free invention,” contrasting this with the typically American approach: “mathematics as serious business.”
Wiener’s way of connecting pure and applied mathematics did not follow the usual path of taking old problems of applied mathematics (such as in classical mechanics and electrical engineering) and tackling them with new, and rigorously sharpened, mathematical tools. Rather the opposite: Wiener used some of the newest, and much debated, results of pure mathematics—such as the LEBESGUE INTEGRAL [III.55], Fourier transformations in the complex domain, and STOCHASTIC PROCESSES [IV.24]—and connected them to several of the newest physical, technological, and biological problems. The types of problem he attacked included those of BROWNIAN MOTION [IV.24], quantum mechanics, radio astronomy, anti-aircraft fire control, noise filtration in radar, the nervous system, and the theory of automata.
Of Wiener’s many analytical results that make connections between very different domains we give only one as an example. Around 1931 Wiener discussed the following (Lebesgue) integral equation with the German mathematical astrophysicist Eberhard Hopf:
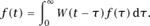
The solution for unknown f (t), which was found with the help of a new and very important factorization technique, and which was dependent on the analytical behavior of the FOURIER TRANSFORMS [III.27] Of the functions involved, could be connected to radiative equilibrium in stars. When t is interpreted as time, equations of this kind can be seen to describe causality: the transition from the influencing “past” to the indeterminate “future.” A decade later the Wiener–Hopf equations would be connected to Wiener’s theories of prediction and filtering.
Wiener’s discussion of such disparate fields of application could not fail to invoke philosophically relevant notions such as causality, information (Wiener is considered together with Claude Shannon as the founder of the modern concept of information), control, feedback, and finally the wide-ranging theory of “cybernetics.” Cybernetics (literally, the art of steering) can be retrospectively connected to earlier discussions in Greek antiquity (Plato), to James Watt’s centrifugal governor, and to Ampère’s philosophical writings. Wiener’s broad outlook resulted from his collaboration with colleagues from very different domains: mathematical (R. E. A. C. Paley), physical (Hopf), technical (Julian Bigelow, Bush), and physiological (Arturo Rosenblueth). However, this outlook left him vulnerable to criticism and to philosophical and political misinterpretation. The prominent mathematician Hans Freudenthal was a sharp-tongued critic of Wiener’s epoch-making book of 1948, Cybernetics or the Control and Communication in the Animal and the Machine, claiming that it “shows there is not much to be reported” and that it has contributed to spreading mistaken ideas of what mathematics really means,” although even he had to admit that the book “earned Wiener the greater part of his public renown” and that its “mathematical readers were more fascinated by the richness of its ideas than by its shortcomings.”
During the period of the Nazi threat Wiener helped refugees from Europe to settle in the United States, while after World War II he cautioned against the repetition of mistakes such as the boycott of German science in the aftermath of World War I. Wiener warned against the arms race and the misuse of technological developments in the postwar world. Having resigned from the National Academy of Sciences in 1941 because of its alleged bureaucracy and complacency, Wiener nevertheless accepted, shortly before his death in 1964 while traveling, the National Medal of Science from President Johnson.
Masani, P. R. 1990. Norbert Wiener 1894–1964. Basel: Birkhäuser.
Reinhard Siegmund-Schultze