VIII.6 Advice to a Young Mathematician
The most important thing that a young mathematician needs to learn is of course mathematics. However, it can also be very valuable to learn from the experiences of other mathematicians. The five contributors to this article were asked to draw on their experiences of mathematical life and research, and to offer advice that they might have liked to receive when they were just setting out on their careers. (The title of this entry is a nod to Sir Peter Medawar’s well-known book, Advice to a Young Scientist.) The resulting contributions were every bit as interesting as we had expected; what was more surprising was that there was remarkably little overlap between the contributions. So here they are, five gems intended for young mathematicians but surely destined to be read and enjoyed by mathematicians of all ages.
I. Sir Michael Atiyah
Warning
What follows is very much a personal view based on my own experience and reflecting my personality, the type of mathematics that I work on, and my style of work. However, mathematicians vary widely in all these characteristics and you should follow your own instinct. You may learn from others but interpret what you learn in your own way. Originality comes by breaking away, in some respects, from the practice of the past.
Motivation
A research mathematician, like a creative artist, has to be passionately interested in the subject and fully dedicated to it. Without strong internal motivation you cannot succeed, but if you enjoy mathematics the satisfaction you can get from solving hard problems is immense.
The first year or two of research is the most difficult. There is so much to learn. One struggles unsuccessfully with small problems and one has serious doubts about one’s ability to prove anything interesting. I went through such a period in my second year of research, and Jean-Pierre Serre, perhaps the outstanding mathematician of my generation, told me that he too had contemplated giving up at one stage.
Only the mediocre are supremely confident of their ability. The better you are, the higher the standards you set yourself—you can see beyond your immediate reach.
Many would-be mathematicians also have talents and interests in other directions and they may have a difficult choice to make between embarking on a mathematical career and pursuing something else. The great Gauss is reputed to have wavered between mathematics and philology, Pascal deserted mathematics at an early age for theology, while Descartes and Leibniz are also famous as philosophers. Some mathematicians move into physics (e.g., Freeman Dyson) while others (e.g., Harish Chandra, Raoul Bott) have moved the other way. You should not regard mathematics as a closed world, and the interaction between mathematics and other disciplines is healthy both for the individual and for society.
Psychology
Because of the intense mental concentration required in mathematics, psychological pressures can be considerable, even when things are going well. Depending on your personality this may be a major or only a minor problem, but one can take steps to reduce the tension. Interaction with fellow students—attending lectures, seminars, and conferences—both widens one’s horizons and provides important social support. Too much isolation and introspection can be dangerous, and time spent in apparently idle conversation is not really wasted.
Collaboration, initially with fellow students or one’s supervisor, has many benefits, and long-term collaboration with coworkers can be extremely fruitful both in mathematical terms and at the personal level. There is always the need for hard quiet thought on one’s own, but this can be enhanced and balanced by discussion and exchange of ideas with friends.
Problems versus Theory
Mathematicians are sometimes categorized as either “problem solvers” or “theorists.” It is certainly true that there are extreme cases that highlight this division (Erd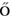 s versus Grothendieck, for example) but most mathematicians lie somewhere in between, with their work involving both the solution of problems and the development of some theory. In fact, a theory that does not lead to the solution of concrete and interesting problems is not worth having. Conversely, any really deep problem tends to stimulate the development of theory for its solution (Fermat’s last theorem being a classic example).
s versus Grothendieck, for example) but most mathematicians lie somewhere in between, with their work involving both the solution of problems and the development of some theory. In fact, a theory that does not lead to the solution of concrete and interesting problems is not worth having. Conversely, any really deep problem tends to stimulate the development of theory for its solution (Fermat’s last theorem being a classic example).
What bearing does this have on a beginning student? Although one has to read books and papers and absorb general concepts and techniques (theory), realistically, a student has to focus on one or more specific problems. This provides something to chew on and to test one’s mettle. A definite problem, which one struggles with and understands in detail, is also an invaluable benchmark against which to measure the utility and strength of available theories.
Depending on how the research goes, the eventual Ph.D. thesis may strip away most of the theory and focus only on the essential problem, or else it may describe a wider scenario into which the problem naturally fits.
The Role of Curiosity
The driving force in research is curiosity. When is a particular result true? Is that the best proof, or is there a more natural or elegant one? What is the most general context in which the result holds?
If you keep asking yourself such questions when reading a paper or listening to a lecture, then sooner or later a glimmer of an answer will emerge—some possible route to investigate. When this happens to me I always take time out to pursue the idea to see where it leads or whether it will stand up to scrutiny. Nine times out of ten it turns out to be a blind alley, but occasionally one strikes gold. The difficulty is in knowing when an idea that is initially promising is in fact going nowhere. At this stage one has to cut one’s losses and return to the main road. Often the decision is not clear-cut, and in fact I frequently return to a previously discarded idea and give it another try.
Ironically, good ideas can emerge unexpectedly from a bad lecture or seminar. I often find myself listening to a lecture where the result is beautiful and the proof ugly and complicated. Instead of trying to follow a messy proof on the blackboard, I spend the rest of the hour thinking about producing a more elegant proof. Usually, but not always, without success, but even then my time is better spent, since I have thought hard about the problem in my own way. This is much better than passively following another person’s reasoning.
Examples
If you are, like me, someone who prefers large vistas and powerful theories (I was influenced but not converted by Grothendieck), then it is essential to be able to test general results by applying them to simple examples. Over the years I have built up a large array of such examples, drawn from a variety of fields. These are examples where one can do concrete calculations, sometimes with elaborate formulas, that help to make the general theory understandable. They keep your feet on the ground. Interestingly enough, Grothendieck eschewed examples, but fortunately he was in close touch with Serre, who was able to rectify this omission. There is no clear-cut distinction between example and theory. Many of my favorite examples come from my early training in classical projective geometry: the twisted cubic, the quadric surface, or the Klein representation of lines in 3-space. Nothing could be more concrete or classical and all can be looked at algebraically or geometrically, but each illustrates and is the first case in a large class of examples which then become a theory: the theory of rational curves, of homogeneous spaces, or of Grassmannians.
Another aspect of examples is that they can lead off in different directions. One example can be generalized in several different ways or illustrate several different principles. For instance, the classical conic is a rational curve, a quadric, and a Grassmannian all in one.
But most of all a good example is a thing of beauty. It shines and convinces. It gives insight and understanding. It provides the bedrock of belief.
Proof
We are all taught that “proof” is the central feature of mathematics, and Euclidean geometry with its careful array of axioms and propositions has provided the essential framework for modern thought since the Renaissance. Mathematicians pride themselves on absolute certainty, in comparison with the tentative steps of natural scientists, let alone the woolly thinking of other areas.
It is true that, since Gödel, absolute certainty has been undermined, and the more mundane assault of computer proofs of interminable length has induced some humility. Despite all this, proof retains its cardinal role in mathematics, and a serious gap in your argument will lead to your paper being rejected.
However, it is a mistake to identify research in mathematics with the process of producing proofs. In fact, one could say that all the really creative aspects of mathematical research precede the proof stage. To take the metaphor of the “stage” further, you have to start with the idea, develop the plot, write the dialogue, and provide the theatrical instructions. The actual production can be viewed as the “proof”: the implementation of an idea.
In mathematics, ideas and concepts come first, then come questions and problems. At this stage the search for solutions begins, one looks for a method or strategy. Once you have convinced yourself that the problem has been well-posed, and that you have the right tools for the job, you then begin to think hard about the technicalities of the proof.
Before long you may realize, perhaps by finding counterexamples, that the problem was incorrectly formulated. Sometimes there is a gap between the initial intuitive idea and its formalization. You left out some hidden assumption, you overlooked some technical detail, you tried to be too general. You then have to go back and refine your formalization of the problem. It would be an unfair exaggeration to say that mathematicians rig their questions so that they can answer them, but there is undoubtedly a grain of truth in the statement. The art in good mathematics, and mathematics is an art, is to identify and tackle problems that are both interesting and solvable.
Proof is the end product of a long interaction between creative imagination and critical reasoning. Without proof the program remains incomplete, but without the imaginative input it never gets started. One can see here an analogy with the work of the creative artist in other fields: writer, painter, composer, or architect. The vision comes first, it develops into an idea that gets tentatively sketched out, and finally comes the long technical process of erecting the work of art. But the technique and the vision have to remain in touch, each modifying the other according to its own rules.
Strategy
In the previous section I discussed the philosophy of proof and its role in the whole creative process. Now let me turn to the most down-to-earth question of interest to the young practitioner. What strategy should one adopt? How do you actually go about finding a proof?
This question makes little sense in the abstract. As I explained in the previous section a good problem always has antecedents: it arises from some background, it has roots. You have to understand these roots in order to make progress. That is why it is always better to find your own problem, asking your own questions, rather than getting it on a plate from your supervisor. If you know where a problem comes from, why the question has been asked, then you are halfway toward its solution. In fact, asking the right question is often as difficult as solving it. Finding the right context is an essential first step.
So, in brief, you need to have a good knowledge of the history of the problem. You should know what sort of methods have worked with similar problems and what their limitations are.
It is a good idea to start thinking hard about a problem as soon as you have fully absorbed it. To get to grips with it, there is no substitute for a hands-on approach. You should investigate special cases and try to identify where the essential difficulty lies. The more you know about the background and previous methods, the more techniques and tricks you can try. On the other hand, ignorance is sometimes bliss. J. E. Littlewood is reported to have set each of his research students to work on a disguised version of the Riemann hypothesis, letting them know what he had done only after six months. He argued that the student would not have the confidence to attack such a famous problem directly, but might make progress if not told of the fame of his opponent! The policy may not have led to a proof of the Riemann hypothesis, but it certainly led to resilient and battle-hardened students.
My own approach has been to try to avoid the direct onslaught and look for indirect approaches. This involves connecting your problem with ideas and techniques from different fields that may shed unexpected light on it. If this strategy succeeds, it can lead to a beautiful and simple proof, which also “explains” why something is true. In fact, I believe the search for an explanation, for understanding, is what we should really be aiming for. Proof is simply part of that process, and sometimes its consequence.
As part of the search for new methods it is a good idea to broaden your horizons. Talking to people will extend your general education and will sometimes introduce you to new ideas and techniques. Very occasionally you may get a productive idea for your own research or even for a new direction.
If you need to learn a new subject, consult the literature but, even better, find a friendly expert and get instruction “from the horse’s mouth”—it gives more insight more quickly.
As well as looking forward, and being alert to new developments, you should not forget the past. Many powerful mathematical results from earlier eras have got buried and have been forgotten, coming to light only when they have been independently rediscovered. These results are not easy to find, partly because terminology and style change, but they can be gold mines. As usual with gold mines, you have to be lucky to strike one, and the rewards go to the pioneers.
Independence
At the start of your research your relationship with your supervisor can be crucial, so choose carefully, bearing in mind subject matter, personality, and track record. Few supervisors score highly on all three. Moreover, if things do not work out well during the first year or so, or if your interests diverge significantly, then do not hesitate to change supervisors or even universities. Your supervisor will not be offended and may even be relieved!
Sometimes you may be part of a large group and may interact with other members of the faculty, so that you effectively have more than one supervisor. This can be helpful in that it provides different inputs and alternative modes of work. You may also learn much from fellow students in such large groups, which is why choosing a department with a large graduate school is a good idea.
Once you have successfully earned your Ph.D. you enter a new stage. Although you may still carry on collaborating with your supervisor and remain part of the same research group, it is healthy for your future development to move elsewhere for a year or more. This opens you up to new influences and opportunities. This is the time when you have the chance to carve out a niche for yourself in the mathematical world. In general, it is not a good idea to continue too closely in the line of your Ph.D. thesis for too long. You have to show your independence by branching out. It need not be a radical change of direction but there should be some clear novelty and not simply a routine continuation of your thesis.
Style
In writing up your thesis your supervisor will normally assist you in the manner of presentation and organization. But acquiring a personal style is an important part of your mathematical development. Although the needs may vary, depending on the kind of mathematics, many aspects are common to all subjects. Here are a number of hints on how to write a good paper.
(i) |
Think through the whole logical structure of the paper before you start to write. |
(ii) |
Break up long complex proofs into short intermediate steps (lemmas, propositions, etc.) that will help the reader. |
(iii) |
Write clear coherent English (or the language of your choice). Remember that mathematics is also a form of literature. |
(iv) |
Be as succinct as it is possible to be while remaining clear and easy to understand. This is a difficult balance to achieve. |
(v) |
Identify papers that you have enjoyed reading and imitate their style. |
(vi) |
When you have finished writing the bulk of your paper go back and write an introduction that explains clearly the structure and main results as well as the general context. Avoid unnecessary jargon and aim at a general mathematical reader, not just a narrow expert. |
(vii) |
Try out your first draft on a colleague and take heed of any suggestions or criticisms. If even your close friend or collaborator has difficulty understanding it, then you have failed and need to try harder. |
(viii) |
If you are not in a desperate hurry to publish, put your paper aside for a few weeks and work on something else. Then return to your paper and read it with a fresh mind. It will read differently and you may see how to improve it. |
(ix) |
Do not hesitate to rewrite the paper, perhaps from a totally new angle, if you become convinced that this will make it clearer and easier to read. Well-written papers become “classics” and are widely read by future mathematicians. Badly written papers are ignored or, if they are sufficiently important, they get rewritten by others. |
II. Béla Bollobás
“There is no permanent place in this world for ugly mathematics,” wrote Hardy; I believe that it is just as true that there is no place in this world for unenthusiastic, dour mathematicians. Do mathematics only if you are passionate about it, only if you would do it even if you had to find the time for it after a full day’s work in another job. Like poetry and music, mathematics is not an occupation but a vocation.
Taste is above everything. It is a miracle of our subject that there seems to be a consensus as to what constitutes good mathematics. You should work in areas that are important and unlikely to dry up for a long time, and you should work on problems that are beautiful and important: in a good area there will be plenty of these, and not just a handful of well-known problems. Indeed, aiming too high all the time may lead to long barren periods: these may be tolerated at some stage of your life, but at the beginning of your career it is best to avoid them.
Strive for a balance in your mathematical activity: research should and does come first for real mathematicians, but in addition to doing research, do plenty of reading and teach well. Have fun with mathematics at all levels, even if it has (almost) no bearing on your research. Teaching should not be a burden but a source of inspiration.
Research should never be a chore (unlike writing up): you should choose problems that you find it difficult not to think about. This is why it is good if you get yourself hooked on problems rather than working on problems as if you were doing a task imposed on you. At the very beginning of your career, when you are a research student, you should use your experienced supervisor to help you judge problems that you have found and like, rather than working on a problem that he has handed to you, which may not be to your taste. After all, your supervisor should have a fairly good idea whether a certain problem is worth your efforts or not, while he may not yet know your strength and taste. Later in your career, when you can no longer rely on your supervisor, it is frequently inspiring to talk to sympathetic colleagues.
I would recommend that at any one time you have problems of two types to work on.
(i) |
A “dream”: a big problem that you would love to solve, but you cannot reasonably expect to solve. |
(ii) |
Some very worthwhile problems that you feel you should have a good chance of solving, given enough time, effort, and luck. |
In addition, there are two more types you should consider, although these are less important than the previous ones.
(i) |
From time to time, work on problems that should be below your dignity and that you can be confident of doing rather quickly, so that time spent on them will not jeopardize your success with the proper problems. |
(ii) |
On an even lower level, it is always fun to do problems that are not really research problems (although they may have been some years ago) but are beautiful enough to spend time on: doing them will give you pleasure and will sharpen your ability to be inventive. |
Be patient and persistent. When thinking about a problem, perhaps the most useful device you can employ is to bear the problem in mind all the time: it worked for Newton, and it has worked for many a mortal as well. Give yourself time, especially when attacking major problems; promise yourself that you will spend a certain amount of time on a big problem without expecting much, and after that take stock and decide what to do next. Give your approach a chance to work, but do not be so wrapped up in it that you miss other ways of attacking the problem. Be mentally agile: as Paul Erd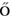 s put it, keep your brain open.
s put it, keep your brain open.
Do not be afraid to make mistakes. A mistake for a chess player is fatal; for a mathematician it is par for the course. What you should be terrified of is a blank sheet in front of you after having thought about a problem for a little while. If after a session your wastepaper basket is full of notes of failed attempts, you may still be doing very well. Avoid pedestrian approaches, but always be happy to put in work. In particular, doing the simplest cases of a problem is unlikely to be a waste of time and may well turn out to be very useful.
When you spend a significant amount of time on a problem, it is easy to underestimate the progress you have made, and it is equally easy to overestimate your ability to remember it all. It is best to write down even your very partial results: there is a good chance that your notes will save you a great deal of time later.
If you are lucky enough to have made a breakthrough, it is natural to feel fed up with the project and to want to rest on your laurels. Resist this temptation and see what else your breakthrough may give you.
As a young mathematician, your main advantage is that you have plenty of time for research. You may not realize it, but it is very unlikely that you will ever again have as much time as you do at the beginning of your career. Everybody feels that there is not enough time to do mathematics, but as the years pass this feeling gets more and more acute, and more and more justified.
Turning to reading, young people are at a disadvantage when it comes to the amount of mathematics they have read, so to compensate for this, read as much as you can, both in your general area and in mathematics as a whole. In your own research area, make sure that you read many papers written by the best people. These papers are often not as carefully written as they could be, but the quality of the ideas and results should amply reward you for the effort you have to make to read them. Whatever you read, be alert: try to anticipate what the author will do and try to think up a better attack. When the author takes the route you had in mind, you will be happy, and when he chooses to go a different way, you can look forward to finding out why. Ask yourself questions about the results and proofs, even if they seem simpleminded: they will greatly help your understanding.
On the other hand, it is often useful not to read up everything about an open problem you are about to attack: once you have thought deeply about it and apparently got nowhere, you can (and should) read the failed attempts of others.
Keep your ability to be surprised, do not take phenomena for granted, appreciate the results and ideas you read. It is all too easy to think that you know what is going on: after all, you have just read the proof. Outstanding people often spend a great deal of time digesting new ideas. It is not enough for them to know a circle of theorems and understand their proofs: they want to feel them in their blood.
As your career progresses, always keep your mind open to new ideas and new directions: the mathematical landscape changes all the time, and you will probably have to as well if you do not want to be left behind. Always sharpen your tools and learn new ones.
Above everything, enjoy mathematics and be enthusiastic about it. Enjoy your research, look forward to reading about new results, feed the love of mathematics in others, and even in your recreation have fun with mathematics by thinking about beautiful little problems you come across or hear from your colleagues.
If I wanted to sum up the advice we should all follow in order to be successful in the sciences and the arts, I could hardly do better than recall what Vitruvius wrote over two thousand years ago:
Neque enim ingenium sine disciplina aut disciplina sine ingenio perfectum artificem potest efficere.
For neither genius without learning nor learning without genius can make a perfect artist.
III. Alain Connes
Mathematics is the backbone of modern science and a remarkably efficient source of new concepts and tools for understanding the “reality” in which we participate. The new concepts themselves are the result of a long process of “distillation” in the alembic of human thought.
I was asked to write some advice for young mathematicians. My first observation is that each mathematician is a special case, and in general mathematicians tend to behave like “fermions,” i.e., they avoid working in areas that are too trendy, whereas physicists behave a lot more like “bosons,” which coalesce in large packs, often “overselling” their achievements—an attitude that mathematicians despise.
It might be tempting at first to regard mathematics as a collection of separate branches, such as geometry, algebra, analysis, number theory, etc., where the first is dominated by the attempt to understand the concept of “space,” the second by the art of manipulating symbols, the third by access to “infinity” and the “continuum,” and so on.
This, however, does not do justice to one of the most important features of the mathematical world, namely that it is virtually impossible to isolate any of the above parts from the others without depriving them of their essence. In this way the corpus of mathematics resembles a biological entity, which can only survive as a whole and which would perish if separated into disjoint pieces.
The scientific life of mathematicians can be pictured as an exploration of the geography of the “mathematical reality” which they unveil gradually in their own private mental frame.
This process often begins with an act of rebellion against the dogmatic descriptions of that space that can be found in existing books. Young, prospective mathematicians begin to realize that their own perception of the mathematical world captures some features that do not quite fit in with the existing dogma. This initial rebellion is, in most cases, due to ignorance, but it can nevertheless be beneficial, as it frees people from reverence for authority and allows them to rely on their intuition, provided that that intuition can be backed up by actual proofs. Once a mathematician truly gets to know, in an original and “personal” manner, some small part of the mathematical world, however esoteric it may look at first,1 the journey can properly start. It is of course vital not to break the “fil d’Arianne” (“Ariadne’s thread”): that way one can constantly keep a fresh eye on whatever one encounters along the way, but one can also go back to the source if one ever begins to feel lost.
It is also vital to keep moving. Otherwise, one risks confining oneself to a relatively small area of extreme technical specialization, thereby limiting one’s perception of the mathematical world and of its huge, even bewildering, diversity.
The fundamental point in this respect is that, even though many mathematicians have spent their lives exploring different parts of that world, with different perspectives, they all agree on its contours and interconnections. Whatever the origin of one’s journey, one day, if one walks far enough, one is bound to stumble on a well-known town: for instance, elliptic functions, modular forms, or zeta functions. “All roads lead to Rome,” and the mathematical world is “connected.” Of course, this is not to say that all parts of mathematics look alike, and it is worth quoting what Grothendieck says (in Récoltes et Semailles) in comparing the landscape of analysis in which he first worked with that of algebraic geometry, in which he spent the rest of his mathematical life:
I will end this article with some more “practical” advice. Note, though, that each mathematician is a “special case” and one should not take the advice too seriously.
Walks. One very sane exercise, when fighting with a very complicated problem (often involving computations), is to go for a long walk (no paper or pencil) and do the computation in one’s head, irrespective of whether one initially feels that “it is too complicated to be done like that.” Even if one does not succeed, it trains the live memory and sharpens one’s skills.
Lying down. Mathematicians usually have a hard time explaining to their partner that the times when they work with most intensity are when they are lying down in the dark on a sofa. Unfortunately, with e-mail and the invasion of computer screens in all mathematical institutions, the opportunity to isolate oneself and concentrate is becoming rarer, and all the more valuable.
Being brave. There are several phases in the process that leads to the discovery of new mathematics. While the checking phase is scary, but involves just rationality and concentration, the first, more creative, phase is of a totally different nature. In some sense, it requires a kind of protection of one’s ignorance, since this also protects one from the billions of reasons there will always be for not looking at a problem that has already been unsuccessfully attacked by many other mathematicians.
Setbacks. Throughout their working lives, including at the very early stages, mathematicians will receive preprints from competitors and feel disrupted. The only suggestion I have here is to try to convert this feeling of frustration into an injection of positive energy for working harder. However, this is not always easy.
Grudging approbation. A colleague of mine once said, “We [mathematicians] work for the grudging approbation of a few friends.” It is true that, since research work is of a rather solitary nature, we badly need that approbation in one way or another, but quite frankly one should not expect much. In fact, the only real judge is oneself. Nobody else is in as good a position to know what work was involved, and caring too much about the opinion of others is a waste of time: so far no theorem has been proved as the result of a vote. As Feynman put it, “Why do you care what other people think?”
IV. Dusa McDuff
I started my adult life in a very different situation from most of my contemporaries. Always brought up to think I would have an independent career, I had also received a great deal of encouragement from my family and school to do mathematics. Unusually, my girls’ school had a wonderful mathematics teacher who showed me the beauty of Euclidean geometry and calculus. In contrast, I did not respect the science teachers, and since those at university were not much better I never really learned any physics.
Very successful within this limited sphere, I was highly motivated to be a research mathematician. While in some respects I had enormous self-confidence, in other ways I grew to feel very inadequate. One basic problem was that somehow I had absorbed the message that women are second rate as far as professional life is concerned and are therefore to be ignored. I had no female friends and did not really value my kind of intelligence, thinking it boring and practical (female), and not truly creative (male). There were many ways of saying this: women keep the home fires burning while men go out into the world, women are muses not poets, women do not have the true soul needed to be a mathematician, etc. And there still are many ways of saying this. Recently an amusing letter circulated among my feminist friends: it listed various common and contradictory prejudices in different scientific fields, the message being that women are perceived to be incapable of whatever is most valued.
Another problem that became apparent a little later was that I had managed to write a successful Ph.D. thesis while learning very little mathematics. My thesis was in von Neumann algebras, a specialized topic that did not relate to anything with real meaning for me. I could see no way forward in that field, and yet I knew almost nothing else. When I arrived in Moscow in my last year of graduate study, Gel’fand gave me a paper to read on the cohomology of the Lie algebra of vector fields on a manifold, and I did not know what cohomology was, what a manifold was, what a vector field was, or what a Lie algebra was.
Though this ignorance was partly the fault of an over-specialized educational system, it also resulted from my lack of contact with the wider world of mathematics. I had solved the problem of how to reconcile being a woman with being a mathematician by essentially leading two separate lives. My isolation increased upon my return from Moscow. Having switched fields from functional analysis to topology, I had little guidance, and I was too afraid of appearing ignorant to ask many questions. Also, I had a baby while I was a post-doc, and was therefore very busy coping with practical matters. At that stage, with no understanding of the process of doing mathematics, I was learning mostly by reading, unaware of the essential role played by formulating questions and trying out one’s own, perhaps naive, ideas. I also had no understanding of how to build a career. Good things do not just happen: one has to apply for fellowships and jobs and keep an eye out for interesting conferences. It would certainly have helped to have had a mentor to suggest better ways of dealing with all these difficulties.
I probably most needed to learn how to ask good questions. As a student, one’s job is not only to learn enough to be able to answer questions posed by others, but also to learn how to frame questions that might lead somewhere interesting. When studying something new I often used to start in the middle, using some complicated theory already developed by others. But often one sees further by starting with the simplest questions and examples, because that makes it easier to understand the basic problem and then perhaps to find a new approach to it. For example, I have always liked working with Gromov’s nonsqueezing theorem in symplectic geometry, which imposes restrictions on the ways a ball can be manipulated in a symplectic way. This very fundamental and geometric result somehow resonates for me, and so forms a solid basis from which to start exploring.
These days people are much more aware that mathematics is a communal endeavor: even the most brilliant idea gets meaning only from its relation to the whole. Once one has an understanding of the context, it is often very important and fruitful to work by oneself. However, while one is learning it is vital to interact with others.
There have been many successful attempts to facilitate such communication, by changing the structure of buildings, of conferences and meetings, of departmental programs, and also, less formally, of seminars and lectures. It is amazing how the atmosphere in a seminar changes when a senior mathematician, instead of going to sleep or looking bored, asks questions that clarify and open up the discussion for everyone there. Often people (both young and old) are intimidated into silence because they fear showing their ignorance, lack of imagination, or other fatal defect. But in the face of a subject as difficult and beautiful as mathematics, everyone has something to learn from others. Now there are many wonderful small conferences and workshops, organized so that it is easy to have discussions both about the details of specific theories and also about formulating new directions and questions.
The problem of how to reconcile being a woman and a mathematician is still of concern, although the idea that mathematics is intrinsically unfeminine is much less prevalent. I do not think that we women are as fully present in the world of mathematics as we could be, but there are enough of us that we can no longer be dismissed as exceptions. I have found meetings intended primarily for women to be unexpectedly worthwhile; the atmosphere is different when a lecture room is full of women discussing mathematics. Also, as is increasingly understood, the real question is how any young person can build a satisfying personal life while still managing to be a creative mathematician. Once people start working on this in a serious way, we will have truly come a long way.
V. Peter Sarnak
I have guided quite a number of Ph.D. students over the years, which perhaps qualifies me to write as an experienced mentor. When advising a brilliant student (and I have been fortunate enough to have had my fair share of these) the interaction is a bit like telling someone to dig for gold in some general area and offering just a few vague suggestions. Once they move into action with their skill and talent they find diamonds instead (and of course, after the fact one cannot resist saying “I told you so”). In these cases, and in most others as well, the role of a senior mentor is more like that of a coach: one provides encouragement and makes sure that the person being mentored is working on interesting problems and is aware of the basic tools that are available. Over the years I have found myself repeating certain comments and suggestions that may have been found useful. Here is a list of some of them.
(i) When learning an area, one should combine reading modern treatments with a study of the original papers, especially papers by the masters of our subject. One of the troubles with recent accounts of certain topics is that they can become too slick. As each new author finds cleverer proofs or treatments of a theory, the treatment evolves toward the one that contains the “shortest proofs.” Unfortunately, these are often in a form that causes the new student to ponder, “How did anyone think of this?” By going back to the original sources one can usually see the subject evolving naturally and understand how it has reached its modern form. (There will remain those unexpected and brilliant steps at which one can only marvel at the genius of the inventor, but there are far fewer of these than you might think.) As an example, I usually recommend reading Weyl’s original papers on the representation theory of compact Lie groups and the derivation of his character formula, alongside one of the many modern treatments. Similarly, I recommend his book The Concept of a Riemann Surface to someone who knows complex analysis and wants to learn about the modern theory of Riemann surfaces, which is of central importance to many areas of mathematics. It is also instructive to study the collected works of superb mathematicians such as Weyl. Besides learning their theorems one uncovers how their minds work. There is almost always a natural line of thought that leads from one paper to the next and certain developments are then appreciated as inevitable. This can be very inspiring.
(ii) On the other hand, you should question dogma and “standard conjectures,” even if these have been made by brilliant people. Many standard conjectures are made on the basis of special cases that one understands. Beyond that, they are sometimes little more than wishful thinking: one just hopes that the general picture is not significantly different from the picture that the special cases suggest. There are a number of instances that I know of where someone set out to prove a result that was generally believed to be true and made no progress until they seriously questioned it. Having said that, I also find it a bit irritating when, for no particularly good reason, skepticism is thrown on certain special conjectures, such as the Riemann hypothesis, or on their provability. While as a scientist one should certainly adopt a critical attitude (especially toward some of the artificial objects that we mathematicians have invented), it is important psychologically that we have beliefs about our mathematical universe and about what is true and what is provable.
(iii) Do not confuse “elementary” with “easy”: a proof can certainly be elementary without being easy. In fact, there are many examples of theorems for which a little sophistication makes the proof easy to understand and brings out the underlying ideas, whereas an elementary treatment that avoids sophisticated notions hides what is going on. At the same time, beware of equating sophistication with quality or with the “beef of an argument” (an expression that I apparently like to use a lot in this context: many of my former students have teased me about it). There is a tendency among some young mathematicians to think that using fancy and sophisticated language means that what they are doing is deep. Nevertheless, modern tools are powerful when they are understood properly and when they are combined with new ideas. Those working in certain fields (number theory, for example) who do not put in the time and substantial effort needed to learn these tools are putting themselves at a great disadvantage. Not to learn the tools is like trying to demolish a building with just a chisel. Even if you are very adept at using the chisel, somebody with a bulldozer will have a huge advantage and will not need to be nearly as skilful as you.
(iv) Doing research in mathematics is frustrating and if being frustrated is something you cannot get used to, then mathematics may not be an ideal occupation for you. Most of the time one is stuck, and if this is not the case for you, then either you are exceptionally talented or you are tackling problems that you knew how to solve before you started. There is room for some work of the latter kind, and it can be of a high quality, but most of the big breakthroughs are earned the hard way, with many false steps and long periods of little progress, or even negative progress. There are ways to make this aspect of research less unpleasant. Many people these days work jointly, which, besides the obvious advantage of bringing different expertise to bear on a problem, allows one to share the frustration. For most people this is a big positive (and in mathematics the corresponding sharing of the joy and credit on making a breakthrough has not, so far at least, led to many big fights in the way that it has in some other areas of science). I often advise students to try to have a range of problems at hand at any given moment. The least challenging should still be difficult enough that solving it will give you satisfaction (for without that, what is the point?) and with luck it will be of interest to others. Then you should have a range of more challenging problems, with the most difficult ones being central unsolved problems. One should attack these on and off over time, looking at them from different points of view. It is important to keep exposing oneself to the possibility of solving very difficult problems and perhaps benefiting from a bit of luck.
(v) Go to your departmental colloquium every week, and hope that its organizers have made some good choices for speakers. It is important to have a broad awareness of mathematics. Besides learning about interesting problems and progress that people are making in other fields, you can often have an idea stimulated in your mind when the speaker is talking about something quite different. Also, you may learn of a technique or theory that could be applied to one of the problems that you are working on. In recent times, a good number of the most striking resolutions of longstanding problems have come about from an unexpected combination of ideas from different areas of mathematics.
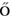 s versus Grothendieck, for example) but most mathematicians lie somewhere in between, with their work involving both the solution of problems and the development of some theory. In fact, a theory that does not lead to the solution of concrete and interesting problems is not worth having. Conversely, any really deep problem tends to stimulate the development of theory for its solution (Fermat’s last theorem being a classic example).
s versus Grothendieck, for example) but most mathematicians lie somewhere in between, with their work involving both the solution of problems and the development of some theory. In fact, a theory that does not lead to the solution of concrete and interesting problems is not worth having. Conversely, any really deep problem tends to stimulate the development of theory for its solution (Fermat’s last theorem being a classic example).