1. C The side length of the square is the radius of the circle, so the area of the circle is πr2 = 4π. Central angle CDA measures 90 degrees because ABCD is a square. 90 degrees represents 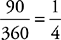 of the circle, so the area of the shaded region will be
of the circle, so the area of the shaded region will be  of the area of the circle, π. The quantities are equal.
of the area of the circle, π. The quantities are equal.
2. 60 The clock is a circle of 360 degrees, and the 12 numbers create 12 equal intervals around the clock. Therefore, each interval between two consecutive numbers must equal 30 degrees. At 10:00, the two hands are two numbers apart, and create an angle of 60 degrees.
3. B The formula for the area of a circle is πr2, where r is the radius of the circle. If you set this formula equal to the area of circle C, you get πr2 = 9π. Dividing by π on both sides of the equation yields r2 = 9, and taking the square root of both sides
results in r = 3. The radius of circle C is 3, giving you choice (B) for the answer.
4. D All diameters in a circle are of equal length. Draw a horizontal diameter in the smallest circle; it must be 2 units long. This diameter is also the radius of the circle with center B, whose diameter must therefore be 4 units long. Draw this diameter horizontally, and you realize that it is also the radius of the circle with center A, whose area is πr2 = 16π.
5. B The circumference of a circle with a diameter of 6 is πd = 6π. The circumference of a circle with a radius of 12 is 2πr = 24π, so choice (B) is larger.
6. C For this problem, use the circle formulas—Area = πr2 and Circumference = 2πr—and do the problem one step at a time. For Quantity A, a circle with a circumference of 4π yields 4π = 2πr, so 2r = 4, and r = 2; thus, the area of the circle is 22π, or
4π, and 4 times that is 16π. For Quantity B, a circle with an area of 64π yields 64π = πr2, so r2 = 64, and r = 8; thus, the circumference of the circle is 2(8)π, or 16π. The quantities are equal.
7. 5 First, determine the number of slices that will satisfy the question: There are 20 employees that need at least two slices each, so you need a total of at least 40 slices. Next, determine how many slices each pizza has: Each slice has a central angle of 40˚ out of 360˚, so each pizza has  = 9
slices. Since 4 pizzas would only provide 36 slices, you need one more pizza, so 5 is the correct response.
= 9
slices. Since 4 pizzas would only provide 36 slices, you need one more pizza, so 5 is the correct response.
8. B Each angle in an equilateral triangle measures 60°. The degree measure of the darkened arc is therefore 60°, which represents  of the 360° in the circle. Thus, the length of the darkened arc will be
of the 360° in the circle. Thus, the length of the darkened arc will be  of the circumference of the circle. If the diameter is 6, the radius is 3, so the circumference is 2πr = 6π.
of the circumference of the circle. If the diameter is 6, the radius is 3, so the circumference is 2πr = 6π.  of 6π is π.
of 6π is π.
9. A For Quantity A, the circumference of C is 2πr = 2π(6) = 12π; the radius is 6. So, the ratio is 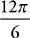 = 2π. For Quantity B, half the diameter is the same as the radius, 6. Ballpark that 2π is a little more than 6, making Quantity A greater.
= 2π. For Quantity B, half the diameter is the same as the radius, 6. Ballpark that 2π is a little more than 6, making Quantity A greater.
10. A Notice that chord AB goes through the center of the circle. Thus, AB is a diameter; a diameter is the longest chord in a circle. Chord CD does not go through the center of the circle, so AB must be longer than CD.
11. 864 Draw the diagram of the circle in the square, and draw the radii of length 3 from the center straight up and down. This allows you to see that the side of the square is equal to the diameter of the circle and equals 6. The perimeter of the square equals the sum of all the sides, or 24, and the area of the square equals the side squared, or 36. Use your on-screen calculator to find that the product of the perimeter and the
area of the square is 864.
12. C, D, and F
Consider all the possible different integer pairs for the dimensions of the rectangle. You cannot try the integer pair of 1 and 9 or 5 and 5, because you know that x < y. If the rectangle has sides of 4 and 6, you can solve for the diagonal (equal to the circle’s diameter) with the Pythagorean theorem, which gives you 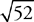 or
or  , correct choice (C). If the rectangle has sides of 3 and 7, the diagonal is
, correct choice (C). If the rectangle has sides of 3 and 7, the diagonal is 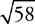 , correct choice (D). If the rectangle has sides of 2 and 8, the diagonal is
, correct choice (D). If the rectangle has sides of 2 and 8, the diagonal is  , or
, or  , correct
choice (F).
, correct
choice (F).
13. A Plug in 10 for the height and radius of the cylinder. So Quantity A is πr2h = π9211 = 891π. Quantity B is π8212 = 768π.
14. C Draw a fourth triangle and semicircle, and you can see that the figure shown represents 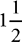 circles and
circles and  of a square. Because the three diameters are perpendicular and congruent, they represent three sides of a square; the isosceles right
triangles shown constitute three of the four triangles in the completed square. The area of a circle with diameter of 8 (and radius of 4) is πr2 = 16π.
of a square. Because the three diameters are perpendicular and congruent, they represent three sides of a square; the isosceles right
triangles shown constitute three of the four triangles in the completed square. The area of a circle with diameter of 8 (and radius of 4) is πr2 = 16π. 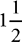 times this area is 24π. Eliminate choices (A), (D), and (E) because they do not contain 24π. The diameter of each semicircle is the length of the side of the square. The area of the entire
square would be s2 = 82 = 64.
times this area is 24π. Eliminate choices (A), (D), and (E) because they do not contain 24π. The diameter of each semicircle is the length of the side of the square. The area of the entire
square would be s2 = 82 = 64.  of this area is 48. Adding the two areas together gives you the expression in choice (C).
of this area is 48. Adding the two areas together gives you the expression in choice (C).
15. B To find the shaded region, subtract the unshaded region (the triangle and semicircle) from the entire triangle. The area of an equilateral triangle of side x is 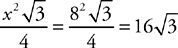 . Triangle BCD is also equilateral, and has sides of length 4, so its area is
. Triangle BCD is also equilateral, and has sides of length 4, so its area is 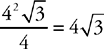 . The radius of the circle is 2, so the area of the semicircle is
. The radius of the circle is 2, so the area of the semicircle is 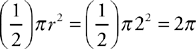 . So the answer is
. So the answer is 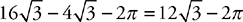 .
.
16. B Plug in a value for p. If p = 8, then the side of the square is 2 and the area is 4. If the circumference of the circle is 8, then the radius is  , and the area is
, and the area is  —approximately 5. Quantity B is larger. Plug in another value for
p and you will find that Quantity B remains larger.
—approximately 5. Quantity B is larger. Plug in another value for
p and you will find that Quantity B remains larger.
17. C Start by plugging in a radius for the smaller circles; try r = 2. The circumference of each circle is 2πr = 4π, and the sum of all three circumferences is 12π. Because the diameter of circle O is equal to the sum of the 3 shorter diameters, the diameter of circle O is 4 + 4 + 4 = 12, its radius is 6, and its circumference is 12π, so the quantities
are equal.
1. B For Quantity B, the side of the square is the same length as the diameter of the circle. The diameter is twice the radius, so the radius is 6. Plug this into the formula for area: A = πr2 to find that A = 36π. Quantity B is greater.
2. D The diameter of the circle is 12, so the radius is 6, and the area is 36π. The total number of parts in the ratio is 3 + 4 + 5 = 12, so each part covers an area of 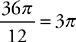 . The largest ratio part is 5 times this amount, or 15π.
. The largest ratio part is 5 times this amount, or 15π.
3. 28 First, draw the circle inside a square. Because the square has an area of 36, each side is 6. This means that the diameter of the circle is 6 and the radius is 3. Using the circle area formula, the answer is 9π, which rounds down to 28.
4. C The four half-cylinders are equivalent to two cylinders of radius 1, whose total volume will therefore be 2(π126) = 12π. The answer is choice (C).
5. D Draw in either diagonal of the square, which also is the diameter of the circle. You have now created two isosceles right triangles, so the length of the diagonal/diameter is  , and the radius is
, and the radius is 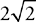 . The area of the circle is
. The area of the circle is 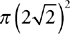 = 8π.
= 8π.
6. E Plug in 4 for circle B’s diameter; thus circle A’s diameter is 32. The radius of A is 2, and the radius of B is 16; circle B has an area of 4π and circle A has an area of 256π. The ratio is 256π:4π, which reduces to 64:1.
7. 3.4 First, draw and label the figure. Each of the triangles formed by the origin and the two vertices has legs of 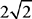 and
and 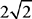 . Since each one is an isosceles right triangle—in other words, a 45-45-90 triangle—the sides are in the ratio x:x:
. Since each one is an isosceles right triangle—in other words, a 45-45-90 triangle—the sides are in the ratio x:x:  , and the long side of each is
, and the long side of each is 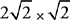 = 4. The long side of a triangle is also the side of the square, so the area of the square is 16. Since the side of the square is the same as the diameter of the circle, the diameter is 4, the radius is 2, and the area of the circle is 4π. The area inside the square but outside the circle, then, is 16 –
4π; use an approximation for π to get 16 – (4 × 3.14) = 3.44. Rounded to the nearest tenth, the answer is 3.4.
= 4. The long side of a triangle is also the side of the square, so the area of the square is 16. Since the side of the square is the same as the diameter of the circle, the diameter is 4, the radius is 2, and the area of the circle is 4π. The area inside the square but outside the circle, then, is 16 –
4π; use an approximation for π to get 16 – (4 × 3.14) = 3.44. Rounded to the nearest tenth, the answer is 3.4.
8. B A tangent to a circle forms a right angle with a radius drawn to the point of tangency. If CO is the hypotenuse of ∆OBC, then you know that the legs of the right triangle must be shorter than 5. Since OB is the radius of the circle, you know that the radius of the circle must be less than 5, so the circumference must be less than 10π.
9. C Try plugging in 5 for x. If circle A has an area of 9π, it has a radius of 3. Circle B then has an area of 9π × 5 = 45π. Circle C has an area of 45π × 5 = 225π, with a radius of 15. Therefore, the ratio of circle A’s radius of 3 to circle C’s radius of 15 is 1:5 or 1:x.
Alternatively, note that circle C’s area is the area of circle A times x2, making the ratio of the areas 1:x2. The ratio of the radii should be the square root of this ratio, because area is πr2, giving you the ratio 1:x. Both solution methods prove that the quantities are equal.
10. D The diameter of the larger circle, in inches, is 1, so the radius is  . Therefore, the area of the larger circle is
. Therefore, the area of the larger circle is 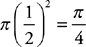 , and the area of the smaller circle is half this area,
, and the area of the smaller circle is half this area, 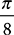 .
Setting this amount equal to the area formula allows you to determine the radius of the smaller circle:
.
Setting this amount equal to the area formula allows you to determine the radius of the smaller circle: 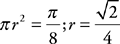 . Therefore, the diameter is
. Therefore, the diameter is 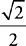 . Subtract this amount from 1 (the diameter of the larger circle):
. Subtract this amount from 1 (the diameter of the larger circle): 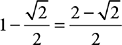 .
.
11. B Plug in a value for the radius of the circle, say r = 2, making the diameter 4; 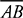 and
and  are both diameters of the circle, so Quantity A is 8. The circumference of the circle is 4π ≈ 12, so Quantity B is greater.
are both diameters of the circle, so Quantity A is 8. The circumference of the circle is 4π ≈ 12, so Quantity B is greater.
12. 40 Draw and label the figures, and then set up your scratch paper to Plug In. If circle O has a radius of 6, it has an area of πr2 = 36π; circle M, then, has a radius of 2 and an area of 4π. If sector AOB has an area of 4π out of a total area of 36π, then the sector takes up  of the entire circle, and ∠AOB represents
of the entire circle, and ∠AOB represents  of 360°. The correct answer is thus
of 360°. The correct answer is thus 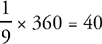 × 360 = 40.
× 360 = 40.
13. A Note that OD must be a diameter because it is the longest possible line segment crossing the circle. OA and OD (draw it in) are both radii, and therefore equal in length (3), and both of them are equal to AD. Therefore, triangle OAD is equilateral, and the measure of ∠AOD is 60°. The central angle for sector
OABC is 120° (the supplement to 60°), making this sector’s area 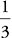 the area of the circle:
the area of the circle: 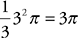 . Because π is slightly greater than 3, 3π is slightly greater than 9, giving you choice (A) for the answer.
. Because π is slightly greater than 3, 3π is slightly greater than 9, giving you choice (A) for the answer.
14. 29 Draw a rough sketch of the wall, the circles, and the spaces. Notice that there is one space for every circle, plus one more space at the end. The area of each circle is 36π, so r = 6, and the diameter of each circle is 12 inches. Convert the length of the wall into inches: 31 × 12 = 373 inches, plus the extra 6 inches equals 379 inches. You know that the wall in covered in a certain number of circles plus
spaces. Let the distance covered by a circle and a space be represented as (12 + x), and the number of circles be represented as y, so now you have y(12 + x) . You also know that there is an extra space, so add an extra x to the end, and this is now the total length of the wall. So, y(12 + x) + 1 = 379 . The question tells us that x must be an integer, and that you need the greatest number of circles, and
thus you want x to be as small as possible. Avoid solving the equation and try plugging in 1 for x, since it’s the smallest positive integer. Now the equation becomes y(12 + 1) + 1 = 379, and you can solve for y . So, 13y + 1 = 379 and 13y = 378, leading to y = 29.
15. B To solve this one, Plug In for r and m: Try r = 2 and m = 4. If  of the pizza has been eaten, and the remaining
of the pizza has been eaten, and the remaining  is divided into 4 equal slices, then each of those remaining pieces is
is divided into 4 equal slices, then each of those remaining pieces is  of the whole pizza. Now plug in 2 for r and 4 for m in the answer choices; only choice (B) hits your target answer of
of the whole pizza. Now plug in 2 for r and 4 for m in the answer choices; only choice (B) hits your target answer of  .
.
16. D The original slice is cut from a pizza with a diameter of 10, and therefore a circumference of 10π. This slice represents 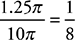 of the circumference and therefore
of the circumference and therefore  of the area,
of the area, 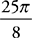 which weighs 4 ounces. A serving weighs 8 ounces, which covers double the area,
which weighs 4 ounces. A serving weighs 8 ounces, which covers double the area, 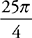 . The area of the six pizzas is (6)π32 = 54π. Dividing this by the area of one serving gives you the total number of servings that the six pizzas represent:
. The area of the six pizzas is (6)π32 = 54π. Dividing this by the area of one serving gives you the total number of servings that the six pizzas represent: 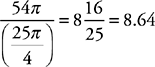 . The six pizzas yield 8
servings.
. The six pizzas yield 8
servings.
![]() of the circle, so the area of the shaded region will be
of the circle, so the area of the shaded region will be ![]() of the area of the circle, π. The quantities are equal.
of the area of the circle, π. The quantities are equal.![]() = 9
slices. Since 4 pizzas would only provide 36 slices, you need one more pizza, so 5 is the correct response.
= 9
slices. Since 4 pizzas would only provide 36 slices, you need one more pizza, so 5 is the correct response.![]() of the 360° in the circle. Thus, the length of the darkened arc will be
of the 360° in the circle. Thus, the length of the darkened arc will be ![]() of the circumference of the circle. If the diameter is 6, the radius is 3, so the circumference is 2πr = 6π.
of the circumference of the circle. If the diameter is 6, the radius is 3, so the circumference is 2πr = 6π. ![]() of 6π is π.
of 6π is π.![]() = 2π. For Quantity B, half the diameter is the same as the radius, 6. Ballpark that 2π is a little more than 6, making Quantity A greater.
= 2π. For Quantity B, half the diameter is the same as the radius, 6. Ballpark that 2π is a little more than 6, making Quantity A greater.![]() or
or ![]() , correct choice (C). If the rectangle has sides of 3 and 7, the diagonal is
, correct choice (C). If the rectangle has sides of 3 and 7, the diagonal is ![]() , correct choice (D). If the rectangle has sides of 2 and 8, the diagonal is
, correct choice (D). If the rectangle has sides of 2 and 8, the diagonal is ![]() , or
, or ![]() , correct
choice (F).
, correct
choice (F).![]() circles and
circles and ![]() of a square. Because the three diameters are perpendicular and congruent, they represent three sides of a square; the isosceles right
triangles shown constitute three of the four triangles in the completed square. The area of a circle with diameter of 8 (and radius of 4) is πr2 = 16π.
of a square. Because the three diameters are perpendicular and congruent, they represent three sides of a square; the isosceles right
triangles shown constitute three of the four triangles in the completed square. The area of a circle with diameter of 8 (and radius of 4) is πr2 = 16π. ![]() times this area is 24π. Eliminate choices (A), (D), and (E) because they do not contain 24π. The diameter of each semicircle is the length of the side of the square. The area of the entire
square would be s2 = 82 = 64.
times this area is 24π. Eliminate choices (A), (D), and (E) because they do not contain 24π. The diameter of each semicircle is the length of the side of the square. The area of the entire
square would be s2 = 82 = 64. ![]() of this area is 48. Adding the two areas together gives you the expression in choice (C).
of this area is 48. Adding the two areas together gives you the expression in choice (C).![]() . Triangle BCD is also equilateral, and has sides of length 4, so its area is
. Triangle BCD is also equilateral, and has sides of length 4, so its area is ![]() . The radius of the circle is 2, so the area of the semicircle is
. The radius of the circle is 2, so the area of the semicircle is ![]() . So the answer is
. So the answer is ![]() .
.![]() , and the area is
, and the area is ![]() —approximately 5. Quantity B is larger. Plug in another value for
p and you will find that Quantity B remains larger.
—approximately 5. Quantity B is larger. Plug in another value for
p and you will find that Quantity B remains larger.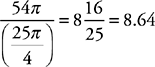 . The six pizzas yield 8
servings.
. The six pizzas yield 8
servings.