CHAPTER SIX
Bootstrapping the Zero Coupon Curve
The value of a bond is equal to the PV of each of its future cash flows discounted by the bond’s YTM or the value of a bond is equal to the present value of each cash flow discounted by its individual spot rate (that is, the yield the cash flow would yield if it was sold as a single cash flow). Figure 6.1 depicts the formula for valuing a bond at a given spot rate.
FIGURE 6.1
Formula for Valuing a Bond Given Spot Rates
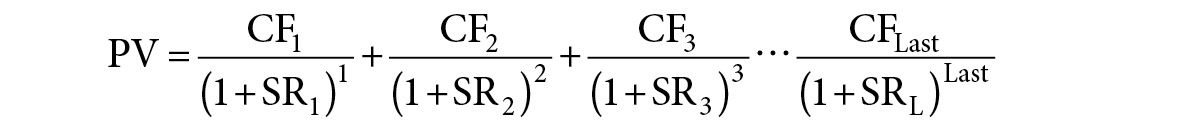
The “spot rates” can be derived from the cash market rates of new bonds issued at par. Consider the following data for newly issued AAA rated eurobonds:
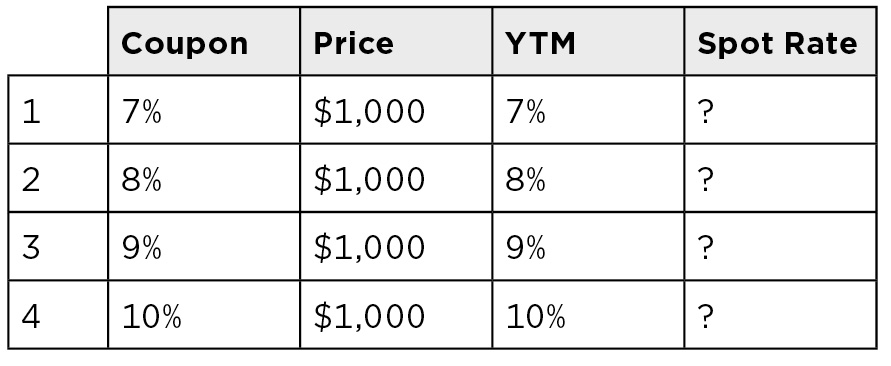
The 1-year bond is a ZCB because it only has one cash flow. The investor buys it for $1,000 and receives $1,070 in 1 year. Thus, the 1-year spot rate and YTM are the same: 7%.
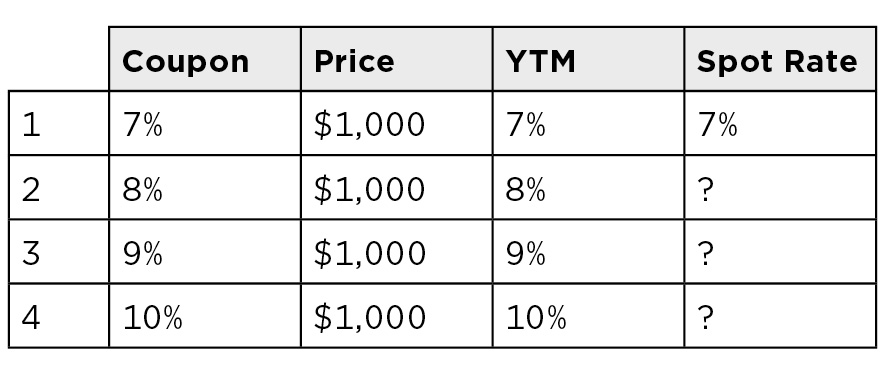
The 2-year bond has two cash flows: $80 in year 1 and $1,080 in year 2. Together, they are offered at an average discount of 8%. However, if they are sold separately, the first cash flow should be sold at a 7% discount because it occurs in 1 year. We can then solve for the second spot rate using the following formula:
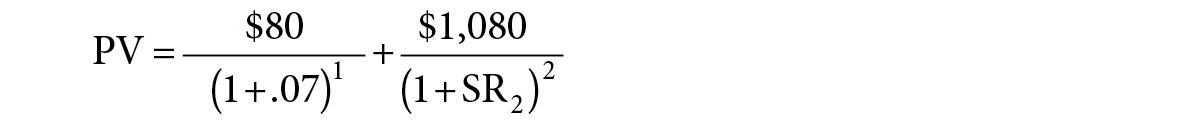
Solving for spot rate 2, we find the second spot rate is 8.04%.

The 3-year bond has three cash flows: $90 in year 1, $90 in year 2, and $1,090 in year 3. Together, they are offered at an average discount of 9%. However, if they are sold separately, the first cash flow should be sold at a 7% discount because it occurs in 1 year, and the second cash flow should be sold at an 8.04% discount. We can then solve for the third spot rate using the following formula:

Solving for spot rate 3, we find the third spot rate is 9.17%.
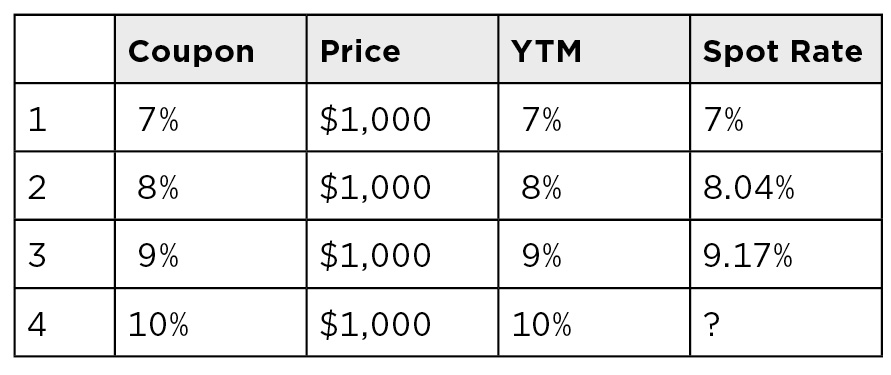
Continuing with the progression, the 4-year bond has four cash flows: $100 in year 1, $100 in year 2, $100 in year 3, and $1,100 in year 4. Together they are offered at an average discount of 10%. However, if they are sold separately, the first cash flow should be sold at a 7% discount because it occurs in 1 year, the second cash flow should be sold at a discount of 8.04%, the third cash flow should be sold at a discount of 9.17%. We can then solve for the 4th spot rate using the following formula:

Solving for spot rate 4, we find it is 10.25%.
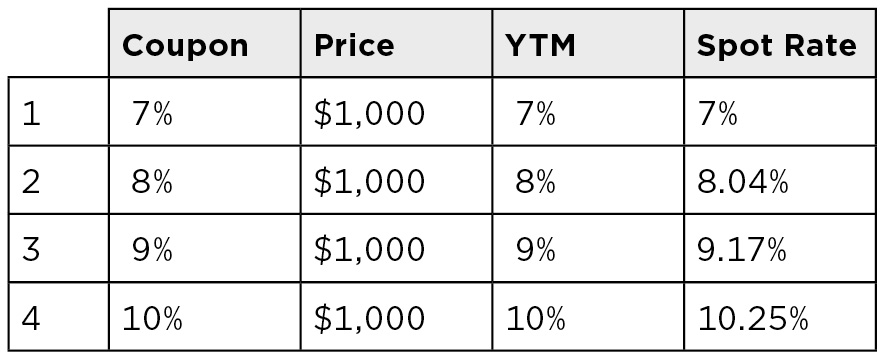
Having solved for the spot rates, we can price 4-year AAA rated bonds. Specifically, let’s price 3 different 4-year AAA rated bonds—with 0%, 10%, and 20% coupons. Note that as the coupon gets higher, more cash proportionally is discounted at the lower rates. Thus, bonds with the same credit rating and same maturity will offer different YTMs if they have different coupons. (See Figure 6.2.)
FIGURE 6.2
Three Different 4-Year Eurobonds with Three Different Coupons
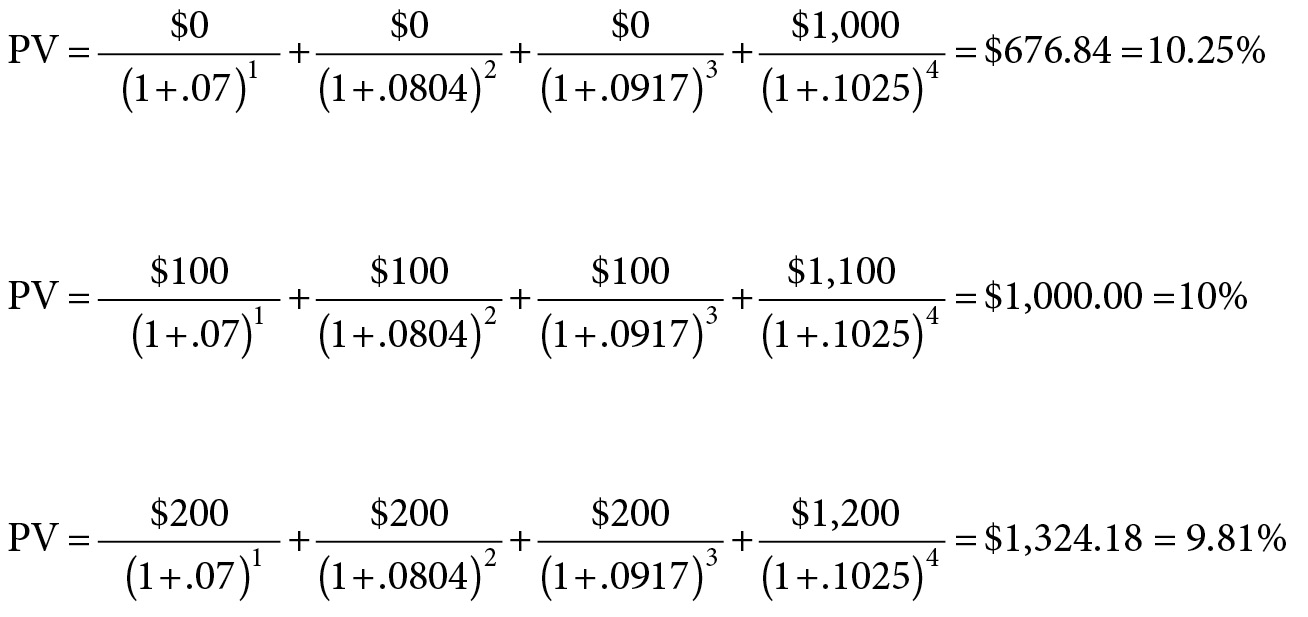
The distribution of the bond’s cash flows along the yield curve is the key to its valuation.