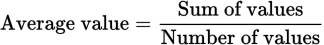
The average (arithmetic mean) of a group of numbers is defined as the sum of the values divided by the number of values.
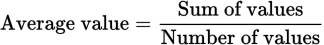
Example: Henry buys 3 items costing $2.00, $0.75, and $0.25. What is the average price?

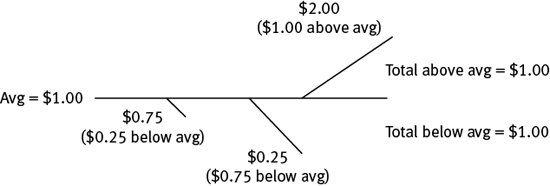
You can save yourself a lot of laborious and error-prone calculation if you think of the average as a “balancing point” between the numbers in the series. That is, the difference between the average and every number below it will equal the difference between the average and every number above it. The “balanced average” approach dramatically reduces the difficulty of the arithmetic.
You’ve already seen that the average of $2.00, $0.75, and $0.25 is $1.00. Here’s how that balances:
Now let’s use this approach to solve a problem.
Example: The average of 43, 44, 45, and x is 45. What is the value of x?

Since the amount above the average must equal the amount below the average, the amount above must be 3. Therefore, x is 3 above the average: 3 + 45 = 48; x = 48.
A further way to calculate averages on the GMAT is to use the “weighted average” formula:
Weighted average of n terms = (Percent1)(Average1) + (Percent2)(Average2) + . . . + (Percentn)(Averagen)
Weighted averages are useful when you know the average of different portions of the whole. For example, if two-fifths of the students in a class have a GPA of 79 and the remaining three-fifths have an average of 84, you could set up the weighted average formula as follows:

Notice the average of the whole class comes out to be closer to 84 than to 79. This will always be the case when the portions of the whole are of different sizes. In fact, this is where the term “weighted average” comes from; the larger the portion of the whole, the more heavily “weighted” that portion is when calculating the overall average. Note that you can use the weighted average formula as long as you have averages for all portions adding up to 100 percent of the whole.
Now let’s use the Kaplan Method on a Data Sufficiency question dealing with averages:
First, determine the type of Data Sufficiency question you’re dealing with. You need one exact value for the average score of the games, so this a Value question.
There’s no direct simplification to be done, but the fact that you’re asked for an average should alert you to the possibility that you can get the answer in a variety of ways—either through direct calculation of the scores or through a weighted average approach.
To answer the question, you’ll need either the number of games and the total of the scores or some way to calculate a weighted average.
Statement (2) is very straightforward, so starting there makes sense. This tells you the number of games but nothing about the scores. Insufficient. Eliminate (B) and (D).
Statement (1) doesn’t allow you to figure out exactly the number of games or the exact sum of the scores. But since the proportions add up to 100% of the total, and since all the bowlers bowled the same number of games, you can calculate the overall average using the weighted average approach. Sufficient. The answer is (A).
Remember, you don’t actually want to calculate the average, just know that you could. That calculation would be: overall average = 0.7(120) + 0.3(140).
The GMAT rewards those who think critically and find the most efficient approach to a problem. By using the weighted average approach, you avoided messy calculations and saved valuable time.
| Points | # of arrows |
| 0 | 1 |
| 1 | 1 |
| 3 | 3 |
| 5 | 6 |
| 7 | 8 |
| 9 | 2 |
In a certain archery contest, archers are awarded a different number of points for each arrow, depending upon the accuracy of the shot. If an arrow hits the bull’s-eye, 10 points are awarded. The results for one archer are shown in the table above, but the values do not include her bull’s-eyes. If the archer’s average score per arrow is 6.0, how many of her attempts were bull’s-eyes?
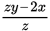

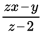
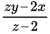

The average price of Emily’s 5 meals was $20. Meals with a price of $25 or more include a free dessert. How many of Emily’s 5 meals included a free dessert?