© Springer International Publishing AG 2017
Abdelazim M. Negm (ed.)Groundwater in the Nile Delta The Handbook of Environmental Chemistry73https://doi.org/10.1007/698_2017_75

Hydrogeophysical Characteristics of the Central Nile Delta Aquifer

Zenhom E. Salem1  , Abdelazim M. Negm2   and Abdelaziz Nahrawy1
(1)
Geology Department, Faculty of Science, Tanta University, Tanta, Egypt
(2)
Department of Water and Water Structure Engineering, Faculty of Engineering, Zagazig University, Zagazig, 44519, Egypt
 
 
Zenhom E. Salem
Email: zenhomsalem@yahoo.com
 
Abdelazim M. Negm (Corresponding author)
Email: amnegm85@yahoo.com
Email: amnegm@zu.edu.eg
1 Introduction
2 Study Area
3 Methodology
3.1 Shale Volume Estimation
3.2 Formation Water Resistivity Determination
3.3 Formation Factor Determination
3.4 Porosity Determination
3.5 Permeability Determination
3.6 Formation Water Salinity Determination
3.7 Estimation of Hydraulic Conductivity
4 Result and Discussion
4.1 Spatial Horizontal Distribution
4.2 Vertical Spatial Distribution
4.3 Hydraulic Conductivity
5 Conclusions
References

Abstract

The present study is carried out in Nile Delta aquifer, where the data of electrical resistivity and gamma ray logs of the 34 well ranging in depth between 80 and 140 m were used to calculate the aquifer parameters. It is aimed to estimate the spatial variability of the formation lithology, porosity, permeability, groundwater salinity, and the hydraulic conductivity. Bilqas Formation showed an increase in the thickness, porosity, and water salinity to the north and northeast directions. Permeability and hydraulic conductivity values decrease in the same direction. Bilqas Formation ranges in thickness from 3 m in the southwest direction to 31 m in the northeast direction. The shale content and porosity ranges are 54% to 97% and 21% to 55%, respectively. This layer has low values of permeability (16 × 10−9 to 78 × 10−9 mD) and hydraulic conductivity (<2 × 10−9 cm/s). The water salinity of this layer ranges from 200 mg to 1,600 mg/l.

In Mit Ghamr Formation, average shale content ranges from 4.5 to 22%. Numbers of scattered clay lenses are detected in different places with high intensity in the northeastern direction. Porosity ranges from 19 to 39%. High permeability values are recorded in this formation and ranged from 0.1 × 10−2 to 8.7 × 10−2 mD. The water salinity average values in this aquifer range from 220 mg to 2,100 mg/l. The calculated hydraulic conductivity values for this formation are of range 5.082 × 10−10 to 2.134 × 10−8 cm/s. In this layer, the increase in the shale content, the increase in porosity, decrease in the permeability and hydraulic conductivity, as well as the increase in salinity, are to the northern and northeastern directions.

Keywords

Hydraulic conductivityNile Delta aquiferPermeabilityPorosityWater salinity

1 Introduction

Well logs are used widely in the exploration of mineral and hydrocarbon resources because they provide detailed and reliable information about the geometrical and petrophysical characteristics of the geological structures [1]. They are applicable also for the investigation of shallow formations, for instance, in water prospecting and solving environmental and engineering geophysical problems [2]. In hydrogeophysics, the main target of well log analysts is to estimate the layer thickness, water saturation, groundwater salinity, effective porosity, clay content, and aquifer hydraulic conductivity accurately as possible.

Well logs can be basically used to distinguish the change of hydraulic conductivity along a well or between neighboring wells. All sorts of borehole-logging suites utilized as a part of hydrocarbon investigation can be used in groundwater prospecting. Gamma ray and SP logs are used for identifying lithology and determining the layer thickness [3]. Water saturation zones and invasion profiles surrounding the borehole could be detected by using high-resolution resistivity tools. Because freshwater has higher resistivity than salt water, traditional resistivity tests are reasonably used for groundwater investigation. While shallow resistivity devices determine the apparent resistivity of the zone invaded by mud, the deep resistivity tools measure the original formation resistivity. The resistivity readings should be corrected to estimate the true resistivity of groundwater formation which is significant for computing the aquifer water saturation [3]. Many researchers used geophysical well logs to calculate the aquifer petrophysical parameters; among them are Winslow and Kister [4], Paillet and Reese [5], Sloto et al. [6], Szabó [7, 8], Szabó and Dobróka [9], Szabó and Dobróka [10], and Szabó et al. [11]. As the Nile Delta aquifer is so important freshwater source for the highly populated area in Egypt, determining the spatial change in its petrophysical, hydrogeological, and hydrogeochemical characteristics is of great importance. This work aims to estimate the aquifer parameters as clay volume, porosity, clay thickness, permeability, TDS, and hydraulic conductivity for Bilqas and Mit Ghamr Formations.

2 Study Area

The study area is located at the central part of the middle Nile Delta (Fig. 1a). The geology of the Nile Delta has been extensively discussed by several researchers over several decades (among them, [12–20]). According to the mentioned studies, the general hydrogeological setting of the Nile Delta is represented by clayey formation (Bilqas Formation) underlined by sandy formation (Mit Ghamr Formation) (Fig. 2). Bilqas Formation is the top cover of the Nile Delta area and mainly consists of silts and clays and sometimes with fine-grained sands. Plant remains and peat deposits are frequent. Continental, fluviatile, lagoonal, and beach environments are the probable depositional environments of these sediments. They deposited in the advent of the third Holocene sea transgression stage progressing practically from the north and northeast directions [21]. The main aquifer in the Nile Delta is Mit Ghamr Formation which is composed of sand and gravels with thin clay intercalations. It is assumed to be of Pliocene to Quaternary age. The whole sequence of Mit Ghamr is capped by Bilqas Formation of the Holocene age. Sediments of Mit Ghamr Formation were probably deposited under shallow marine to fluvial conditions [21]. On both sides of the present Delta, these deposits form a series of gravel terraces at various heights [22].
../images/437178_1_En_75_Chapter/437178_1_En_75_Fig1_HTML.png
Fig. 1

Location map of the study area (a), boreholes location map (b) and representative geophysical log of well 9 (c). AA′ is the location of the hydrogeological cross-section shown in Fig. 2

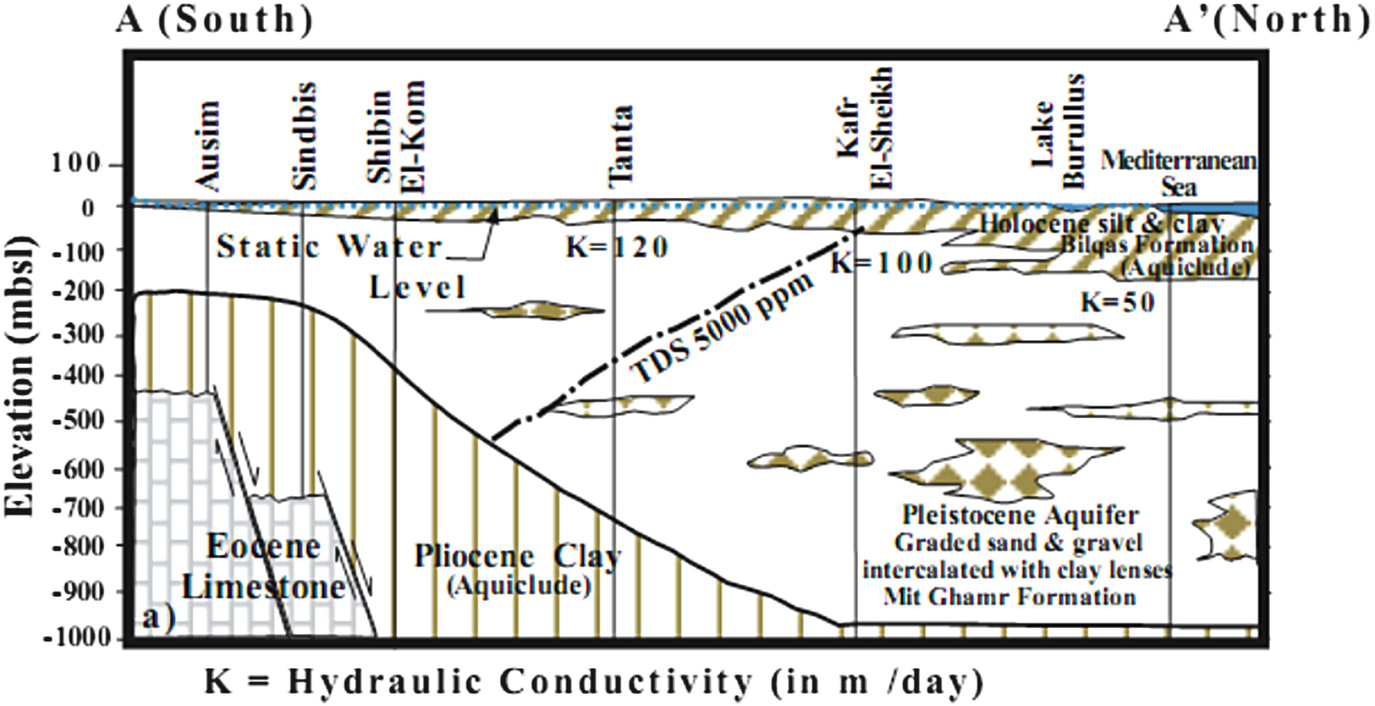
../images/437178_1_En_75_Chapter/437178_1_En_75_Fig2_HTML.png
Fig. 2

The hydrogeological cross section from south to north in the Nile Delta shows the framework groundwater regime

3 Methodology

Petrophysical data derived from 34 well logs with depths ranging between 80 and 140 m (Fig. 1b, c). Logging parameters collected included are gamma ray and resistivity logs. These logs were selected to estimate the spatial variability of the formation lithology, porosity, permeability, and water salinity. These logs were also used to estimate the hydraulic conductivity of the aquifer.

3.1 Shale Volume Estimation

Gamma ray method is considered as the best indicator method to calculate and identify the shale volume [23]. The Gamma ray readings after correction for borehole effects may be expressed as a linear function of shale content by the following equation [24]:
$$ {I}_{\mathrm{GR}}=\left({\mathrm{GR}}_{\mathrm{c}}\hbox{--} {\mathrm{GR}}_{\mathrm{min}}\right)/\left({\mathrm{GR}}_{\mathrm{max}}\hbox{--} {\mathrm{GR}}_{\mathrm{min}}\right) $$
(1)
where:

  • I GR is the gamma ray index.

  • GRc is the corrected value of gamma ray at the interest intervals.

  • GRmin is the minimum value of gamma ray opposite clean intervals.

  • GRmax is the maximum value of gamma ray opposite shale intervals.

The volume of shale (V sh) can be calculated from the gamma ray index by using the following equation [25]:
$$ {V}_{\mathrm{sh}}=0.083\left[{2}^{3.7\mathrm{IGR}}\hbox{--} 1\right]\kern1em \mathrm{Larionov}\ \mathrm{equation} $$
(2)

3.2 Formation Water Resistivity Determination

It is the water, uncontaminated by drilling mud that saturates the porous formation rock. The resistivity of the formation water (R w) is important interpretation since it is required for the calculation of saturation water from basic resistivity logs. Archie determined experimentally that the water saturation of clean formation could be expressed in the term of its true resistivity as:

R t is true resistivity which can be computed from long (LLD) and short (LLS) by using the following formula:
$$ \mathrm{If}\ \mathrm{LLD}&gt;\mathrm{LLS}\kern1em {R}_{\mathrm{t}}=1.7\ \mathrm{LLD}\hbox{--} 0.7\ \mathrm{LLS} $$
(3)
$$ \mathrm{If}\ \mathrm{LLD}&lt;\mathrm{LLS}\kern1em {R}_{\mathrm{t}}=2.4\ \mathrm{LLD}\hbox{--} 1.4\ \mathrm{LLS} $$
(4)
(Schlumberger log interpretation principle 1972)
$$ {R}_{\mathrm{o}}/{R}_{\mathrm{t}}={R}_{\mathrm{mf}}/{R}_{\mathrm{w}} $$
(5)
$$ {R}_{\mathrm{w}}=\left({R}_{\mathrm{mf}}/{R}_{\mathrm{t}}\right)/{R}_{\mathrm{o}} $$
(6)
Then
$$ {R}_{\mathrm{w}}=\left({R}_{\mathrm{mf}}\times {R}_{\mathrm{t}}\right)/\mathrm{LLS} $$
(7)

R mf is the resistivity of the mud filtrate.

R o is the flushed zone resistivity which equals (LLS) short normal resistivity (R16″).

The formation water resistivity (R w) for the Nile Delta aquifer is computed using Archie Eq. (6) after correcting the LLD readings.

3.3 Formation Factor Determination

The formation factor (F) is generally defined as the ratio of rock resistivity to water resistivity of a fully saturated rock. It is calculated as follows Archie [26]:
$$ F={R}_{\mathrm{t}}/{R}_{\mathrm{w}} $$
(8)

where R t is the true resistivity in Ω m and R w is formation water resistivity.

3.4 Porosity Determination

It is generally agreed that an empirical relationship exists between formation factor and porosity. The formation water factor is a function of porosity and also of pore structure and pore size distribution. Archie introduce this relationship in the following equation:

$$ F=\frac{a}{\ {\Phi}^m} $$
(9)
where:

a is the pore geometry coefficient, dimensionless, generally, ranges from 0.6 to 2.

Ф is porosity in percent.

m is cementation factor, dimensionless, ranges from 1 to 3.

3.5 Permeability Determination

Permeability is one of the most important and least predictable fluid transport characteristics of materials. Permeability must be known to understand several natural phenomena, such as the basin-scale hydrogeological circulation [27], fault dynamics [28], the safety of waste repositories [29], and several other subsurface hydrology problems [30]. The used methods for calculating the permeability from the well logs are discussed as follows:

3.5.1 Clean Sand

Intrinsic permeability (K) is evaluated using the following equation:

$$ K=1.828\times {10}^5\left({P}^{1.1}\right) $$
(10)
where:

  • K is the intrinsic permeability in millidarcy [31].

  • P is a porosity factor which represented by the following equation.

$$ P=\frac{\Phi^{m+2}}{{\left(1-\Phi \right)}^2} $$
(11)

3.5.2 Permeability of Clay and Clayey Sand

Based on Revil and Cathles model (1999) (Fig. 3), The classical Kozeny-Carman relationship was improved by using electric parameters which separate pore throat from total porosity and hydraulic radius. The permeability of clean sand is estimated as a function of the grain diameter, the porosity, and the electrical cementation exponent. The permeability of pure shale is derived in a similar way but is strongly dependent on clay mineralogy.
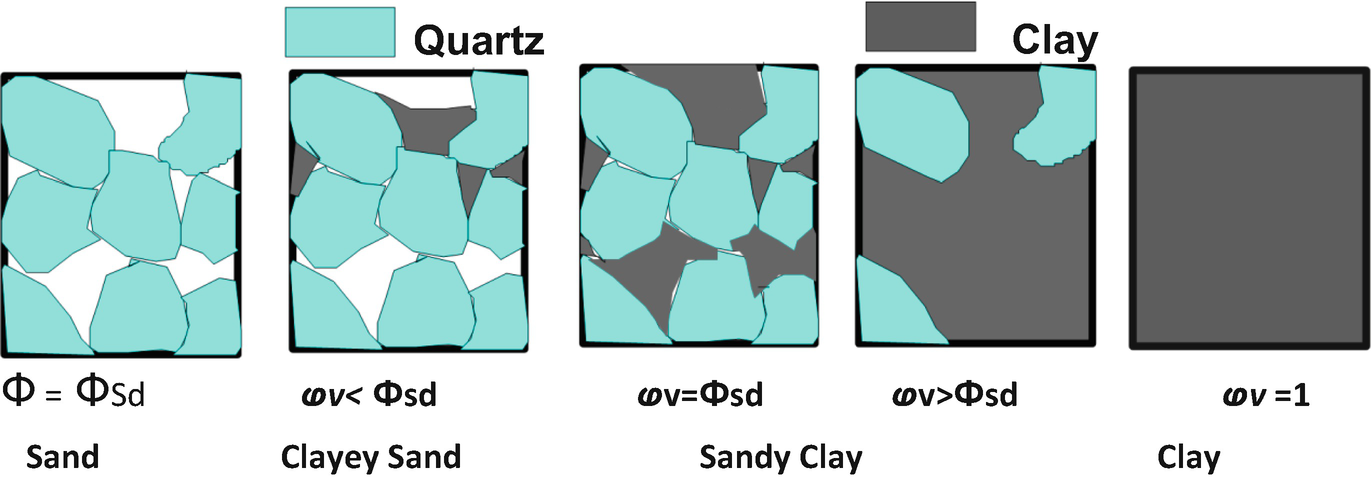
../images/437178_1_En_75_Chapter/437178_1_En_75_Fig3_HTML.png
Fig. 3

Sand-shale mixture for various shale contents. The shale content increases from the left to the right. For a clay-free sand (first end-member), the noncompacted porosity is equal to Фsd. For a clayey sand, the porosity decreases because of the presence of clay particles in the pore space. This decrease continues until the critical point where all the pore space of a clean sand is occupied by clay particles, i.e., when the shale content is equal to the porosity of a clean sand. After this point the rock is sandy shale, and an increase in shale content is only possible through replacement of quartz grains by clay particles, and the porosity increases with the shale content. The second end-member is pure shale with no quartz grains and with a porosity equal to Фsh (Note: this figure and its description are taken from [30])

Clay
The shale end-member permeability k Sh is related to the shale end-member porosity; ФSh is represented by the following equation:
$$ {k}_{\mathrm{Sh}}={K}_0\ {\left({\Phi}_{\mathrm{Sh}}/{\Phi}_0\right)}^{3\mathrm{msh}} $$
(12)
where k 0 and Ф0 are the permeability and porosity in a reference state, respectively (we take Ф0 = 0.50). Using Eq. (12), m and k 0 are calculated for each end-member clay mineralogy (kaolinite, illite, and smectite) as in (Fig. 3). The most dominant end-member clay mineral in the Nile Delta is smectite where its percent reaches more than 67% [32].
Sand-Clay Mixture
In sand-shale sediments, porosity does not depend on the shale ratio. Pure sand and shale have porosities higher than that sand-shale mixture. Porosity is decreased when pores between sand grains are filled by clay or when sand is scattered in shale. Marion et al. [33] discussed how porosity of sand and shale mixtures could be determined as follows:
$$ \Phi ={\Phi}_{\mathrm{sd}}-\omega \upsilon \left(1-{\Phi}_{\mathrm{sh}}\right)\kern1em \omega \upsilon \le {\Phi}_{\mathrm{sd}} $$
(13)
$$ \Phi =\omega \upsilon \cdot {\Phi}_{\mathrm{sh}}\kern1em \omega \upsilon \ge {\Phi}_{\mathrm{sd}} $$
(14)
where ωυ is the clay volume fraction and Фsd and Фsh are the porosity of the clean sand and pure shale end-members, respectively. The permeability of a clayey sand (K Ф) is related to the permeability of a clean sand (K sd and Фsd):
$$ K\Phi ={K}_{\mathrm{sd}}{\left(\frac{\Phi}{\Phi_{\mathrm{sd}}}\right)}^{3\mathrm{mcs}} $$
(15)
where mcs is the cementation exponent. As fine-grained clays filling the pores prevent fluid flow, mcs, therefore, depend mainly on the shale ratio, particularly near the boundary between the clayey sand and sandy shale domains. Because the flow blockage is severe near this boundary, it is intuitively expected that mcs is not just a positive function of the shale ratio yet can achieve values substantially higher than 2. mcs was expanded as a power function of ωυ:
$$ \mathrm{mcs}={\mathrm{m}}_{\mathrm{cs}}^0+{\mathrm{m}}_{\mathrm{cs}}^1\cdot \omega \upsilon +\mathrm{O}\left({\omega}^2\upsilon \right) $$
(16)
where mcs = msd is the cementation exponent of the clean sand and is in the range 1.5–2.0. Combining Eqs. (13) and (16), the permeability of clayey sand is calculated as follows:
$$ K={k}_{\mathrm{sd}}{\left[1-\omega \upsilon \left(\frac{1-{\Phi}_{\mathrm{sh}}}{\Phi_{\mathrm{sd}}}\right)\right]}^{3\mathrm{mcs}}\kern1em \omega \upsilon &lt;{\Phi}_{\mathrm{sh}} $$
(17)
Clayey Sand-Sandy Clay Boundary
In this case, ωυ = Фsd and permeability are calculated using this formula:
$$ K=k\mathrm{sd}{\left({\Phi}_{\mathrm{sh}}\right)}^{3\mathrm{mcs}} $$
(18)

where

$$ {m}_{\mathrm{cs}}={m^0}_{\mathrm{cs}}+{m^1}_{\mathrm{cs}}\ {\Phi}_{\mathrm{sd}} $$
Sandy-Clay Domain
Sandy-clay permeability can be obtained by taking into account the flow blockage by the sand grains, where the permeability in the sandy shale domain is given by:
$$ K={k}_{\mathrm{sh}}{\left(\omega v\right)}^{\mathrm{msd}}\kern1em \omega v&gt;{\Phi}_{\mathrm{sd}} $$
(19)

3.6 Formation Water Salinity Determination

Assessment of groundwater quality from well logs usually centers on values for total dissolved solids (TDS) and specific conductance (C w).

The relationship between the two terms is
$$ {R}_{\mathrm{w}}\ \left(\Omega\ \mathrm{m}\right)=10,000/{C}_{\mathrm{w}}\ \left(\upmu\ \mathrm{m}\mathrm{hos}/\mathrm{cm}\right) $$
(20)
where:

  • C w is conductivity in micromhos/cm.

  • R w is formation water resistivity.

Then the total dissolved salt (TDS) in (ppm) of the formation water is given by:
$$ \mathrm{TDS}=0.64\times {C}_{\mathrm{w}} $$
(21)

3.7 Estimation of Hydraulic Conductivity

The proportionality constant, particularly for the water flow through sediment connected pores, is known as the hydraulic conductivity; penetrability is a part of this and is just a character of the permeable media, not the liquid. Utilizing the intrinsic permeability, hydraulic conductivity can be put in term of the permeability. Given the value of hydraulic conductivity for a subsurface system, the permeability can be calculated as follows:
$$ K= ki\left(\frac{\rho g}{\mu}\right) $$
(22)
where K is the permeability (m2), kiis the hydraulic conductivity (m/s), ρ is the density (g/cm3), and μ is the kinematic viscosity of the fluid phase (g/cm/s). g is the acceleration due to gravity (cm/s). Intrinsic permeability is often expressed in square centimeters or in darcys. Intrinsic permeability is regularly communicated in square centimeters or in darcys. 9.87 × 10−9 cm2 equals one darcy.

4 Result and Discussion

4.1 Spatial Horizontal Distribution

4.1.1 Bilqas Formation

Figure 4 shows the horizontal spatial distribution of the petrophysical parameters of Bilqas Formation (upper clay layer). The thickness of this layer increases from 3 m at well 20 in the southwestern part to 31 m at well 34 in the northeastern direction (Fig. 4a). However, the calculated average values of shale volume increase from 54% at well 19 in the southwest to 97% at well 22 in the northeast with the average value 69.83% (Fig. 4b). The calculated average values of porosity increases from 21% at well 3 in the central parts to 55% at well 10 in northern parts of the study area with average value 24.71% (Fig. 4c). Average values of the calculated permeability increases from 16 × 10−9 mD at well 6 to 78 × 10−9 mD at well 5 in the northeastern direction of the study area with average value 30.71 × 10−9 mD (Fig. 4d). TDS of the water that contained in the clay of Bilqas Formation is an indication of the evaporation process and the chemical composition of the recharged water to the main aquifer (Mit Ghamr Formation). The minimum value of the calculated average TDS (200 mg/l) is recognized at well 23 in the southeastern parts and increases to north and northwestern direction (well 11, 1,450 mg/l). Well 33 (TDS = 1,600 mg/l) and well 5 (TDS = 1,560 mg/l) are forming a local higher TDS values which might be related to local contamination (Fig. 4e).
../images/437178_1_En_75_Chapter/437178_1_En_75_Fig4_HTML.png
Fig. 4

Spatial distributions maps of average values of different petrophysical parameters for Bilqas formation

4.1.2 Mit Ghamr Formation

As Mit Ghamr Formation is the main aquifer in the Nile Delta, therefore, studying the spatial change in its petrophysical properties is quite important. The lowest average values of shale volume are 4.5%, 5%, and 7.1% at wells 17, 28, and 29 in the southern part, respectively, and increase to 22% at well 30 in the northern direction (Fig. 5a). Generally, the average value of shale volume increases from southern part toward northeastern parts of the study area. The minimum average value of porosity is 19% at well 2 in the central part and increases to 39% at well 10 and 11 in the northeastern and northwestern directions of the study, respectively (Fig. 5b). As a general trend, porosity increases from the south to the northwest. The minimum counted permeability value as shown in Fig. 5c is 0.1 × 10−2 mD at the location of well 35 in the northeast, and the maximum value (8.7 × 10−2 mD) is encountered to the northwestern direction where well 11 is located. As a general trend, the average values of permeability increase from southern, eastern, and northeastern parts to the northwestern direction of the study area. In comparison, porosity and permeability have mostly the same decreasing and increasing trends. The calculated TDS values range from 220 mg/l at the location of well 28 in the central part to 2,100 mg/l at the location of well 35 in the northeastern direction (Fig. 5d). Generally, the calculated average TDS of Mit Ghamr Formation increase to the northeastern direction. The complete geochemical investigation should be done to find out whether the higher TDS in the northeastern part is related to the effect of seawater intrusion or not.
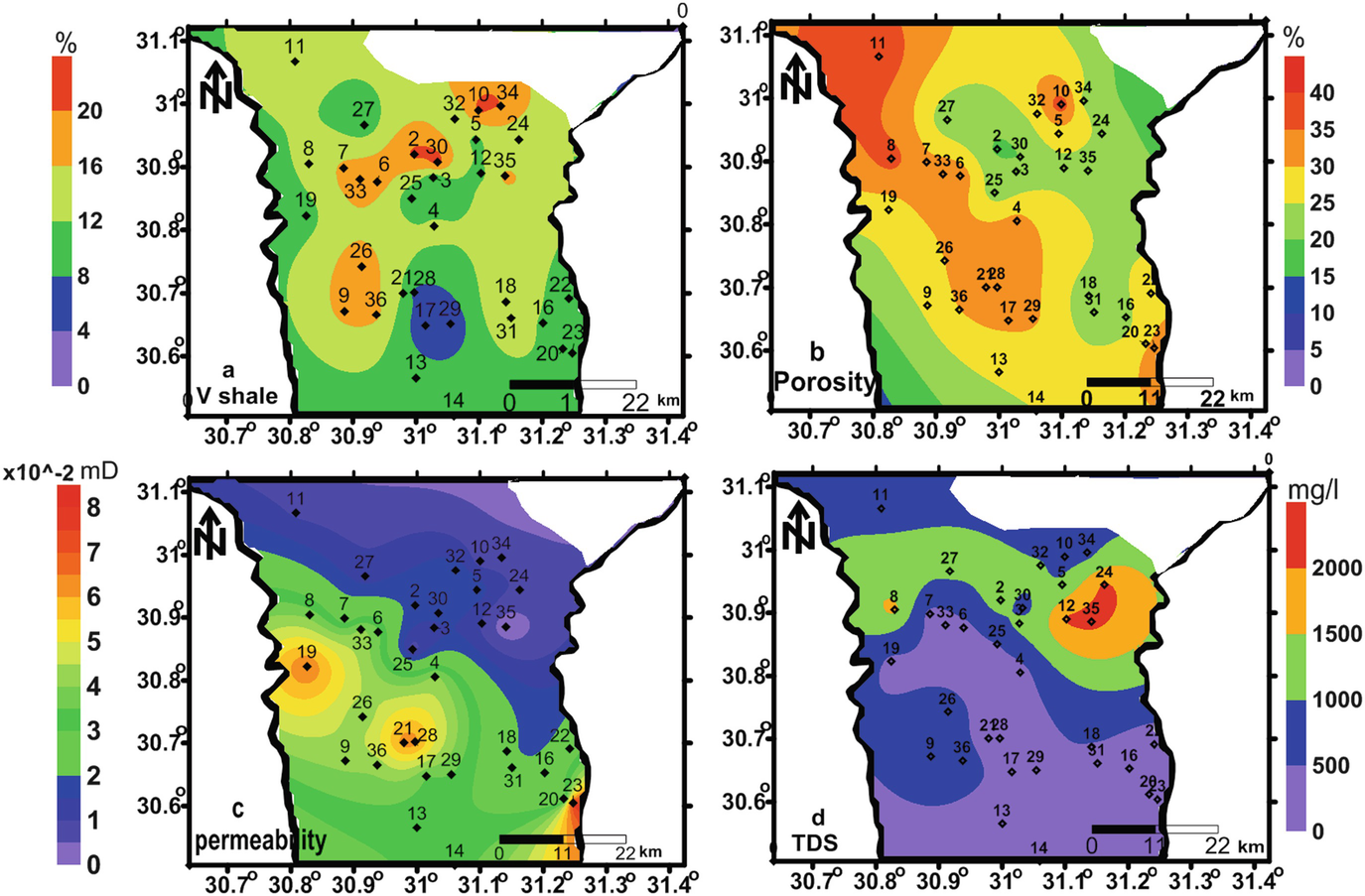
../images/437178_1_En_75_Chapter/437178_1_En_75_Fig5_HTML.png
Fig. 5

Spatial distributions maps of the calculated average values of different petrophysical parameters for Mit Ghamr formation

4.2 Vertical Spatial Distribution

The vertical distributions of the deduced petrophysical parameters along three cross sections at different locations and directions (Fig. 1b) are shown in Figs. 6, 7, 8, 9, 10, and 11. The main purpose of these cross sections is to clarify the two-dimensional images for the subsurface distributions of the petrophysical parameters. The cross section AA′ extends from south to north and includes wells 27, 2, 30, 3, 25, 4, 28, 21, 17, 13, and 14 (Figs. 6 and 7). The cross section BB′ extends east-west in the northern part of the study area and includes wells 35, 12, 30, 3, 2, 6, 33, 7, and 8 (Figs. 8 and 9). Cross section CC′ also extends east-west in the southern part of the study area and includes wells 20, 22, 16, 31, 18, 29, 17, 28, 21, 36, and 9 (Figs. 10 and 11).
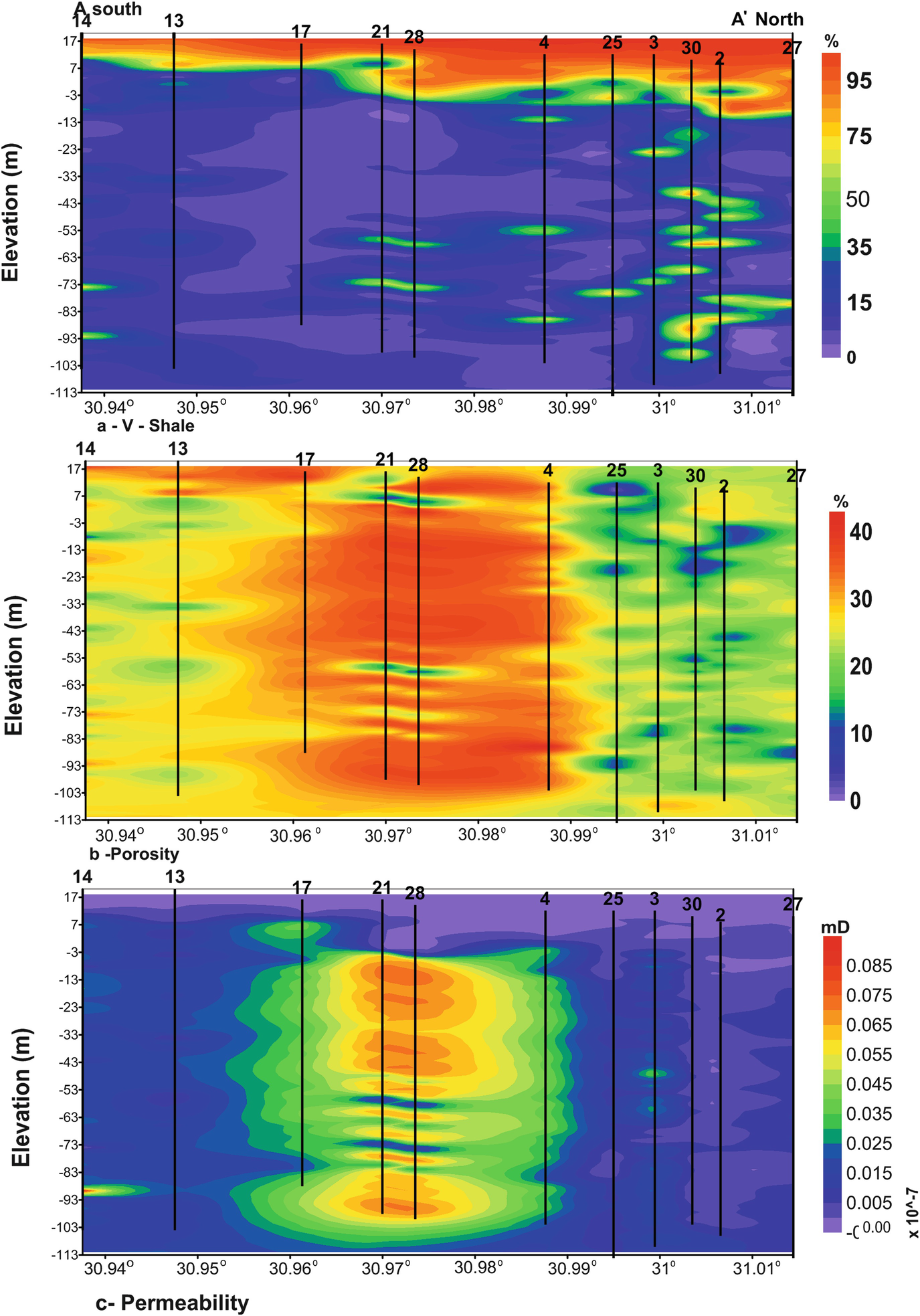
../images/437178_1_En_75_Chapter/437178_1_En_75_Fig6_HTML.png
Fig. 6

Vertical distributions of the calculated values of different petrophysical parameters along the cross section AA′

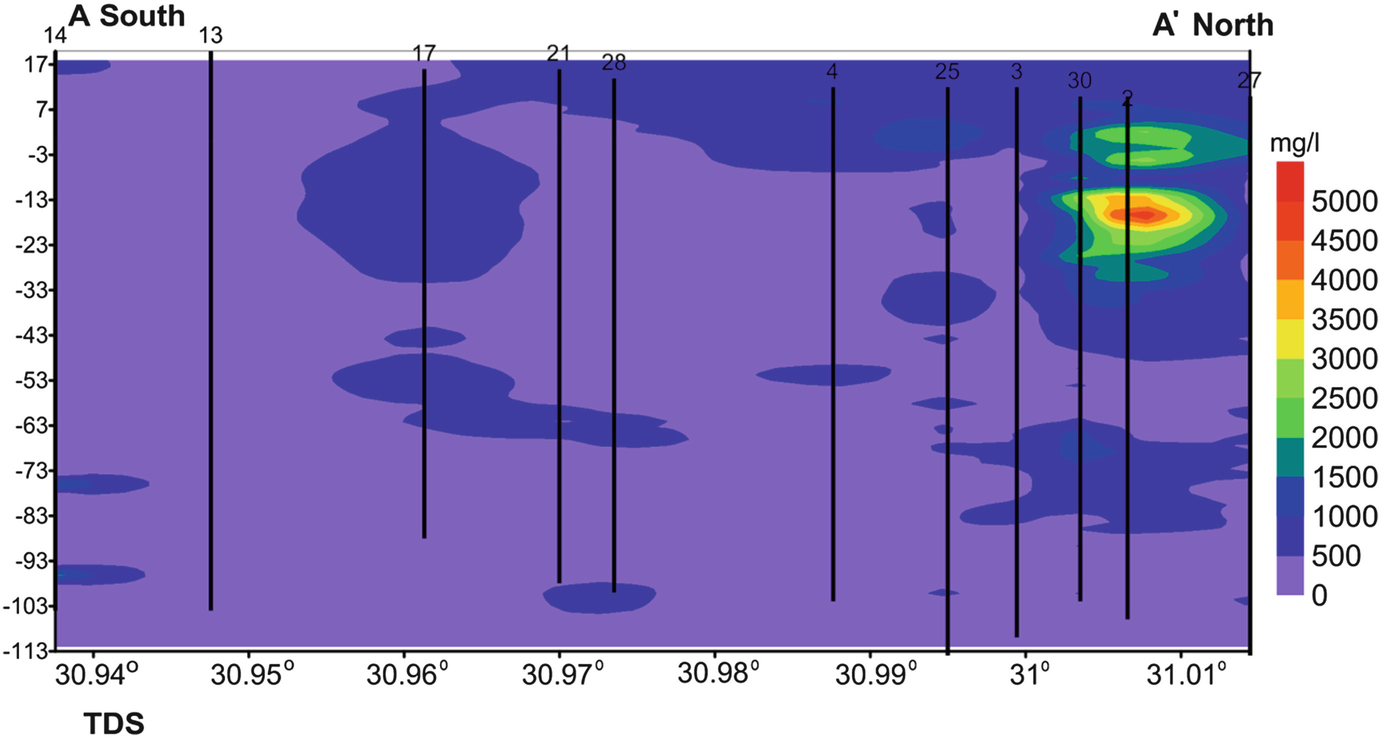
../images/437178_1_En_75_Chapter/437178_1_En_75_Fig7_HTML.png
Fig. 7

Vertical distributions of the calculated values of different groundwater salinity along the cross section AA′

../images/437178_1_En_75_Chapter/437178_1_En_75_Fig8_HTML.png
Fig. 8

Vertical distributions of the calculated values of different petrophysical parameters along the cross section BB′

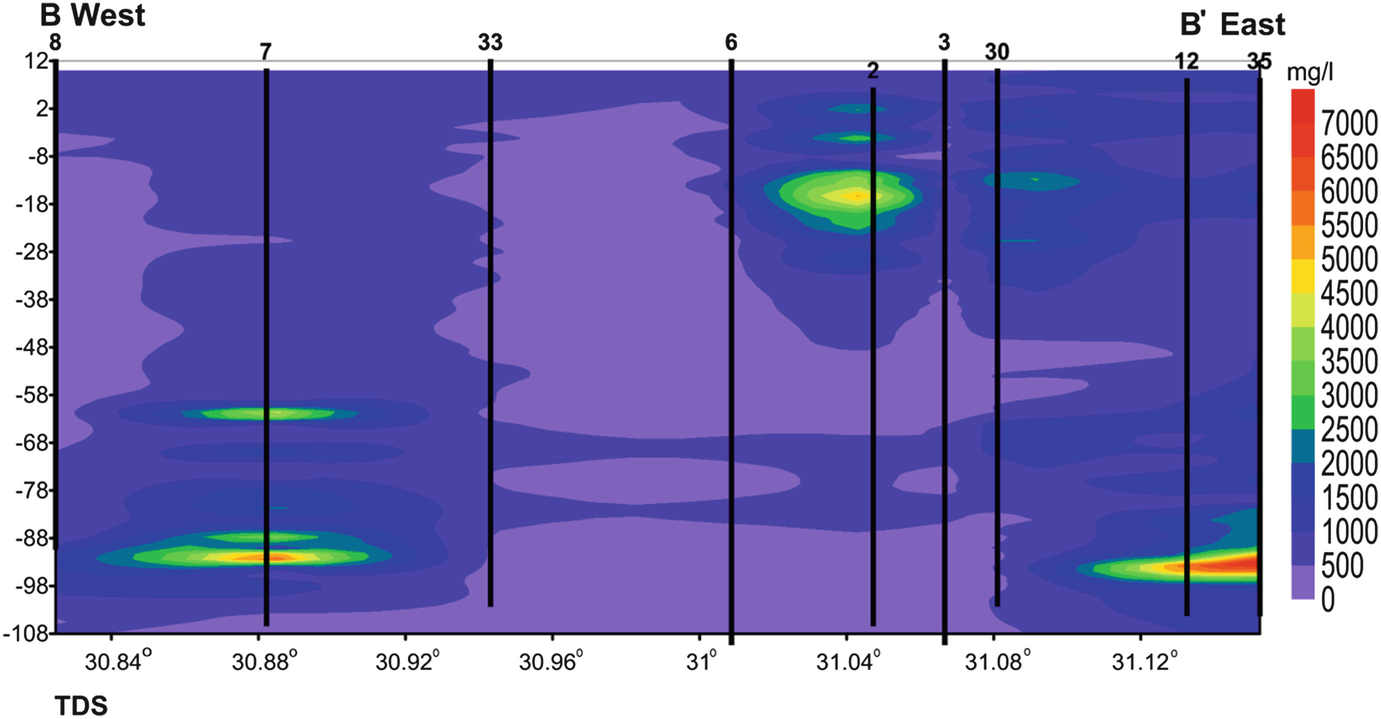
../images/437178_1_En_75_Chapter/437178_1_En_75_Fig9_HTML.png
Fig. 9

Vertical distributions of the calculated values of different groundwater salinity along the cross section BB′

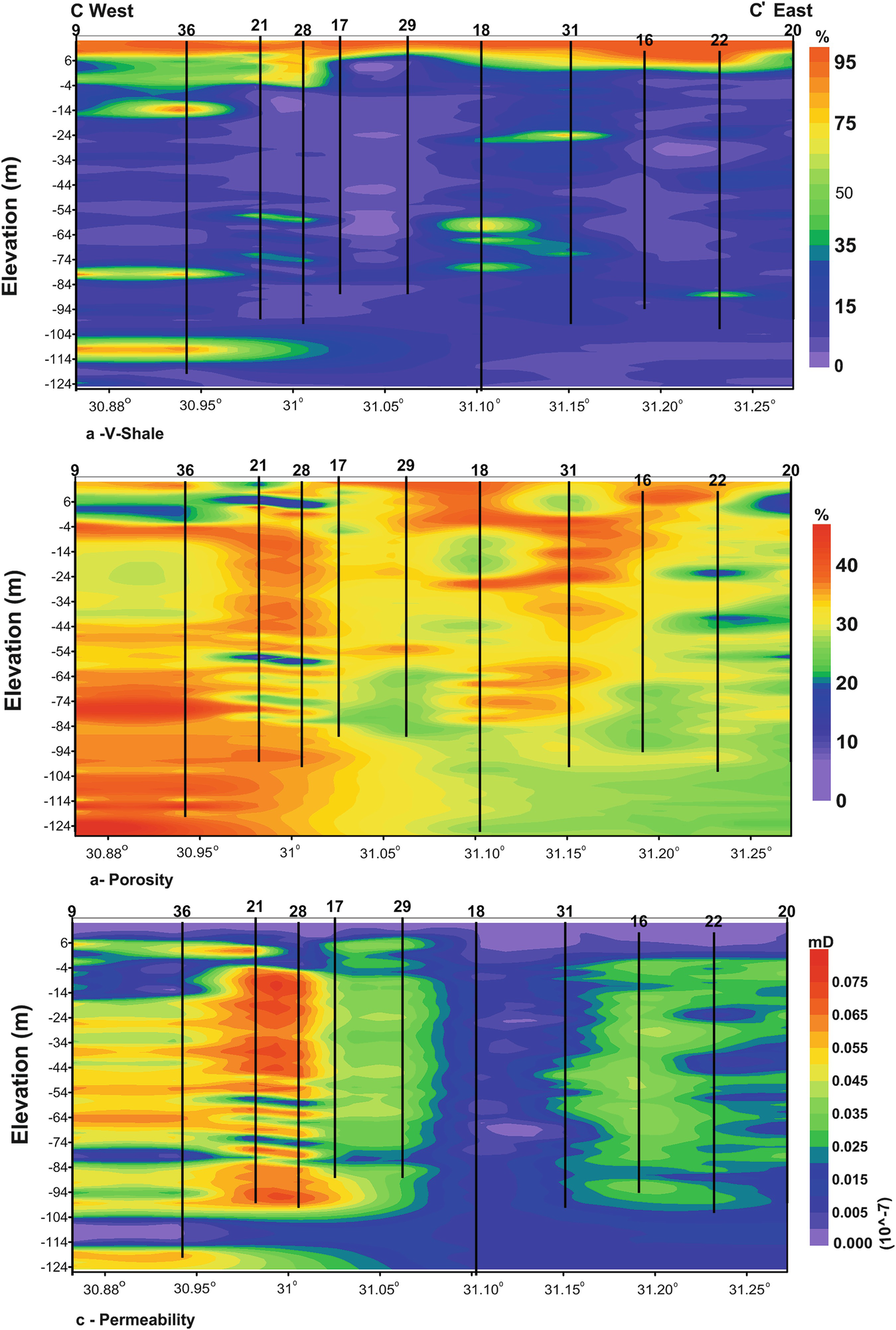
../images/437178_1_En_75_Chapter/437178_1_En_75_Fig10_HTML.png
Fig. 10

Vertical distributions of the calculated values of different petrophysical parameters along the cross section CC′

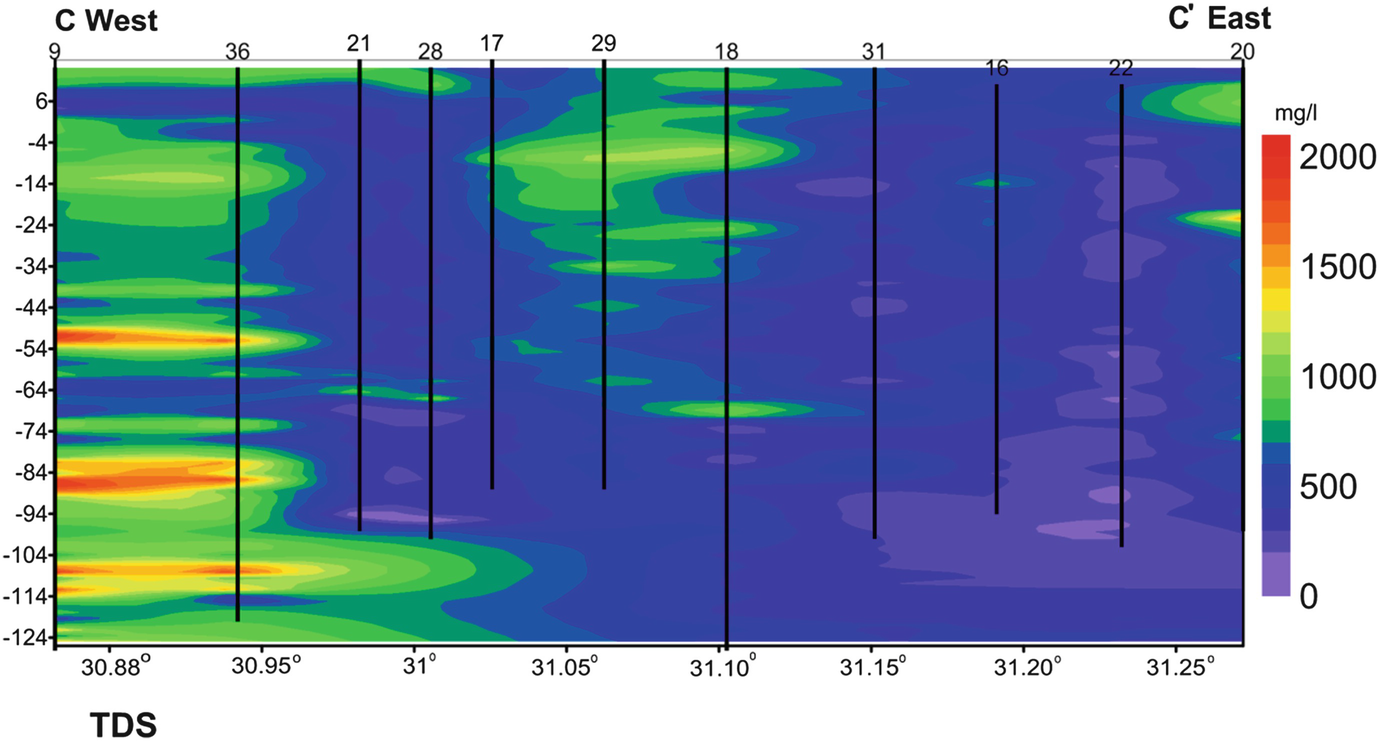
../images/437178_1_En_75_Chapter/437178_1_En_75_Fig11_HTML.png
Fig. 11

Vertical distributions of the calculated values of different groundwater salinity along the cross section CC′

4.2.1 The Clay Layer and Clay Content

The clay layer (V-shale is more than 75%) occupies the uppermost layer of the study area where its thickness increases gradually from the location of well 14 at the south to location of well 27 at the north and as lenses in wells 25, 30, 2, and 27 (cross section AA′, Fig. 6a), from location of well 8 at the west to location of well 27 at the eastern part (cross section BB′, Fig. 8a) and from well 9 at the south to location of well 22 at the eastern part (cross section CC′, Fig. 10a). The layers with V-shale ranging from 50 to 75% extends from well 14 at south to well 27 at north and as lenses in wells 25, 30, 2, 27, 3, 4, 21, 28, and 14 (cross section AA′, Fig. 6a), from location of well 8 at the west to location of well 27 at the eastern part and as lenses in wells 30, 2, 6, 33, 7, and 8 (cross section BB′, Fig. 8a) and from location of well 9 at the west to the location of well 20 at the eastern part and as lenses in wells 31, 18, 36, 9, 21, 28, and 22 (cross section CC′, Fig. 10a). As shown in the three cross sections, the sandy layer is interbedded with lenses of sandy clay and clayey sand.

4.2.2 Porosity

Porosity vertical distributions showed variable ranges in vertical and horizontal directions. Low values (0–20%) are recognized as lenses at different depths in wells, 25, 2, 3, 30, and 27 at the northern part (cross section AA′, Fig. 6b), well 7 at western part and wells 2, 3, 12, and 35 at the eastern part (cross section BB′, Fig. 8b), and wells 9, 36, 21, and 28 at western part and wells 22 and 20 at the eastern part (cross section CC′, Fig. 10b). Porosity with values ranging from 20 to 30% occupies most sections of wells 25, 3, 2, and 27 at the north and as lenses in wells 13 and 14 in southern parts (cross section AA′, Fig. 6b), most wells at the eastern part and in wells 7 and 8 at western part (cross section BB′, Fig. 8b) and most wells of the cross section CC′ (Fig. 10b). The porosity values higher than 30% were represented in wells 17, 21, 28, and 4 which occupies the central part of the study area and also found in the upper parts of wells 13 and 14 and extend toward south direction (cross section AA′, Fig. 6b), wells 33 and 6 at the central part and as lenses in well 7 and 8 at the western part (cross section BB′, Fig. 8b) and most of the wells of the cross section CC′ (Fig. 10b).

4.2.3 Permeability

Permeability vertical distributions showed variable ranges in vertical and horizontal directions. Very low values (>0.005 × 10−7 mD) are recognized at the upper most layer of the study area (upper clay layer of Bilqas Formation) which represented in the three cross sections (Figs. 6c, 8c, and 10c). Low permeability values of Mit Ghamr Formation ranging from 0.005 × 10−7 mD to 0.01 × 10−4 mD are shown in the northern part of cross section AA′ (Fig. 6c), in the eastern part of cross section BB′ (Fig. 8c), and in wells 18 and as lenses in wells 22, 31, 36, and 9 (section CC′, Fig. 10c). The permeability values generally increase to its highest values toward, the central part (wells 21 and 28) in cross section AA′ (Fig. 6c), the western direction (wells 7, 8, 33, 6) in cross section BB′ (Fig. 8c), and the eastern and western parts especially wells 21 and 28 in cross section CC′ (Fig. 10c).

4.2.4 Salinity

The salinity values (in term of the calculated TDS) distribution showed variable ranges in vertical and horizontal directions. TDS with values less than 500 mg/l occupy wells 13 and 14 and most parts of the other wells at depths more than 20 m (cross section AA′, Fig. 7), wells 8, 33, 6, 2, 3, 30, 2, and 30 as lenses at certain depths (cross section BB′, Fig. 9), and wells 22 and 31 and as lenses in wells 36, 21, 28, 17, 18, and 16 (cross section CC′, Fig. 11). TDS values ranging from 500 to 1,000 mg/l exhibit as lenses in wells 17, 21, 29, 2, 4, 25, 30, and 27(cross section A-A′, Fig. 7), wells 7 and as lenses in wells 2, 30, 12, and 35 at the eastern part (cross section BB′, Fig. 9), and wells 18, 29, 26, and 9 at the west and well 20 at the east (cross section CC′, Fig. 11). The highest TDS values (>1,000 mg/l) are represented in well 2 at the northern part of the study area (cross section A-A′, Fig. 7) and as lenses in wells 7, 2, 12, and 35 (cross section CC′, Fig. 11).

4.3 Hydraulic Conductivity

According to Eq. (22), the average of the calculated hydraulic conductivity of Mit Ghamr Formation (Fig. 12) ranges from 5.082 × 10−10 cm/s at well 35 in the northeast and increase towards the middle and the western parts of the area. The maximum value of the hydraulic conductivity is represented at well 19 (3.35 × 10−8) in the western direction and well 23 at the southeast (3.96 × 10−8).
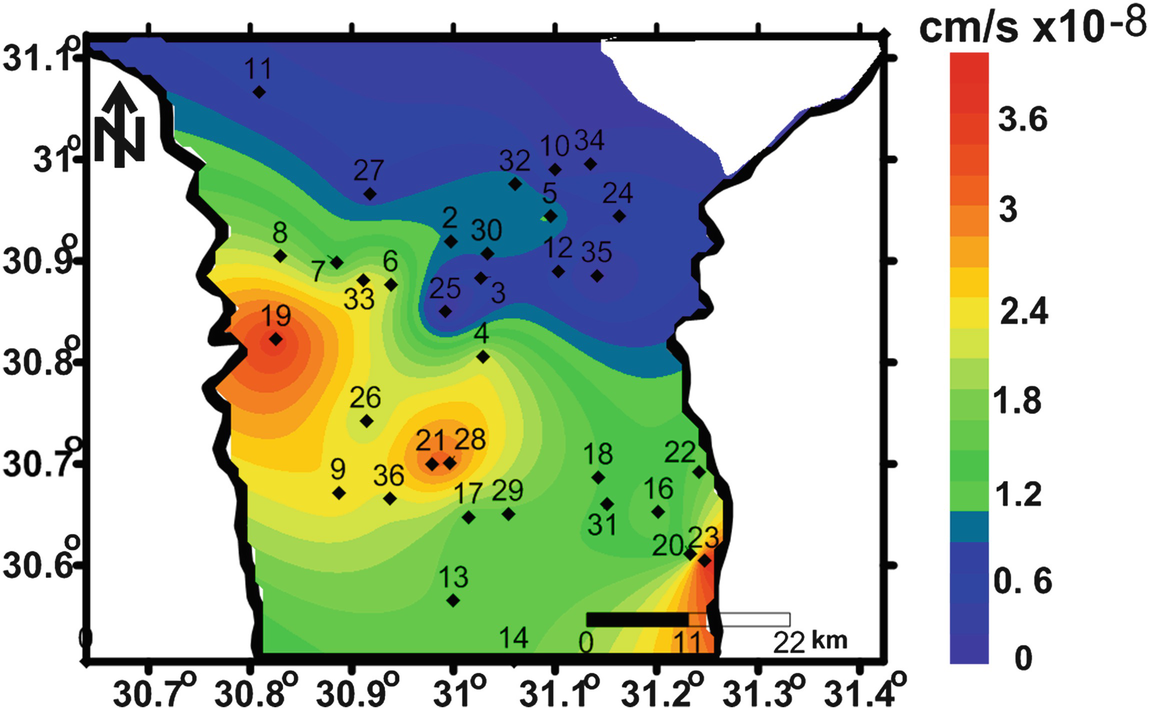
../images/437178_1_En_75_Chapter/437178_1_En_75_Fig12_HTML.png
Fig. 12

Spatial distributions of the calculated average values of the hydraulic conductivity of Mit Ghamr formation

Vertical distributions of the hydraulic conductivity showed variable ranges in vertical and horizontal directions. Very low values (<2 × 10−9 cm/s) are recognized mainly at the uppermost layer (Bilqas Formation) as shown in Fig. 13a–c. Low hydraulic conductivity values are also recognized in the northern and eastern parts of the cross sections AA′ and BB′, respectively. The hydraulic conductivity values increase toward the middle parts of the area between wells 4 and 7 (2.13 × 10−8 to 1.27 × 10−8 cm/s), and the highest values (1.93 × 10−8 cm/s) are recorded in well 8 to the west and the area between wells 9 and 17. Such horizontal and vertical change in the hydraulic conductivity could be related to the depositional system of the Nile Delta sediments.
../images/437178_1_En_75_Chapter/437178_1_En_75_Fig13_HTML.png
Fig. 13

Vertical distributions of the calculated values of the aquifer hydraulic conductivity along the cross sections AA′, BB′, and CC′

5 Conclusions

Electrical resistivity and gamma ray logs were used for 34 wells to identify the petrophysical, chemical, and hydrogeological characteristics of the Nile Delta aquifer due to its significant importance. The Nile Delta reservoir consists of two formations. The upper is the Bilqas Formation, which is of a clay nature and the lower formation is of a sandy nature and called Mit Ghamr Formation. According to the calculations used, the thickness of Bilqas Formation ranges from three meters in the southwest direction to 31 m in the northeast direction. The shale content ranges from 54 to 97%. The porosity ranges from 21 to 55% with an average of 24.71%. This layer has low permeability values ranged from 16 × 10−9 to 78 × 10−9 mD with an average of 30.71 × 10−9 mD. The calculations showed that the hydraulic conductivity of this layer is weak and less than 2 × 10−9 cm/s. The water salinity of this layer ranges from 200 mg to 1,600 mg/l. The increase in the thickness of this layer, the increase in porosity, the decrease in permeability and hydraulic conductivity, as well as the increase in the water salinity are to the north and northeast directions.

Mit Ghamr is the main aquifer in the region where its minimum value of the shale content is 4.5% and the maximum value is 22%. Lenses from the clay are scattered in different places and increasing in intensity in the northeastern direction of the region. Porosity ranges from a minimum of 19% to a maximum of 39%. The permeability recorded high values in this formation where it ranged from 0.1 × 10−2 to 8.7 × 10−2 mD. The calculations also showed that the hydraulic conductivity values for this formation ranged from 5.082 × 10−10 to 2.134 × 10−8 cm/s. In this layer, the increase in the shale content, the increase in porosity, decrease in the permeability and hydraulic conductivity, as well as the increase in salinity are to the north and northeast.

Acknowledgments

The authors are grateful to Tanta University for the financial support offered by the project number “TU-01-12-03” during the course of this paper.