Explanation
The test makers often set up percentage problems to reward those who do two important things. The first is to be careful about what number the percentage is applied to. The second is to remember to look for ways to simplify calculations.
This problem tells you that an increase of 25% raises the price of a car to $42,000. Be careful to apply that 25% increase to the unknown original price, not the $42,000. Decreasing $42,000 by 25% yields $31,500, which is the most common wrong answer. Avoid that one error, and your odds of getting the right answer go up dramatically.
If you set up the arithmetic the way the GMAT presents it, you get:
Original price × 1.25 = 42,000
This would have you dividing 42,000 by 1.25—a simple task for a calculator but a time-consuming one for a person. On the GMAT, you’ll often have more success by converting percentages
to fractions. An increase of 25% means an increase of
 , bringing the total up to
, bringing the total up to
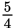 of the original:
of the original:
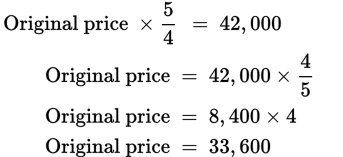
Choice (D) is correct.
More GMAT by the Numbers . . .

To see more questions with answer choice statistics, be sure to review the full-length CATs in your online resources.