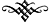
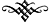
The method of first and ultimate ratios, for use in demonstrating what follows
Lemma 1
Quantities, and also ratios of quantities, which in aany finite timea constantly tend to equality, and which before the end of that time approach so close to one another that their difference is less than any given quantity, become ultimately equal.
If you deny this, blet them become ultimately unequal, andb let their ultimate difference be D. Then they cannot approach so close to equality that their difference is less than the given difference D, contrary to the hypothesis.
Lemma 2
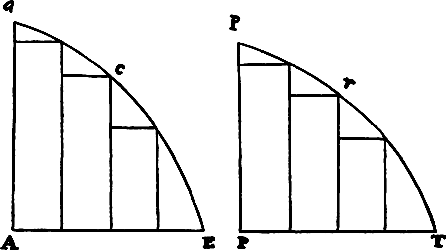
If in any figure AacE, comprehended by the straight lines Aa and AE and the curve acE, any number of parallelograms Ab, Bc, Cd, . . . are inscribed upon 
equal bases AB, BC, CD, . . . and have sides Bb, Cc, Dd, . . . parallel to the side Aa of the figure; and if the parallelograms aKbl, bLcm, cMdn, . . . are completed; if then the width of these parallelograms is diminished and their number increased indefinitely, I say that the ultimate ratios which the inscribed figure AKbLcMdD, the circumscribed figure AalbmcndoE, and the curvilinear figure AabcdE have to one another are ratios of equality.
For the difference of the inscribed and circumscribed figures is the sum of the parallelograms Kl, Lm, Mn, and Do, that is (because they all have equal bases), the rectangle having as base Kb (the base of one of them) and as altitude Aa (the sum of the altitudes), that is, the rectangle ABla. But this rectangle, because its width AB is diminished indefinitely, becomes less than any given rectangle. Therefore (by lem. 1) the inscribed figure and the circumscribed figure and, all the more, the intermediate curvilinear figure become ultimately equal. Q.E.D.
Lemma 3
The same ultimate ratios are also ratios of equality when the widths AB, BC, CD, . . . of the parallelograms are unequal and are all diminished indefinitely.
For let AF be equal to the greatest width, 
and let the parallelogram FAaf be completed. This parallelogram will be greater than the difference of the inscribed and the circumscribed figures; but if its width AF is diminished indefinitely, it will become less than any given rectangle. Q.E.D.
COROLLARY 1. Hence the ultimate sum of the vanishing parallelograms coincides with the curvilinear figure in its every part.
COROLLARY 2. And, all the more, the rectilinear figure that is comprehended by the chords of the vanishing arcs ab, be, cd, . . . coincides ultimately with the curvilinear figure.
COROLLARY 3. And it is the same for the circumscribed rectilinear figure that is comprehended by the tangents of those same arcs.
COROLLARY 4. And therefore these ultimate figures (with respect to their perimeters acE) are not rectilinear, but curvilinear limits of rectilinear figures.
Lemma 4
If in two figures AacE and PprT two series of parallelograms are inscribed (as above) and the number of parallelograms in both series is the same; and if when their widths are diminished indefinitely, the ultimate ratios of the parallelograms in one figure to the corresponding parallelograms in the other are the same; then I say that the two figures AacE and PprT are to each other in that same ratio.
For as the individual parallelograms in the one figure are to the corresponding individual parallelograms in the other, so (by composition [or componendo]) will the sum of all the parallelograms in the one become to the sum of all the parallelograms in the other, and so also the one figure to the other—the first figure, of course, being (by lem. 3) to the first sum, and the second figure to the second sum, in a ratio of equality. Q.E.D.
COROLLARY. Hence, if two quantities of any kind are divided in any way into the same number of parts, and these parts—when their number is increased and their size is diminished indefinitely—maintain a given ratio to one another, the first to the first, the second to the second, and so on in sequence, then the wholes will be to each other in the same given ratio. For if the parallelograms in the figures of this lemma are taken in the same proportion to one another as those parts, the sums of the parts will always be as the sums of the parallelograms; and therefore, when the number of parts and parallelograms is increased and their size diminished indefinitely, the sums of the parts will be in the ultimate ratio of a parallelogram in one figure to a corresponding parallelogram in the other, that is (by hypothesis), in the ultimate ratio of part to part.
Lemma 5
All the mutually corresponding sides—curvilinear as well as rectilinear—of similar figures are proportional, and the areas of such figures are as the squares of their sides.
Lemma 6
If any arc ACB, given in position, is subtended by the chord AB and at some point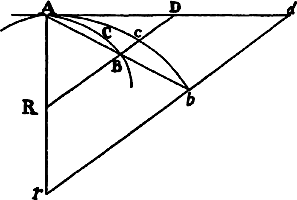 
A, in the middle of the continuous curvature, is touched by the straight line AD, produced in both directions, and if then points A and B approach each other and come together, I say that the angle BAD contained by the chord and the tangent will be indefinitely diminished and will ultimately vanish.
For aif that angle does not vanish, the angle contained by the arc ACB and the tangent AD will be equal to a rectilinear angle, and therefore the curvature at point A will not be continuous, contrary to the hypothesis.a
Lemma 7
With the same suppositions, I say that the ultimate ratios of the arc, the chord, and the tangent to one another are ratios of equality.
For while point B approaches point A, let AB and AD be understood always to be produced to the distant points b and d; and let bd be drawn parallel to secant BD. And let arc Acb be always similar to arc ACB. Then as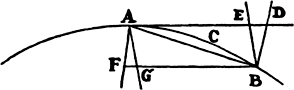 
points A and B come together, the angle dAb will vanish (by lem. 6), and thus the straight lines Ab and Ad (which are always finite) and the intermediate arc Acb will coincide and therefore will be equal. Hence, the straight lines AB and AD and the intermediate arc ACB (which are always proportional to the lines Ab and Ad and the arc Acb respectively) will also vanish and will have to one another an ultimate ratio of equality. Q.E.D.
COROLLARY 1. Hence, if BF is drawn through B parallel to the tangent and always cutting at F any straight line AF passing through A, then BF will ultimately have a ratio of equality to the vanishing arc ACB, because, if parallelogram AFBD is completed, BF always has a ratio of equality to AD.
COROLLARY 2. And if through B and A additional straight lines BE, BD, AF, and AG are drawn cutting the tangent AD and its parallel BF, the ultimate ratios of all the abscissas AD, AE, BF, and BG and of the chord and arc AB to one another will be ratios of equality.
COROLLARY 3. And therefore all these lines can be used for one another interchangeably in any argumentation concerning ultimate ratios.
Lemma 8
If the given straight lines AR and BR, together with the arc ACB, its chord AB, and the tangent AD, constitute three triangles RAB, RACB, and RAD, and if then points A and B approach each other, I say that the triangles as they vanish are similar in their ultimate form, and that their ultimate ratio is one of equality.
For while point B approaches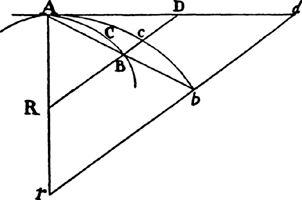 
point A, let AB, AD, and AR be understood always to be produced to the distant points b, d, and r, and rbd to be drawn parallel to RD; and let arc Acb be always similar to arc ACB. Then as points A and B come together, the angle bAd will vanish, and therefore the three triangles rAb, rAcb, and rAd, which are always finite, will coincide and on that account are similar and equal. Hence also RAB, RACB, and RAD, which will always be similar and proportional to these, will ultimately become similar and equal to one another. Q.E.D.
COROLLARY. And hence those triangles can be used for one another interchangeably in any argumentation concerning ultimate ratios.
Lemma 9
If the straight line AE and the curve ABC, both given in position, intersect each other at a given angle A, and if BD and CE are drawn as ordinates to the straight line AE at another given angle and meet the curve in B and C, and if then points B and C simultaneously approach point A, I say that the areas of the triangles ABD and ACE will ultimately be to each other as the squares of the sides.
For while points B and C approach point A, let AD be understood always to be produced to the distant points d and e, so that Ad and Ae are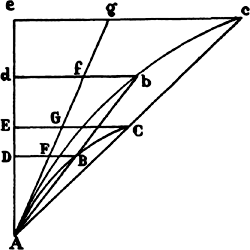 
proportional to AD and AE; and erect ordinates db and ec parallel to ordinates DB and EC and meeting AB and AC, produced, at b and c. Understand the curve Abc to be drawn similar to ABC, and the straight line Ag to be drawn touching both curves at A and cutting the ordinates DB, EC, db, and ec at F, G, f, and g. Then, with the length Ae remaining the same, let points B and C come together with point A; and as the angle cAg vanishes, the curvilinear areas Abd and Ace will coincide with the rectilinear areas Afd and Age, and thus (by lem. 5) will be in the squared ratio of the sides Ad and Ae. But areas ABD and ACE are always proportional to these areas, and sides AD and AE to these sides. Therefore areas ABD and ACE also are ultimately in the squared ratio of the sides AD and AE. Q.E.D.
Lemma 10
The spaces which a body describes when urged by any afinitea force, bwhether that force is determinate and immutable or is continually increased or continually decreased,b are at the very beginning of the motion in the squared ratio of the times.
Let the times be represented by lines AD and AE, and the generated velocities by ordinates DB and EC; then the spaces described by these velocities will be as the areas ABD and ACE described by these ordinates, that is, at the very beginning of the motion these spaces will be (by lem. 9) in the squared ratio of the times AD and AE. Q.E.D.
COROLLARY 1. And hence it is easily concluded that when bodies describe similar parts of similar figures in proportional times, the errors that are generated by any equal forces similarly applied to the bodies, and that are measured by the distances of the bodies from those points on the similar figures at which the same bodies would arrive in the same proportional times without these forces, are very nearly as the squares of the times in which they are generated.
COROLLARY 2. But the errors that are generated by proportional forces similarly applied to similar parts of similar figures are as the forces and the squares of the times jointly.
Lemma 11
In all curves having a finite curvature at the point of contact, the vanishing subtense of the angle of contact is ultimately in the squared ratio of the subtense of the conterminous arc.
CASE 1. Let AB be the arc, AD its tangent, BD the subtense of the angle of contact perpendicular to the tangent [angle BAD], and [the line] AB the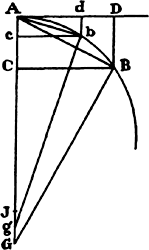 
subtense [i.e., the conterminous chord] of the arc [AB]. Erect BG and AG perpendicular to this subtense AB and tangent AD and meeting in G; then let points D, B, and G approach points d, b, and g, and let J be the intersection of lines BG and AG, which ultimately occurs when points D and B reach A. It is evident that the distance GJ can be less than any assigned distance. And (from the nature of the circles passing through points A, B, G and a, b, g) AB2 is equal to AG × BD, and Ab2 is equal to Ag × bd, and thus the ratio of AB2 to Ab2 is compounded of the ratios of AG to Ag and BD to bd. But since GJ can be taken as less than any assigned length, it can happen that the ratio of AG to Ag differs from the ratio of equality by less than any assigned difference, and thus that the ratio of AB2 to Ab2 differs from the ratio of BD to bd by less than any assigned difference. Therefore, by lem. 1, the ultimate ratio of AB2 to Ab2 is the same as the ultimate ratio of BD to bd. Q.E.D.
CASE 2. Now let BD be inclined to AD at any given angle, and the ultimate ratio of BD to bd will always be the same as before and thus the same as AB2 to Ab2. Q.E.D.
CASE 3. And even when angle D is not given, if the straight line BD converges to a given point or is drawn according to any other specification, still the angles D and d (constructed according to the specification common to both) will always tend to equality and will approach each other so closely that their difference will be less than any assigned quantity, and thus will ultimately be equal, by lem. 1; and therefore lines BD and bd are in the same ratio to each other as before. Q.E.D.
COROLLARY 1. Hence, since tangents AD and Ad, arcs AB and Ab, and their sines BC and bc become ultimately equal to chords AB and Ab, their squares will also be ultimately as the subtenses BD and bd.
COROLLARY 4. The rectilinear triangles ADB and 
Adb are ultimately in the cubed ratio of the sides AD and Ad, and in the sesquialteral ratio [i.e., as the 3/2 power] of the sides DB and db, inasmuch as these triangles are in a ratio compounded of the ratios of AD and DB to Ad and db. So also the triangles ABC and Abc are ultimately in the cubed ratio of the sides BC and bc. bThe ratio that I call sesquialteral is the halved of the tripled, namely, the one that is compounded of the simple and the halved.b
COROLLARY 5. And since DB and db are ultimately parallel and in the squared ratio of AD and Ad, the ultimate curvilinear areas ADB and Adb will be (from the nature of the parabola) two-thirds of the rectilinear triangles ADB and Adb; and the segments AB and Ab will be thirds of these same triangles. And hence these areas and segments will be in the cubed ratio of both of the tangents AD and Ad and of the chords AB and Ab and their arcs.
Scholium
But we suppose throughout that the angle of contact is neither infinitely greater nor infinitely less than the angles of contact that circles contain with their tangents, that is, that the curvature at point A is neither infinitely small nor infinitely great—in other words, that the distance AJ is of a finite magnitude. For DB can be taken proportional to AD3, in which case no circle can be drawn through point A between tangent AD and curve AB, and accordingly the angle of contact will be infinitely less than those of circles. And, similarly, if DB is made successively proportional to AD4, AD5, AD6, AD7, . . . , there will be a sequence of angles of contact going on to infinity, any succeeding one of which is infinitely less than the preceding one. And if DB is made successively proportional to AD2, AD3/2, AD4/3, AD5/4, AD6/5, AD7/6, . . . , there will be another infinite sequence of angles of contact, the first of which is of the same kind as those of circles, the second infinitely greater, and any succeeding one infinitely greater than the preceding one. Moreover, between any two of these angles a sequence of intermediate angles, going on to infinity in both directions, can be inserted, any succeeding one of which will be infinitely greater or smaller than the preceding one—as, for example, if between the terms AD2 and AD3 there were inserted the sequence AD13/6, AD11/5, AD9/4, AD7/3, AD5/2, AD8/3, AD11/4, AD14/5, AD17/6, . . . . And again, between any two angles of this sequence a new sequence of intermediate angles can be inserted, differing from one another by infinite intervals. And nature knows no limit.
What has been demonstrated concerning curved lines and the [plane] surfaces comprehended by them is easily applied to curved surfaces and their solid contents. In any case, I have presented these lemmas before the propositions in order to avoid the tedium of working out clengthyc proofs by reductio ad absurdum in the manner of the ancient geometers. Indeed, proofs are rendered more concise by the method of indivisibles. But since the hypothesis of indivisibles is dproblematicald and this method is therefore accounted less geometrical, I have preferred to make the proofs of what follows depend on the ultimate sums and ratios of vanishing quantities and the first sums and ratios of nascent quantities, that is, on the limits of such sums and ratios, and therefore to present proofs of those limits beforehand as briefly as I could. For the same result is obtained by these as by the method of indivisibles, and we shall be on safer ground using principles that have been proved. Accordingly, whenever in what follows I consider quantities as consisting of particles or whenever I use curved line-elements [or minute curved lines] in place of straight lines, I wish it always to be understood that I have in mind not indivisibles but evanescent divisibles, and not sums and ratios of definite parts but the limits of such sums and ratios, and that the force of such proofs always rests on the method of the preceding lemmas.
It may be objected that there is no such thing as an ultimate proportion of vanishing quantities, inasmuch as before vanishing the proportion is not ultimate, and after vanishing it does not exist at all. But by the same argument it could equally be contended that there is no ultimate velocity of a body reaching a certain place at which the motion ceases; for before the body arrives at this place, the velocity is not the ultimate velocity, and when it arrives there, there is no velocity at all. But the answer is easy: to understand the ultimate velocity as that with which a body is moving, neither before it arrives at its ultimate place and the motion ceases, nor after it has arrived there, but at the very instant when it arrives, that is, the very velocity with which the body arrives at its ultimate place and with which the motion ceases. And similarly the ultimate ratio of vanishing quantities is to be understood not as the ratio of quantities before they vanish or after they have vanished, but the ratio with which they vanish. Likewise, also, the first ratio of nascent quantities is the ratio with which they begin to exist [or come into being]. And the first and the ultimate sum is the sum with which they begin and cease to exist (or to be increased or decreased). There exists a limit which their velocity can attain at the end of the motion, but cannot exceed. This is their ultimate velocity. And it is the same for the limit of all quantities and proportions that come into being and cease existing. And since this limit is certain and definite, the determining of it is properly a geometrical problem. But everything that is geometrical is legitimately used in determining and demonstrating whatever else may be geometrical.
It can also be contended that if the ultimate ratios of vanishing quantities are given, their ultimate magnitudes will also be given; and thus every quantity will consist of indivisibles, contrary to what Euclid had proved concerning incommensurables in the tenth book of his Elements. But this objection is based on a false hypothesis. Those ultimate ratios with which quantities vanish are not actually ratios of ultimate quantities, but limits which the ratios of quantities decreasing without limit are continually approaching, and which they can approach so closely that their difference is less than any given quantity, but which they can never exceed and can never reach before the quantities are decreased indefinitely. This matter will be understood more clearly in the case of quantities that are indefinitely great. If two quantities whose difference is given are increased indefinitely, their ultimate ratio will be given, namely the ratio of equality, and yet the ultimate or maximal quantities of which this is the ratio will not on this account be given. Therefore, whenever, to make things easier to comprehend, I speak in what follows of quantities as minimally small or vanishing or ultimate, take care not to understand quantities that are determinate in magnitude, but always think of quantities that are to be decreased without limit.
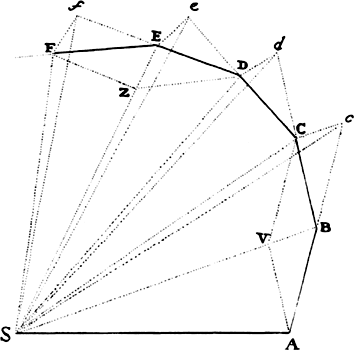
Proposition 1a, Theorem 1
The areas which bodies bmade to move in orbitsb describe by radii drawn to an unmoving center of forces lie in unmoving planes and are proportional to the times.
Let the time be divided into equal parts, and in the first part of the time let a body by its inherent force describe the straight line AB. In the second part of the time, if nothing hindered it, this body would (by law 1) go straight on to c, describing line Bc equal to AB, so that—when radii AS, BS, and cS were drawn to the center—the equal areas ASB and BSc would be described. But when the body comes to B, let a centripetal force act with a single but great impulse and make the body deviate from the straight line Bc and proceed in the straight line BC. Let cC be drawn parallel to BS and meet BC at C; then, when the second part of the time has been completed, the body (by corol. 1 of the laws) will be found at C in the same plane as triangle ASB. Join SC; and because SB and Cc are parallel, triangle SBC will be equal to triangle SBc and thus also to triangle SAB. By a similar argument, if the centripetal force acts successively at C, D, E, . . . , making the body in each of the individual particles of time describe the individual straight lines CD, DE, EF, . . . , all these lines will lie in the same plane; and triangle SCD will be equal to triangle SBC, SDE to SCD, and SEF to SDE. Therefore, in equal times equal areas are described in an unmoving plane; and by composition [or componendo], any sums SADS and SAFS of the areas are to each other as the times of description. Now let the number of triangles be increased and their width decreased indefinitely, and their ultimate perimeter ADF will (by lem. 3, corol. 4) be a curved line; and thus the centripetal force by which the body is continually drawn back from the tangent of this curve will act uninterruptedly, while any areas described, SADS and SAFS, which are always proportional to the times of description, will be proportional to those times in this case. Q.E.D.
Proposition 2, Theorem 2
Every body that moves in some curved line described in a plane and, by a radius drawn to a point, either unmoving or moving uniformly forward with a rectilinear motion, describes areas around that point proportional to the times, is urged by a centripetal force tending toward that same point.
CASE 1. For every body that moves in a curved line is deflected from a rectilinear course by some force acting upon it (by law 1). And that force by which the body is deflected from a rectilinear course and in equal times is made to describe, about an immobile point S, the equal minimally small triangles SAB, SBC, SCD, . . . , acts in place B along a line parallel to cC (by book 1, prop. 40, of the Elements, and law 2), that is, along the line BS; and in place C, the force acts along a line parallel to dD, that is, along the line SC, . . . . Therefore it always acts along lines tending toward that unmoving point S. Q.E.D.
CASE 2. And, by corol. 5 of the laws, it makes no difference whether the surface on which the body describes a curvilinear figure is at rest or whether it moves uniformly straight forward, together with the body, the figure described, and the point S.
aCOROLLARY 1. bIn nonresisting spaces or mediums, if the areas are not proportional to the times, the forces do not tend toward the point where the radii meet but deviate forward [or in consequentia] from it, that is, in the direction toward which the motion takes place, provided that the description of the areas is accelerated; but if it is retarded, they deviate backward [or in antecedentia, i.e., in a direction contrary to that in which the motion takes place].b
COROLLARY 2. cIn resisting mediums also, if the description of areas is accelerated, the directions of the forces deviate from the point where the radii meet in the direction toward which the motion takes place.a c
Scholium
A body can be urged by a centripetal force compounded of several forces. In this case the meaning of the proposition is that the force which is compounded of all the forces tends toward point S. Further, if some force acts continually along a line perpendicular to the surface described, it will cause the body to deviate from the plane of its motion, but it will neither increase nor decrease the quantity of the surface-area described and is therefore to be ignored in the compounding of forces.
Scholium
Since the uniform description of areas indicates the center toward which that force is directed by which a body is most affected and by which it is drawn away from rectilinear motion and kept in orbit, why should we not in what follows use uniform description of areas as a criterion for a center about which all orbital motion takes place in free spaces?
Proposition 4, Theorem 4
The centripetal forces of bodies that describe different circles with uniform motion tend toward the centers of those circles and are to one another as the squares of the arcs described in the same time divided by the radii of the circles.
aThese forces tend toward the centers of the circles by prop. 2 and prop. 1, corol. 2, and are to another as the versed sines of the arcs described in minimally small equal times, by prop. 1, corol. 4, that is, as the squares of those arcs divided by the diameters of the circles, by lem. 7; and therefore, since these arcs are as the arcs described in any equal times and the diameters are as their radii, the forces will be as the squares of any arcs described in the same time divided by the radii of the circles. Q.E.D.a
bCOROLLARY 1. cSince those arcs are as the velocities of the bodies, the centripetal forces will be in a ratio compounded of the squared ratio of the velocities directly and the simple ratio of the radii inversely.c
COROLLARY 2. dAnd since the periodic times are in a ratio compounded of the ratio of the radii directly and the ratio of the velocities inversely, the centripetal forces are in a ratio compounded of the ratio of the radii directly and the squared ratio of the periodic times inversely.d
COROLLARY 4. eIf both the periodic times and the velocities are as the square roots of the radii, the centripetal forces will be equal to one another; and conversely.e
COROLLARY 5. fIf the periodic times are as the radii, and therefore the velocities are equal, the centripetal forces will be inversely as the radii; and conversely.f
COROLLARY 6. gIf the periodic times are as the 3/2 powers of the radii, and therefore the velocities are inversely as the square roots of the radii, the centripetal forces will be inversely as the squares of the radii; and conversely.bg
Scholium
iThe case of corol. 6 holds for the heavenly bodies (as our compatriots Wren, Hooke, and Halley have also found out independently). Accordingly, I have decided that in what follows I shall deal more fully with questions relating to the centripetal forces that decrease as the squares of the distances from centers [i.e., centripetal forces that vary inversely as the squares of the distances].
Further, with the help of the preceding proposition and its corollaries the proportion of a centripetal force to any known force, such as that of gravity, may also be determined. jFor if a body revolves by the force of its gravity in a circle concentric with the earth, this gravity is its centripetal force. Moreover, by prop. 4, corol. 9, both the time of one revolution and the arc described in any given time are given from the descent of heavy bodies.j And by propositions of this sort Huygens in his excellent treatise On the Pendulum Clock compared the force of gravity with the centrifugal forces of revolving bodies.
Proposition 5, Problem 1
Given, in any places, the velocity with which a body describes a given curve when acted on by forces tending toward some common center, to find that center.
Let the curve so described be touched in three points P, Q, and R by three straight lines PT, TQV, and VR, meeting in T and V. Erect PA, QB, and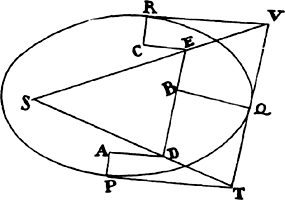 
RC perpendicular to the tangents and inversely proportional to the velocities of the body at the points P, Q, and R from which the perpendiculars are erected—that is, so that PA is to QB as the velocity at Q to the velocity at P, and QB to RC as the velocity at R to the velocity at Q. Through the ends A, B, and C of the perpendiculars draw AD, DBE, and EC at right angles to those perpendiculars, and let them meet in D and E; then TD and VE, when drawn and produced, will meet in the required center S.
For the perpendiculars dropped from center S to tangents PT and QT are (by prop. 1, corol. 1) inversely as the velocities of the body at points P and Q, and therefore, by the construction, as the perpendiculars AP and BQ directly, that is, as the perpendiculars dropped from point D to the tangents. Hence it is easily gathered that points S, D, and T are in one straight line. And, by a similar argument, the points S, E, and V are also in one straight line; and therefore the center S is at the point where the straight lines TD and VE meet. Q.E.D.
Proposition 6a, Theorem 5
bIf in a nonresisting space a body revolves in any orbit about an immobile center and describes any just-nascent arc in a minimally small time, and if the sagitta of the arc is understood to be drawn so as to bisect the chord and, when produced, to pass through the center of forces, the centripetal force in the middle of the arc will be as the sagitta directly and as the time twice [i.e., as the square of the time] inversely.
This proposition is also easily proved by lem. 10, corol. 4.
Proposition 7, Problem 2
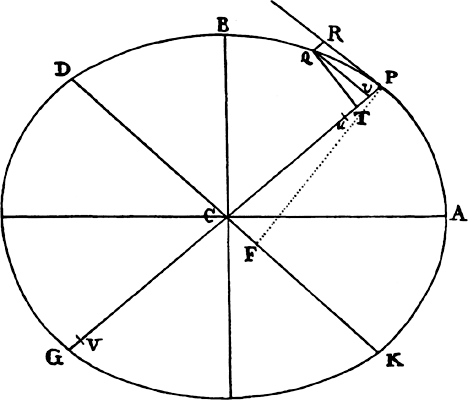
Let a body revolve in the circumference of a circle; it is required to find the law of the centripetal force tending toward any given point.
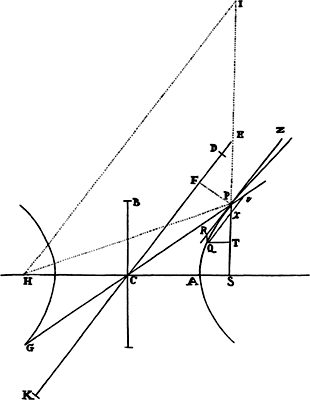
Let VQPA be the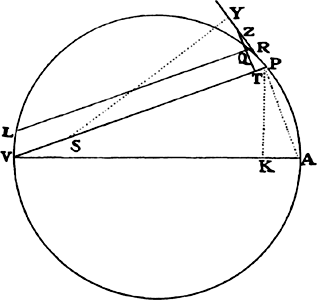 
circumference of the circle, S the given point toward which the force tends as to its center, P the body revolving in the circumference, Q the place to which it will move next, and PRZ the tangent of the circle at the previous place. Through point S draw chord PV; and when the diameter VA of the circle has been drawn, join AP; and to SP drop perpendicular QT, which when produced meets the tangent PR at Z; and finally through point Q draw LR parallel to SP and meeting both the circle at L and the tangent PZ at R. Then because the triangles ZQR, ZTP, and VPA are similar, RP2 (that is, QR × RL) will be to QT2 as AV2 to PV2. And therefore 
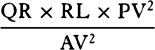is equal to QT2. Multiply these equals by 
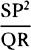and, the points P and Q coming together, write PV for RL. Thus 
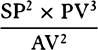will become equal to 
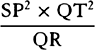. Therefore (by prop. 6, corols. 1 and 5), the centripetal force is inversely as 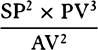, that is (because AV2 is given), inversely as the square of the distance or altitude SP and the cube of the chord PV jointly. Q.E.I.
Draw SY perpendicular to the tangent PR produced; then, because triangles SYP and VPA are similar, AV will be to PV as SP to SY, and thus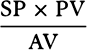 
will be equal to SY, and 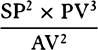will be equal to SY2 × PV. And therefore (by prop. 6, corols. 3 and 5), the centripetal force is inversely as 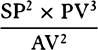, that is, because AV is given, inversely as SP2 × PV3. Q.E.I.
COROLLARY 1. Hence, if the given point S to which the centripetal force always tends is located in the circumference of this circle, say at V, the centripetal force will be inversely as the fifth power of the altitude SP.
COROLLARY 2. The force by which body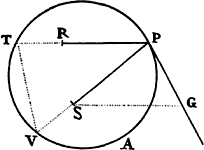 
P revolves in the circle APTV around the center of forces S is to the force by which the same body P can revolve in the same circle and in the same periodic time around any other center of forces R as RP2 × SP to the cube of the straight line SG, which is drawn from the first center of forces S to the tangent of the orbit PG and is parallel to the distance of the body from the second center of forces. For by the construction of this proposition the first force is to the second force as RP2 × PT3 to SP2 × PV3, that is, as SP × RP2 to 
, or (because the triangles PSG and TPV are similar) to SG3.
COROLLARY 3. The force by which body P revolves in any orbit around the center of forces S is to the force by which the same body P can revolve in the same orbit and in the same periodic time around any other center of forces R as the solid SP × RP2—contained under the distance of the body from the first center of forces S and the square of its distance from the second center of forces R—to the cube of the straight line SG, which is drawn from the first center of forces S to the tangent of the orbit PG and is parallel to the distance RP of the body from the second center of forces. For the forces in this orbit at any point of it P are the same as in a circle of the same curvature.
Proposition 8, Problem 3
Let a body move in the semicircle PQA; it is required to find the law of the centripetal force for this effect, when the centripetal force tends toward a point S so distant that all the lines PS and RS drawn to it can be considered parallel.
From the center C of the semicircle 
draw the semidiameter CA, intersecting those parallels perpendicularly at M and N, and join CP. Because triangles CPM, PZT, and RZQ are similar, CP2 is to PM2 as PR2 to QT2, and from the nature of a circle PR2 is equal to the rectangle QR × (RN + QN), or, the points P and Q coming together, to the rectangle QR × 2PM. Therefore, CP2 is to PM2 as QR × 2PM to QT2, and thus 
is equal to 
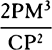, and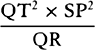 
is equal to 
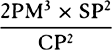. Therefore (by prop. 6, corols. 1 and 5), the centripetal force is inversely as 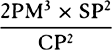, that is 
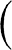neglecting the determinatea ratio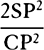 
, inversely as PM3. Q.E.I.
The same is easily gathered also from the preceding proposition.
Scholium
And by a not very different argument, a body will be found to move in an ellipse, or even in a hyperbola or a parabola, under the action of a centripetal force that is inversely as the cube of the ordinate tending toward an extremely distant center of forces.
Proposition 9, Problem 4
Let a body revolve in a spiral PQS intersecting all its radii SP, SQ, . . . , at a given angle; it is required to find the law of the centripetal force tending toward the center of the spiral.
Let the indefinitely small angle PSQ be given, and because all the angles are given, the species [i.e., the ratio of all the parts] of the figure SPRQT will be given. Therefore, the ratio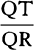 
is given, and 
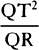is as QT, that is (because the species of the figure is given), as SP. Now change the angle PSQ in any way, and the straight line QR subtending the angle of contact QPR will be changed (by lem. 11) as the square of PR or QT. Therefore, 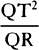will remain the same as before, that is, as SP. And therefore 
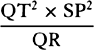is as SP3, and thus (by prop. 6, corols. 1 and 5) the centripetal force is inversely as the cube of the distance SP. Q.E.I.
The perpendicular SY dropped to the tangent, and the chord PV of the circle cutting the spiral concentrically, are to the distance SP in given ratios; and thus SP3 is as SY2 × PV, that is (by prop. 6, corols. 3 and 5), inversely as the centripetal force.
Lemma 12
All the parallelograms described about any conjugate diameters of a given ellipse or hyperbola are equal to one another.
This is evident from the Conics.
Proposition 10, Problem 5
Let a body revolve in an ellipse; it is required to find the law of the centripetal force tending toward the center of the ellipse.
Let CA and CB be the semiaxes of the ellipse, GP and DK other conjugate diameters, PF and QT perpendiculars to those diameters, Qv an ordinate to diameter GP; then, if parallelogram QvPR is completed, the rectangle Pv × vG will (from the Conicsa) be to Qv2 as PC2 to CD2, and (because triangles QvT and PCF are similar) Qv2 is to QT2 as PC2 to PF2, and, when these ratios are combined, the rectangle Pv × vG is to QT2 as PC2 to CD2 and PC2 to PF2; that is, vG is to 
as PC2 to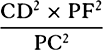 
. Write QR for Pv and (by lem. 12) BC × CA for CD × PF, and also (points P and Q coming together) 2PC for vG, and, multiplying the extremes and means together, 
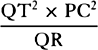will become equal to 
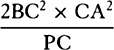. Therefore (by prop. 6, corol. 5), the centripetal force is as 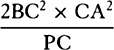inversely, that is (because 2BC2 × CA2 is given), as 
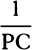inversely, that is, as the distance PC directly. Q.E.I.
On the straight line PG take a point u on the other side of point T, so that Tu is equal to Tv; then take uV such that it is to vG as DC2 is to PC2. And since (from the Conics) Qv2 is to Pv × vG as DC2 to PC2, Qv2 will be equal to Pv × uV. Add the rectangle uP × Pv to both sides, and the square of the chord of arc PQ will come out equal to the rectangle VP × Pv; and therefore a circle that touches the conic section at P and passes through point Q will also pass through point V. Let points P and Q come together, and the ratio of uV to vG, which is the same as the ratio of DC2 to PC2, will become the ratio of PV to PG or PV to 2PC; and therefore PV will be equal to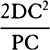 
. Accordingly, the force under the action of which body P revolves in the ellipse will (by prop. 6, corol. 3) be as 
inversely, that is (because 2DC2 × PF2 is given), as PC directly. Q.E.I.
COROLLARY 1. Therefore, the force is as the distance of the body from the center of the ellipse; and, conversely, if the force is as the distance, the body will move in an ellipse having its center in the center of forces, or perhaps it will move in a circle, into which an ellipse can be changed.
COROLLARY 2. And the periodic times of the revolutions made in all ellipses universally around the same center will be equal. For in similar ellipses those times are equal (by prop. 4, corols. 3 and 8), while in ellipses having a common major axis they are to one another as the total areas of the ellipses directly and the particles of the areas described in the same time inversely; that is, as the minor axes directly and the velocities of bodies in their principal vertices inversely; that is, as those minor axes directly and the ordinates to the same point of the common axis inversely; and therefore (because of the equality of the direct and inverse ratios) in the ratio of equality.
Scholium
If the center of the ellipse goes off to infinity, so that the ellipse turns into a parabola, the body will move in this parabola, and the force, now tending toward an infinitely distant center, will prove to be uniform. This is Galileo’s theorem. And if (by changing the inclination of the cutting plane to the cone) the parabolic section of the cone turns into a hyperbola, the body will move in the perimeter of the hyperbola, with the centripetal force turned into a centrifugal force. And just as in a circle or an ellipse, if the forces tend toward a figure’s center located in the abscissa, and if the ordinates are increased or decreased in any given ratio or even if the angle of the inclination of the ordinates to the abscissa is changed, these forces are always increased or decreased in the ratio of the distances from the center, provided that the periodic times remain equal; so also in all figures universally, if the ordinates are increased or decreased in any given ratio or the angle of inclination of the ordinates is changed in any way while the periodic time remains the same, the forces tending toward any center located in the abscissa are, for each individual ordinate, increased or decreased in the ratio of the distances from the center.
The motion of bodies in eccentric conic sections
Proposition 11a Problem 6
Let a body revolve in an ellipse; it is required to find the law of the centripetal force tending toward a focus of the ellipse.
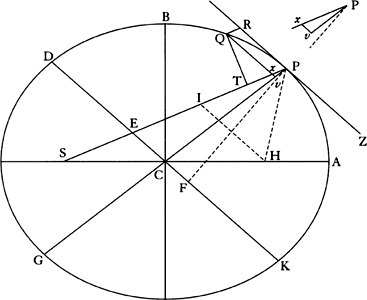
Let S be a focus of the ellipse. Draw SP cutting both the diameter DK of the ellipse in E and the ordinate Qv in x, and complete the parallelogram QxPR. It is evident that EP is equal to the semiaxis major AC because when line HI is drawn parallel to EC from the other focus H of the ellipse, ES and EI are equal because CS and CH are equal; so that EP is the half-sum of PS and PI, that is (because HI and PR are parallel and angles IPR and HPZ are equal), the half-sum of PS and PH (which taken together equal the whole axis 2AC). Drop QT perpendicular to SP, and if L denotes the principal latus rectum of the ellipse 
, L × QR will be to L × Pv as QR to Pv, that is, as PE or AC to PC; and L × Pv will be to Gv × vP as L to Gv; andb Gv × vP will be to Qv2 as PC2 to CD2; and (by lem. 7, corol. 2) the ratio of Qv2 to Qx2, with the points Q and P coming together, is the ratio of equality; and Qx2 or Qv2 is to QT2 as EP2 to PF2, that is, as CA2 to PF2 or (by lem. 12) as CD2 to CB2. And when all these ratios are combined, L × QR will be to QT2 as AC × L × PC2 × CD2, or as 2CB2 × PC2 × CD2 to PC × Gv × CD2 × CB2, or as 2PC to Gv. But with the points Q and P coming together, 2PC and Gv are equal. Therefore, L × QR and QT2, which are proportional to these, are also equal. Multiply these equals by 
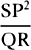, and L × SP2 will become equal to 
. Therefore (by prop. 6, corols. 1 and 5) the centripetal force is inversely as L × SP2, that is, inversely as the square of the distance SP. Q.E.I.
The force which tends toward the center of the ellipse, and by which body P can revolve in that ellipse, is (by prop. 10, corol. 1) as the distance CP of the body from the center C of the ellipse; hence, if CE is drawn parallel to the tangent PR of the ellipse and if CE and PS meet at E, then the force by which the same body P can revolve around any other point S of the ellipse will (by prop. 7, corol. 3) be as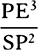 
; that is, if point S is a focus of the ellipse, and therefore PE is given, this force will be inversely as SP2. Q.E.I.
This solution could be extended to the parabola and the hyperbola as concisely as in prop. 10, but because of the importance of this problem and its use in what follows, it will not be too troublesome to confirm each of these other cases by a separate demonstration.
Proposition 12, Problem 7
Let a body move in a hyperbola; it is required to find the law of the centripetal force tending toward the focus of the figure.
Let CA and CB be the semiaxes of the hyperbola, PG and KD other conjugate diameters, PF a perpendicular to the diameter KD, and Qv an ordinate to the diameter GP. Draw SP cutting diameter DK in E and ordinate Qv in x, and complete the parallelogram QRPx. It is evident that EP is equal to the transverse semiaxis AC, because when line HI is drawn parallel to EC from the other focus H of the hyperbola, ES and EI are equal because CS and CH are equal; so that EP is the half-difference of PS and PI, that is (because IH and PR are parallel and the angles IPR and HPZ are equal), of PS and PH, the difference of which equals the whole axis 2AC. Drop QT perpendicular to SP. Then, if L denotes the principal latus rectum of the hyperbola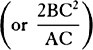 
, L × QR will be to L × Pv as QR to Pv, or Px to Pv, that is (because the triangles Pxv and PEC are similar), as PE to PC, or AC to PC. L × Pv will also be to Gv × Pv as L to Gv; and (from the nature of conics) the rectangle Gv × vP will be to Qv2 as PC2 to CD2; and (by lem. 7, corol. 2) the ratio of Qv2 to Qx2, the points Q and P coming together, comes to be the ratio of equality; and Qx2 or Qv2 is to AT2 as EP2 to PF2, that is, as CA2 to PF2, or (by lem. 12) as CD2 to CB2; and if all these ratios are combined, L × QR will be to QT2 as AC × L × PC2 × CD2 or 2CB2 × PC2 × CD2 to PC × Gv × CD2 × CB2, or as 2PC to Gv. But, the points P and Q coming together, 2PC and Gv are equal. Therefore, L × QR and QT2, which are proportional to these, are also equal. Multiply these equals by 
, and L × SP2 will become equal to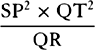 
. Therefore (by prop. 6, corols. 1 and 5), the centripetal force is inversely as L × SP2, that is, inversely as the square of the distance SP. Q.E.I.
Find the force that tends from the center C of the hyperbola. This will come out proportional to the distance CP. And hence (by prop. 7, corol. 3) the force tending toward the focus S will be as 
, that is, because PE is given, inversely as SP2. Q.E.I.
It is shown in the same way that if this centripetal force is turned into a centrifugal force, a body will move in the opposite branch of the hyperbola.
Lemma 13
In a parabola the latus rectum belonging to any vertex is four times the distance of that vertex from the focus of the figure.
This is evident from the Conics.
Lemma 14
A perpendicular dropped from the focus of a parabola to its tangent is a mean proportional between the distance of the focus from the point of contact and its distance from the principal vertex of the figure.
For let AP be the parabola, S its focus, A the principal vertex, P the point of contact, PO an ordinate to the principal diameter, PM a tangent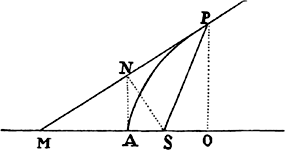 
meeting the principal diameter in M, and SN a perpendicular line from the focus to the tangent. Join AN, and because MS and SP, MN and NP, and MA and AO are equal, the straight lines AN and OP will be parallel; and hence triangle SAN will be right-angled at A and similar to the equal triangles SNM and SNP; therefore, PS is to SN as SN to SA. Q.E.D.
COROLLARY 1. PS2 is to SN2 as PS to SA.
COROLLARY 2. And because SA is given, SN2 is as PS.
COROLLARY 3. And the point where any tangent PM meets the straight line SN, which is drawn from the focus perpendicular to that tangent, occurs in the straight line AN, which touches the parabola in the principal vertex.
Proposition 13, Problem 8
Let a body move in the perimeter of a parabola; it is required to find the law of the centripetal force tending toward a focus of the figure.
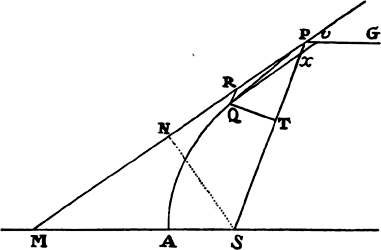
Let the construction be the same as in lem. 14, and let P be the body in the perimeter of the parabola; from the place Q into which the body moves next, draw QR parallel and QT perpendicular to SP and draw Qv parallel to the tangent and meeting both the diameter PG in v and the distance SP in x. Now, because triangles Pxv and SPM are similar and the sides SM and SP of the one are equal, the sides Px or QR and Pv of the other are equal. But from the Conics the square of the ordinate Qv is equal to the rectangle contained by the latus rectum and the segment Pv of the diameter, that is (by lem. 13), equal to the rectangle 4PS × Pv, or 4PS × QR, and, the points P and Q coming together, the ratio of Qv to Qx (by lem. 7, corol. 2) becomes the ratio of equality. Therefore, in this case Qx2 is equal to the rectangle 4PS × QR. Moreover (because triangles QxT and SPN are similar), Qx2 is to QT2 as PS2 to SN2, that is (by lem. 14, corol. 1), as PS to SA, that is, as 4PS × QR to 4SA × QR, and hence (by Euclid’s Elements, book 5, prop. 9) QT2 and 4SA × QR are equal. Multiply these equals by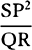 
, and 
will become equal to SP2 × 4SA; and therefore (by prop. 6, corols. 1 and 5) the centripetal force is inversely as SP2 × 4SA, that is, because 4SA is given, inversely as the square of the distance SP. Q.E.I.
COROLLARY 1. From the last three propositions it follows that if any body P departs from the place P along any straight line PR with any velocity whatever and is at the same time acted upon by a centripetal force that is inversely proportional to the square of the distance of places from the center, this body will move in some one of the conics having a focus in the center of forces; and conversely. For if the focus and the point of contact and the position of the tangent are given, a conic can be described that will have a given curvature at that point. But the curvature is given from the given centripetal force and velocity of the body; and two different orbits touching each other cannot be described with the same centripetal force and the same velocity.
COROLLARY 2. If the velocity with which the body departs from its place P is such that the line-element PR can be described by it in some minimally small particle of time, and if the centripetal force is able to move the same body through space QR in that same time, this body will move in some conic whose principal latus rectum is the quantity 
which ultimately results when the line-elements PR and QR are diminished indefinitely. In these corollaries I include the circle along with the ellipse, but not for the case where the body descends straight down to a center.
Proposition 14, Theorem 6
If several bodies revolve about a common center and the centripetal force is inversely as the square of the distance of places from the center, I say that the principal latera recta of the orbits are as the squares of the areas which the bodies describe in the same time by radii drawn to the center.
For (by prop. 13, corol. 2) the latus rectum L is equal to the 
quantity that results ultimately when points P and Q come together. But the minimally small line QR is in a given time as the generating centripetal force, that is (by hypothesis), inversely as SP2. Therefore, is as QT2 × SP2, that is, the latus rectum L is as the square of the area QT × SP. Q.E.D.
COROLLARY. Hence the total area of the ellipse and, proportional to it, the rectangle contained by the axes is as the square root of the latus rectum and as the periodic time. For the total area is as the area QT × SP, which is described in a given time, multiplied by the periodic time.
Proposition 15, Theorem 7
Under the same suppositions as in prop. 14, I say that the squares of the periodic times in ellipses are as the cubes of the major axes.
For the minor axis is a mean proportional between the major axis and the latus rectum, and thus the rectangle contained by the axes is as the square root of the latus rectum and as the 3/2 power of the major axis. But this rectangle (by prop. 14, corol.) is as the square root of the latus rectum and as the periodic time. Take away from both sides [i.e., divide through by] the square root of the latus rectum, and the result will be that the squares of the periodic times are as the cubes of the major axes. Q.E.D.
COROLLARY. Therefore the periodic times in ellipses are the same as in circles whose diameters are equal to the major axes of the ellipses.
Proposition 16, Theorem 8
Under the same suppositions as in prop. 15, if straight lines are drawn to the bodies in such a way as to touch the orbits in the places where the bodies are located, and if perpendiculars are dropped from the common focus to these tangents, I say that the velocities of the bodies are inversely as the perpendiculars and directly as the square roots of the principal latera recta.
From focus S to tangent PR drop 
perpendicular SY, and the velocity of body P will be inversely as the square root of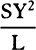 
. For this velocity is as the minimally small arc PQ described in a given particle of time, that is (by lem. 7), as the tangent PR, that is—because the proportion of PR to QT is as SP to SY—as 
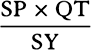, or as SY inversely and SP × QT directly; and SP × QT is as the area described in the given time, that is (by prop. 14), as the square root of the latus rectum. Q.E.D.
COROLLARY 1. The principal latera recta are as the squares of the perpendiculars and as the squares of the velocities.
COROLLARY 2. The velocities of bodies at their greatest and least distances from the common focus are inversely as the distances and directly as the square roots of the principal latera recta. For the perpendiculars are now the distances themselves.
COROLLARY 3. And thus the velocity in a conic, at the greatest or least distance from the focus, is to the velocity with which the body would move in a circle, at the same distance from the center, as the square root of the principal latus rectum is to the square root of twice that distance.
COROLLARY 4. The velocities of bodies revolving in ellipses are, at their mean distances from the common focus, the same as those of bodies revolving in circles at the same distances, that is (by prop. 4, corol. 6), inversely as the square roots of the distances. For the perpendiculars now coincide with the semiaxes minor, and these are as mean proportionals between the distances and the latera recta. Compound this ratio [of the semiaxes] inversely with the square root of the ratio of the latera recta directly, and it will become the square root of the ratio of the distances inversely.
COROLLARY 5. In the same figure, or even in different figures whose principal latera recta are equal, the velocity of a body is inversely as the perpendicular dropped from the focus to the tangent.
COROLLARY 6. In a parabola the velocity is inversely as the square root of the distance of the body from the focus of the figure; in an ellipse the velocity varies in a ratio that is greater than this, and in a hyperbola in a ratio that is less. For (by lem. 14, corol. 2) the perpendicular dropped from the focus to the tangent of a parabola is as the square root of that distance. In a hyperbola the perpendicular is smaller, and in an ellipse greater, than in this ratio.
COROLLARY 7. In a parabola the velocity of a body at any distance from the focus is to the velocity of a body revolving in a circle at the same distance from the center as the square root of the ratio of 2 to 1; in an ellipse it is smaller and in a hyperbola greater than in this ratio. For by corol. 2 of this proposition the velocity in the vertex of a parabola is in this ratio, and—by corol. 6 of this proposition and by prop. 4, corol. 6—the same proportion is kept at all distances. Hence, also, in a parabola the velocity everywhere is equal to the velocity of a body revolving in a circle at half the distance; in an ellipse it is smaller and in a hyperbola greater.
COROLLARY 8. The velocity of a body revolving in any conic is to the velocity of a body revolving in a circle at a distance of half the principal latus rectum of the conic as that distance is to the perpendicular dropped from the focus to the tangent of the conic. This is evident by corol. 5.
COROLLARY 9. Hence, since (by prop. 4, corol. 6) the velocity of a body revolving in this circle is to the velocity of a body revolving in any other circle inversely in the ratio of the square roots of the distances, it follows from the equality of the ratios [or ex aequo] that the velocity of a body revolving in a conic will have the same ratio to the velocity of a body revolving in a circle at the same distance that a mean proportional between that common distance and half of the principal latus rectum of the conic has to the perpendicular dropped from the common focus to the tangent of the conic.
Proposition 17, Problem 9
Supposing that the centripetal force is inversely proportional to the square of the distance of places from the center and that the absolute quantity of this force is known, it is required to find the line which a body describes when going forth from a given place with a given velocity along a given straight line.
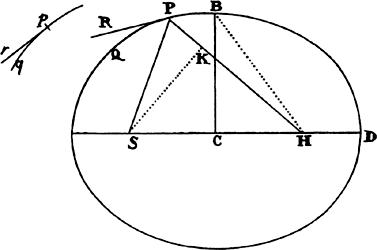
Let the centripetal force tending toward a point S be such that a body p revolves by its action in any given orbit pq, and let its velocity in the place p be found out. Let body P go forth from place P along line PR with a given velocity and thereupon be deflected from that line into a conic PQ under the compulsion of the centripetal force. Therefore the straight line PR will touch this conic at P. Let some straight line pr likewise touch the orbit pq at p, and if perpendiculars are understood to be dropped from S to these tangents, the principal latus rectum of the conic will (by prop. 16, corol. 1) be to the principal latus rectum of the orbit in a ratio compounded of the squares of the perpendiculars and the squares of the velocities and thus is given. Let L be the latus rectum of the conic. The focus S of the conic is also given. Let angle RPH be the complement of angle RPS to two right angles [i.e., the supplement of angle RPS]; then the line PH, on which the other focus H is located, will be given in position. Drop the perpendicular SK to PH and understand the conjugate semiaxis BC to be erected; then SP2 − 2KP × PH + PH2 = SH2 = 4CH2 = 4BH2 − 4BC2 = (SP + PH)2 − L × (SP + PH) = SP2 + 2SP × PH + PH2 − L × (SP + PH). Add to each side 2(KP × PH) − SP2 − PH2 + L × (SP + PH), and L × (SP + PH) will become = 2(SP × PH) + 2(KP × PH), or SP + PH will be to PH as 2SP + 2KP to L. Hence PH is given in length as well as in position. Specifically, if the velocity of the body at P is such that the latus rectum L is less than 2SP + 2KP, PH will lie on the same side of the tangent PR as the line PS; and thus the figure will be an ellipse and will be given from the given foci S and H and the given principal axis SP + PH. But if the velocity of the body is so great that the latus rectum L is equal to 2SP + 2KP, the length PH will be infinite; and accordingly the figure will be a parabola having its axis SH parallel to the line PK, and hence will be given. But if the body goes forth from its place P with a still greater velocity, the length PH will have to be taken on the other side of the tangent; and thus, since the tangent goes between the foci, the figure will be a hyperbola having its principal axis equal to the difference of the lines SP and PH, and hence will be given. For if the body in these cases revolves in a conic thus found, it has been demonstrated in props. 11, 12, and 13 that the centripetal force will be inversely as the square of the distance of the body from the center of forces S; and thus the line PQ is correctly determined, which a body will describe under the action of such a force, when it goes forth from a given place P with a given velocity along a straight line PR given in position. Q.E.F.
COROLLARY 1. Hence in every conic, given the principal vertex D, the latus rectum L, and a focus S, the other focus H is given when DH is taken to DS as the latus rectum is to the difference between the latus rectum and 4DS. For the proportion SP + PH to PH as 2SP + 2KP to L in the case of this corollary becomes DS + DH to DH as 4DS to L and, by separation [or dividendo], becomes DS to DH as 4DS − L to L.
COROLLARY 2. Hence, given the velocity of a body in the principal vertex D, the orbit will be found expeditiously, namely, by taking its latus rectum to twice the distance DS as the square of the ratio of this given velocity to the velocity of a body revolving in a circle at a distance DS (by prop. 16, corol. 3), and then taking DH to DS as the latus rectum to the difference between the latus rectum and 4DS.
COROLLARY 3. Hence also, if a body moves in any conic whatever and is forced out of its orbit by any impulse, the orbit in which it will afterward pursue its course can be found. For by compounding the body’s own motion with that motion which the impulse alone would generate, there will be found the motion with which the body will go forth from the given place of impulse along a straight line given in position.
COROLLARY 4. And if the body is continually perturbed by some force impressed from outside, its trajectory can be determined very nearly, by noting the changes which the force introduces at certain points and estimating from the order of the sequence the continual changes at intermediate places.a
Scholium
If a body P, under the action of a centripetal force tending toward any given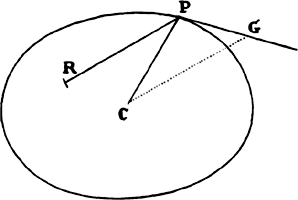 
point R, moves in the perimeter of any given conic whatever, whose center is C, and the law of the centripetal force is required, let CG be drawn parallel to the radius RP and meeting the tangent PG of the orbit at G; then the force (by prop. 10, corol. 1 and schol.; and prop. 7, corol. 3) will be as 
.
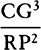To find elliptical, parabolic, and hyperbolic orbits, given a focus
Lemma 15
If from the two foci S and H of any ellipse or hyperbola two straight lines SV and HV are inclined to any third point V, one of the lines HV being equal to the principal axis of the figure, that is, to the axis on which the foci lie, and the other line SV being bisected in T by TR perpendicular to it, then the perpendicular TR will touch the conic at some point; and conversely, if TR touches the conic, HV will be equal to the principal axis of the figure.
For let the perpendicular TR cut the straight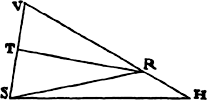 
line HV (produced, if need be) in R; and join SR. Because TS and TV are equal, the straight lines SR and VR and the angles TRS and TRV will be equal. Hence the point R will be on the conic, and the perpendicular TR will touch that conic, and conversely. Q.E.D.
Proposition 18, Problem 10
Given a focus and the principal axes, to describe elliptical and hyperbolic trajectories that will pass through given points and will touch straight lines given in position.
Let S be the common focus of the figures, AB the length of the principal axis of any trajectory, P a point through which the trajectory ought to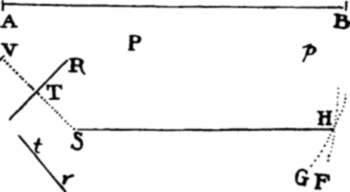 
pass, and TR a straight line which it ought to touch. Describe the circle HG with P as center and AB − SP as radius if the orbit is an ellipse, or AB + SP if it is a hyperbola. Drop the perpendicular ST to the tangent TR and produce ST to V so that TV is equal to ST, and with center V and radius AB describe the circle FH. By this method, whether two points P and p are given, or two tangents TR and tr, or a point P and a tangent TR, two circles are to be described. Let H be their common intersection, and with foci S and H and the given axis, describe the trajectory. I say that the problem has been solved. For the trajectory described (because PH + SP in an ellipse, or PH − SP in a hyperbola, is equal to the axis) will pass through point P and (by lem. 15) will touch the straight line TR. And by the same argument, this trajectory will pass through the two points P and p or will touch the two straight lines TR and tr. Q.E.F.
Proposition 19, Problem 11
To describe about a given focus a parabolic trajectory that will pass through given points and will touch straight lines given in position.
Let S be the focus, P a given point, and TR a tangent of the trajectory to be described. With center P and radius PS describe the circle FG. Drop 
the perpendicular ST from the focus to the tangent and produce ST to V, so that TV is equal to ST. In the same manner, if a second point p is given, a second circle fg is to be described; or if a second tangent tr is given, or a second point v is to be found, then the straight line IF is to be drawn touching the two circles FG and fg if the two points P and p are given, or passing through the two points V and v if the two tangents TR and tr are given, or touching the circle FG and passing through the point V if the point P and tangent TR are given. To FI drop the perpendicular SI, and bisect it in K; and with axis SK and principal vertex K describe a parabola. I say that the problem has been solved. For, because SK and IK are equal, and SP and FP are equal, the parabola will pass through point P; and (by lem. 14, corol. 3) because ST and TV are equal and the angle STR is a right angle, the parabola will touch the straight line TR. Q.E.F.
Proposition 20, Problem 12
To describe about a given focus any trajectory, given in species [i.e., of given eccentricity], that will pass through given points and will touch straight lines given in position.
CASE 1. Given a focus S, let it be required to describe a trajectory ABC through two points B and C. Since the trajectory is given in species, the 
ratio of the principal axis to the distance between the foci will be given. Take KB to BS in this ratio and also LC to CS. With centers B and C and radii BK and CL, describe two circles, and drop the perpendicular SG to the straight line KL, which touches those circles in K and L, and cut SG in A and a so that GA is to AS, and Ga to aS, as KB is to BS; and describe a trajectory with axis Aa and vertices A and a. I say that the problem has been solved. For let H be the other focus of the figure described, and since GA is to AS as Ga to aS, then by separation [or dividendo] Ga − GA or Aa to aS − AS or SH will be in the same ratio and thus in the ratio which the principal axis of the figure that was to be described has to the distance between its foci; and therefore the figure described is of the same species as the one that was to be described. And since KB to BS and LC to CS are in the same ratio, this figure will pass through the points B and C, as is manifest from the Conics.
CASE 2. Given a focus S, let it be required to describe a trajectory which somewhere touches the two straight lines TR and tr. Drop the perpendiculars 
ST and St from the focus to the tangents and produce ST and St to V and v, so that TV and tv are equal to TS and tS. Bisect Vv in O, and erect the indefinite perpendicular OH, and cut the straight line VS, indefinitely produced, in K and k, so that VK is to KS and Vk to kS as the principal axis of the trajectory to be described is to the distance between the foci. On the diameter Kk describe a circle cutting OH in H; and with foci S and H and a principal axis equal to VH, describe a trajectory. I say that the problem has been solved. For bisect Kk in X, and draw HX, HS, HV, and Hv. Since VK is to KS as Vk to kS and, by composition [or componendo], as VK + Vk to KS + kS and, by separation [or dividendo], as Vk − VK to kS − KS, that is, as 2VX to 2KX and 2KX to 2SX and thus as VX to HX and HX to SX, the triangles VXH and HXS will be similar, and therefore VH will be to SH as VX to XH and thus as VK to KS. Therefore the principal axis VH of the trajectory which has been described has the same ratio to the distance SH between its foci as the principal axis of the trajectory to be described has to the distance between its foci and is therefore of the same species. Besides, since VH and vH are equal to the principal axis and since VS and vS are perpendicularly bisected by the straight lines TR and tr, it is clear (from lem. 15) that these straight lines touch the trajectory described. Q.E.F.
CASE 3. Given a focus S, let it be required to describe a trajectory which will touch the straight line TR in a given point R. Drop the perpendicular 
ST to the straight line TR and produce ST to V so that TV is equal to ST. Join VR and cut the straight line VS, indefinitely produced, in K and k so that VK is to SK and Vk to Sk as the principal axis of the ellipse to be described is to the distance between the foci; and after describing a circle on the diameter Kk, cut the straight line VR, produced, in H, and with foci S and H and a principal axis equal to the straight line VH, describe a trajectory. I say that the problem has been solved. For, from what has been demonstrated in case 2, it is evident that VH is to SH as VK to SK and thus as the principal axis of the trajectory which was to be described to the distance between its foci, and therefore the trajectory which was described is of the same species as the one which was to be described, while it is evident from the Conics that the straight line TR by which the angle VRS is bisected touches the trajectory at point R. Q.E.F.
CASE 4. About a focus S let it be now required to describe a trajectory APB which touches the straight line TR and passes through any point P outside the given tangent and which is similar to the figure apb described with principal axis ab and foci s and h. Drop the perpendicular ST to the tangent TR and produce ST to V so that TV is equal to ST. Next make the angles hsq and shq equal to the angles VSP and SVP; and with center q and a radius that is to ab as SP to VS, describe a circle cutting the figure apb in p. Join sp and draw SH such that it is to sh as SP is to sp and makes the angle PSH equal to the angle psh and the angle VSH equal to the angle psq. Finally with foci S and H and with principal axis AB equaling the distance VH, describe a conic. I say that the problem has been solved. For if SV is drawn such that it is to sp as sh is to sq, and makes the angle vsp equal to the angle hsq and the angle vsh equal to the angle psq, the triangles svh and spq will be similar, and therefore vh will be to pq as sh is to sq, that is (because the triangles VSP and bsq are similar), as VS is to SP or ab to pq. Therefore vh and ab are equal. Furthermore, because the triangles VSH and vsh are similar, VH is to SH as vh to sh; that is, the axis of the conic just described is to the distance between its foci as the axis ab to the distance sh between the foci; and therefore the figure just described is similar to the figure apb. But because the triangle PSH is similar to the triangle psh, this figure passes through point P; and since VH is equal to the axis of this figure and VS is bisected perpendicularly by the straight line TR, the figure touches the straight line TR. Q.E.F.
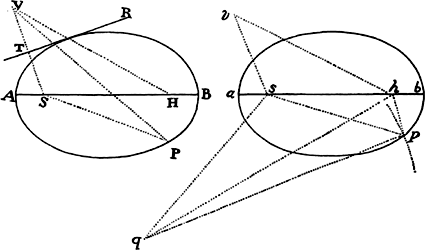
Lemma 16
From three given points to draw three slanted straight lines to a fourth point, which is not given, when the differences between the lines either are given or are nil.
CASE 1. Let the given points be A, B,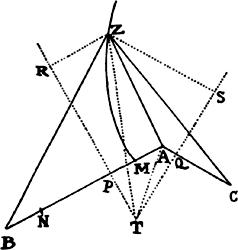 
and C, and let the fourth point be Z, which it is required to find; because of the given difference of the lines AZ and BZ, point Z will be located in a hyperbola whose foci are A and B and whose principal axis is the given difference. Let the axis be MN. Take PM to MA as MN is to AB, and let PR be erected perpendicular to AB and let ZR be dropped perpendicular to PR; then, from the nature of this hyperbola, ZR will be to AZ as MN is to AB. By a similar process, point Z will be located in another hyperbola, whose foci are A and C and whose principal axis is the difference between AZ and CZ; and QS can be drawn perpendicular to AC, whereupon, if the normal ZS is dropped to QS from any point Z of this hyperbola, ZS will be to AZ as the difference between AZ and CZ is to AC. Therefore the ratios of ZR and ZS to AZ are given, and consequently the ratio of ZR and ZS to each other is given; and thus if the straight lines RP and SQ meet in T, and TZ and TA are drawn, the figure TRZS will be given in species, and the straight line TZ, in which point Z is somewhere located, will be given in position. The straight line TA will also be given, as will also the angle ATZ; and because the ratios of AZ and TZ to ZS are given, their ratio to each other will be given; and hence the triangle ATZ, whose vertex is the point Z, will be given. Q.E.I.
CASE 2. If two of the three lines, say AZ and BZ, are equal, draw the straight line TZ in such a way that it bisects the straight line AB; then find the triangle ATZ as above.
CASE 3. If all three lines are equal, point Z will be located in the center of a circle passing through points A, B, and C. Q.E.I.
The problem dealt with in this lemma is also solved by means of Apollonius’s book On Tangencies, restored by Viète.
Proposition 21, Problem 13
To describe about a given focus a trajectory that will pass through given points and will touch straight lines given in position.
Let a focus S, a point P, and a tangent TR be given; the second focus H is to be found. Drop the perpendicular ST to the tangent and produce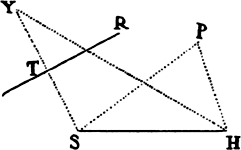 
ST to Y so that TY is equal to ST, and YH will be equal to the principal axis. Join SP and also HP, and SP will be the difference between HP and the principal axis. In this way, if more tangents TR or more points P are given, there will always be the same number of lines YH or PH, which can be drawn from the said points Y or P to the focus H, and which either are equal to the axes or differ from them by given lengths SP and so either are equal to one another or have given differences; and hence, by lem. 16, that second focus H is given. And once the foci are found, together with the length of the axis (which length is either YH, or PH + SP if the trajectory is an ellipse, but PH − SP if the trajectory is a hyperbola), the trajectory is found. Q.E.I.
Scholium
When the trajectory is a hyperbola, I do not include the opposite branch of the hyperbola as part of the trajectory. For a body going on with an uninterrupted motion cannot pass from one branch of a hyperbola into the other.
The case in which three points are given is solved more speedily as follows: Let the points B, C, and D be given. Join BC and also CD and produce them to E and F so that EB is to EC as SB to SC and FC is to FD as SC to SD. Draw EF, and drop the normals SG and BH to EF produced, and on GS indefinitely produced take GA to AS and Ga to aS as HB is to BS; then A will be the vertex and Aa the principal axis of the trajectory. According as GA is greater than, equal to, or less than AS, this trajectory will be an ellipse, a parabola, or a hyperbola, with point a in the first case falling on the same side of the line GF as point A, in the second case going off to infinity, in the third falling on the other side of the line GF. For if the perpendiculars CI and DK are dropped to GF, IC will be to HB as EC to EB, that is, as SC to SB; and by alternation [or alternando], IC will be to SC as HB to SB or as GA to SA. And by a similar argument it will be proved that KD is to SD in the same ratio. Therefore points B, C, and D lie in a conic described about the focus S in such a way that all the straight lines drawn from the focus S to the individual points of the conic are to the perpendiculars dropped from the same points to the straight line GF in that given ratio.
By a method that is not very different, the eminent geometer La Hire presents a solution of this problem in his Conics, book 8, prop. 25.
To find orbits when neither focus is given
Lemma 17
If four straight lines PQ, PR, PS, and PT are drawn at given angles from any point P of a given conic to the four indefinitely produced sides AB, CD, AC, and DB of some quadrilateral ABDC inscribed in the conic, one line being drawn to each side, the rectangle PQ × PR of the lines drawn to two opposite sides will be in a given ratio to the rectangle PS × PT of the lines drawn to the other two opposite sides.
CASE 1. Let us suppose first that the lines drawn to opposite sides are parallel to either one of the other sides, say PQ and PR parallel to side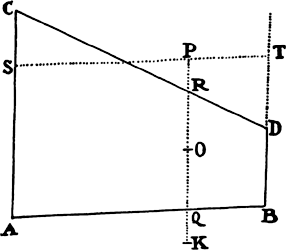 
AC, and PS and PT parallel to side AB. In addition, let two of the opposite sides, say AC and BD, be parallel to each other. Then the straight line which bisects those parallel sides will be one of the diameters of the conic and will bisect RQ also. Let O be the point in which RQ is bisected, and PO will be an ordinate to that diameter. Produce PO to K so that OK is equal to PO, and OK will be the ordinate on the opposite side of the diameter. Therefore, since points A, B, P, and K are on the conic and PK cuts AB at a given angle, the rectangle PQ × QK will be to the rectangle AQ × QB in a given ratio (by book 3, props. 17, 19, 21, and 23, of the Conics of Apollonius). But QK and PR are equal, inasmuch as they are differences of the equal lines OK and OP, and OQ and OR, and hence also the rectangles PQ × QK and PQ × PR are equal, and therefore the rectangle PQ × PR is to the rectangle AQ × QB, that is, to the rectangle PS × PT, in a given ratio. Q.E.D.
CASE 2. Let us suppose now that the opposite sides AC and BD of the quadrilateral are not parallel. Draw Bd parallel to AC, meeting the straight line ST in t and the conic in d. Join Cd cutting PQ in r; and draw DM parallel to PQ, cutting Cd in M and AB in N. Now, because triangles BTt and DBN are similar, Bt or PQ is to Tt as DN to NB. So also Rr is to AQ or PS as DM to AN. Therefore, multiplying the antecedents by the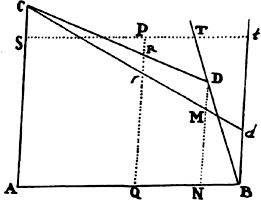 
antecedents and the consequents by the consequents, the rectangle PQ × Rr is to the rectangle PS × Tt as the rectangle ND × DM is to the rectangle AN × NB, and (by case 1) as the rectangle PQ × Pr is to the rectangle PS × Pt, and by separation [or dividendo] as the rectangle PQ × PR is to the rectangle PS × PT. Q.E.D.
CASE 3. Let us suppose finally that the four lines PQ, PR, PS, and PT are not parallel to the sides AC and AB, but are inclined to them in any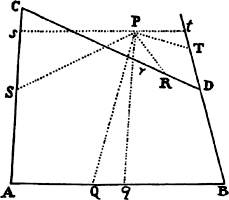 
way whatever. In place of these lines draw Pq and Pr parallel to AC, and Ps and Pt parallel to AB; then because the angles of the triangles PQq, PRr, PSs, and PTt are given, the ratios of PQ to Pq, PR to Pr, PS to Ps, and PT to Pt will be given, and thus the compound ratios of PQ × PR to Pq × Pr, and PS × PT to Ps × Pt. But, by what has been demonstrated above, the ratio of Pq × Pr to Ps × Pt is given, and therefore also the ratio of PQ × PR to PS × PT. Q.E.D.
Lemma 18
With the same suppositions as in lem. 17, if the rectangle PQ × PR of the lines drawn to two opposite sides of the quadrilateral is in a given ratio to the rectangle PS × PT of the lines drawn to the other two sides, the point P from which the lines are drawn will lie on a conic circumscribed about the quadrilateral.
Suppose that a conic is described through points A, B, C, D, and some one of the infinite number of points P, say p; I say that point P always lies on this conic. If you deny it, join AP cutting this conic in some point other than P, if possible, say in b. Therefore, if from these points p and b the straight lines pq, pr, ps, pt and bk, bn, bf, bd are drawn at given angles to the sides of the quadrilateral, then bk × bn will be to bf × bd as (by lem. 17) pq × pr is to ps × pt, and as (by hypothesis) PQ × PR is to PS × PT. Also, because the quadrilaterals bkAf and PQAS are similar, bk is to bf as PQ to PS. And therefore, if the terms of the previous 
proportion are divided by the corresponding terms of this one, bn will be to bd as PR to PT. Therefore the angles of the quadrilateral Dnbd are respectively equal to the angles of quadrilateral DRPT and the quadrilaterals are similar, and consequently their diagonals Db and DP coincide. And thus b falls upon the intersection of the straight lines AP and DP and accordingly coincides with point P. And therefore point P, wherever it is taken, falls on the assigned conic. Q.E.D.
COROLLARY. Hence if three straight lines PQ, PR, and PS are drawn at given angles from a common point P to three other straight lines given in position, AB, CD, and AC, one line being drawn to each of the other lines, and if the rectangle PQ × PR of two of the lines drawn is in a given ratio to the square of the third line PS, then the point P, from which the straight lines are drawn, will be located in a conic which touches lines AB and CD at A and C, and conversely. For let line BD coincide with line AC, while the position of the three lines AB, CD, and AC remains the same, and let line PT also coincide with line PS; then the rectangle PS × PT will come to be PS2, and the straight lines AB and CD, which formerly cut the curve in points A and B, C and D, can no longer cut the curve in those points which now coincide, but will only touch it.
Scholium
The term “conic” [or “conic section”] is used in this lemma in an extended sense, so as to include both a rectilinear section passing through the vertex of a cone and a circular section parallel to the base. For if point p falls on a straight line which joins points A and D or C and B, the conic section will turn into twin straight lines, one of which is the straight line on which point p falls and the other the straight line which joins the other two of the four points.
If two opposite angles of the quadrilateral, taken together, are equal to two right angles, and the four lines PQ, PR, PS, and PT are drawn to its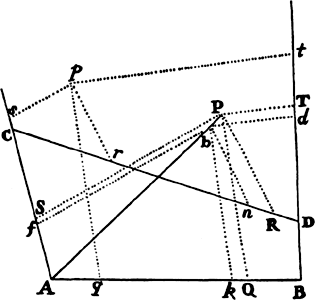 
sides either perpendicularly or at any equal angles, and the rectangle PQ × PR of two of the lines drawn is equal to the rectangle PS × PT of the other two, the conic will turn out to be a circle. The same will happen if the four lines are drawn at any angles and the rectangle PQ × PR of two of the lines drawn is to the rectangle PS × PT of the other two as the rectangle of the sines of the angles S and T, at which the last two lines PS and PT are drawn, is to the rectangle of the sines of the angles Q and R, at which the first two lines PQ and PR are drawn.
In the other cases the locus of point P will be some one of the three figures that are commonly called conic sections [or conics]. In place of the quadrilateral ABCD, however, there can be substituted a quadrilateral whose two opposite sides decussate each other as diagonals do. But also, one or two of the four points A, B, C, and D can go off to infinity, and in this way the sides of the figure which converge to these points can turn out to be parallel, in which case the conic will pass through the other points and will go off to infinity in the direction of the parallels.
Lemma 19
To find a point P such that if four straight lines PQ, PR, PS, and PT are drawn from it at given angles to four other straight lines AB, CD, AC, and BD given in position, one line being drawn from the point P to each of the four other straight lines, the rectangle PQ × PR of two of the lines drawn will be in a given ratio to the rectangle PS × PT of the other two.
Let lines AB and CD, to which the two straight lines PQ and PR containing one of the rectangles are drawn, meet the other two lines given in position in the points A, B, C, and D. From some one of them A draw any straight line AH, in which you wish point P to be found. Let this line AH cut the opposite lines BD and CD—that is, BD in H and CD in I—and because all the angles of the figure are given, the ratios of PQ to PA and PA to PS, and consequently the ratio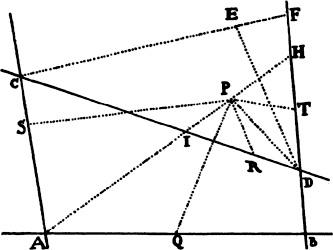 
of PQ to PS, will be given. On eliminating this ratio of PQ to PS from the given ratio of PQ × PR to PS × PT, the ratio of PR to PT will be given; and when the given ratios of PI to PR and PT to PH are combined, the ratio of PI to PH, and thus the point P, will be given. Q.E.I.
COROLLARY 1. Hence also a tangent can be drawn to any point D of the locus of the infinite number of points P. For when points P and D come together—that is, when AH is drawn through the point D—the chord PD becomes a tangent. In this case the ultimate ratio of the vanishing lines IP and PH will be found as above. Therefore, draw CF parallel to AD and meeting BD in F and being cut in E in that ultimate ratio; then DE will be a tangent, because CF and the vanishing line IH are parallel and are similarly cut in E and P.
COROLLARY 2.a Hence also, the 
locus of all the points P can be determined. Through any one of the points A, B, C, D—say A—draw the tangent AE of the locus, and through any other point B draw BF parallel to the tangent and meeting the locus in F. The point F will be found by means of lem. 19. Bisect BF in G, and the indefinite line AG, when drawn, will be the position of the diameter to which BG and FG are ordinates. Let this line AG meet the locus in H, and AH will be the diameter or latus transversum [i.e., transverse diameter] to which the latus rectum will be as BG2 to AG × GH. If AG nowhere meets the locus, the line AH being indefinitely produced, the locus will be a parabola, and its latus rectum corresponding to the diameter AG will be 
. But if AG does meet the locus somewhere, the locus will be a hyperbola when points A and H are situated on the same side of G, and an ellipse when G is between points A and H, unless angle AGB happens to be a right angle and additionally BG2 is equal to the rectangle AG × GH, in which case the locus will be a circle.
And thus there is exhibited in this corollary not an [analytical] computation but a geometrical synthesis, such as the ancients required, of the classical problem of four lines, which was begun by Euclid and carried on by Apollonius.
Lemma 20
If any parallelogram ASPQ touches a conic in points A and P with two of its opposite angles A and P, and if the sides AQ and AS, indefinitely produced, of one of these angles meet the said conic in B and C, and if from the meeting points B and C two straight lines BD and CD are drawn to any fifth point D of the conic, meeting the other two indefinitely produced sides PS and PQ of the parallelogram in T and R; then PR and PT, the parts cut off from the sides, will always be to each other in a given ratio. And conversely, if the parts which are cut off are to each other in a given ratio, the point D will touch a conic passing through the four points A, B, C, and P.
CASE 1. Join BP and also 
CP, and from point D draw two straight lines DG and DE, the first of which (DG) is parallel to AB and meets PB, PQ, and CA at H, I, and G, while the second (DE) is parallel to AC and meets PC, PS, and AB at F, K, and E; then (by lem. 17) the rectangle DE×DF will be to the rectangle DG × DH in a given ratio. But PQ is to DE (or IQ) as PB to HB and thus as PT to DH; and by alternation [or alternando] PQ is to PT as DE to DH. Additionally, PR is to DF as RC to DC and hence as (IG or) PS to DG; and by alternation [or alternando] PR is to PS as DF to DG; and when the ratios are combined, the rectangle PQ × PR comes to be to the rectangle PS × PT as the rectangle DE × DF to the rectangle DG × DH, and hence in a given ratio. But PQ and PS are given, and therefore the ratio of PR to PT is given. Q.E.D.
CASE 2. But if PR and PT are supposed in a given ratio to each other, then on working backward with a similar argument, it will follow that the rectangle DE × DF is to the rectangle DG × DH in a given ratio and consequently that point D (by lem. 18) lies in a conic passing through points A, B, C, and P. Q.E.D.
COROLLARY 1. Hence, if BC is drawn cutting PQ in r, and if Pt is taken on PT in the ratio to Pr which PT has to PR, Bt will be a tangent of the conic at point B. For conceive of point D as coming together with point B in such a way that, as chord BD vanishes, BT becomes a tangent; then CD and BT will coincide with CB and Bt.
COROLLARY 2. And vice versa, if Bt is a tangent and BD and CD meet in any point D of the conic, PR will be to PT as Pr to Pt. And conversely, if PR is to PT as Pr to Pt, BD and CD will meet in some point D of the conic.
COROLLARY 3. One conic does not intersect another conic in more than four points. For, if it can be done, let two conics pass through five points A, B, C, P, and O, and let the straight line BD cut these conics in points D and d, and let the straight line Cd cut PQ in q. Then PR is to PT as Pq to PT; hence PR and Pq are equal to each other, contrary to the hypothesis.
Lemma 21
If two movable and infinite straight lines BM and CM, drawn through given points B and C as poles, describe by their meeting-point M a third straight line MN given in position, and if two other infinite straight lines BD and CD are drawn, making given angles MBD and MCD with the first two lines at those given points B and C; then I say that the point D, where these two lines BD and CD meet, will describe a conic passing through points B and C. And conversely, if the point D, where the straight lines BD and CD meet, describes a conic passing through the given points B, C, and A, and the angle DBM is always equal to the given angle ABC, and the angle DCM is always equal to the given angle ACB; then point M will lie in a straight line given in position.
For let point N be given in the straight line MN; and when the movable point M falls on the stationary point N, let the movable point D fall on the stationary point P. Draw CN, BN, CP, and BP, and from point P draw the straight lines PT and PR meeting BD and CD in T and R and forming an angle BPT equal to the given angle BNM, and an angle CPR equal to the given angle CNM. Since therefore (by hypothesis) angles MBD and NBP are equal, as are also angles MCD and NCP, take away the angles NBD and NCD that are common, and there will remain the equal angles NBM and PBT, NCM and PCR; and therefore triangles NBM and PBT are similar, as are also triangles NCM and PCR. And therefore PT is to NM as PB to NB, and PR is to NM as PC to NC. But the points B, C, N, and P are stationary. Therefore, PT and PR have a given ratio to NM and accordingly a given ratio to each other; and thus (by lem. 20) the point D, the perpetual meeting-point of the movable straight lines BT and CR, lies in a conic passing through points B, C, and P. Q.E.D.
And conversely, if the movable point D lies in a conic passing through the given points B, C, and A; and if angle DBM is always equal to the given angle ABC, and the angle DCM is always equal to the given angle ACB; and if, when point D falls successively on any two stationary points p and P of the conic, the movable point M falls successively on the two stationary points n and N; then through these same points n and N draw the straight line nN, and this will be the perpetual locus of the movable point M. For, if it can be done, let point M move in some curved line. Then the point D will lie in a conic passing through the five points B, C, A, p, and P when the point M perpetually lies in a curved line. But from what has already been demonstrated, point D will also lie in a conic passing through the same five points B, C, A, p, and P when point M perpetually lies in a straight line. Therefore, two conics will pass through the same five points, contrary to lem. 20, corol. 3. Therefore, it is absurd to suppose the point M to be moving in a curved line. Q.E.D.
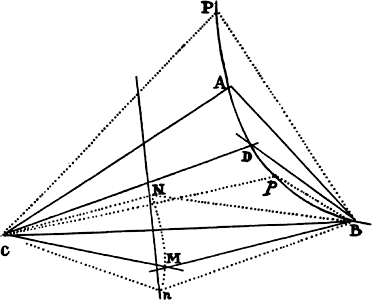
Proposition 22, Problem 14
To describe a trajectory through five given points.
Let five points A, B, C, P, and D be given. From one of them A to any other two B and C (let B and C be called poles), draw the straight lines AB and AC, and parallel to these draw TPS and PRQ through the fourth point P. Then from the two poles B and C draw two indefinite lines BDT and CRD through the fifth point D, BDT meeting the line TPS (just drawn) in T, and CRD meeting PRQ in R. Finally, draw the straight line tr parallel to TR, and cut off from the straight lines PT and PR any straight lines Pt and Pr proportional to PT and PR; then, if through their ends t and r and poles B and C the lines Bt and Cr are drawn meeting in d, that point d will be located in the required trajectory. For that point d (by lem. 20) lies in a conic passing through the four points A, B, C, and P; and, the lines Rr and Tt vanishing, point d coincides with point D. Therefore, the conic section passes through the five points A, B, C, P, and D. Q.E.D.
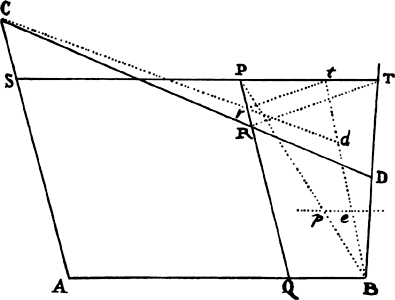
Join any three of the given points, A, B, and C; and, rotating the angles ABC and ACB, given in magnitude, around two of these points B and C as poles, apply the legs BA and CA first to point D and then to point P, and note the points M and N in which the other legs BL and CL cross in each case. Draw the indefinite straight line MN, and rotate these movable angles around their poles B and C in such a way that the intersection of the legs BL and CL or BM and CM (which now let be m) always falls on that indefinite straight line MN; and the intersection of the legs BA and CA or BD and CD (which now let be d) will trace out the required trajectory PADdB. For point d (by lem. 21) will lie in a conic passing through points B and C: and when point m approaches points L, M, and N, point d (by construction) will approach points A, D, and P. Therefore a conic will be described passing through the five points A, B, C, P, and D. Q.E.F.
COROLLARY 1. Hence a straight line can readily be drawn that will touch the required trajectory in any given point B. Let point d approach point B, and the straight line Bd will come to be the required tangent.
COROLLARY 2. Hence also the centers, diameters, and latera recta of the trajectories can be found, as in lem. 19, corol. 2.
Scholium
The first of the constructions of prop. 22 will become a little simpler by joining BP, producing it if necessary, and in it taking Bp to BP as PR is to 
PT, and then drawing through p the indefinite straight line pe parallel to SPT and in it always taking pe equal to Pr, and then drawing the straight lines Be and Cr meeting in d. For since the ratios Pr to Pt, PR to PT, pB to PB, and pe to Pt are equal, pe and Pr will always be equal. By this method the points of the trajectory are found most readily, unless you prefer to describe the curve mechanically, as in the second construction.
Proposition 23, Problem 15
To describe a trajectory that will pass through four given points and touch a straight line given in position.
CASE 1. Let the tangent HB, the point of contact B, and three other points C, D, and P be given. Join BC, and by drawing PS parallel to the straight line BH, and PQ parallel to the straight line BC, complete the parallelogram BSPQ. Draw BD cutting SP in T, and CD cutting PQ in R. Finally, by drawing any line tr parallel to TR, cut off Pr and Pt from PQ and PS in such a way that Pr and Pt are proportional respectively to PR and PT; then draw Cr and Bt, and their meeting-point d (by lem. 20) will always fall on the trajectory to be described.
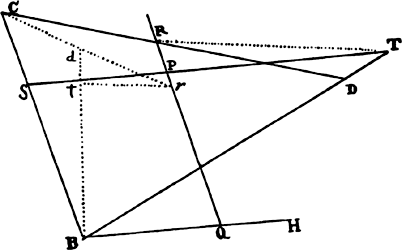
Revolve the angle CBH, given in 
magnitude, about the pole B, and revolve about the pole C any rectilinear radius DC, produced at both ends. Note the points M and N at which the leg BC of the angle cuts that radius when the other leg BH meets the same radius in points P and D. Then draw the indefinite line MN, and let that radius CP or CD and the leg BC of the angle meet perpetually in the line MN; and the meeting-point of the other leg BH with the radius will trace out the required trajectory.
For if, in the constructions of prop. 22, point A approaches point B, lines CA and CB will coincide, and line AB in its ultimate position will come to be the tangent BH; and therefore the constructions set forth in prop. 22 will come to be the same as the constructions described in this proposition. Therefore, the meeting-point of the leg BH with the radius will trace out a conic passing through points C, D, and P and touching the straight line BH in point B. Q.E.F.
CASE 2. Let four points B, C, D, and P be given, situated outside the tangent HI. Join them in pairs by the lines BD and CP coming together in G and meeting the tangent in H and I. Cut the tangent in A in such a way that HA is to IA as the rectangle of the mean proportional between CG and GP and the mean proportional between BH and HD is to the rectangle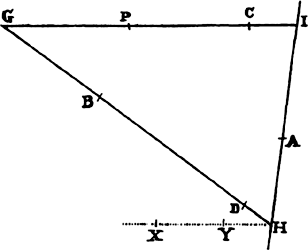 
of the mean proportional between DG and GB and the mean proportional between PI and IC, and A will be the point of contact. For if HX, parallel to the straight line PI, cuts the trajectory in any points X and Y, then (from the Conics) point A will have to be so placed that HA2 is to AI2 in a ratio compounded of the ratio of the rectangle XH × HY to the product BH × HD, or of the rectangle CG × GP to the rectangle DG × GD, and of the ratio of the rectangle BH × HD to the rectangle PI × IC. And once the point of contact A has been found, the trajectory will be described as in the first case. Q.E.F.
But point A can be taken either between points H and I or outside them, and accordingly two trajectories can be described as solutions to the problem.
Proposition 24, Problem 16
To describe a trajectory that will pass through three given points and touch two straight lines given in position.
Let tangents HI and KL and points B, C, and D be given. Through any two of the points, B and D, draw an indefinite straight line BD 
meeting the tangents in points H and K. Then, likewise, through any two other points, C and D, draw the indefinite straight line CD meeting the tangents in points I and L. Cut BD in R and CD in S in such a way that HR will be to KR as the mean proportional between BH and HD is to the mean proportional between BK and KD and that IS will be to LS as the mean proportional between CI and ID is to the mean proportional between CL and LD. And cut these lines at will either between points K and H, and between I and L, or outside them; then draw RS cutting the tangents in A and P, and A and P will be the points of contact. For if A and P are supposed to be the points of contact situated anywhere on the tangents, and if through any one of the points H, I, K, and L, say I, situated in either tangent HI, the straight line IY is drawn parallel to the other tangent KL and meeting the curve in X and Y; and if in this line, IZ is taken so as to be the mean proportional between IX and IY; then, from the Conics, the rectangle XI × IY or IZ2 will be to LP2 as the rectangle CI × ID to the rectangle CL × LD, that is (by construction), as SI2 to SL2, and thus IZ will be to LP as SI to SL. Therefore the points S, P, and Z lie in one straight line. Furthermore, since the tangents meet in G, the rectangle XI × IY or IZ2 will be to IA2 (from the Conics) as GP2 to GA2, and hence IZ will be to IA as GP to GA. Therefore, the points P, Z, and A lie in one straight line, and thus the points S, P, and A are in one straight line. And by the same argument it will be proved that the points R, P, and A are in one straight line. Therefore the points of contact A and P lie in the straight line RS. And once these points have been found, the trajectory will be described as in prop. 23, case 1. Q.E.F.
In this proposition and in prop. 23, case 2, the constructions are the same whether or not the straight line XY cuts the trajectory in X and Y, and they do not depend on this cut. But once the constructions have been demonstrated for the case in which the straight line does cut the trajectory, the constructions for the case in which it does not cut the trajectory also can be found; and for the sake of brevity I do not take the time to demonstrate them further.
Lemma 22
To change figures into other figures of the same class.
Let it be required to transmute any figure HGI. Draw at will two parallel straight lines AO and BL cutting in A and B any third line AB, given in position; and from any point G of the figure draw to the straight line AB any other straight line GD parallel to OA. Then from some point O, given in line OA, draw to the point D the straight line OD meeting BL at d, and from the meeting-point erect the straight line dg containing any given angle with the straight line BL and having the same ratio to Od that DG has to OD; and g will be the point in the new figure hgi corresponding to point G. By the same method, each of the points in the first figure will yield a corresponding point in the new figure. Therefore, suppose point G to be running through all the points in the first figure with a continual motion; 
then point g—also with a continual motion—will run through all the points in the new figure and will describe that figure. For the sake of distinction let us call DG the first ordinate, dg the new ordinate, AD the first abscissa, ad the new abscissa, O the pole, OD the abscinding radius, OA the first ordinate radius, and Oa (which completes the parallelogram OAba) the new ordinate radius.
I say now that if point G traces a straight line given in position, point g will also trace a straight line given in position. If point G traces a conic, point g will also trace a conic. I here count a circle among the conic sections. Further, if point G traces a curved line of the third analytic order, point g will likewise trace a curved line of the third order; and so on with curves of higher orders, the two curved lines which points G and g trace will always be of the same analytic order. For as ad is to OA, so are Od to OD, dg to DG, and AB to AD; and hence AD is equal to 
, and DG is equal to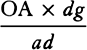 
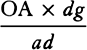. Now, if point G traces a straight line and consequently, in any equation which gives the relation between the abscissa AD and the ordinate DG, the indeterminate lines AD and DG rise to only one dimension, and if in this equation is written for AD and for DG, then the result will be a new equation in which the new abscissa ad and the new ordinate dg will rise to only one dimension and which therefore designates a straight line. But if AD and DG or either one of them rose to two dimensions in the first equation, then ad and dg will also rise to two dimensions in the second equation. And so on for three or more dimensions. The indeterminates ad and dg in the second equation, and AD and DG in the first, will always rise to the same number of dimensions, and therefore the lines which points G and g trace are of the same analytic order.
I say further that if some straight line touches a curved line in the first figure, this straight line—after being transferred into the new figure in the same manner as the curve—will touch that curved line in the new figure; and conversely. For if any two points of the curve approach each other and come together in the first figure, the same points—after being transferred—will approach each other and come together in the new figure; and thus the straight lines by which these points are joined will simultaneously come to be tangents of the curves in both figures.
The demonstrations of these assertions could have been composed in a more geometrical style. But I choose to be brief.
Therefore, if one rectilinear figure is to be transmuted into another, it is only necessary to transfer the intersections of the straight lines of which it is made up and to draw straight lines through them in the new figure. But if it is required to transmute a curvilinear figure, then it is necessary to transfer the points, tangents, and other straight lines which determine the curved line. Moreover, this lemma is useful for solving more difficult problems by transmuting the proposed figures into simpler ones. For any converging straight lines are transmuted into parallels by using for the first ordinate radius any straight line that passes through the meeting-point of the converging lines; and this is so because the meeting-point goes off this way to infinity, and lines that nowhere meet are parallel. Moreover, after the problem is solved in the new figure, if this figure is transmuted into the first figure by the reverse procedure, the required solution will be obtained.
This lemma is useful also for solving solid problems. For whenever two conics occur by whose intersection a problem can be solved, either one of them, if it is a hyperbola or parabola, can be transmuted into an ellipse; then the ellipse is easily changed into a circle. Likewise, in constructing plane problems, a straight line and a conic are turned into a straight line and a circle.
Proposition 25, Problem 17
To describe a trajectory that will pass through two given points and touch three straight lines given in position.
Through the meeting-point of any two tangents with each other and the meeting-point of a third tangent with the straight line that passes through two given points, draw an indefinite straight line; and using it as the first ordinate radius, transmute the figure, by lem. 22, into a new figure. In this figure the two tangents will come to be parallel to each other, and the third tangent will become parallel to the straight line passing through the two given points. Let hi and kl be the two parallel tangents, 
ik the third tangent, and hl the straight line parallel to it, passing through the points a and b through which the conic ought to pass in this new figure and completing the parallelogram hikl. Cut the straight lines hi, ik, and kl in c, d, and e, so that hc is to the square root of the rectangle ah × hb, and ic is to id, and ke is to kd, as the sum of the straight lines hi and kl is to the sum of three lines, of which the first is the straight line ik and the other two are the square roots of the rectangles ah × hb and al × lb; then c, d, and e will be the points of contact. For, from the Conics, hc2 is to the rectangle ah × hb in the same ratio as ic2 to id2, and ke2 to kd2, and el2 to the rectangle al × lb; and therefore hc is to the square root of ah × hb, and ic is to id, and ke is to kd, and el is to the square root of al × lb, as the square root of that ratio and hence, by composition [or componendo], in the given ratio of all the antecedents hi and kl to all the consequents, which are the square root of the rectangle ah × hb, the straight line ik, and the square root of the rectangle al × lb [i.e., in the given ratio of hi + kl to √(ah × hb) + ik + √(al × lb)]. Therefore, the points of contact c, d, and e in the new figure are obtained from that given ratio. By the reverse procedure of lem. 22, transfer these points to the first figure, and there (by prop. 22) the trajectory will be described. Q.E.F.
But according as points a and b lie between points h and l or lie outside them, points c, d, and e must be taken either between points h, i, k, and l, or outside them. If either one of the points a and b falls between points h and l, and the other outside, the problem is impossible.
Proposition 26, Problem 18
To describe a trajectory that will pass through a given point and touch four straight lines given in position.
From the common intersection of any two of the tangents to the common intersection of the other two, draw an indefinite straight line; then, using this as the first ordinate radius, transmute the figure (by lem. 22) into 
a new figure; then the tangents, which formerly met in the first ordinate radius, will now come to be parallel in pairs. Let those tangents be hi and kl, ik and hl, forming the parallelogram hikl. And let p be the point in this new figure corresponding to the given point in the first figure. Through the center O of the figure draw pq, and, on Oq being equal to Op, q will be another point through which the conic must pass in this new figure. By the reverse procedure of lem. 22 transfer this point to the first figure, and in that figure two points will be obtained through which the trajectory is to be described. And that trajectory can be described through these same points by prop. 25.
Lemma 23
If two straight lines AC and BD, given in position, terminate at the given points A and B and have a given ratio to each other; and if the straight line CD, by which the indeterminate points C and D are joined, is cut in K in a given ratio; I say that point K will be located in a straight line given in position.
For let the straight lines AC and BD meet in E, and in BE take BG 
to AE as BD is to AC, and let FD always be equal to the given line EG; then, by construction, EC will be to GD, that is, to EF, as AC to BD, and thus in a given ratio, and therefore the species of the triangle EFC will be given. Cut CF in L so that CL is to CF in the ratio of CK to CD; then, because that ratio is given, the species of the triangle EFL will also be given, and accordingly point L will be located in the straight line EL given in position. Join LK, and the triangles CLK and CFD will be similar; and because FD and the ratio of LK to FD are given, LK will be given. Take EH equal to LK, and ELKH will always be a parallelogram. Therefore, point K is located in the side HK, given in position, of the parallelogram. Q.E.D.
COROLLARY. Because the species of the figure EFLC is given, the three straight lines EF, EL, and EC (that is, GD, HK, and EC) have given ratios to one another.
Lemma 24
If three straight lines, two of which are parallel and given in position, touch any conic section, I say that the semidiameter of the section which is parallel to the two given parallel lines is a mean proportional between their segments that are intercepted between the points of contact and the third tangent.
Let AF and GB be two parallel 
lines touching the conic ADB in A and B; and let EF be a third straight line touching the conic in I and meeting the first tangents in F and G; and let CD be the semidiameter of the figure parallel to the tangents; then I say that AF, CD, and BG are continually proportional.
For if the conjugate diameters AB and DM meet the tangent FG in E and H and cut each other in C, and the parallelogram IKCL is completed, then, from the nature of conics, EC will be to CA as CA to CL, and by separation [or dividendo] as EC − CA to CA − CL, or EA to AL; and by composition [or componendo], EA will be to EA + AL or EL as EC to EC + CA or EB; and therefore, because the triangles EAF, ELI, ECH, and EBG are similar, AF will be to LI as CH to BG. And likewise, from the nature of conics, LI or CK is to CD as CD to CH and therefore from the equality of the ratios in inordinate proportion [or ex aequo perturbate] AF will be to CD as CD to BG. Q.E.D.
COROLLARY 1. Hence if two tangents FG and PQ meet the parallel tangents AF and BG in F and G, P and Q, and cut each other in O; then, from the equality of the ratios in inordinate proportion [or ex aequo perturbate] AF will be to BQ as AP to BG, and by separation [or dividendo] as FP to GQ, and thus as FO to OG.
COROLLARY 2. Hence also, two straight lines PG and FQ drawn through points P and G, F and Q, will meet in the straight line ACB that passes through the center of the figure and the points of contact A and B.
Lemma 25
If the indefinitely produced four sides of a parallelogram touch any conic and are intercepted at any fifth tangent, and if the intercepts of any two conterminous sides are taken so as to be terminated at opposite corners of the parallelogram; I say that either intercept is to the side from which it is intercepted as the part of the other conterminous side between the point of contact and the third side is to the other intercept.
Let the four sides ML, IK, KL, and MI of the parallelogram MLIK touch the conic section in A, B, C, and D, and let a fifth tangent FQ cut those sides in F, Q, H, and E; and take the intercepts ME and KQ of the sides MI and KI or the intercepts KH and MF of the sides KL and ML; I say that ME is to MI as BK to KQ, and KH is to KL as AM to MF. For by lem. 24, corol. 1, ME is to EI as AM or BK to BQ, and by composition [or componendo] ME is to MI as BK to KQ. Q.E.D. Likewise, KH is to HL as BK or AM to AF, and by separation [or dividendo] KH is to KL as AM to MF. Q.E.D.
COROLLARY 1. Hence if the parallelogram IKLM is given, described about a given conic, the rectangle KQ × ME will be given, as will also the rectangle KH × MF equal to it. For those rectangles are equal because the triangles KQH and MFE are similar.
COROLLARY 2. And if a sixth tangent eq is drawn meeting the tangents KI and MI at q and e, the rectangle KQ × ME will be equal to the rectangle Kq × Me, and KQ will be to Me as Kq to ME, and by separation [or dividendo] as Qq to Ee.
COROLLARY 3. Hence also, if Eq and eQ are drawn and bisected and a straight line is drawn through the points of bisection, this line will pass through the center of the conic. For since Qq is to Ee as KQ to Me, the same straight line will (by lem. 23) pass through the middle of all the lines Eq, eQ, and MK, and the middle of the straight line MK is the center of the section.
Proposition 27, Problem 19
To describe a trajectory that will touch five straight lines given in position.
Let the tangents ABG, BCF, GCD, FDE, and EA be given in position. Bisect in M and N the diagonals AF and BE of the quadrilateral figure ABFE formed by any four of those tangents, and (by lem. 25, corol. 3) the straight line MN drawn through the points of bisection will pass through the center of the trajectory. Again, bisect in P and Q the diagonals (as I call them) BD and GF of the quadrilateral figure BGFD formed by any other four tangents; then the straight line PQ drawn through the points of bisection will pass through the center of the trajectory. Therefore, the center will be given at the meeting-point of the bisecting lines. Let that center be O. Parallel to any tangent BC draw KL at such a distance that the center O is located midway between the parallels, and KL so drawn will touch the trajectory to be described. Let this line KL cut any other two tangents GCD and FDE in L and K. Through the meeting-points C and K, F and L, of these nonparallel tangents CL and FK with the parallels CF and KL, draw CK and FL meeting in R, and the straight line OR, drawn and produced, will cut the parallel tangents CF and KL in the points of contact. This is evident by lem. 24, corol. 2. By the same method other points of contact may be found, and then finally the trajectory may be described by the construction of prop. 22. Q.E.F.
Scholium
What has gone before includes problems in which either the centers or the asymptotes of trajectories are given. For when points and tangents are 
given together with the center, the same number of other points and tangents are given equally distant from the center on its other side. Moreover, an asymptote is to be regarded as a tangent, and its infinitely distant end-point (if it is permissible to speak of it in this way) as a point of contact. Imagine the point of contact of any tangent to go off to infinity, and the tangent will be turned into an asymptote, and the constructions of the preceding problems will be turned into constructions in which the asymptote is given.
After the trajectory has been described, its axes and foci may be found by the following method. In the construction and figure of lem. 21 make the legs BP and CP (by the meeting of which the trajectory was there described) of the mobile angles PBN and PCN be parallel to each other, and let them—while maintaining that position—revolve about their poles B and C in that figure. Meanwhile, let the circle BGKC be described by the point K or k in which the other legs CN and BN of those angles meet. Let the center of this circle be O. From this center to the ruler MN, at which those other legs CN and BN met while the trajectory was being described, drop the normal OH meeting the circle in K and L. And when those other legs CK and BK meet in K, the point that is nearer to the ruler, the first legs CP and BP will be parallel to the major axis and perpendicular to the minor axis; and the converse will occur if the same legs meet in the farther point L. Hence, if the center of a trajectory is given, the axes will be given. And when these are given, the foci are apparent.
But the squares of the axes are to 
each other as KH to LH, and hence it is easy to describe a trajectory, given in species, through four given points. For if two of the given points constitute the poles C and B, a third will give the mobile angles PCK and PBK; and once these are given, the circle BGKC can be described. Then, because the species of the trajectory is given, the ratio of OH to OK, and thus OH itself, will be given. With center O and radius OH describe another circle, and the straight line that touches this circle and passes through the meeting-point of the legs CK and BK when the first legs CP and BP meet in the fourth given point will be that ruler MN by means of which the trajectory will be described. Hence, in turn, a quadrilateral given in species can (except in certain impossible cases) also be inscribed in any given conic.
There are also other lemmas by means of which trajectories given in species can be described if points and tangents are given. An example: if a straight line, drawn through any point given in position, intersects a given conic in two points, and the distance between the intersections is bisected, the point of bisection will lie on another conic that is of the same species as the first one and that has its axes parallel to the axes of the first. But I pass quickly to what is more useful.
Lemma 26
To place the three corners of a triangle given in species and magnitude on three straight lines given in position and not all parallel, with one corner on each line.
aThree indefinite straight lines, AB, AC, and BC, are given in position, and it is required to place triangle DEF in such a way that its corner D touches line AB, corner E line AC, and corner F line BC.a On DE, DF, and 
EF describe three segments DRE, DGF, and EMF of circles, containing angles equal respectively to angles BAC, ABC, and ACB. And let these segments be described on those sides of the lines DE, DF, and EF that will make the letters DRED go round in the same order as the letters BACB, the letters DGFD in the same order as ABCA, and the letters EMFE in the same order as ACBA; then complete these segments into full circles. Let the first two circles cut each other in G, and let their centers be P and Q. Joining GP and also PQ, take Ga to AB as GP is to PQ; and with center G and radius Ga describe a circle that cuts the first circle DGE in a. Join aD cutting the second circle DFG in b, and aE cutting the third circle EMF in c. And now the figure ABCdef may be constructed similar and equal to the figure abcDEF. This being done, the problem is solved.
For draw Fc meeting aD in n, and join aG, bG, QG, QD, and PD. By construction, angle EaD is equal to angle CAB, and angle acF is equal to angle ACB, and thus the angles of triangle anc are respectively equal to the angles of triangle ABC. Therefore angle anc or FnD is equal to angle ABC, and hence equal to angle FbD; and therefore point n coincides with point b. Further, angle GPQ, which is half of angle GPD at the center, is equal to angle GaD at the circumference; and angle GQP, which is half of angle GQD at the center, is equal to the supplement of angle GbD at the circumference, and hence equal to angle Gba; and therefore triangles GPQ and Gab are similar, and Ga is to ab as GP to PQ, that is (by construction), as Ga to AB. And thus ab and AB are equal; and therefore triangles abc and ABC, which we have just proved to be similar, are also equal. Hence, since in addition the corners D, E, and F of the triangle DEF touch the sides ab, ac, and bc respectively of the triangle abc, the figure ABCdef can be completed similar and equal to the figure abcDEF; and by its completion the problem will be solved. Q.E.F.
COROLLARY. Hence a straight line can be drawn whose parts given in length will lie between three straight lines given in position. Imagine that triangle DEF, with point D approaching side EF and sides DE and DF placed in a straight line, is changed into a straight line whose given part DE is to be placed between the straight lines AB and AC given in position and whose given part DF is to be placed between the straight lines AB and BC given in position; then, by applying the preceding construction to this case, the problem will be solved.
Proposition 28, Problem 20
To describe a trajectory given in species and magnitude, whose given parts will lie between three straight lines given in position.
Let it be required to describe a trajectory that is similar and equal to the curved line DEF and that will be cut by three straight lines AB, AC, and BC, given in position, into parts similar and equal to the given parts DE and EF of this curved line.
Draw the straight lines DE, EF, and DF, place one of the corners D, E, and F of this triangle DEF on each of those straight lines given in position (by lem. 26); then about the triangle describe a trajectory similar and equal to the curve DEF. Q.E.F.
Lemma 27
To describe a quadrilateral given in species, whose corners will lie on four straight lines, given in position, which are not all parallel and do not all converge to a common point—each corner lying on a separate line.
Let four straight lines ABC, AD, BD, and CE 
be given in position, the first of which cuts the second in A, cuts the third in B, and cuts the fourth in C; let it be required to describe a quadrilateral fghi which is similar to the quadrilateral FGHI and whose corner f, equal to the given corner F, touches the straight line ABC, and whose other corners g, h, and i, equal to the other given corners G, H, and I, touch the other lines AD, BD, and CE respectively. Join FH, and on FG, FH, and FI describe three segments of circles, FSG, FTH, and FVI, of which the first (FSG) contains an angle equal to angle BAD, the second (FTH) contains an angle equal to angle CBD, and the third (FVI) contains an angle equal to angle ACE. The segments ought, moreover, to be described on those sides of the lines FG, FH, and FI that will make the circular order of the letters FSGF the same as that of the letters BADB, and will make the letters FTHF go round in the same order as CBDC, and the letters FVIF in the same order as ACEA. 
Complete the segments into whole circles, and let P be the center of the first circle FSG, and Q the center of the second circle FTH. Join PQ and produce it in both directions; and in it take QR in the ratio to PQ that BC has to AB. And take QR on the side of the point Q which makes the order of the letters P, Q, and R the same as that of the letters A, B, and C; and then with center R and radius RF describe a fourth circle FNc cutting the third circle FVI in c. Join Fc cutting the first circle in a and the second in b. Draw aG, bH, and cI, and the figure ABCfghi can be constructed similar to the figure abcFGHI. When this is done, the quadrilateral fghi will be the very one which it was required to construct.
For let the first two circles FSG and FTH intersect each other in K. Join PK, QK, RK, aK, bK, and cK, and produce QP to L. The angles FaK, FbK, and FcK at the circumferences are halves of the angles FPK, FQK, and FRK at the centers, and hence are equal to the halves LPK, LQK, and LRK of these angles. Therefore, the angles of figure PQRK are respectively equal to the angles of figure abcK, and the figures are similar; and hence ab is to bc as PQ to QR, that is, as AB to BC. Besides, the angles fAg, fBh, and fCi are (by construction) equal to the angles FaG, FbH, and FcI. Therefore, ABCfghi, a figure similar to the figure abcFGHI, can be completed. When this is done, the quadrilateral fghi will be constructed similar to the quadrilateral FGHI with its corners f, g, h, and i touching the straight lines ABC, AD, BD, and CE. Q.E.F.
COROLLARY. Hence a straight line can be drawn whose parts, intercepted in a given order between four straight lines given in position, will have a given proportion to one another. Increase the angles FGH and GHI until the straight lines FG, GH, and HI lie in a single straight line; and by constructing the problem in this case, a straight line fghi will be drawn, whose parts fg, gh, and hi, intercepted between four straight lines given in position, AB and AD, AD and BD, BD and CE, will be to one another as the lines FG, GH, and HI, and will keep the same order with respect to one another. But the same thing is done more expeditiously as follows.
Produce AB to K and BD to L so that BK is to AB as HI to GH, and DL to BD as GI to FG; and join KL meeting the straight line CE in i. Produce iL to M, so that LM is to iL; as GH to HI; and draw MQ parallel to LB and meeting the straight line AD in g, and draw gi cutting AB and BD in f and h. I declare it done.
For let Mg cut the straight line AB in Q, and let AD cut the straight line KL in S, and draw AP parallel to BD and meeting iL in P; then gM will be to Lh (gi to hi, Mi to Li, GI to HI, AK to BK) and AP to BL in the same ratio. Cut DL in R so that DL is to RL in that same ratio; then, because gS to gM, AS to AP, and DS to DL are proportional, from the equality of the ratios [or ex aequo] ASa will be to BL, and DS to RL, as gS to Lh, and by a mixture of operations BL − RL will be to Lh − BL as AS − DS to gS − AS. That is, BR will be to Bh as AD to Ag and thus as BD to gQ. And by alternation [or alternando] BR is to BD as Bh to gQ or as fh to fg. But by construction the line BL was cut in D and R in the same ratio as the line FI in G and H; and therefore BR is to BD as FH to FG. As a result, fh is to fg as FH to FG. Therefore, since gi is also to hi as Mi to Li, that is, as GI to HI, it is evident that the lines FI and fi are similarly cut in g and h, G and H. Q.E.F.
In the construction of this corollary, after LK is drawn cutting CE in i, it is possible to produce iE to V, so that EV is to Ei as FH to HI, and then to draw Vf parallel to BD. It comes to the same thing if with center i and radius IH a circle is described cutting BD in X, and if iX is produced to Y, so that iY is equal to IF, and if Yf is drawn parallel to BD.
Other solutions of this problem were devised some time ago by Wren and Wallis.
Proposition 29, Problem 21
To describe a trajectory, given in species, which four straight lines given in position will cut into parts given in order, species, and proportion.
Let it be required to describe a trajectory that is similar to the curved line FGHI and whose parts, similar and proportional to the parts FG, GH, and HI of the curve, are intercepted between the straight lines AB and AD, AD and BD, BD and CE given in position, the first part between the first two lines, the second between the second two lines, and the third between the third two lines. After drawing the straight lines FG, GH, HI, and FI, describe (by lem. 27) a quadrilateral fghi that is similar to the quadrilateral FGHI and whose corners f, g, h, and i touch the straight lines AB, AD, BD, and CE, given in position, each corner touching a separate line in the order stated. Then about this quadrilateral describe a trajectory exactly similar to the curved line FGHI.
Scholium
This problem can also be constructed as follows. After joining FG, GH, HI, and FI, produce GF to V, join FH and IG, and make angles CAK and DAL equal to angles FGH and VFH. Let AK and AL meet the straight line BD in K and L, and from these points draw KM and LN, of which KM makes an angle AKM equal to angle GHI and is to AK as HI is to GH, and LN makes an angle ALN equal to angle FHI and is to AL as HI to FH. And draw AK, KM, AL, and LN on those sides of the lines AD, AK, and AL that will make the letters CAKMC, ALKA, and DALND go round in the same order as the letters FGHIF; and draw MN meeting the straight line CE in i. Make angle iEP equal to the angle IGF, and let PE be to Ei as FG to GI; and through P draw PQf, which with the straight line ADE contains the angle PQE equal to the angle FIG and meets the straight line AB in f; and join fi. Now draw PE and PQ on those sides of the lines CE and PE that will make the circular order of the letters PEiP and PEQP the same as that of the letters FGHIF; and then, if on line fi a quadrilateral fghi similar to the quadrilateral FGHI is constructed (with the same order of the letters), and a trajectory given in species is circumscribed about the quadrilateral, the problem will be solved.
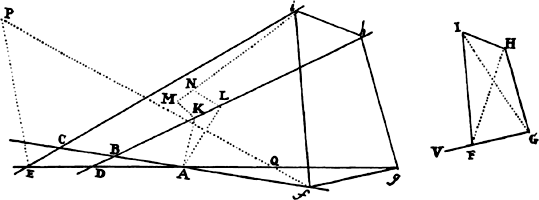
So much for the finding of orbits. It remains to determine the motions of bodies in the orbits that have been found.
To find motions in given orbits
Proposition 30, Problem 22
If a body moves in a given parabolic trajectory, to find its position at an assigned time.
Let S be the focus and A the principal vertex of the parabola, and let 4AS × M be equal to the parabolic area APS to be cut off, which 
either was described by the radius SP after the body’s departure from the vertex or is to be described by that radius before the body’s arrival at the vertex. The quantity of that area to be cut off can be found from the time, which is proportional to it. Bisect AS in G, and erect the perpendicular GH equal to 3M, and a circle described with center H and radius HS will cut the parabola in the required place P. For, when the perpendicular PO has been dropped to the axis and PH has been drawn, then AG2 + GH2 (= HP2 = (AO − AG)2 + (PO − GH)2) = AO2 + PO2 − 2GA × AO − 2GH × PO + AG2 + GH2. Hence 2GH × PO (= AO2 + PO2 − 2GA × AO) = AO2 + ¾PO2. For AQ2 write 
, and if all the terms are divided by 3PO and multiplied by 2AS, it will result that 4/3GH × AS = ⅙AO × PO + ½AS × PO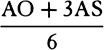 
× PO = 
× PO = area (APO − SPO) = area APS. But GH was 3M, and hence 4/3GH × AS is 4AS × M. Therefore, the area APS that was cut off is equal to the area 4AS × M that was to be cut off. Q.E.D.
COROLLARY 1. Hence GH is to AS as the time in which the body described the arc AP is to the time in which it described the arc between the vertex A and a perpendicular erected from the focus S to the axis.
COROLLARY 2. And if a circle ASP continually passes through the moving body P, the velocity of point H is to the velocity which the body had at the vertex A as 3 to 8, and thus the line GH is also in this ratio to the straight line which the body could describe in the time of its motion from A to P with the velocity which it had at the vertex A.
COROLLARY 3. Hence also, conversely, the time can be found in which the body described any assigned arc AP. Join AP and at its midpoint erect a perpendicular meeting the straight line GH in H.
Lemma 28
No oval figure exists whose area, cut off by straight lines at will, can in general be found by means of equations finite in the number of their terms and dimensions.
Within an oval let any point be given about which, as a pole, a straight line revolves continually with uniform motion, and meanwhile in that straight line let a mobile point go out from the pole and proceed always with the velocity that is as the square of that straight line within the oval. By this motion that point will describe a spiral with an infinite number of gyrations. Now, if the portion of the area of the oval cut off by that straight line can be found by means of a finite equation, there will also be found by the same equation the distance of the point from the pole, a distance that is proportional to this area, and thus all the points of the spiral can be found by means of a finite equation; and therefore the intersection of any straight line, given in position, with the spiral can also be found by means of a finite equation. But every infinitely produced straight line cuts a spiral in an infinite number of points; and the equation by which some intersection of two lines [i.e., curved lines] is found gives all their intersections by as many roots [as there are intersections] and therefore rises to as many dimensions as there are intersections. Since two circles cut each other in two points, one intersection will not be found except by an equation of two dimensions, by which the other intersection may also be found. Since two conics can have four intersections, one of these intersections cannot generally be found except by an equation of four dimensions, by means of which all four of the intersections may be found simultaneously. For if those intersections are sought separately, since they all have the same law and condition, the computation will be the same in each case, and therefore the conclusion will always be the same, which accordingly must comprehend all the intersections together and give them indiscriminately. Hence also the intersections of conics and of curves of the third power, because there can be six such intersections, are found simultaneously by equations of six dimensions; and intersections of two curves of the third power, since there can be nine of them, are found simultaneously by equations of nine dimensions. If this did not happen necessarily, all solid problems might be reduced to plane problems, and higher than solid to solid problems. I am speaking here of curves with a power that cannot be reduced. For if the equation by which the curve is defined can be reduced to a lower power, the curve will not be simple, but will be compounded of two or more curves whose intersections can be found separately by different computations. In the same way, the pairs of intersections of straight lines and conics are always found by equations of two dimensions; the trios of intersections of straight lines and of irreducible curves of the third power, by equations of three dimensions; the quartets of intersections of straight lines and of irreducible curves of the fourth power, by equations of four dimensions; and so on indefinitely. Therefore, the intersections of a straight line and of a spiral, which are infinite in number (since this curve is simple and cannot be reduced to more curves), require equations infinite in the number of their dimensions and roots, by which all the intersections can be given simultaneously. For they all have the same law and computation. For if a perpendicular is dropped from the pole to the intersecting straight line, and the perpendicular, together with the intersecting straight line, revolves about the pole, the intersections of the spiral will pass into one another, and the one that was the first or the nearest to the pole will be the second after one revolution, and after two revolutions will be third, and so on; nor will the equation change in the meantime except insofar as there is a change in the magnitude of the quantities by which the position of the intersecting line is determined. Hence, since the quantities return to their initial magnitudes after each revolution, the equation will return to its original form, and thus one and the same equation will give all the intersections and therefore will have an infinite number of roots by which all of the intersections can be given. Therefore, it is not possible for the intersection of a straight line and a spiral to be found universally by means of a finite equation, and on that account no oval exists whose area, cut off by prescribed straight lines, can universally be found by such an equation.
By the same argument, if the distance between the pole and the point by which the spiral is described is taken proportional to the intercepted part of the perimeter of the oval, it can be proved that the length of the perimeter cannot universally be found by a finite equation. aBut here I am speaking of ovals that are not touched by conjugate figures extending out to infinity.a
COROLLARY. Hence the area of an ellipse that is described by a radius drawn from a focus to a moving body cannot be found, from a time that has been given, by means of a finite equation, and therefore cannot be determined by describing geometrically rational curves. I call curves “geometrically rational” when all of their points can be determined by lengths defined by equations, that is, by involved ratios of lengths, and I call the other curves (such as spirals, quadratrices, and cycloids) “geometrically irrational.” For lengths that are or are not as integer to integer (as in book 10 of the Elements) are arithmetically rational or irrational. Therefore I cut off an area of an ellipse proportional to the time by a geometrically irrational curve as follows.
Proposition 31a, Problem 23
If a body moves in a given elliptical trajectory, to find its position at an assigned time.
Let A be the principal vertex of the ellipse APB, S a focus, and O the center, and let P be the position of the body. Produce OA to G so that OG is to OA as OA to OS. Erect the perpendicular GH, and with center O and radius OG describe the circle GEF; then, along the rule GH as a base let the wheel GEF move progressively forward, revolving about its own axis, while the point A on the wheel describes the cycloid ALI. When this has been done, take GK so that it will have the same ratio to the perimeter GEFG of the wheel as the time in which the body, in moving forward from A, described the arc AP has to the time of one revolution in the ellipse. Erect the perpendicular KL meeting the cycloid in L; and when LP has been drawn parallel to KG, it will meet the ellipse in the required position P of the body.
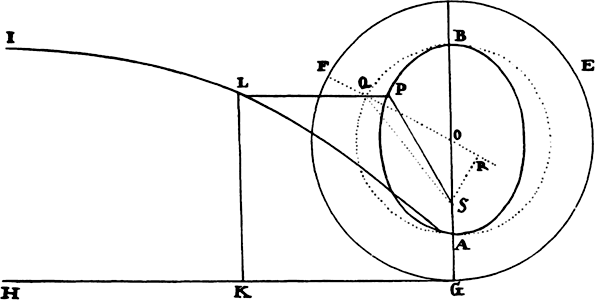
For with center O and radius OA describe the semicircle AQB, and let LP, produced if necessary, meet the arc AQ in Q, and join SQ and also OQ. Let OQ meet the arc EFG in F, and drop the perpendicular SR to OQ. Area APS is as area AQS, that is, as the difference between sector OQA and triangle OQS, or as the difference of the rectangles ½OQ × AQ and ½OQ × SR, that is, because ½OQ is given, as the difference between the arc AQ and the straight line SR, and hence (because of the equality of the given ratios of SR to the sine of the arc AQ, OS to OA, OA to OG, AQ to GF, and so by separation [or dividendo] AQ − SR to GF − the sine of the arc AQ) as GK, the difference between the arc GF and the sine of the arc AQ. Q.E.D.
Scholium
But the description of this curve is difficult; hence it is preferable to use a solution that is approximately true. Find a certain angle B that is to the angle of 57.29578° (which an arc equal to the radius subtends) as the distance SH between the foci is to the diameter AB of the ellipse; and also find a certain length L that is to the radius in the inverse of that ratio. Once these have been found, the problem can thereupon be solved by the following analysis.
By any construction, or by making any kind of guess, find the body’s position P very close to its true position p. Then, when the ordinate PR has been dropped to the axis of the ellipse, the ordinate RQ of the circumscribed circle AQB will be given from the proportion of the diameters of the ellipse, where the ordinate RQ is the sine of the angle AOQ (AO being the radius) and cuts the ellipse in P. It is sufficient to find this angle AOQ approximately by a rough numerical calculation. Also find the angle proportional to the time, that is, the angle that is to four right angles as the time in which the body described the arc Ap is to the time of one revolution in the ellipse. Let that angle be N. Then take an angle D that will be to angle B as the sine of angle AOQ is to the radius, and also take an angle E that will be to angle N − AOQ + D as the length L is to this same length L minus the cosine of angle AOQ when that angle is less than a right angle, but plus that cosine when it is greater. Next take an angle F that will be to angle B as the sine of angle AOQ + E is to the radius, and take an angle G that will be to angle N − AOQ − E + F as the length L is to this same length minus the cosine of angle AOQ + E when that angle is less than a right angle, and plus that cosine when it is greater. Thirdly, take an angle H that will be to angle B as the sine of angle AOQ + E + G is to the radius, and take an angle I that will be to angle N − AOQ − E − G + H as the length L is to this same length L minus the cosine of angle AOQ + E + G when that angle is less than a right angle, but plus that cosine when it is greater. And so on indefinitely. Finally take angle AOq equal to angle AOQ + E + G + I + · · · . And from its cosine Or and ordinate pr, which is to its sine qr as the minor axis of the ellipse to the major axis, the body’s corrected place p will be found. If the angle N − AOQ + D is negative, the + sign of E must everywhere be changed to −, and the − sign to +. The same is to be understood of the signs of G and I when the angles N − AOQ − E + F and N − AOQ − E − G + H come out negative. But the infinite series AOQ + E + G + I + · · · converges so very rapidly that it is scarcely ever necessary to proceed further than the second term E. And the computation is based on this theorem: that the area APS is as the difference between the arc AQ and the straight line dropped perpendicularly from the focus S to the radius OQ.
In the case of a hyperbola the problem is solved by a similar computation. Let O be its center, A a vertex, S a focus, and OK an asymptote. Find the quantity of the area to be cut off, which is proportional to the time. Let this quantity be A, and guess the position of the straight line SP that cuts off an approximately true area APS. Join OP, and from A and P to the asymptote OK draw AI and PK parallel to the second asymptote; then a table of logarithms will give the area AIKP and the equal area OPA,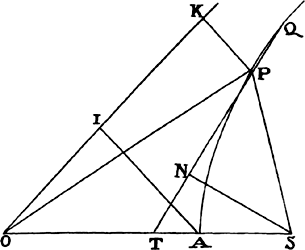 
which, on being subtracted from the triangle OPS, will leave the cut-off area APS. Divide 2APS − 2A or 2A − 2APS (twice the difference of the area A to be cut off and the cut-off area APS) by the line SN, which is perpendicular to the tangent TP from the focus S, so as to obtain the length of the chord PQ. Now, draw the chord PQ between A and P if the cut-off area APS is greater than the area A to be cut off, but otherwise draw PQ on the opposite side of point P, and then the point Q will be a more accurate position of the body. And by continually repeating the computation, a more and more accurate position will be obtained.
And by these computations a general analytical solution of the problem is achieved. But the particular computation that follows is more suitable for 
astronomical purposes. Let AO, OB, and OD be the semiaxes of the ellipse, and L its latus rectum, and D the difference between the semiaxis minor OD and half of the latus rectum ½L; find an angle Y, whose sine is to the radius as the rectangle of that difference D and the half-sum of the axes AO+OD is to the square of the major axis AB; and find also an angle Z, whose sine is to the radius as twice the rectangle of the distance SH between the foci and the difference D is to three times the square of the semiaxis major AO. Once these angles have been found, the position of the body will thereupon be determined as follows: Take an angle T proportional to the time in which arc BP was described, or equal to the mean motion (as it is called); and an angle V (the first equation of the mean motion) that shall be to angle Y (the greatest first equation) as the sine of twice angle T is to the radius; and an angle X (the second equation) that shall be to angle Z (the greatest second equation) as the cube of the sine of angle T is to the cube of the radius. Then take the angle BHP (the equated mean motion) equal either to the sum T + X + V of angles T, X, and V if angle T is less than a right angle, or to the difference T + X − V if angle T is greater than a right angle and less than two right angles; and if HP meets the ellipse in P, SP (when drawn) will cut off the area BSP very nearly proportional to the time.
This technique seems expeditious enough because it is sufficient to find the first two or three figures of the extremely small angles V and X (reckoned in seconds, if it is agreeable). This technique is also accurate enough for the theory of the planets. For even in the orbit of Mars itself, whose greatest equation of the center is ten degrees, the error will hardly exceed one second. But when the angle BHP of equated mean motion has been found, the angle BSP of true motion and the distance SP are readily found by the very well known method.
So much for the motion of bodies in curved lines. It can happen, however, that a moving body descends straight down or rises straight up; and I now go on to expound what relates to motions of this sort.
The rectilinear ascent and descent of bodies
Proposition 32a, Problem 24
Given a centripetal force inversely proportional to the square of the distance of places from its center, to determine the spaces which a body in falling straight down describes in given times.
CASE 1. If the body does not fall perpendicularly, it will (by prop. 13, corol. 1) describe some conic having a focus coinciding with the center of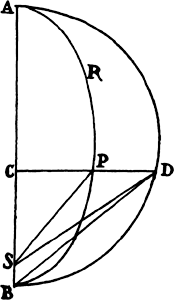 
forces. Let the conic be ARPB, and its focus S. And first, if the figure is an ellipse, on its major axis AB describe the semicircle ADB, and let the straight line DPC pass through the falling body and be perpendicular to the axis; and when DS and PS have been drawn, area ASD will be proportional to area ASP and thus also to the time. Keeping the axis AB fixed, continually diminish the width of the ellipse, and area ASD will always remain proportional to the time. Diminish that width indefinitely; and, the orbit APB now coming to coincide with the axis AB, and the focus S with the terminus B of the axis, the body will descend in the straight line AC, and the area ABD will become proportional to the time. Therefore 
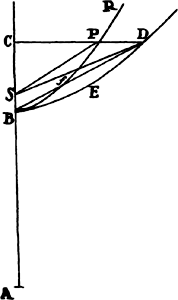the space AC will be given, which the body in falling perpendicularly from place A describes in a given time, provided that area ABD is taken proportional to that time and the perpendicular DC is dropped from point D to the straight line AB. Q.E.I.
CASE 2. If the figure RPB is a hyperbola, describe the rectangular hyperbola BED on the same principal diameter AB; and since the areas CSP, CBfP, and SPfB are respectively to the areas CSD, CBED, and SDEB in the given ratio of the distances CP and CD, and the area SPfB is proportional to the time in which body P will move through the arc PfB, the area SDEB will also be proportional to that same time. Diminish the latus rectum of the hyperbola RPB indefinitely, keeping the principal diameter fixed, and the arc PB will coincide with the straight line CB, and the focus S with the vertex B, and the straight line SD with the straight line BD. Accordingly, the area BDEB will be proportional to the time in which body C, falling straight down, describes the line CB. Q.E.I.
CASE 3. And by a similar argument, let the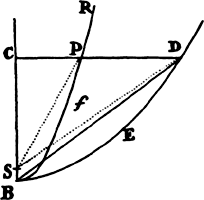 
figure RPB be a parabola and let another parabola BED with the same principal vertex B be described and always remain given, while the latus rectum of the first parabola (in whose perimeter the body P moves) is diminished and reduced to nothing, so that this parabola comes to coincide with the line CB; then the parabolic segment BDEB will become proportional to the time in which the body P or C will descend to the center S or B. Q.E.I.
Proposition 33, Theorem 9
Supposing what has already been found, I say that the velocity of a falling body at any place C is to the velocity of a body describing a circle with center B and radius BC as the square root of the ratio of AC (the distance of the body from the further vertex A of the circle or rectangular hyperbola) to ½AB (the principal semidiameter of the figure).
Bisect AB, the common diameter of both figures RPB and DEB, in O; and draw the straight line PT touching the figure RPB in P and also cutting the common diameter AB (produced if necessary) in T, and let SY be perpendicular to this straight line and BQ be perpendicular to this diameter, and take the latus rectum of the figure RPB to be L. It is established by prop. 16, corol. 9, that at any place P the velocity of a body moving about the center S in the [curved] line RPB is to the velocity of a body describing a circle about the same center with the radius SP as the square root of the ratio of the rectangle ½L × SP to SY2. But from the Conics, AC × CB is to CP2 as 2AO to L, and thus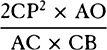 
is equal to L. Therefore, the velocities are to each other as the square root of the ratio of 
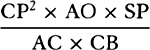to SY2. Further, from the Conics, CO is to BO as BO to TO, and by composition [or componendo] or by separation [or dividendo], as CB to BT. Hence, either by separation or by composition, BO ∓ CO becomes to BO as CT to BT, that is, AC to AO as CP to BQ; and hence 
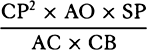is equal to 
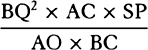. Now let the width CP of the figure RPB be diminished indefinitely, in such a way that point P comes to coincide with point C and point S with point B and the line SP with the line BC and the line SY with the line BQ; then the velocity of the body now descending straight down in the line CB will become to the velocity of a body describing a circle with center B and radius BC as the square root of the ratio of 
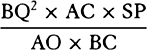to SY2, that is (neglecting the ratios of equality SP to BC and BQ2 to SY2), as the square root of the ratio of AC to AO or ½AB. Q.E.D.
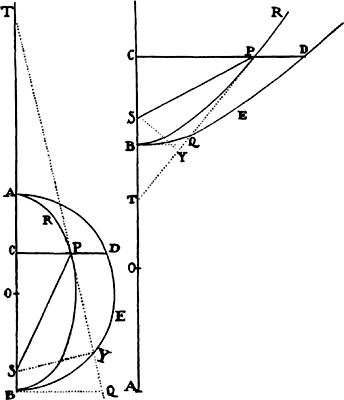
COROLLARY 1. When the points B and S come to coincide, TC becomes to TS as AC to AO.
COROLLARY 2. A body revolving in any circle at a given distance from the center will, when its motion is converted to an upward motion, ascend to twice that distance from the center.
Proposition 34, Theorem 10
If the figure BED is a parabola, I say that the velocity of a falling body at any place C is equal to the velocity with which a body can uniformly describe a circle with center B and a radius equal to one-half of BC.
For at any place P the velocity of a body describing the parabola RPB about the center S is (by prop. 16, corol. 7) equal to the velocity of a body 
uniformly describing a circle about the same center S with a radius equal to half of the interval SP. Let the width CP of the parabola be diminished indefinitely, so that the parabolic arc PfB will come to coincide with the straight line CB, the center S with the vertex B, and the interval SP with the interval BC, and the proposition will be established. Q.E.D.
Proposition 35, Theorem 11
Making the same suppositions, I say that the area of the figure DES described by the indefinite radius SD is equal to the area that a body revolving uniformly in orbit about the center S can describe in the same time by a radius equal to half of the latus rectum of the figure DES.
For suppose that body C falling in a minimally small particle of time describes the line-element Cc while another body K, revolving uniformly in the circular orbit OKk about the center S, describes the arc Kk. Erect the perpendiculars CD and cd meeting the figure DES in D and d. Join SD, Sd, SK, and Sk, and draw Dd meeting the axis AS in T, and drop the perpendicular SY to Dd.
CASE 1. Now, if the figure DES is a circle or a rectangular hyperbola, bisect its transverse diameter AS in O, and SO will be half of the latus rectum. And since TC is to TD as Cc to Dd, and TD to TS as CD to SY, from the equality of the ratios [or ex aequo] TC will be to TS as CD × Cc to SY × Dd. But (by prop. 33, corol. 1) TC is to TS as AC to AO, if, say, in the coming together of points D and d the ultimate ratios of the lines are taken. Therefore, AC is to AO or SK as CD × Cc is to SY × Dd. Further, the velocity of a descending body at C is to the velocity of a body describing a circle about the center S with radius SC as the square root of the ratio of AC to AO or SK (by prop. 33). And this velocity is to the velocity of a body describing the circle OKk as the square root of the ratio of SK to SC (by prop. 4, corol. 6), and from the equality of the ratios [or ex aequo] the first velocity is to the ultimate velocity, that is, the line-element Cc is to the arc Kk, as the square root of the ratio of AC to SC, that is, in the ratio of AC to CD. Therefore, CD × Cc is equal to AC × Kk, and thus AC is to SK as AC × Kk to SY × Dd, and hence SK × Kk is equal to SY × Dd, and ½SK × Kk is equal to ½SY × Dd, that is, the area KSk is equal to the area SDd. Therefore, in each particle of time, particles KSk and SDd of the two areas are generated such that, if their magnitude is diminished and their number increased indefinitely, they obtain the ratio of equality; and therefore (by lem. 4, corol.), the total areas generated in the same times are always equal. Q.E.D.
CASE 2. But if the figure DES is 
a parabola, then it will be found that, as above, CD × Cc is to SY × Dd as TC to TS, that is, as 2 to 1, and thus ¼CD × Cc will be equal to ½SY × Dd. But the velocity of the falling body at C is equal to the velocity with which a circle can be described uniformly with the radius ½SC (by prop. 34). And this velocity is to the velocity with which a circle can be described with the radius SK, that is, the line-element Cc is to the arc Kk (by prop. 4, corol. 6), as the square root of the ratio of SK to ½SC, that is, in the ratio of SK to ½CD. And therefore ½SK × Kk is equal to ¼CD × Cc and thus equal to ½SY × Dd; that is, the area KSk is equal to the area SDd, as above. Q.E.D.
Proposition 36, Problem 25
To determine the times of descent of a body falling from a given place A.
Describe a semicircle ADS with diameter AS (the 
distance of the body from the center at the beginning of the descent), and about the center S describe a semicircle OKH equal to ADS. From any place C of the body erect the ordinate CD. Join SD, and construct the sector OSK equal to the area ASD. It is evident by prop. 35 that the body in falling will describe the space AC in the same time in which another body, revolving uniformly in orbit about the center S, can describe the arc OK. Q.E.F.
Proposition 37, Problem 26
To define the times of the ascent or descent of a body projected upward or downward from a given place.
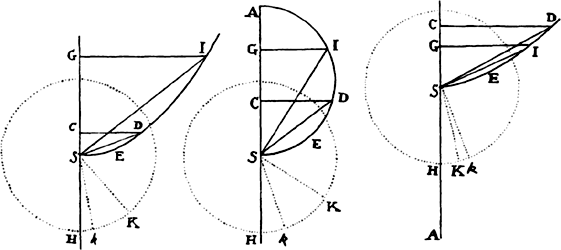
Let the body depart from the given place G along the line GS with any velocity whatever. Take GA to ½AS as the square of the ratio of this velocity to the uniform velocity in a circle with which the body could revolve about the center S at the given interval (or distance) SG. If that ratio is as 2 to 1, point A is infinitely distant, in which case a parabola is to be described with vertex S, axis SG, and any latus rectum, as is evident by prop. 34. But if that ratio is smaller or greater than the ratio of 2 to 1, then in the former case a circle, and in the latter case a rectangular hyperbola, must be described on the diameter SA, as is evident by prop. 33. Then, with center S and a radius equaling half of the latus rectum, describe the circle HkK, and to the place G of the descending or ascending body and to any other place C, erect the perpendiculars GI and CD meeting the conic or the circle in I and D. Then joining SI and SD, let the sectors HSK and HSk be made equal to the segments SEIS and SEDS, and by prop. 35 the body G will describe the space GC in the same time as the body K can describe the arc Kk. Q.E.F.
Proposition 38, Theorem 12
Supposing that the centripetal force is proportional to the height or distance of places from the center, I say that the times of falling bodies, their velocities, and the spaces described are proportional respectively to the arcs, the right sines, and the versed sines.
Let a body fall from any place A along the 
straight line AS; and with center of forces S and radius AS describe the quadrant AE of a circle, and let CD be the right sine of any arc AD; then the body A, in the time AD, will in falling describe the space AC and at place C will acquire the velocity CD.
This is demonstrated from prop. 10 in the same way that prop. 32 was demonstrated from prop. 11.
COROLLARY 1. Hence the time in which one body, falling from place A, arrives at the center S is equal to the time in which another body, revolving, describes the quadrantal arc ADE.
COROLLARY 2. Accordingly, all the times are equal in which bodies fall from any places whatever as far as to the center. For all the periodic times of revolving bodies are (by prop. 4, corol. 3) equal.
Proposition 39, Problem 27
Suppose a centripetal force of any kind, and grant the quadratures of curvilinear figures; it is required to find, for a body ascending straight up or descending straight down, the velocity in any of its positions and the time in which the body will reach any place; and conversely.
Let a body E fall from any place A whatever in the straight line ADEC, and let there be always erected from the body’s place E the perpendicular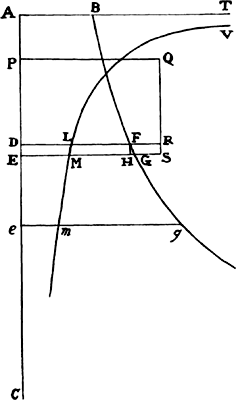 
EG, proportional to the centripetal force in that place tending toward the center C; and let BFG be the curved line which the point G continually traces out. At the very beginning of the motion let EG coincide with the perpendicular AB; then the velocity of the body in any place E will be as the straight line whose square is equal to the curvilinear area ABGE. Q.E.I.
In EG take EM inversely proportional to the straight line whose square is equal to the area ABGE, and let VLM be a curved line which the point M continually traces out and whose asymptote is the straight line AB produced; then the time in which the body in falling describes the line AE will be as the curvilinear area ABTVME. Q.E.I.
For in the straight line AE take a minimally small line DE of a given length, and let DLF be the location of the line EMG when the body was at D; then, if the centripetal force is such that the straight line whose square is equal to the area ABGE is as the velocity of the descending body, the area itself will be as the square of the velocity, that is, if V and V + I are written for the velocities at D and E, the area ABFD will be as V2, and the area ABGE as V2 + 2VI + I2, and by separation [or dividendo] the area DFGE will be as 2VI + I2, and thus 
will be as 
, that is, if the first ratios of nascent quantities are taken, the length DF will be as the quantity 
, and thus also as half of that quantity, or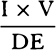 
. But the time in which the body in falling describes the line-element DE is as that line-element directly and the velocity V inversely, and the force is as the increment I of the velocity directly and the time inversely, and thus—if the first ratios of nascent quantities are taken—as 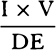, that is, as the length DF. Therefore a force proportional to DF or EG makes the body descend with the velocity that is as the straight line whose square is equal to the area ABGE. Q.E.D.
Moreover, since the time in which any line-element DE of a given length is described is as the velocity inversely, and hence inversely as the straight line whose square is equal to the area ABFD, and since DL (and hence the nascent area DLME) is as the same straight line inversely, the time will be as the area DLME, and the sum of all the times will be as the sum of all the areas, that is (by lem. 4, corol.), the total time in which the line AE is described will be as the total area ATVME. Q.E.D.
COROLLARY 1. Let P be the place from which a body must fall so that, under the action of some known uniform centripetal force (such as gravity is commonly supposed to be), it will acquire at place D a velocity equal to the velocity that another body, falling under the action of any force whatever, acquired at the same place D. In the perpendicular DF take DR such that it is to DF as that uniform force is to the other force at the place D. Complete the rectangle PDRQ and cut off the area ABFD equal to it. Then A will be the place from which the other body fell.
For, when the rectangle DRSE has been completed, the area ABFD is to the area DFGE as V2 to 2VI and hence as ½V to I, that is, as half of the total velocity to the increment of the velocity of the body falling under the action of the nonuniform force; and similarly, the area PQRD is to the area DRSE as half of the total velocity is to the increment of the velocity of 
the body falling under the action of the uniform force, and those increments (because the nascent times are equal) are as the generative forces, that is, as the ordinates DF and DR, and thus as the nascent areas DFGE and DRSE. Therefore, the total areas ABFD and PQRD will then from the equality of the ratios [or ex aequo] be to each other as halves of the total velocities and therefore are equal because the velocities are equal.
COROLLARY 2. Hence if any body is projected with a given velocity either upward or downward from any place D and the law of centripetal force is given, the velocity of the body at any other place e will be found by erecting the ordinate eg and taking that velocity at place e to the velocity at place D as the straight line whose square is equal to the rectangle PQRD, either increased by the curvilinear area DFge (if place e is lower than place D) or diminished by DFge (if place e is higher), is to the straight line whose square is equal to the rectangle PQRD alone.
COROLLARY 3. The time, also, will be determined by erecting the ordinate em inversely proportional to the square root of PQRD ± DFge, and by taking the time in which the body described the line De to the time in which the other body fell under the action of a uniform force from P and (by so falling) reached D as the curvilinear area DLme to the rectangle 2PD × DL. For the time in which the body descending under the action of a uniform force described the line PD is to the time in which the same body described the line PE as the square root of the ratio of PD to PE, that is (the line-element DE being just now nascent), in the ratio of PD to PD + ½DE or 2PD to 2PD + DE and by separation [or dividendo] to the time in which the same body described the line-element DE as 2PD to DE, and thus as the rectangle 2PD × DL to the area DLME; and the time in which each of the two bodies described the line-element DE is to the time in which the second body with nonuniform motion described the line De as the area DLME to the area DLme, and from the equality of the ratios [or ex aequo] the first time is to the ultimate time as the rectangle 2PD × DL to the area DLme.
To find the orbits in which bodies revolve when acted upon by any centripetal forces
Proposition 40, Theorem 13
If a body, under the action of any centripetal force, moves in any way whatever, and another body ascends straight up or descends straight down, and if their velocities are equal in some one instance in which their distances from the center are equal, their velocities will be equal at all equal distances from the center.
Let some body descend from A through D and E to the center C, and let another body move from V in the curved line VIKk. With center C and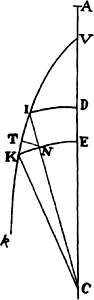 
any radii describe the concentric circles DI and EK meeting the straight line AC in D and E and the curve VIK in I and K. Join IC meeting KE in N, and to IK drop the perpendicular NT, and let the interval DE or IN between the circumferences of the circles be minimally small, and let the bodies have equal velocities at D and I. Since the distances CD and CI are equal, the centripetal forces at D and I will be equal. Represent these forces by the equal line-elements DE and IN; then, if one of these forces IN is (by corol. 2 of the laws) resolved into two, NT and IT, the force NT, acting along the line NT perpendicular to the path ITK of the body, will in no way change the velocity of the body in that path but will only draw the body back from a rectilinear path and make it turn aside continually from the tangent of the orbit and move forward in the curvilinear path ITKk. That whole force will be spent in producing this effect, while the whole of the other force IT, acting along the body’s path, will accelerate the body and in a given minimally small time will generate an acceleration proportional to itself. Accordingly, the accelerations of the bodies at D and I that are made in equal times (if the first ratios of the nascent lines DE, IN, IK, IT, and NT are taken) are as the lines DE and IT, but in unequal times they are as those lines and the times jointly. Now, the times in which DE and IK are described are as the described paths DE and IK (because the velocities are equal), and hence the accelerations in the path of the bodies along the lines DE and IK are jointly as DE and IT, DE and IK, that is, as DE2 and the rectangle IT × IK. But the rectangle IT × IK is equal to IN2, that is, equal to DE2, and therefore the accelerations generated in the passing of the bodies from D and I to E and K are equal. Therefore the velocities of the bodies at E and K are equal, and by the same argument they will always be found equal at subsequent equal distances. Q.E.D.
But also by the same argument bodies that have equal velocities and are equally distant from the center will be equally retarded in ascending to equal distances. Q.E.D.
COROLLARY 1. Hence if a body either oscillates while hanging by a thread or is compelled by any very smooth and perfectly slippery impediment to move in a curved line, and another body ascends straight up or descends straight down, and their velocities are equal at any identical height, their velocities at any other equal heights will be equal. For the thread of the pendent body or the impediment of an absolutely slippery vessel produces the same effect as the transverse force NT. The body is neither retarded nor accelerated by these, but only compelled to depart from a rectilinear course.
COROLLARY 2. Now let the quantity P be the greatest distance from the center to which a body, either oscillating or revolving in any trajectory whatever, can ascend when projected upward from any point of the trajectory with the velocity that it has at that point. Further, let the quantity A be the distance of the body from the center at any other point of the orbit. And let the centripetal force be always as any power An−1 of A, the index n − 1 being any number n diminished by unity. Then the velocity of the body at every height A [i.e., distance A] will be as √(Pn − An) and therefore is given. For the velocity of a body ascending straight up and descending straight down is (by prop. 39) in this very ratio.
Proposition 41a, Problem 28
Supposing a centripetal force of any kind and granting the quadratures of curvilinear figures, it is required to find the trajectories in which bodies will move and also the times of their motions in the trajectories so found.
Let any force tend toward a center C; and let it be required to find the trajectory VIKk. Let the circle VR be given, described about the center C with any radius CV; and about the same center let there be described any other circles ID and KE cutting the trajectory in I and K and cutting the straight line CV in D and E. Then draw the straight line CNIX cutting the circles KE and VR in N and X, and also draw the straight line CKY meeting the circle VR in Y. Let the points I and K be very close indeed to each other, and let the body proceed from V through I and K to k; and let point A be the place from which another body must fall so as to acquire at place D a velocity equal to the velocity of the first body at I. And with everything remaining as it was in prop. 39, the line-element IK, described in a given minimally small time, will be as the velocity and hence as the straight line whose square equals the area ABFD, and the triangle ICK proportional to the time will be given; and therefore KN will be inversely as the height IC, that is, if some quantity Q is given and the height IC is called A, as 
. Let us denote this quantity by Z, and let us suppose the magnitude of Q to be such that in some one case √ABFD is to Z as IK is to KN, and in every case √ABFD will be to Z as IK to KN and ABFD to Z2 as IK2 to KN2, and by separation [or dividendo] ABFD − Z2 will be to Z2 as IN2 to KN2, and therefore √(ABFD − Z2) will be to Z, or , as IN to KN, and therefore A × KN will be equal to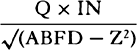 
. Hence, since YX × XC is to A × KN as CX2 to A2, the rectangle XY × XC will be equal to 
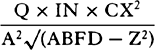. Therefore, in the perpendicular DF take Db and Dc always equal respectively to 
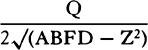and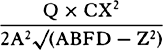 
, and describe the curved lines ab and ac which the points b and c continually trace out, and from point V erect Va perpendicular to the line AC so as to cut off the curvilinear areas VDba and VDca, and also erect the ordinates Ez and Ex. Then, since the rectangle Db × IN or DbzE is equal to half of the rectangle A × KN or is equal to the triangle ICK, and the rectangle Dc × IN or DcxE is equal to half of the rectangle YX × XC or is equal to the triangle XCY—that is, since the nascent particles DbzE and ICK of the areas VDba and VIC are always equal, and the nascent particles DcxE and XCY of the areas VDca and VCX are always equal—the generated area VDba will be equal to the generated area VIC and hence will be proportional to the time, and the generated area VDca will be equal to the generated sector VCX. Therefore, given any time that has elapsed since the body set out from place V, the area VDba proportional to it will be given and hence the body’s height CD or CI will be given, and the area VDca and, equal to that area, the sector VCX along with its angle VCI. And given the angle VCI and the height CI, the place I will be given, in which the body will be found at the end of that time. Q.E.I.
COROLLARY 1. Hence the greatest and least heights of bodies (that is, the apsides of their trajectories) can be found expeditiously. For the apsides are those points in which the straight line IC drawn through the center falls perpendicularly upon the trajectory VIK, which happens when the straight lines IK and NK are equal, and thus when the area ABFD is equal to Z2.
COROLLARY 2. The angle KIN, in which the trajectory anywhere cuts the line IC, is also expeditiously found from the given height IC of the body, namely, by taking its sine to the radius as KN to IK, that is, as Z to the square root of the area ABFD.
COROLLARY 3. If with center C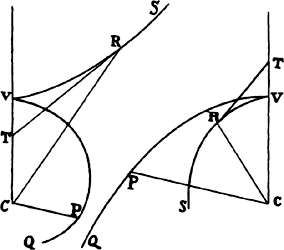 
and principal vertex V any conic VRS is described, and from any point R of it the tangent RT is drawn so as to meet the axis CV, indefinitely produced, at point T; and, joining CR, there is drawn the straight line CP, which is equal to the abscissa CT and makes an angle VCP proportional to the sector VCR; then, if a centripetal force inversely proportional to the cube of the distance of places from the center tends toward that center C, and the body leaves the place V with the proper velocity along a line perpendicular to the straight line CV, the body will move forward in the trajectory VPQ which point P continually traces out; and therefore, if the conic VRS is a hyperbola, the body will descend to the center. But if the conic is an ellipse, the body will ascend continually and will go off to infinity.
And conversely, if the body leaves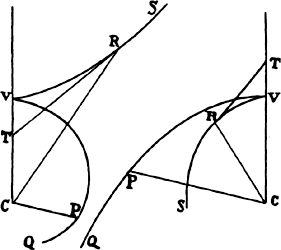 
the place V with any velocity and, depending on whether the body has begun either to descend obliquely to the center or to ascend obliquely from it, the figure VRS is either a hyperbola or an ellipse, the trajectory can be found by increasing or diminishing the angle VCP in some given ratio. But also, if the centripetal force is changed into a centrifugal force, the body will ascend obliquely in the trajectory VPQ, which is found by taking the angle VCP proportional to the elliptic sector VRC and by taking the length CP equal to the length CT, as above. All this follows from the foregoing (prop. 41), by means of the quadrature of a certain curve, the finding of which, as being easy enough, I omit for the sake of brevity.
Proposition 42, Problem 29
Let the law of centripetal force be given; it is required to find the motion of a body setting out from a given place with a given velocity along a given straight line.
With everything remaining as it was in the three preceding propositions, let the body go forth from the place I along the line-element IK, with the velocity which another body, falling from the place P under the action of some uniform centripetal force, could acquire at D; and let this uniform force be to the force with which the first body is urged at I as DR to DF. Let the body go on toward k; and with center C and radius Ck describe the circle ke meeting the straight line PD at e, and erect the ordinates eg, ev, and ew of the curves BFg, abv, and acw. From the given rectangle PDRQ and the given law of the centripetal force acting on the first body, the curved line BFg is given by the construction of prop. 39 and its corol. 1. Then, from the given angle CIK, the proportion of the nascent lines IK and KN is given, and hence, by the construction of prop. 41, the quantity Q is given, along with the curved lines abv and acw; and therefore, when any time Dbve is completed, the body’s height Ce or Ck is given and the area Dcwe and the sector XCy equal to it and the angle ICk and the place k in which the body will then be. Q.E.I.
In these propositions we suppose that the centripetal force in receding from the center varies according to any law which can be imagined, but that at equal distances from the center it is everywhere the same. And so far we have considered the motion of bodies in nonmoving orbits. It remains for us to add a few things about the motion of bodies in orbits that revolve about a center of forces.
The motion of bodies in mobile orbits, and the motion of the apsides
Proposition 43, Problem 30
aIt is required to find the force that makes a body capable of moving in any trajectory that is revolving about the center of forces in the same way as another body in that same trajectory at rest.a
Let a body P revolve in the orbit VPK given in position, moving forward from V toward K. From center C continually draw Cp equal to CP 
and making the angle VCp which is proportional to the angle VCP; and the area that the line Cp describes will be to the area VCP that the line CP simultaneously describes as the velocity of the describing line Cp to the velocity of the describing line CP, that is, as the angle VCp to the angle VCP and thus in a given ratio and therefore proportional to the time. Since the area that line Cp describes in the immobile plane is proportional to the time, it is manifest that the body, under the action of a centripetal force of just the right quantity, can revolve along with point p in the curved line that the same point p, in the manner just explained, describes in an immobile plane. Let the angle VCu be made equal to the angle PCp, and the line Cu equal to the line CV, and the figure uCp equal to the figure VCP; then the body, being always at the point p, will move in the perimeter of the revolving figure uCp, and will describe its arc up in the same time in which another body P can describe the arc VP, similar and equal to up, in the figure VPK at rest. Determine, therefore, by prop. 6, corol. 5, the centripetal force by which a body can revolve in the curved line that point p describes in an immobile plane, and the problem will be solved. Q.E.F.
Proposition 44, Theorem 14
The difference between the forces under the action of which two bodies are able to move equally—one in an orbit that is at rest and the other in an identical orbit that is revolving—is inversely as the cube of their common height.
Let the parts up and pk of the revolving orbit be similar and equal to the parts VP and PK of the orbit at rest; and let it be understood that the distance between points P and K is minimally small. From point k drop the perpendicular kr to the straight line pC, and produce kr to m so that mr is to kr as the angle VCp to the angle VCP. Since the heights PC and pC, KC and kC, of the bodies are always equal, it is manifest that the increments and decrements of the lines PC and pC are always equal, and hence, if the motions of each of these bodies, when they are at places P and p, are resolved (by corol. 2 of the laws) into two components, of which one is directed toward the center, or along the line PC or pC, and the second is transverse to the first and has a direction along a line perpendicular to PC or pC, the components of motion toward the center will be equal, and the transverse component of motion of body p will be to the transverse component of motion of body P as the angular motion of line pC to the angular motion of line PC, that is, as the angle VCp to the angle VCP. Therefore, in the same time in which body P by the two components of its motion reaches point K, body p by its equal component of motion toward the center will move equally from p toward C and thus, when that time is completed, will be found somewhere on the line mkr (which is perpendicular to the line pC through point k) and by its transverse motion will reach a distance from the line pC that is to the distance from the line PC (which the other body P reaches) as the transverse motion of body p is to the transverse motion of the other body P. Therefore, since kr is equal to the distance from the line PC which body P reaches, and since mr is to kr as the angle VCp to the angle VCP, that is, as the transverse motion of body p to the transverse motion of body P, it is manifest that body p, at the completion of the time, will be found at the place m.
This will be the case when bodies p and P move equally along lines pC and PC and thus are urged along those lines by equal forces. But now, take the angle pCn to the angle pCk as the angle VCp is to the angle VCP, and let nC be equal to kC, and then body p—at the completion of the time—will actually be found at the place n; and thus body p is urged by a greater force than that by which body P is urged, provided that the angle nCp is greater than the angle kCp, that is, if the orbit upk either moves forward [or in consequential or moves backward [or in antecedentia] with a speed greater than twice that with which the line CP is carried forward [or in consequential and it is urged by a smaller force if the orbit moves backward [or in antecedentia] more slowly. And the difference between the forces is as the intervening distance mn through which the body p ought to be carried by the action of that difference in the given space of time.
Understand that a circle is described, with center C and radius Cn or Ck, cutting in s and t the lines mr and mn produced; then the rectangle mn × mt will be equal to the rectangle mk × ms, and thus mn will be equal to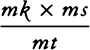 
. But since the triangles pCk and pCn are, in a given time, given in magnitude, kr and mr and their difference mk and sum ms are inversely as the height pC, and thus the rectangle mk × ms is inversely as the square of the height pC. Also, mt is directly as ½mt, that is, as the height pC. These are the first ratios of the nascent lines; and hence 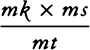(that is, the nascent line-element mn and, proportional to it, the difference between the forces) becomes inversely as the cube of the height pC. Q.E.D.
COROLLARY 1. Hence the difference of the forces in the places P and p or K and k is to the force by which a body would be able to revolve with circular motion from R to K in the same time in which body P in an immobile orbit describes the arc PK as the nascent line-element mn is to the versed sine of the nascent arc RK, that is, as 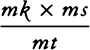to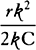 
or as mk × ms to rk2, that is, if the given quantities F and G are taken in the ratio to each other that the angle VCP has to the angle VCp, as G2 − F2 to F2. And therefore, if with center C and any radius CP or Cp a circular sector is described equal to the total area VPC which the body P revolving in an immobile orbit has described in any time by a radius drawn to the center, the difference between the forces by which body P in an immobile orbit and body p in a mobile orbit revolve will be to the centripetal force by which some body, by a radius drawn to the center, would have been able to describe that sector uniformly in the same time in which the area VPC was described, as G2 − F2 to F2. For that sector and the area pCk are to each other as the times in which they are described.
COROLLARY 2. If the orbit VPK is an ellipse having a focus C and an upper apsis V, and the mobile ellipse upk is supposed similar and equal to it, so that pC is always equal to PC and the angle VCp is to the angle VCP in the given ratio of G to F; and if A is written for the height PC or pC, and 2R is put for the latus rectum of the ellipse; then the force by which a body can revolve in the mobile ellipse will be as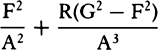 
, and conversely. For let the force by which a body revolves in the unmoving ellipse be represented by the quantity 
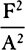, and then the force at V will be 
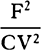. But the force by which a body could revolve in a circle at the distance CV with the velocity that a body revolving in an ellipse has at V is to the force by which a body revolving in an ellipse is urged at the apsis V as half of the latus rectum of the ellipse to the semidiameter CV of the circle, and thus has the value 
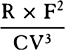; and the force that is to this as G2 − F2 to F2 has the value 
; and this force (by corol. 1 of this prop.) is the difference between the forces at V by which body P revolves in the unmoving ellipse VPK and body p revolves in the mobile ellipse upk. Hence, since (by this proposition) that difference at any other height A is to itself at the height CV as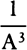 
to 
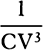, the same difference at every height A will have the value 
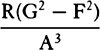. Therefore, add the excess 
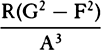to the force 
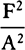by which a body can revolve in the immobile ellipse VPK, and the result will be the total force 
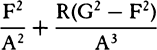by which a body may be able to revolve in the same times in the mobile ellipse upk.
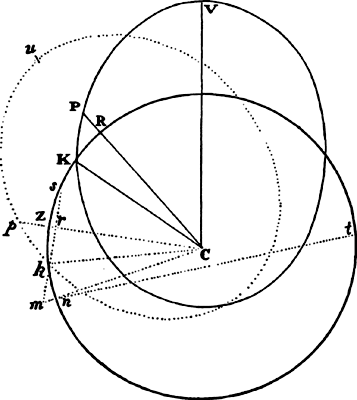
COROLLARY 3. In the same way it will be gathered that if the immobile orbit VPK is an ellipse having its center at the center C of forces, and a mobile ellipse upk is supposed similar, equal, and concentric with it; and if 2R is the principal latus rectum of this ellipse, and 2T the principal diameter or major axis, and the angle VCp is always to the angle VCP as G to F; then the forces by which bodies can revolve in equal times in the immobile ellipse and the mobile ellipse will be as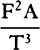 
and 
respectively.
COROLLARY 4. And universally, if the greatest height CV of a body is called T; and the radius of the curvature which the orbit VPK has at V (that is, the radius of a circle of equal curvature) is called R; and the centripetal force by which a body can revolve in any immobile trajectory VPK at place V is called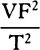 
and at other places P is indefinitely styled X, while the height CP is called A; and if G is taken to F in the given ratio of the angle VCp to the angle VCP; then the centripetal force by which the same body can complete the same motions in the same times in the same trajectory upk which is moving circularly will be as the sum of the forces 
.
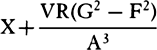COROLLARY 5. Therefore, given the motion of a body in any immobile orbit, its angular motion about the center of forces can be increased or diminished in a given ratio, and hence new immobile orbits can be found in which bodies may revolve by new centripetal forces.
COROLLARY 6. Therefore, if on the straight line CV, given in position, there is erected the perpendicular VP of indeterminate length, and CP is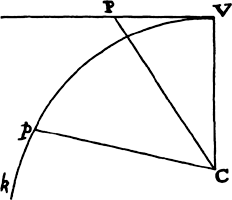 
joined, and Cp is drawn equal to it making the angle VCp that is to the angle VCP in a given ratio; then the force by which a body can revolve in the curve Vpk which the point p continually traces out will be inversely as the cube of the height Cp. For body P, by its own force of inertia, and with no other force urging it, can move forward uniformly in the straight line VP. Add the force toward the center C, inversely proportional to the cube of the height CP or Cp, and (by what has just been demonstrated) the rectilinear motion will be bent into the curved line Vpk. But this curve Vpk is the same as the curve VPQ found in prop. 41, corol. 3, and (as we said there) bodies attracted by forces of this kind ascend obliquely in this curve.
, that is, that A and 
are to each other in a given ratio.c
about a center S, describes the curved line APQ, while the straight line ZPR touches the curve at any point P; and QR, parallel to distance SP, is drawn to the tangent from any other point Q of the curve, and QT is drawn perpendicular to that distance SP; then the centripetal force will be inversely as the solid 
, provided that the magnitude of that solid is always taken as that which it has ultimately when the points P and Q come together. For QR is equal to the sagitta of an arc that is twice the length of arc QP, with P being in the middle; and twice the triangle SQP (or SP × QT) is proportional to the time in which twice that arc is described and therefore can stand for the time.
, provided that SY is a perpendicular dropped from the center of forces to the tangent PR of the orbit. For the rectangles SY × QP and SP × QT are equal.
.
or the solid SY2 × PV, inversely proportional to this force, is to be found by computation. We will give examples of this in the following problems.b