
Some Philosophical Questions

HE DID NOT KNOW what he wanted to be or do, but it was not tend sheep or follow the plow and the dung cart. He spent more time gathering herbs and lying with a book among the asphodel and moonwort, out of the household’s sight.1 He built waterwheels in the stream while his sheep trampled the neighbors’ barley. He watched the flow of water, over wood and around rocks, noting the whorls and eddies and waves, gaining a sense of fluid motion.2 He defied his mother and scolded his half-sisters.3 He was fined in the manor court for allowing his swine to trespass and his fences to lie in disrepair.4
His Grantham schoolmaster, Stokes, and his mother’s brother, the rector William Ayscough, finally intervened. Ayscough had prepared for the clergy at the College of the Holy and Undivided Trinity, the greatest of the sixteen colleges at the University of Cambridge, so they arranged for Isaac to be sent there. He made the journey south, three days and two nights, and was admitted in June 1661. Cambridge recognized students in three categories: noblemen, who dined at high table, wore sophisticated gowns, and received degrees with little examination; pensioners, who paid for tuition and board and aimed, mainly, for the Anglican ministry; and sizars, who earned their keep by menial service to other students, running errands, waiting on them at meals, and eating their leftovers. The widowed Hannah Smith was wealthy now, by the standards of the countryside, but chose to provide her son little money; he entered Trinity College as a subsizar. He had enough for his immediate needs: a chamber pot; a notebook of 140 blank pages, three and a half by five and a half inches, with leather covers; “a quart bottle and ink to fill it”; candles for many long nights, and a lock for his desk.5 For a tutor he was assigned an indifferent scholar of Greek. Otherwise he kept to himself.
He felt learning as a form of obsession, a worthy pursuit, in God’s service, but potentially prideful as well. He taught himself a shorthand of esoteric symbols—this served both to save paper and encrypt his writing—and he used it, at a moment of spiritual crisis, to record a catalogue of his sins. Among them were neglecting to pray, negligence at the chapel, and variations on the theme of falling short in piety and devotion. He rebuked himself for a dozen ways of breaching the Sabbath. On one Sunday he had whittled a quill pen and then lied about it. He confessed uncleane thoughts words and actions and dreamese. He regretted, or tried to regret, setting my heart on money learning pleasure more than Thee.6 Money, learning, pleasure: three sirens calling his heart. Of these, neither money nor pleasure came in abundance.
The Civil War had ended and so had the Protectorate of Oliver Cromwell, dead from malaria, buried and then exhumed so his head could be stuck on a pole atop Westminster Hall. During the rebellion Puritan reformers had gained control of Cambridge and purged the colleges of many Royalist scholars. Now, with the restoration of Charles II to the crown, Puritans were purged, Cromwell was hanged in effigy, and the university’s records from the Protectorate years were burned. This riverside town was a place of ferment, fifty miles from London, a hundredth its size, a crossroads for information and commerce. Each year between harvest and plowing, tradesmen gathered for Stourbridge Fair, England’s largest: a giant market for wool and hops, metal-ware and glass-ware, silk and stationery, books, toys, and musical instruments—a bedlam of languages and apparel, and “an Abstract of all sorts of mankind,” as a pamphleteer described it.7 Newton, scrupulous with his limited funds, bought books there and, one year, a glass prism—a toy, imprecisely ground, flawed with air bubbles. Often enough, the complex human traffic had another consequence: Cambridge suffered visitations of plague.
The curriculum had grown stagnant. It followed the scholastic tradition laid down in the university’s medieval beginnings: the study of texts from disintegrated Mediterranean cultures, preserved in Christian and Islamic sanctuaries through a thousand years of European upheaval. The single authority in all the realms of secular knowledge was Aristotle—doctor’s son, student of Plato, and collector of books. Logic, ethics, and rhetoric were all his, and so—to the extent they were studied at all—were cosmology and mechanics. The Aristotelian canon enshrined systematization and rigor, categories and rules. It formed an edifice of reason: knowledge about knowledge. Supplemented by ancient poets and medieval divines, it was a complete education, which scarcely changed from generation to generation. Newton began by reading closely, but not finishing, the Organon and the Nicomachean Ethics (“For the things we have to learn before we can do them, we learn by doing them”).8
He read Aristotle through a mist of changing languages, along with a body of commentary and disputation. The words crossed and overlapped. Aristotle’s was a world of substances. A substance possesses qualities and properties, which taken together amount to a form, depending ultimately on its essence. Properties can change; we call this motion. Motion is action, change, and life. It is an indispensable partner of time; the one could not exist without the other. If we understood the cause of motion, we would understand the cause of the world.
For Aristotle motion included pushing, pulling, carrying, and twirling; combining and separating; waxing and waning. Things in motion included a peach ripening, a fish swimming, water warming over a fire, a child growing into an adult, an apple falling from a tree.9 The heavy thing and the light thing move to their proper positions: the light thing up and the heavy thing down.10 Some motion is natural; some violent and unnatural. Both kinds revealed the connections between things. “Everything that is in motion must be moved by something,” Aristotle asserted (and proved, by knotted logic).11 A thing cannot be at once mover and moved. This simple truth implied a first mover, put in motion by no other, to break what must otherwise be an infinite loop:
Since everything that is in motion must be moved by something, let us take the case in which a thing is in locomotion and is moved by something that is itself in motion,… and that by something else, and so on continually: then the series cannot go on to infinity, but there must be some first mover.
To the Christian fathers, this first mover could only be God. It was a testament to how far pure reason could take a philosopher; and to how involuted and self-referential a chain of reasoning could become, with nothing to feed on but itself.
This all-embracing sense of motion left little place for quantity, measurement, and number. If objects in motion could include a piece of bronze becoming a statue,12 then philosophers were not ready to make fine distinctions, like the distinction between velocity and acceleration. Indeed, the Greeks had a principled resistance to mathematicizing our corruptible, flawed, sublunary world. Geometry belonged to the celestial sphere; it might relate music and the stars, but projectiles of rock or metal were inappropriate objects for mathematical treatment. So technology, advancing, exposed Aristotelian mechanics as quaint and impotent. Gunners understood that a cannonball, once in flight, was no longer moved by anything but a ghostly memory of the explosion inside the iron barrel; and they were learning, roughly, to compute the trajectories of their projectiles. Pendulums, in clockwork, however crude, demanded a mathematical view of motion. And in turn the clockwork made measurement possible—first hours, then minutes. Of an object falling from a tower or rolling down an inclined plane, people could begin to ask: what is the distance? what is the time?
What, therefore, is the velocity? And how does the velocity, itself, change?
Nor was Aristotle’s cosmology faring well outside Cambridge’s gates. It was harmonious and immutable: crystalline spheres round the earth, solid and invisible, carrying the celestial orbs within them. Ptolemy had perfected his universe and then, for hundreds of years, Christian astronomers embraced and extended it, reconciled it with biblical scripture, and added a heaven of heavens, deep and pure, perhaps infinite, the home of God and angels, beyond the sphere of fixed stars. But as stargazers made increasingly detailed notations, they catalogued planetary motions too irregular for concentric spheres. They saw freaks and impurities, such as comets glowing and vanishing. By the 1660s—new news every day—readers of esoterica knew well enough that the earth was a planet and that the planets orbited the sun. Newton’s notes began to include measurements of the apparent magnitude of stars.
Although the library of Trinity College had more than three thousand books, students could enter only in the company of a fellow. Still, Newton found his way to new ideas and polemics: from the French philosopher René Descartes, and the Italian astronomer Galileo Galilei, who had died in the year of Newton’s birth. Descartes proposed a geometrical and mechanical philosophy. He imagined a universe filled throughout with invisible substance, forming great vortices that sweep the planets and stars forward. Galileo, meanwhile, applied geometrical thinking to the problem of motion. Both men defied Aristotle explicitly—Galileo by claiming that all bodies are made of the same stuff, which is heavy, and therefore fall at the same rate.
Not the same speed, however. After long gestation, Galileo created a concept of uniform acceleration. He considered motion as a state rather than a process. Without ever using a word such as inertia, he nonetheless conceived that bodies have a tendency to remain in motion or to remain motionless. The next step demanded experiment and measure. He measured time with a water-clock. He rolled balls down ramps and concluded, wrongly, that their speed varied in proportion to the distance they rolled. Later, trying to understand free fall, he reached the modern definition, correctly assimilating units of distance, units of speed, and units of time. Newton began to absorb this, at second or third hand; Galileo had written mostly in Italian, a language few in England could read.13
In Newton’s second year, having filled the beginning and end of his notebook with Aristotle, he started a new section deep inside: Questiones quædam philosophicæ—some philosophical questions. He set authority aside. Later he came back to this page and inscribed an epigraph borrowed from Aristotle’s justification for dissenting from his teacher. Aristotle had said, “Plato is my friend, but truth my greater friend.” Newton inserted Aristotle’s name in sequence: Amicus Plato amicus Aristoteles magis amica veritas.14 He made a new beginning. He set down his knowledge of the world, organized under elemental headings, expressed as questions, based sometimes on his reading, sometimes on speculation. It showed how little was known, altogether. The choice of topics—forty-five in all—suggested a foundation for a new natural philosophy.
Of the First Matter. Of Atoms. Could he know, by the force of logic, whether matter was continuous and infinitely divisible, or discontinuous and discrete? Were its ultimate parts mathematical points or actual atoms? Since a mathematical point lacks body or dimension—“is but an imaginary entity”—it seemed implausible that even an infinite number of them could combine to form matter with real extension,15 even if bits of vacuum (“interspersed inanities”) separated the parts. The question of God’s role, as creator, could be dangerous territory. “Tis a contradiction to say the first matter depends on some other subject”—in parentheses he added, “except God”; then, on second thought, he crossed that out—“since that implies some former matter on which it must depend.” Reasoning led him, as it had led ancient Greeks, to atoms—not by observation or experiment, but by eliminating alternatives. Newton declared himself a corpuscularian and an atomist. “The first matter must be attoms. And that Matter may be so small as to be indiscernible.” Very small, but finite, not zero. Indiscernible, but unbreakable and indivisible. This was an unsettled conception, because Newton also saw a world of smooth change, of curves, and of flow. What about the smallest parts of time and motion? Were these continuous or discrete?
Quantity. Place. “Extension is related to places, as time to days yeares &c.”16 He invoked God on another controversial question: Is space finite or infinite? Not the imaginary abstract space of geometers, but the real space in which we live. Infinite, surely! “To say that extension is but indefinite”—Descartes said this, in fact—“is as much to say God is but indefinitely perfect because wee cannot apprehend his whole perfection.”
Time and Eternity. No abstract disputation here; he just sketched a wheel-shaped clock, to be driven by water or sand, and raised wholly practical questions about making clocks with various materials, such as “metalline globular dust.” Only then did he reach Motion, and again, he began by looking for the root constituents, the equivalent of atoms. Motion led to Celestiall Matter & Orbes—which took Newton, encountering the early echoes of Continental thought, to Descartes. In Descartes’s universe, there could be no vacuum, for the universe was space, and space meant extension, and extension surely implied substance. Also, the world’s principles were mechanical: all action propagated through contact, one object directly pushing another, no mystical influences from afar.
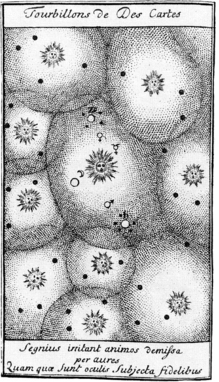
In the cosmos of Descartes, matter fills all space and forms whirling vortices. (illustration credit 2.1)
So a vacuum could not transmit light. Light was a form of pression, Descartes said—imaginatively, because philosophers had barely begun to conceive of pressure as a quality that an invisible fluid, the air, could possess. But now Newton had heard of Robert Boyle’s experiments with an air-pump, and pressure was the word Boyle used in this new sense. Newton began again:
Whether Cartes his first element can turne about the vortex & yet drive the matter of it continually from the  [sun] to produce light, & spend most of its motion in filling up the chinks between the globuli.17
[sun] to produce light, & spend most of its motion in filling up the chinks between the globuli.17
From matter to motion, to light, and to the structure of the cosmos. The sun drove the vortex by its beams. The ubiquitous vortex could drive anything: Newton sketched some ideas for perpetual motion machines. But light itself played a delicate part in the Cartesian scheme, and Newton, attempting to take Descartes literally, already sensed contradictions. Pressure does not restrict itself to straight lines; vortices whirl around corners. “Light cannot be by pression,” Newton asserted, “for then wee should see in the night a[s] wel or better than in the day we should se[e] a bright light above us becaus we are pressed downewards.…” Eclipses should never darken the sky. “A man goeing or running would see in the night. When a fire or candle is extinguished we lookeing another way should see a light.”18
Another elusive word, gravity, began to appear in the Questiones. Its meanings darted here and there. It served as half of a linked pair: Gravity & Levity. It represented the tendency of a body to descend, ever downward. But how could this happen? “The matter causing gravity must pass through all the pores of a body. It must ascend againe, for else the bowells of the earth must have had large cavitys & inanitys to containe it in.…”19 It must be crowded in that unimaginable place, the center of the earth—all the world’s streams coming home. “When the streames meet on all sides in the midst of the Earth they must needs be coarcted into a narrow roome & closely press together.”
Then again, perhaps an object’s gravity was inherent, a quantity to be exactly measured, even if it varied from place to place: “The gravity of a body in diverse places as at the top and bottom of a hill, in different latitudes &c. may be measured by an instrument”—he sketched a balance scale. He speculated about “rays of gravity.” Then, gravity could also refer to a body’s tendency to move, not downward, but in any direction; its tendency to remain in motion, once started. If such a tendency existed, no language yet had a word for it. Newton considered the problem of the cannonball, still rising, long after leaving the gun. “Violent motion is made”—he struck the word made—“continued either by the aire or by motion”—struck the word motion and replaced it with force:

Violent motion (Newton’s drawing). (illustration credit 2.2)
Violent motion is made continued either by the aire or by motion force imprest or by the natural gravity in the body moved.
Yet how could the cannonball be helped along by the air? He noted that the air crowds more upon the front of a projectile than on the rear, “& must therefore rather hinder it.” So the continuing motion must come from some natural tendency in the object. But—gravity?
Some of his topics—for example, Fluidity Stability Humidity Siccity20—never progressed past a heading. No matter. He had set out his questions. Of Heate & Cold. Atraction Magneticall. Colours. Sounds. Generation & Coruption. Memory. They formed a program, girded with measurements, clocks and scales, experiments both practical and imaginary. Its ambition encompassed the whole of nature.
One more mystery: the Flux & Reflux of the Sea. He considered a way to test whether the moon’s “pressing the atmosphere” causes the tides. Fill a tube with mercury or water; seal the top; “the liquor will sink three or four inches below it leaving a vacuum (perhaps)”; then as the air is pressed by the moon, see if the water will rise or fall. He wondered whether the sea level rose by day and fell by night; whether it was higher in the morning or evening. Though fishermen and sailors around the globe had studied the tides for thousands of years, people had not amassed enough data to settle those questions.21