

CHARLES MASON, with his expertise in astronomy and mathematics, directed the duo’s work during the early weeks of the boundary-line mission. Dixon’s expertise as a surveyor took center stage as the focus shifted from the stars to the ground. But before Dixon could begin his work, he needed a vista — a clear line of sight — along the meridian he planned to follow south. Within forty-eight hours of the lunar eclipse, four axmen, wood chips flying, steadily cut a “visto in the Meridian Southward.”
Surveying across the land required using different instruments from those Mason had used so far. The zenith sector, perfect for measuring angles on the celestial sphere, couldn’t sight or measure land distances. Instead, Dixon used a circumferentor, an instrument that looks like a large compass with built-in leveling bubbles and two upright bars used to sight distant objects. A circumferentor indicates direction (north, south, east, and west) and can measure horizontal angles that are necessary for accurate land surveys. The land survey also required the addition of a few new crew members, all with previous surveying experience.
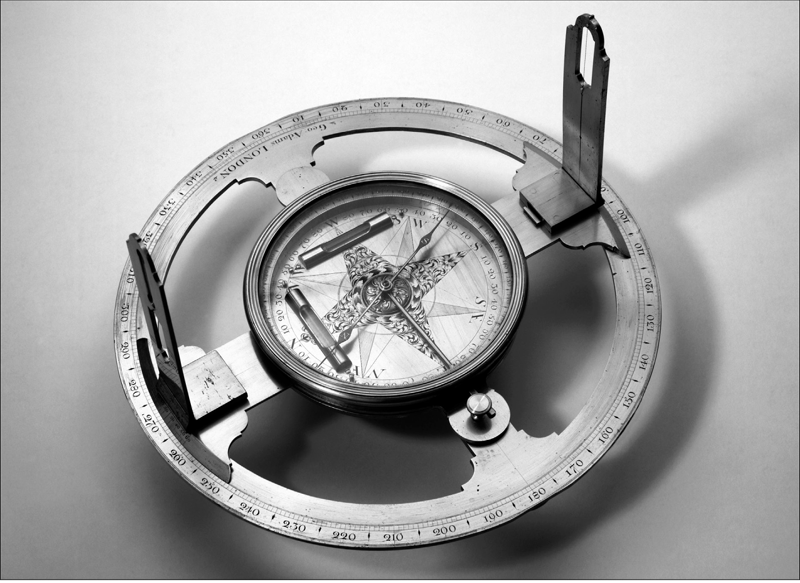
Dixon used this circumferentor to keep the chain crew on course as they surveyed south from Harlan’s garden. The instrument in the middle is a compass, and the two liquid-filled tubes are levels.
In Harlan’s garden, outside the observatory, Dixon aligned his circumferentor with the meridian. A crewman stood several hundred feet away, holding a wooden stake. Dixon peered through the instrument’s sighting slits (one on each upright bar) and signaled the crewman to move right or left until the stake was aligned with the instrument’s sights. Aligning the crewman with the two sights ensured a straight line. After setting the stake in place, it was time to haul out the chain — a two-man job.
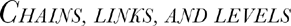
Under Dixon’s direction, two chain carriers measured a distance from the circumferentor with a surveyor’s tool called a Gunter’s chain. This sixty-six-foot-long metal chain consists of one hundred links, each of which is 7.92 inches long. A link of a Gunter’s chain is formed from a straight metal bar. Each end of the bar is bent to form a small circle. Small measured metal circles connect the links. Eighty chains equal one mile (5,280 feet). One chain carrier firmly anchored the end of the chain at the starting point outside the observatory. The other chain carrier unfolded the chain as he walked toward the distant wooden stake. When the chain was completely extended, he stopped walking and stretched the chain taut. On the ground, he marked the spot beneath the end of the chain with an iron pin. This completed one chain.
A Gunter’s chain was the chain bearers’ constant companion. From time to time, the circles connecting the links became stretched out and had to be reshaped to keep the length of the chain accurate.
Keeping a tally, Dixon and the chain carriers repeated these steps until they reached the distant wooden stake or a land feature that created an obstacle, such as a creek. At 9 chains and 61 links (634 feet 3.12 inches) from Harlan’s garden, the crew reached the sloped bank of Brandywine Creek. Surveying up or down a slope with a chain is difficult, if not impossible. Plus, measuring directly along the ground up and down short, steep slopes would have considerably shortened how far south the fifteen-mile line extended, something the Pennsylvania commissioners vociferously opposed. Dixon’s men replaced the Gunter’s chain with a wooden rod called a level that was twenty-two feet long.
To measure the distance from the top of the creek bank to the water’s edge as a straight rather than sloped line, two chain carriers held a level, one man at each end, so that neither end was higher or lower than the other. Two more crewmen stepped into place, holding one end of a second level directly beneath the front end of the first level. As the crewmen repeated this process, Dixon and his circumferentor kept them on course by aligning the men with a stake placed on the meridian across the creek. It took the crew four levels, or steps, to reach the edge of Brandywine Creek closest to Harlan’s house.
Brandywine Creek loops around near the Harlan house. Before Dixon’s men had surveyed a mile, Mason noted that they’d splashed across the creek three times. That meant the crewmen had to work in sopping-wet leather shoes or boots and wet wool pants, which may not have dried out during the day, due to chilly air temperatures. Most colonists owned few changes of clothes and only one pair of shoes; changing outfits frequently was not an option. So the waders squelched when they walked, and their legs itched as wet wool chafed their skin. A long hike in these conditions was uncomfortable, as was using the difficult-to-maneuver twenty-two-foot-long levels. After crossing Brandywine Creek once, the crew switched to less cumbersome levels that were only sixteen and a half feet long. As they proceeded south, the men became an efficient and precise survey team.
Periodically, Mason observed the stars to confirm that the line was still true to the meridian of Harlan’s garden (or to determine how the line needed to be shifted if it had veered off course). On April 5, he noted with satisfaction that he had “Proved the Meridian and found it very exact.” In addition to checking their course, Mason and Dixon also had to check their Gunter’s chain. Repeatedly pulling the chain taut could cause the wire circles at the end of each link to stretch slightly, making the chain inaccurate. Mason and Dixon measured the links’ length against a three-foot-long brass rod. They reshaped any links that had lengthened. On April 5, Mason “Found the chain a little too long. Corrected it.” When he measured the chain again on April 9 and 12, it was exact. The Penns and Lord Baltimore had hired Mason and Dixon as the best men for the job, and the best is what they got.
By April 12, the crew had traveled over fourteen miles. Now was the time to account for the fact that their starting point, at the observatory in Harlan’s garden, was already 10.5 seconds south of Philadelphia’s southernmost point. That distance had to be included in the overall length of their fifteen-mile-long line south. Mathematically, Mason converted the seconds into yards. He found that 10.5 seconds of a degree of latitude was equal to 357 yards. This distance is more than three and a half times the length of a football field. While that may not seem like much, if the line were placed that much too far south and extended west over the 233-mile-long distance, the acreage added to Pennsylvania’s area would add up. In fact, if the acreage involved were reconfigured as a chunk of land rather than a long, thin strip, it would occupy an area the size of Disney World or the city of San Francisco. The Maryland commissioners would never have ceded that much land to Pennsylvania.

IT TAKES THE EARTH one year to orbit the sun. Because Earth’s axis is tilted, during half of the year, one hemisphere receives more sunlight than the other, creating the summer season. Earth’s tilt also affects the seasonal visibility of certain stars. For example, in December 1763, when Mason and Dixon first began observations in Philadelphia, Capella, one of the brightest stars in the sky, quickly caught their eyes. In the Northern Hemisphere, Capella is a star of the fall and winter sky and would not be visible in July.

These are some of the constellations and stars in the Northern Hemisphere that Mason and Dixon regularly observed during different seasons. In these diagrams, other constellations have been omitted in order to highlight those especially important to the surveyors. But Mason and Dixon had to find their stars from among the thousands that were visible.
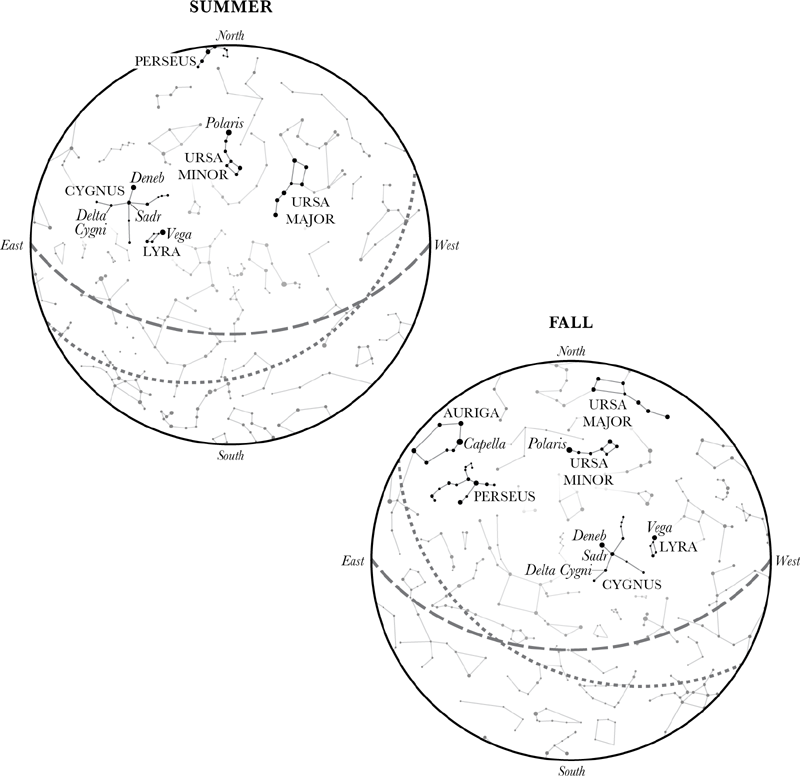
East and West are not labeled incorrectly on these seasonal sky maps. A sky map represents the celestial sphere. To orient a sky map, face north. Hold the sky map above your head, as if you were looking up at the stars. Rotate the sky map until its label for North points toward north. East and west are now properly located.
During the summer, Earth’s tilt and orbital position placed Capella below Mason’s horizon. Seasonally, Mason and Dixon adjusted which stars they observed according to their visibility. The North Star, also called Polaris, is almost straight north of Earth’s axis. It is unaffected by the annual orbit and is visible in the Northern Hemisphere year round, as are the stars that closely surround it.

Mason then converted 357 yards to chains, arriving at the figure 16.23 chains. Dixon added this to the number of chains he and the chain bearers had already measured southward from Harlan’s garden. Together, the figures added up to 15 miles, 2 chains, and 93 links. The crew’s journey south was complete. The end of the fifteen-mile line was on squishy, damp land owned by a man named Alexander Bryan.
During the next week, the crew returned to Harlan’s farm, dismantled the observatory, and packed the instruments into four wagons — Mason employing “five Laborers in carrying one of the instruments,” most likely the zenith sector. Then they returned to the end of the fifteen-mile line, where five men, hammering in the rain, reconstructed the observatory. Meanwhile, Mason and Dixon rode to Philadelphia, where they met with the commissioners to report their progress and receive further instructions.
By June 9, the surveyors had determined that the latitude of the southern end of the fifteen-mile line was 39 degrees 43 minutes 18.2 seconds north (39°43′18.2″ N). This latitude would be the very long east-west boundary line, called the West Line, between Maryland and Pennsylvania. They marked the spot with a wooden post set several feet deep in the ground and called the marker the Post Marked West. They carved the word WEST into the side facing west. Then, following the commissioners’ instructions, the surveyors turned their attention to the Tangent Line, determined to succeed where so many colonial surveyors had failed.
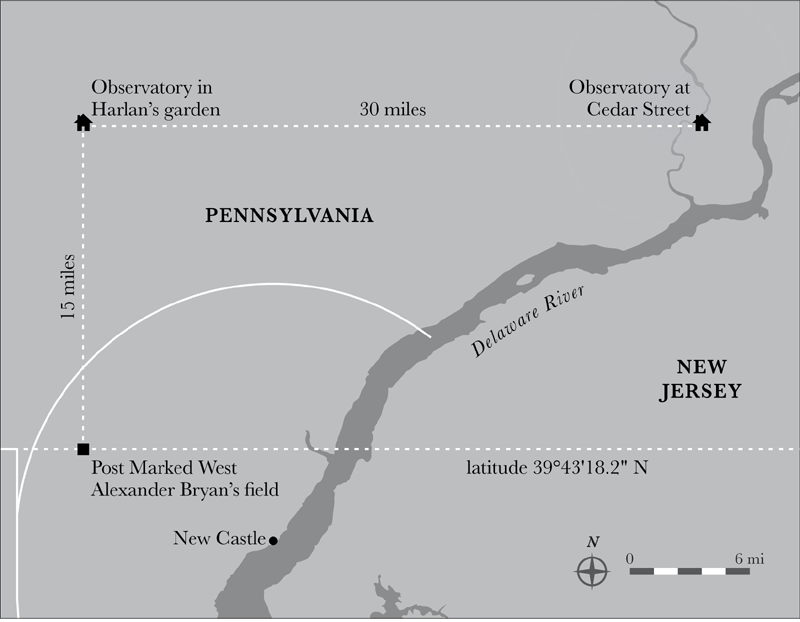
After their trip west from Philadelphia, Mason and Dixon prepared themselves for another trek, this time directly south, in their quest for the latitude of the West Line.
The commissioners expected Mason and Dixon to begin work on the Tangent Line, Maryland’s eighty-mile-long eastern border, by June 15, 1764. With that date fast approaching, Mason and Dixon began assembling a larger crew and plenty of provisions. Knowing that John Vause’s apothecary shop, on the corner of Market and Third Streets in Philadelphia, supplied medicine chests for ship captains, the army, and the navy, Mason ordered a large box stocked with medicines and remedies for treating cuts, fevers, snakebites, and stomach ailments — anything likely to plague a sick or injured crewman on the trail.
Running a large crew was a full-time job. On the recommendation of the commissioners, Mason and Dixon hired twenty-six-year-old Moses McClean as their steward, a position he had held during the previous Tangent Line survey. (His older brother Archibald had been a surveyor on the 1760 crew.) In this capacity, McClean purchased food, tents, and horses. He handled monies sent by the commissioners, took care of payroll logistics, hired crewmen as needed, and oversaw equipment maintenance, arranging for repairs when necessary. In short, McClean was Mason and Dixon’s go-to man. Without him, they could not have focused their full attention on the survey.
Surveying the Tangent Line took Mason and Dixon on their first long American journey. A distance of eighty miles separated the two ends of the line — the southern end, at a spot called the Middle Point, and the northern end, at the tangent point. The surveyors had to travel nearly the whole length of the peninsula shared by Maryland and Pennsylvania before they could start their new task. Under McClean’s supervision, the crew struck camp in Mr. Bryan’s field and packed their gear into the wagons. The surveyors stored their astronomical instruments at a nearby home, a practice that became routine. The team then made its way to New Castle, where Mason and Dixon found comfortable lodgings at an inn, a welcome change from camp life. After rehiring old hands from the Brandywine, McClean and the crew departed for the Middle Point, where work on the Tangent Line would begin.
By the time everyone reached the bank of the Nanticoke River, the crew had swelled to thirty-nine men and the camp bustled with activity. While the axmen honed their blades, tent keepers pounded tent pegs into the ground. Cooks lit fires and prepared the evening meal. Wagoners unhitched their teams and tethered the horses. Moses McClean didn’t want any runaway horses. Two of the horses used during the 1760 Tangent Line survey had strayed from camp, and he’d had to offer a reward for them in the Pennsylvania Gazette. That same issue contained notices about three more lost horses, three found horses, and two stolen horses. Horses were a necessity in colonial America. So McClean and his wagon drivers kept a sharp eye on their animals, cared for them well, and tethered them securely.
The black rectangle inset is the area of the Middle Point.

Jeremiah Dixon’s final map of the boundary line included details such as the Nanticoke River, which they crossed north of the Middle Point.
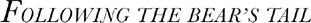
A boundary-line survey must be accurate. No matter how hard the 1760–1763 survey team had tried, surveying the Tangent Line had defeated them; the lines they surveyed had always missed the tangent point along the arc of the circle drawn around New Castle. Rather than attempting an eighty-mile-long survey line aimed at the tangent point (as the earlier survey crews had), Mason just decided to run an eighty-mile-long straight line, knowing that it would end a bit west of the tangent point. Mason planned to then shift their straight line, first mathematically and then, with Dixon’s surveying expertise, physically, until it touched the tangent point. But for Mason’s plan to work, he needed a guiding star to keep Dixon and the chain carriers on an absolutely straight path for eighty miles.
Left: A transit and equal altitude instrument mounted on a tripod. Right: The telescope and level of Dixon’s transit. The transit on the tripod is missing a level. Dixon always suspended a level from his telescope to ensure that the transit was level at all times, which was crucial for accurate measurements. He probably used a tripod but may also have supported the transit on a tree stump.


AN ACCURATE WATCH was essential to the success of Mason and Dixon’s survey. Dixon tested the accuracy of their watch with the transit and equal altitude instrument. First he placed the instrument on the Middle Point. A crewman set a new wooden post half a mile due north of the Middle Point, close to the first wooden marking post set by the 1760 Tangent Line crew. Since it was dark outside, the crewman placed a lit candle atop the post so Dixon could sight the new wooden post with the instrument’s telescope. Despite Dixon’s distance from the candle, he easily saw the flame because no other outside lighting existed to overwhelm it.
Looking into the telescope of the transit and equal altitude instrument, Dixon saw one horizontal cross wire and three vertical cross wires. The vertical wires were perpendicular to the horizontal wire. The middle vertical wire crossed the horizontal wire at the center of the viewing lens. When the candle flame was centered, Dixon tightened screws on the telescope that prevented it from shifting to the left or right. The transit and equal altitude instrument was now aligned with the meridian (the direction true north) for that location. Then he tilted the telescope skyward on the instrument’s vertical axis and tightened it in place so it couldn’t shift up or down. Finally, he looked through the telescope and waited for a specific star to transit, or cross, the horizontal wire in the viewing lens. Mason recorded the hour, minute, and second when it did. As Dixon kept watch, Mason also recorded the exact hour, minute, and second when that same star transited each of the vertical wires.
During the course of a night, Earth’s rotation causes the stars to appear as though they are traveling westward across the sky in an arc-shaped path. Dixon monitored the star’s path until it transited the horizontal wire a second time, this time on the opposite side of the three vertical wires. Mason recorded that time, too. By dividing the time that elapsed between the star’s first and second crossings of the horizontal wire in half, Mason and Dixon knew the exact time when the star reached its zenith, or highest point in the sky, according to their watch. At that point, the star crossed the meridian — the line that encircles the celestial sphere in a north-south direction. This precise time was another piece of information Mason and Dixon factored into their calculations. If the watch gained or lost time when compared to established times published in the star tables provided by the Royal Society, Mason needed to compensate mathematically for the difference. Satisfied that their watch was accurate, they began to implement Mason’s creative plan for running the Tangent Line.

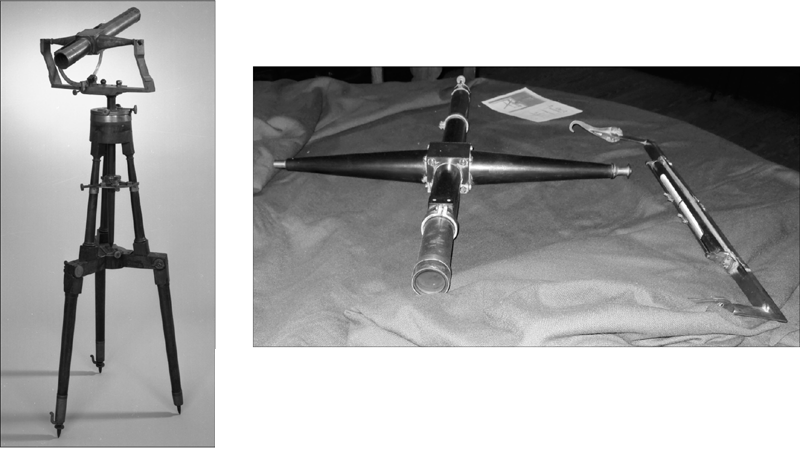
The star Mason chose is named Delta Ursae Minoris, located in the constellation Ursa Minor, the Little Bear. Delta Ursae Minoris is the second star in the bear’s tail. Mason didn’t note why he chose this star, but it is close to the North Star and is directionally north, which was generally the direction they were heading. And unlike the North Star, whose position doesn’t shift measurably, Delta Ursae Minoris appears to move in an arc that Dixon could easily observe with the transit and equal altitude instrument. These observations would provide Mason with the data he needed for his calculations that would enable them to remain steadfastly on a straight course.
As he had done during the watch test, a crewman placed a lighted candle atop the recently set wooden post half a mile north of the Middle Point. Dixon centered the flame in the viewing lens of the transit and equal altitude’s telescope and tightened the scope to prevent it from shifting to the left or right.
As he had done before, Dixon tipped the telescope skyward and fixed it in place. Then he and Mason waited for Delta Ursae Minoris to appear. Slowly, as the minutes ticked by, the small star moved into view and approached the horizontal cross wire. Mason noted the exact hour, minute, and second when it first touched the horizontal cross wire, as well as the second time it did so. In the daytime, with the transit’s alignment still firmly fixed in place, Dixon lowered the telescope and directed a chain carrier, who stood at a point some distance away, to move his position until he was precisely aligned with Dixon’s transit. Then Dixon instructed the other chain carriers to measure the distance to that man. They did so using the same procedure they had used in 1764 when surveying to the point fifteen miles south from the observatory in John Harlan’s garden. Chain after chain, the crew surveyed a straight line northwestward, marking each new mile with a wooden post. Meanwhile, Mason consulted the sidereal times in the Royal Society’s star tables and compared them to the times he and Dixon noted. Mason needed this information for mathematical computations that would help him precisely establish the Tangent Line at a later date. For the next two months, Dixon’s task was to keep the transit and equal altitude instrument precisely aligned with the course set by Delta Ursae Minoris. At every fifth milepost, the chain carriers measured the offset, or distance, between the new posts they were setting along their line and those set during the failed attempt by John Lukens and his crew during the summer of 1763.
Setting their course on Delta Ursae Minoris, the surveyors began their quest for the Tangent Line by setting up the transit and equal altitude instrument at the Middle Point. Stargazing at night and measuring with a Gunter’s chain during the day, the surveyors moved in a slightly northwestern direction until they’d traveled more than eighty miles.
During their eighty-mile journey, the crew frequently encountered waterways. Chaining across small creeks was no problem; they just waded across. But measuring the width of the Nanticoke River, the first large waterway they encountered, presented a mathematical challenge, since the river was far wider than a Gunter’s chain.
At the river’s edge, Dixon set up an instrument called a Hadley’s quadrant, which is used to measure angles between distant objects. Peering through the sight, he adjusted the quadrant so a mirror on the instrument reflected two separate objects on the opposite side of the river. A scale on the quadrant measured the angle created by Dixon and the two objects. Dixon used the angle in a mathematical computation called triangulation. The result provided him with the width of the river, which he added to their Gunter’s chain measurements. While the Hadley’s quadrant was sufficiently accurate for determining the width of a river, it was not an efficient tool for surveying the hilly terrain Dixon and Mason encountered along much of the Pennsylvania-Maryland boundary. Afterward, the surveyors crossed the river in canoes. Since canoes are a Native American watercraft and not used in England, this was probably Mason and Dixon’s first canoe trip. Mason did not note whether either one of them attempted paddling.

Charles Mason’s daily journal from August 15 through 26, 1764, records mileposts 68 through 81, which signified the end of their eighty-mile-long straight line. On the 26th, he noted that a wagon needed repair.
By August 18, Mason and Dixon’s straight line was seventy-three miles long. They sent express riders to the provincial governors to report that they expected to complete their line in eight to ten days. Wearily, they stopped after setting the eighty-first milepost, judging their position sufficiently north and west of the tangent point. Everyone was hot and exhausted. Makeshift repairs on shopworn equipment were no longer possible. McClean sent one of the wagons to Philadelphia for repair and directed the wagoner to bring four small tents when he returned.
Knowing their straight line was long enough — indeed, it extended farther north and west than the tangent point — Mason and Dixon’s next job was to locate the actual tangent point. After doing so, they could begin the process of shifting their line eastward, where it could be correctly positioned and marked as the Tangent Line.
Trudging toward the New Castle Circle, crewmen cut back trees and shrubs until they found the mileposts, set by the 1760–1763 crew, that marked the twelve-mile radius from the courthouse in New Castle to the tangent point. The last post of the radius, which was set on the circle’s circumference, was the tangent point. After they had revealed the posts, the crew cleared a vista westward from the tangent point to the straight line Mason and Dixon had just completed. Then the crew extended the radius of the New Castle Circle from the tangent point until it intersected Mason and Dixon’s new line. They measured the distance with a Gunter’s chain and found it to be 22.51 chains (almost 1,486 feet) long. Measuring this distance accurately was crucial for the calculations Mason would use to shift their new line over to the tangent point. And, like toppling dominos, that shift would help them determine the correct placement of all the other wooden mileposts between the tangent point and the Middle Point. (Later, the crew would physically move the posts.) To be certain that his calculations would be as accurate as possible, Mason insisted on double-checking the distance the crew had just chained: “To prove that the Chain Carriers had made no error in the measurement . . . I took a man with me, a few days after, and measured it myself: and made it within a Link of the same.”
The black rectangle inset includes the beginning of the West Line.

Dixon’s map shows where the New Castle Circle touched the Tangent Line, creating the Tangent Point.
After nearly a week with no word about the fixed wagon or new tents, Mason and Dixon started back to the Middle Point. They were armed with crucial information. They knew their new line was straight. They knew the exact locations of the tangent point and the Middle Point. They knew the distance from the end of their straight line to the end of Lukens’s 1763 survey line. They also knew the distance between the end of Lukens’s line and the tangent point. And finally, they knew the locations of the 1763 five-mile markers. With this information, Mason calculated how much each of these markers would have to be moved eastward to be accurately set to create a true Tangent Line.
As they continued south, stories of the Pocomoke Swamp and its dark labyrinth of waterways that threaded through tangled shrubs and bear-filled woods piqued Mason and Dixon’s curiosity about the mysterious land east of the Middle Point. One clear morning, they saddled their horses and trotted off to visit the swamp. The lush vegetation enchanted Mason: “There is the greatest quantity of Timber I ever saw: Above the Tallest Oak, Beech, Poplar, Hickory, Holly and Fir; Towers the lofty Cedar (without a Branch) ’till its ever green conical top seems to reach the clouds.”
The closer to the Middle Point the crew got, the smaller the offsets became between the old, incorrect line and the true Tangent Line, which ended only twenty-six inches to the west of the Middle Point. The next morning, beginning at this revised Middle Point, they headed back toward the tangent point. Along the way, they would take fresh measurements to double-check and tweak the accuracy of the Tangent Line and to ensure that the mileposts were set properly.

This diagram in Mason’s journal includes the survey crew’s measurements and some of the mathematical calculations he made to shift their eighty-mile-long line over to the tangent point.
The meticulous measuring made the already tedious journey seem endless. It took the crew forty-four days to reach milepost 65. For three days in November, work ground to a halt when thick fog made surveying impossible. During the downtime, Mason and Dixon met with the commissioners at George Town and updated them on their progress.
Finally, on November 10, the crew reached the eighty-mile mark. They found their line was sixteen feet nine inches east of the tangent point. Mason divided the difference proportionately over the entire length of the Tangent Line. By doing this, he could tell which of the mileposts, if any, were seriously out of position and, if so, have them reset. But the results satisfied him; none of the markers was set far enough off the Tangent Line to affect the line overall. The Tangent Line was as accurate as it could be.
Yet Mason insisted on one final accuracy check for the post he and Dixon had placed at the end of their Tangent Line. A line that is tangent to a circle is always perpendicular to the radius at the tangent point. Mason measured the angle between the end of their Tangent Line and the radius that extended from the belfry of the New Castle courthouse. Mason found “it was so near a right angle, that, on a mean from our Lines, the above mentioned Post is the true tangent Point.”
On the eve of their first anniversary in America, Mason and Dixon were satisfied that they and their crew had successfully completed the Tangent Line. They’d done the impossible. They discharged the axmen and notified the governors of their results. The duo was delighted to hear that the equally satisfied boundary commissioners came to a resolution that “what we had done relating to the Lines should stand as finished.”
As 1764 drew to a close, Mason and Dixon returned to John Harlan’s home at the fork of the Brandywine, where they spent Christmas with their American friends.

McClean’s account book of cash paid out (left side) and monies received (right side) for November 24, 1764, through April 6, 1765