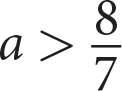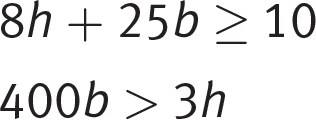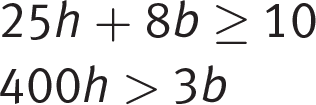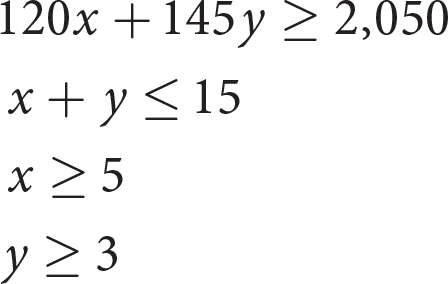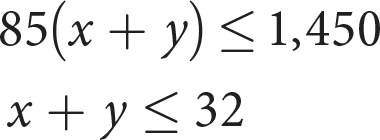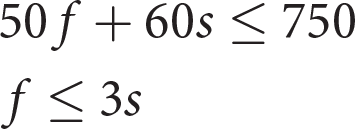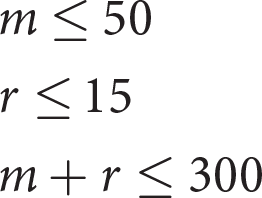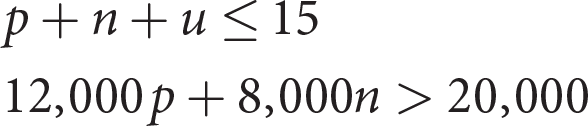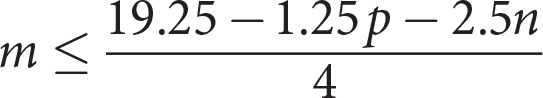Page 93

Chapter 6

Inequalities

Page 94

How Much Do You Know?

Directions: Try the questions that follow. Show your work so that you can compare your solutions to the ones found in the Check Your Work section immediately after this question set. The “Category” heading in the explanation for each question gives the title of the lesson that covers how to solve it. If you answered the question(s) for a given lesson correctly, and if your scratchwork looks like ours, you may be able to move quickly through that lesson. If you answered incorrectly or used a different approach, you may want to take your time on that lesson.

  1. If , what is the least possible value of  ?

    1. 7
    2. 10
    3. 16
    4. 18

  2. If , then what is one possible value of 12h − 4 ?

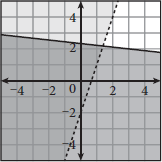
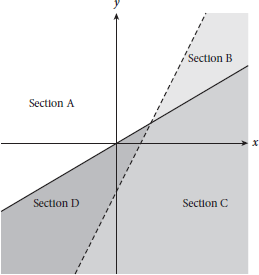
    xy coordinate graph showing the lines for the given equations. Their intersection creates 4 sections labeled A, B, C, and D proceeding clockwise from top left.

  3. The graph above depicts the system of inequalities shown. Which of the labeled section or sections of the graph could represent all of the solutions of the system?

    1. Sections A and D
    2. Section B
    3. Sections C and D
    4. Section D

  4. A bowling alley charges a flat $6.50 fee for shoe and ball rental plus $3.75 per game and 6.325 percent sales tax. If each person in a group of seven people has $20 to spend on a bowling outing, and at least some members of the group must rent shoes and a ball, which inequality best describes this situation, given that the number of shoe and ball rentals is represented by r and the number of games is represented by g ?

    1. 1.06325(6.5r + 3.75g) ≤ 140
    2. 1.06325(6.5r + 3.75g) ≤ 20
    4. 0.06325(6.5r + 3.75g) ≤ 20
    Page 95

  5. Marco is paid $80 per day plus $15 per hour for overtime. If he works five days per week and wants to make a minimum of $520 this week, what is the fewest number of hours of overtime he must work?

    image

  6. An architect in an arid region determines that a building’s current landscaping uses $1,640 worth of water monthly. The architect plans to replace the current landscaping with arid-zone landscaping at a cost of $15,900, which will reduce the monthly watering cost to $770. Which of the following inequalities can be used to find m, the number of months after replacement that the savings in water costs will be at least as much as the cost of replacing the landscaping?

    1. 15,900 ≥ (1,640 − 770)m
    2. 15,900 > 770m
    3. 15,900 ≤ (1,640 − 770)m
    4. 15,900 ≤ 770m

Page 96

Check Your Work

  1. C

    Difficulty: Medium

    Category: Linear Inequalities

    Getting to the Answer: You might be tempted to solve for p, but some critical thinking and algebra make that unnecessary. First, get 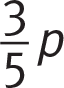 by itself by adding 2 to both sides: . Next, multiply both sides by 2 to get the value range of . Finally, add 2 to both sides to get 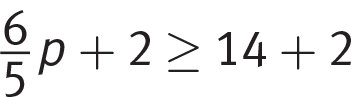. Thus, , so (C) is correct.

  2. Any value greater than or equal to 0 and less than 3.5

    Difficulty: Hard

    Category: Linear Inequalities

    Getting to the Answer: Don’t automatically start solving the two inequalities separately. Instead, look for a series of quick manipulations to convert 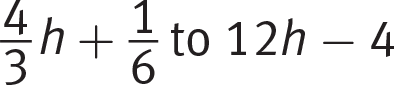to 12h − 4. Start by multiplying the entire inequality by 9 to yield . Next, subtract  and then 4 more (to get the desired −4) from all parts of the inequality (converting the fraction component to a decimal will make this step easier), which will become −32.5 < 12h − 4 < 3.5. Because grid-in answers cannot be negative, pick any value that is greater than or equal to 0 but less than 3.5.

  3. D

    Difficulty: Medium

    Category: Systems of Inequalities

    Getting to the Answer: Sections A and D are all the y values greater than 2x − 3, so those sections are the solution set for the inequality y > 2x − 3. Similarly, Sections C and D are the solution set for 5y ≤ 3x. The solutions for a system of inequalities are all the points that satisfy all (both, in this case) of the inequalities. The points in Section D are in the solution set for both inequalities, so (D) is correct.

  4. A

    Difficulty: Medium

    Category: Modeling Real-Life Situations with Inequalities

    Getting to the Answer: The question has defined the variables for you (r and g). The bowling alley charges $6.50 for shoe and ball rental and $3.75 per game. Sales tax is 6.325% (0.06325 in decimal form). Rental and game costs are given by 6.5r and 3.75g, respectively. To incorporate sales tax into the total cost, multiply the sum of 6.5r and 3.75g by 1.06325 (not 0.06325, which gives the cost of sales tax only). The question asks for an inequality that represents the amount that the group can spend, which can be no more than 7 × 20 = 140; therefore, the correct inequality is 1.06325(6.5r + 3.75g) ≤ 140. This matches (A).

  5. 8

    Difficulty: Medium

    Category: Modeling Real-Life Situations with Inequalities

    Getting to the Answer: Translate from English into math to create an inequality in which h represents the number of hours of overtime Marco must work. Marco gets paid a daily wage plus an hourly rate for overtime, so his weekly pay is his daily rate ($80) times 5 days, plus the number of hours of overtime he works (h) times his overtime rate ($15). If he wants to make at least $520, which means that much or more, the inequality is (80 × 5) + 15h ≥ 520. Solve for h:

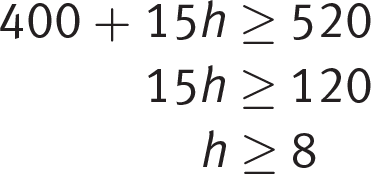
    Marco must work at least 8 hours of overtime to make $520 or more this week. Grid in 8.Page 97

  6. C

    Difficulty: Medium

    Category: Modeling Real-Life Situations with Inequalities

    Getting to the Answer: The question asks for the point at which the savings will be at least as much as the cost of replacing the landscaping, so the value range could include a value equal to the exact cost of replacement; eliminate the strict inequality in (B). Because you need a value range that is at least as much as the cost of landscaping, you need the cost of landscaping, $15,900, to be less than or equal to the value range. Eliminate (A). 

    The value range will be the value of the monthly savings times the number of months, which will be m times the difference between the old monthly water cost and the new monthly water cost: (1,640 − 770)m. Therefore, the entire inequality expression is 15,900 ≤ (1,640 − 770)m, so (C) is correct. Page 98

Linear Inequalities

To answer a question like this:

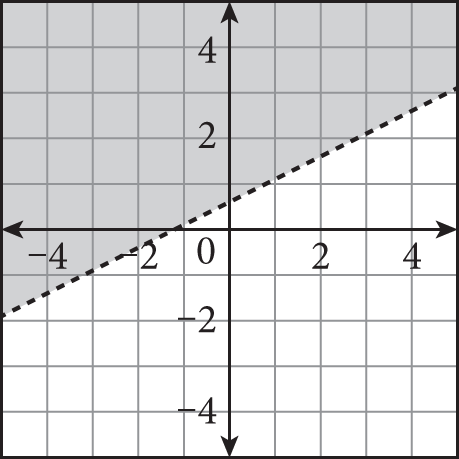
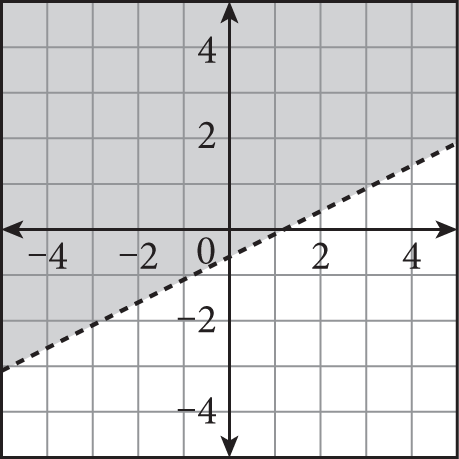
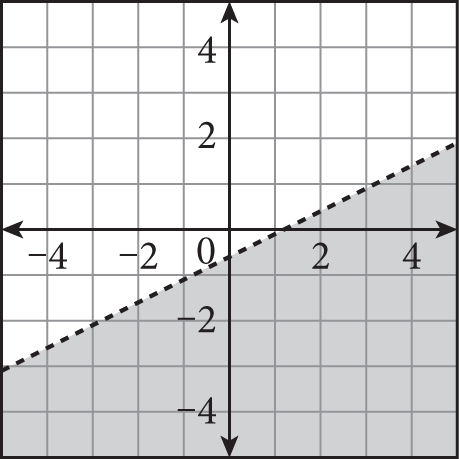
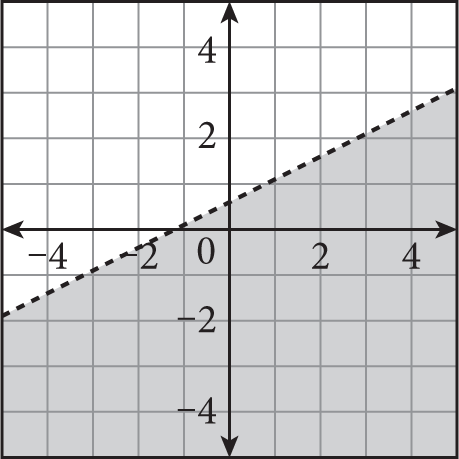
  1. Which of the following graphs represents the solution set for 5x − 10y > 6 ?

    1. A dashed line graphed on a coordinate plane that rises from left to right, has a y-intercept of zero point 6, and is shaded above the line.
    2. A dashed line graphed on a coordinate plane that rises from left to right, has a y-intercept of negative zero point 6, and is shaded above the line.
    3. A dashed line graphed on a coordinate plane that rises from left to right, has a y-intercept of negative zero point 6, and is shaded below the line.
    4. A dashed line graphed on a coordinate plane that rises from left to right, has a y-intercept of zero point 6, and is shaded below the line.

Page 99

You need to know this:

Linear inequalities are similar to linear equations but have two differences: 

While linear equations graph as simple lines, inequalities graph as shaded regions. Use solid lines for inequalities with ≤ or ≥ signs because the line itself is included in the solution set. Use dashed lines for inequalities with < or > signs because, in these cases, the line itself is not included in the solution set. The shaded region represents all points that make up the solution set for the inequality.

You need to do this:

To graph an inequality, start by writing the inequality in slope-intercept form, then graph the solid or dashed line. Finally, add shading:

If it’s hard to tell which region is above/below the line (which can happen when the line is steep), compare the y-values on both sides of the line.

Explanation:

Rewrite the inequality in slope-intercept form and then identify which half-plane should be shaded. Subtract 5x from both sides of the inequality, divide both sides by −10, and flip the inequality symbol to yield 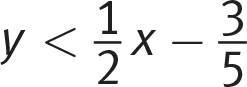. Eliminate (A) and (D) because they have positive y-intercepts. The “less than” symbol indicates that the half-plane below the line should be shaded, making (C) the correct answer.Page 100

Try on Your Own

Directions: Take as much time as you need on these questions. Work carefully and methodically. There will be an opportunity for timed practice at the end of the chapter.

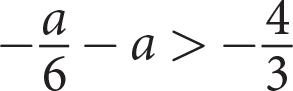
  1. Which of the following is equivalent to the inequality above?


  1. If −5c − 7 ≤ 8, what is the least possible value of 15c + 7 ?

    1. −38
    2. –4
    3. 15
    4. 22

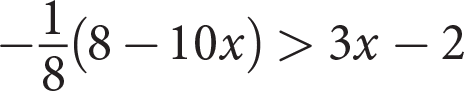
  1. Which of the following describes all possible values of x ?

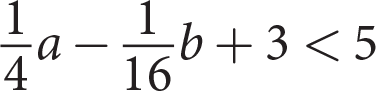
  2. Which of the following is equivalent to the inequality above?

    1. 4a − b < 8
    2. 4a − b < 32
    3. a − 4b < 32
    4. 4b − a < 4

  3. If 4c + 20 ≥ 31, what is the least possible value of 12c + 7 ?

    1. 18
    2. 40
    3. 51
    4. 58

Page 101

Systems of Inequalities

To answer a question like this:

  1. If 12X − 4y > 8 and form a system of inequalities, which of the following graphs shows the solution set for the system?

    1. A solid line with a negative slope and y-intercept just above two, with shading below the line. A dashed line with y-intercept of negative two and positive slope, shaded above the dashed line.
    2. A solid line with a y-intercept of just above 2 and a negative slope, with shading above the line. A dashed line with y-intercept of negative 2 and positive slope, shaded below the line.
    3. A dashed line with y-intercept just above 2 and a negative slope, shaded above the line. A solid line with y-intercept of negative 2 and a positive slope, shaded below the line.
    4. A solid line with y-intercept just above 2 and a negative slope, shaded below the line. A dashed line with y-intercept of negative 2 and positive slope, shaded below the line.

Page 102

You need to know this:

The solution set for a system of inequalities is not a single point (a single x-value and y-value) but a region of overlap between the two inequalities: that is, a range of x-values and y-values. It is easiest to see this graphically.

Systems of inequalities can be presented graphically with multiple boundary lines and multiple shaded regions. Follow the same rules as for graphing single inequalities, but keep in mind that the solution set is the region where the shading overlaps.

Note that you generally cannot use substitution or combination to solve a system of two inequalities where both have two variables. That said, the SAT may ask for the maximum or minimum x- or y-value of a system of inequalities. These questions are actually asking about the intersection of the boundary lines of the system. If you see one of these questions, use substitution or combination to solve for the point of intersection, as you learned to do in chapter 5. For an example of this type of question (which is rare on the SAT), see question number 8 in the Try on Your Own set for this lesson.

You may also see an occasional question without a graph asking you to solve a system of one inequality in two variables and another inequality in one variable. As long as both inequalities have the same symbol, you can do this by substitution. Question number 6 in this lesson’s Try on Your Own set is an example of this rare question type.

You need to do this:

Explanation:

Rewrite the inequalities in slope-intercept form. Once complete, determine whether each line should be solid or dashed and which half of the plane (above or below the line) should be shaded for each. The correct graph should have a dashed line with a positive slope (y < 3x − 2) and a solid line with a negative slope ; eliminate (C) because the dashed and solid lines are incorrect. According to the inequality symbols, the half-plane above the solid line and the half-plane below the dashed line should be shaded; the only match is (B).Page 103

Try on Your Own

Directions: Take as much time as you need on these questions. Work carefully and methodically. There will be an opportunity for timed practice at the end of the chapter.

  1. Which of the following consists of all the a-values that satisfy the system of inequalities above?

    1. a < 20
    2. a < 16
    3. a < 12 
Coordinate grid with Quadrants labeled I, II, III, and IV counter-clockwise from top right.

  1. If the system of inequalities and  is graphed on the above plane, which of the quadrants contain(s) no solutions to the system?

    1. Quadrant I
    2. Quadrant II
    3. Quadrant III
    4. Quadrants I and II

  2. Given the system of inequalities above, if point (a, b) lies within the solution set, what is the minimum possible value of b ?

    image

  3. Which of the following ordered pairs satisfies both of the inequalities above?

    1. (−1, 3)
    2. (1, 1)
    3. (2, −3)
    4. (4, 4)

Page 104

  1. If x = y = 1 is a solution to the system of inequalities above, which of the following ordered pairs could correspond to (r, s) ?

    1. (−1, 1)
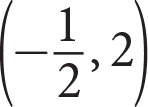
    4. (3, −1)

Page 105

Modeling Real-Life Situations with Inequalities

To answer a question like this:

  1. To make its sales goals for the month, a toy manufacturer must sell at least $10,400 of toy hoops and basketballs. Toy hoops sell for $8 and basketballs sell for $25. The company hopes to sell more than three times as many basketballs as toy hoops. If h represents the number of toy hoops and b represents the number of basketballs, where h and b are positive integers, which of the following systems of inequalities best describes this situation?

You need to know this:

Word problems involving inequalities require you to do the same sort of translation that you learned in chapter 4. They also require you to get the direction of the inequality sign right. The following table shows which symbols correspond to which words.

English Symbol
more, greater, longer, heavier >
less, fewer, shorter, lighter <
no less than, no fewer than, at least ≥
no more than, no greater than, at most ≤

You need to do this:

Break down the word problem one inequality at a time. For each one:

Page 106

Explanation:

“At least $10,400” means ≥ 10,400. It’s the money from the sales of toy hoops and basketballs that has to be greater than or equal to $10,400, so that’s what needs to go on the left of the ≥ sign. Each toy hoop costs $8, so the cash generated by sales of toy hoops will be 8h. Each basketball costs $25, so basketballs will generate 25b. Add them: 8h + 25b ≥ 10,400. Eliminate (C) and (D).

The company wants to sell “more than three times as many basketballs as toy hoops,” so write down > and work out what needs to go on each side. The company wants to sell more basketballs than toy hoops, so b should go on the left. Specifically, they want basketball sales to be more than 3 times toy hoop sales, so the final statement is b > 3h. (A) is correct.Page 107

Try on Your Own

Directions: Take as much time as you need on these questions. Work carefully and methodically. There will be an opportunity for timed practice at the end of the chapter.

  1. Ariel enters a contest to sell advertisements in her school’s yearbook. To qualify for a prize, she has to sell at least $1,500  worth of advertisements consisting of no fewer than 15 individual ads. Each full-page ad costs $110, each half-page ad costs $70, and each quarter-page ad costs $50. Which of the following systems of inequalities represents this situation, where x is the number of full-page ads she sells, y is the number of half-page ads she sells, and z is the number of quarter-page ads she sells?

  2. A farmer sells watermelons, cantaloupes, and tomatoes from a small cart at a county fair. He needs to sell at least $200 of produce each day. His watermelons are priced at $0.50 per pound, his cantaloupes at $1 per pound, and his tomatoes at $2.50 per pound. His cart can hold no more than 250 pounds. Which of the following inequalities represents this scenario, if w is the number of pounds of watermelons, c is the number of pounds of cantaloupes, and t is the number of pounds of tomatoes?

  3. Page 108Allison is planting a garden with at least 15 trees. There will be a combination of apple trees, which cost $120 each, and pear trees, which cost $145 each. Allison’s budget for purchasing the trees is no more than $2,050. She must plant at least 5 apple trees and at least 3 pear trees. Which of the following systems of inequalities represents the situation described if x is the number of apple trees and y is the number of pear trees?

  4. A utility shelf in a warehouse is used to store containers of paint and containers of varnish. Containers of paint weigh 50 pounds each and containers of varnish weigh 35 pounds each. The shelf can hold up to 32 containers, the combined weight of which must not exceed 1,450 pounds. Let x be the number of containers of paint and y be the number of containers of varnish. Which of the following systems of inequalities represents this relationship?

  1. A bakery is buying flour and sugar from its supplier. The supplier will deliver no more than 750 pounds in a shipment. Each bag of flour weighs 50 pounds and each bag of sugar weighs 20 pounds. The bakery wants to buy at least three times as many bags of sugar as bags of flour. If f represents the number of bags of flour and s represents the number of bags of sugar, where f and s are nonnegative integers, which of the following systems of inequalities represents this situation?

Page 109

How Much Have You Learned?

Directions: For testlike practice, give yourself 15 minutes to complete this question set. Be sure to study the explanations, even for questions you got right. They can be found at the end of this chapter.

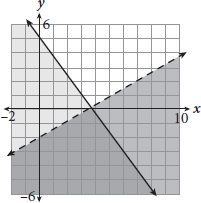
A solid line and a dashed line graphed on a coordinate plane, with shading. The solid line intersects the Y-axis at 5, falls from left to right, and the graph is shaded below the line. The dashed line intersects the Y-axis at negative 2, rises from left to right, and the graph is shaded below the line.

  1. The figure above shows the solution set for this system of inequalities:

    Which of the following is NOT a solution to this system?

    1. (−1, −4)
    2. (1, −1)
    3. (4, −1)
    4. (6, −3)

  2. Ezekiel has $5.00 to spend on snacks. Candy bars cost $0.60 each, gum costs $0.50 per pack, and nuts are priced at $1.29 per small bag. If c represents the number of candy bars, g represents the number of packs of gum, and n represents the number of bags of nuts, which of the following inequalities correctly describes Ezekiel’s choices?

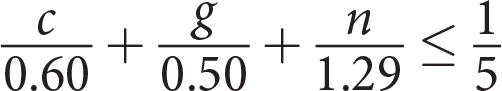
    2. c + g + n ≤ 5
    3. 0.60c + 0.50g + 1.29n ≤ 5.00
    4. 0.60c + 0.50g + 1.29n ≥ 5.00

  3. A shipping company employee is in charge of packing cargo containers for shipment. He knows a certain cargo container can hold a maximum of 50 microwaves or a maximum of 15 refrigerators. Each microwave takes up 6 cubic feet of space, and each refrigerator takes up 20 cubic feet. The cargo container can hold a maximum of 300 cubic feet. The employee is trying to figure out how to pack a container containing both microwaves and refrigerators. Which of the following systems of inequalities can the employee use to determine how many of each item (microwaves, m, and refrigerators, r) he can pack into one cargo container?

    Page 111

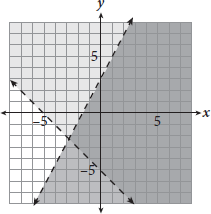
    Two intersecting dashed lines graphed on a coordinate plane with shading. The upper line intersects the Y-axis at 3 and rises from left to right. The graph is shaded below this line. The lower line intersects the Y-axis at negative 5 and falls from left to right. The graph is shaded above this line. The lines intersect at approximately negative 3 comma negative 2.

  4. The figure above shows the solution for the system of inequalities shown. Suppose (a, b) is a solution to the system. If a = 0, what is the greatest possible integer value of b ?

    image

  5. Which of the following describes the range of x ?

    1. x > 1
    2. x > 9
    3. −1 < x < 9
    4. 9 > x > 1

  6. In the xy plane, the point (a, b) lies in the solution set of the system of inequalities above. What is the minimum possible value of b ?

    2. 3

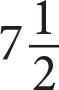
  7. Francine sells advertising time packages for a local television station. She is able to make up to 15 sales calls per week offering potential advertisers either a prime time package for $12,000 or a non prime time package for $8,000. Her weekly sales goal is to sell more than $20,000 worth of advertising. Which of the following systems of inequalities represents this situation in terms of p, the number of prime time packages Francine sells in a week, n, the number of non prime time packages, and u, the number of unsuccessful sales calls for which she sells neither offering?

    Page 112

  8. Luis has $25 to spend on school supplies. Pencils (p) cost $1.25 per package, notebooks (n) are priced at $2.50 each, and markers (m) sell for $4 per pack. He must buy exactly one calendar/planner for $5.75. Which of the following describes how many markers Luis can buy?

  9. Let a and b be numbers such that −a < b + 1 < a. Which of the following must be true?

    1. a > 0
    2. |b| < a
    3. b > a + 1
    1. I only 
    2. I and II
    3. II only
    4. I, II, and III


  10. The variable x is a positive integer. If 3(x − 1) + 5 > 11 and −5x + 18 ≥ −12, how many possible values are there for x ?

    image

Page 113

Reflect

Directions: Take a few minutes to recall what you’ve learned and what you’ve been practicing in this chapter. Consider the following questions, jot down your best answer for each one, and then compare your reflections to the expert responses on the following page. Use your level of confidence to determine what to do next.

What is the difference between a linear inequality and a linear equation?

The rules for manipulating an inequality are very similar to those for manipulating an equation. What is the major difference?

When two lines cross in the coordinate plane, they create four regions. How many regions will be shaded if the system of inequalities uses the word “and”? The word “or”?

Page 114

Expert Responses

What is the difference between a linear inequality and a linear equation?

A linear equation is solved for a single value, whereas a linear inequality is solved for a range of values.

The rules for manipulating an inequality are very similar to those for manipulating an equation. What is the major difference?

When solving an inequality, you can do the same thing to both sides, just as you could for an equation. The big difference is that if you divide or multiply both sides of an inequality by a negative number, you have to flip the inequality sign.

When two lines cross in the coordinate plane, they create four regions. How many regions will be shaded if the system of inequalities uses the word “and”? The word “or”?

Of the four regions, one represents values that satisfy neither inequality. One represents values that satisfy both inequalities. The other two regions represent values that satisfy one inequality but not the other. For an “and” inequality, only the region of values that satisfy both inequalities will be shaded. For an “or” inequality, the only region not shaded will be the one that satisfies neither inequality; the other three regions will be shaded.

Next Steps

If you answered most questions correctly in the “How Much Have You Learned?” section, and if your responses to the Reflect questions were similar to those of the SAT expert, then consider inequalities an area of strength and move on to the next chapter. Come back to this topic periodically to prevent yourself from getting rusty.

If you don’t yet feel confident, review those parts of this chapter that you have not yet mastered and try the questions you missed again. As always, be sure to review the explanations closely.Page 115

Answers and Explanations

Linear Inequalities

  1. C

    Difficulty: Easy

    Getting to the Answer: Begin by multiplying all parts of the inequality by 6 to clear the fractions: , which, when simplified, is −7a > −8. Divide both sides by −7, remembering to switch the direction of the sign: . Therefore, (C) is correct.

  2. A

    Difficulty: Medium

    Getting to the Answer: Don’t solve for c on autopilot. Instead, solve for 15c, then add 7 to both sides. To do this, first multiply both sides of the inequality by −1 to get 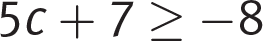. (Notice that the inequality sign had to be flipped due to multiplication by a negative number.) Then subtract 7 from both sides to yield . Multiply both sides by 3: . Finally, add 7 to both sides: . Choice (A) is correct.

  3. C

    Difficulty: Medium

    Getting to the Answer: First, to clear the fraction, multiply both sides of the inequality by −8, remembering to flip the direction of the inequality sign:

    Next, subtract 8 from both sides, and then add 24x to both sides. Divide both sides by 14, and then simplify the fraction.

    Thus, (C) is correct.

  4. B

    Difficulty: Medium

    Getting to the Answer: Begin by subtracting 3 from both sides of the inequality to get 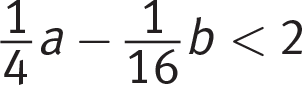. Next, clear the fractions in that inequality by multiplying all terms by 16: . (B) is correct.

  5. B

    Difficulty: Medium

    Strategic Advice: Because the given inequality contains 4c and the value you’re solving for has 12c (a multiple of 4c), solve for 4c and then multiply by 3 to find 12c.

    Getting to the Answer: Subtract 20 from both sides of the inequality to find that . Multiply by 3: . Finally, add 7 to both sides: . So the least permissible value for  is 40. (B) is correct.

Systems of Inequalities

  1. A

    Difficulty: Easy

    Getting to the Answer: The question offers a value range of a in terms of 6b and a value range for 3b. Therefore, use the known value range of 3b to find the value range of 6b. 

    Since 6b is two times 3b, multiply both sides of the second inequality by 2 to find the value range of 6b: 2 × 3b < 2 × 8, so 6b < 16. 

    Because the signs are the same in the two inequalities (both are less-than signs), you can plug 16 in for b in the first inequality: a < 16 + 4, or a < 20. Hence, (A) is correct.

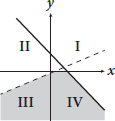
  2. B

    Difficulty: Medium

    Getting to the Answer: Draw a sketch of the two lines to help visualize the system of inequalities.

    Given lines shown on the quadrant graph with the area below their intersection shaded.

    Clearly, quadrants III and IV contain solutions to the system. Eliminate (C). Quadrant II contains no solutions, so you can also eliminate (A). Look closely at quadrant I. The line for intersects both the x and y axes at +1. The line for 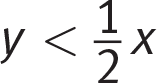 passes through the origin and upward into quadrant I. Thus, there is a very small triangle of solutions to both inequalities that lies in quadrant I. Therefore, (B) is correct.Page 116

  3. 400

    Difficulty: Medium

    Getting to the Answer: The first task is to express both inequalities in terms of y. Multiply −y ≤ 6x − 2200 by −1 to get y ≥ −6x + 2200; don’t forget to flip the sign since you are multiplying by a negative number. Divide 3y ≥ 9x − 1500 by 3 to get y ≥ 3x − 500.

    The solution set for these inequalities is the area of the coordinate plane on or above both lines. Thus, the minimum y-value will occur at the intersection of the two lines, whose equations are y = −6x + 2200 and y = 3x − 500. To find the point of intersection, set these two equations equal to each other: −6x + 2200 = 3x − 500. Isolate the x-values on one side to yield −9x = −2700 and x = 300. Plug this value into one of the equations (it doesn’t matter which one because x = 300 is where they intersect) to obtain the y-coordinate at the point of intersection: 3(300) − 500 = 400. The y-coordinate at the point of intersection is the minimum possible value of b that the question was asking for, so grid in 400.

  4. C

    Difficulty: Medium

    Getting to the Answer: You could plot the two lines and identify the solution set on a graph, but testing the choices to see if they satisfy both inequalities is a more efficient approach, particularly since one inequality is “less than” and the other is “less than or equal to.” If you take this approach, you don’t even need to rearrange the inequalities to isolate y on one side. 

    (A): Substituting these values for x and y in x < 4 − 2y gives you (−1) < 4 − 2(3), which is −1 < −2 (not a true statement). You don’t need to evaluate the other inequality since this ordered pair is not in the solution set for x < 4 − 2y. Eliminate (A).

    (B):  Substituting these values for x and y in x < 4 − 2y gives you (1) < 4 −2(1), which is 1 < 2. The ordered pair is in the solution set for this inequality, so plug the values into y ≤ −2x + 1 to get (1) ≤ −2(1) + 1, which simplifies to 1 ≤ −1. Thus, this ordered pair is not in the solution set for the second inequality. Eliminate (B).

    (C):  Substituting these values for x and y in x < 4 − 2y gives you (2) < 4 − 2(−3), which is 2 < 10. The ordered pair is in the solution set for this inequality, so plug the values into y ≤ −2x + 1 to get (−3) ≤ −2(2) + 1, which simplifies to −3 ≤ −3. Since the sign for this inequality is “less than or equal to,” this ordered pair is in the solution set. (C) is correct.

    (D): Since you already identified the correct choice, you do not need to check this pair. 

  5. C

    Difficulty: Hard

    Getting to the Answer: Since the question states that x = y = 1 is a solution to the system, plug those values in to get 1 > 1 + r and 1 < s − 1. These inequalities further simplify to r < 0 and s > 2. Check each pair of values in the answer choices to see which complies with these limitations. Only (C) has both  r < 0 and s > 2, and is correct. (B) is incorrect because s must be greater than 2, and (D) reverses r and s.

Modeling real-Life Situations with Inequalites

  1. C

    Difficulty: Medium

    Getting to the Answer: Translate each part of the word problem into its mathematical equivalent. Because x, y, and z represent the numbers of each of the three ad sizes, the total number of ads will be x + y + z. “No fewer than 15 ads” means x + y + z ≥ 15, so eliminate (A) and (B). 

    The total cost of the ads Ariel sells will be represented by the number of each size ad sold times the respective cost of each size ad. Thus, 110x + 70y + 50z represents the total cost of all of the ads; “at least 1,500” means that 110x + 70y + 50z ≥ 1,500. Therefore, (C) is correct.

  2. A

    Difficulty: Medium

    Getting to the Answer: Translate each part of the word problem into its mathematical equivalent. The total weight of produce will be represented by the combined pounds of watermelons, w,  cantaloupes, c, and tomatoes, t. This combined weight cannot exceed 250 pounds, so the inequality is w + c + t ≤ 250. Eliminate (C) and (D).

    The total money for the produce sold is represented by the price per pound of each type of produce times the number of pounds of that type. This must be at least (greater than or equal to) $200, so the inequality is 0.5w + 1c + 2.5t ≥ 200. (A) is correct.Page 117

  3. D

    Difficulty: Medium

    Getting to the Answer: Translate each part of the word problem into its mathematical equivalent, beginning with the easiest-to-translate components. Allison needs at least 5 apple trees and at least 3 pear trees, so the correct inequalities are x ≥ 5 and y ≥ 3. Eliminate (B) and (C). The total number of apple and pear trees combined that Allison purchases must be at least 15, so the correct inequality is x + y ≥ 15. Eliminate (A). Only (D) is left and is correct.

    Note that, by being strategic, you never even have to determine the first, most complicated inequality in each of the answer choices. For the record: apple trees cost $120 and pear trees cost $145, so the total amount Allison will spend on trees is 120x + 145y. This total amount cannot go above $2,050, which means it must be less than or equal to $2,050. Therefore: 120x + 145y ≤ 2,050.

  4. B

    Difficulty: Medium

    Getting to the Answer: First, define the relationship between the weight of each kind of container and the weight the shelf can hold. Since x is the number of 50-pound containers of paint and y is the number of 35-pound containers of varnish, the combined weight of the containers will be represented by 50x + 35y. This needs to be no more than 1,450 pounds, so the inequality that represents this is 50x + 35y ≤ 1,450. Eliminate (A) and (C).

    The question also states that the total number of containers the shelf can hold is no more than 32, so the combined number of containers of paint and containers of varnish must be no more than 32. This is represented by the inequality x + y ≤ 32. Thus, (B) is correct.

  5. C

    Difficulty: Hard

    Getting to the Answer: Begin by translating the weight of the combined bags of flour and sugar. The weight of each bag of flour times the number of bags of flour—plus the weight of each bag of sugar times the number of bags of sugar—will yield the total. Thus, 50f + 20s will describe the weight of all of the bags combined. Since the weight that the supplier can deliver is no more than 750 pounds, the inequality that describes this situation is 50f + 20y  ≤ 750. Eliminate (A) and (D).

    The question also specifies that the bakery needs to buy at least three times as many bags of sugar as bags of flour. In other words, the number of bags of sugar must be equal to or greater than three times the number of bags of flour. This is represented by s ≥ 3f, which can be rewritten as 3f ≤ s. (C) is correct.

  1. A

    Difficulty: Hard

    Strategic Advice: All the numbers in the answer choices are the same, so you don’t actually have to calculate the cost of one carnation or one daisy. The answer choices have done that for you.

    Getting to the Answer: Focus on the keywords that tell you the direction of the inequality signs. The florist plans to order a “maximum” of 500 flowers, so that’s ≤ 500. Eliminate (C) and (D). The florist wants to earn “at least” $400, or ≥ 400. The correct answer is (A).

  1. B

    Difficulty: Medium

    Category: Systems of Inequalities

    Getting to the Answer: The intersection (overlap) of the two shaded regions is the solution to the system of inequalities. Check each point to see whether it lies in the region where the shading overlaps. Be careful—you are looking for the point that is not a solution to the system. Choices (A) and (C) lie in the overlap, so you can eliminate them. Choice (D), which is the point (6, −3), lies on a boundary line, and because the line is solid, the point is included in the solution region. The only point that does not lie within the overlap must be (B). To check this, plug (1, −1) into the first inequality:

    Because −1 is not less than , (B) is correct.Page 118

  2. C

    Difficulty: Easy

    Category: Modeling Real-Life Situations with Inequalities

    Getting to the Answer: The fact that Ezekiel has exactly $5.00 means that he can spend up to and including $5.00, so use the less than or equal symbol (≤) for the limit on how much he can spend.  For that reason alone you can eliminate (D). The amount of money Ezekiel spends on each type of snack is the number of units of that snack times its price. So, 0.60c + 0.50g + 1.29n ≤ 5.00. (C) is correct.

  3. C

    Difficulty: Medium

    Category: Modeling Real-Life Situations with Inequalities

    Getting to the Answer: The clue “holds a maximum” means the container can hold exactly that much or less, so use the less than or equal to symbol (≤) throughout. The cargo container can hold a maximum of 50 microwaves, so the first inequality is m ≤ 50.  Eliminate (A). The container can hold a maximum of 15 refrigerators, so the second inequality is r ≤ 15. The third inequality deals with the size of each appliance. The cargo container can hold m microwaves multiplied by the size of the microwave, which is 6 cubic feet; it can hold r refrigerators multiplied by the size of the refrigerator, which is 20 cubic feet; and it can hold a maximum of 300 cubic feet total. Put these together to write the final inequality: 6m + 20r ≤ 300, which is (C).

  4. 2

    Difficulty: Hard

    Category: Systems of Inequalities

    Getting to the Answer: If (a, b) is a solution to the system, then a is the x-coordinate of any point in the region where the shading overlaps, and b is the corresponding y-coordinate. When a = 0 (or x = 0), the maximum possible value for b lies on the upper boundary line, y < 2x + 3. It looks like the y-coordinate is 3, but to be sure, substitute x = 0 into the equation and simplify. You can replace the inequality symbol with an equal sign to make it an equation because you are finding a point on the boundary line, not a range:

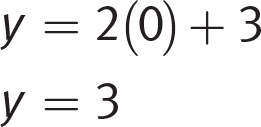
    The point on the boundary line is (0, 3). The boundary line is dashed (because the inequality is strictly less than), so (0, 3) is not a solution to the system. This means 2 is the greatest possible integer value for b when a = 0. Grid in 2.

  5. D

    Difficulty: Medium

    Category: Linear Inequalities

    Getting to the Answer: Isolate x in each of the given inequalities, then consider the results in combination. For 3x + 2 > 5, subtract 2 from each side to get 3x > 3, which means that x > 1. For −2x + 8 > −10, subtract 8 from each side to get −2x > −18. Divide each side by −2, which means that you have to flip the inequality sign to get x < 9. Taking the two inequalities together, x must be greater than 1 but less than 9. This can be expressed as 1 < x < 9 or, alternatively, 9 > x > 1. (D) is correct.

  6. D

    Difficulty: Medium

    Category: Systems of Inequalities

    Strategic Advice: If the given inequalities are graphed in the xy‑plane, the solution set is the portion of the plane on or above both lines. Since the value of y for both lines increases moving away from the point where they intersect, the minimum value of y will occur at the intersection of these lines, which is the point (a, b). 

    Getting to the Answer: The boundary lines of this system of inequalities are y = −3x + 18 and y = 9x. To find their intersection point, set them equal to each other to get 9x = −3x + 18. So, 12x = 18 and 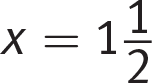 . That represents a, but the question asks for the value of b. Substitute  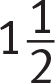for x in either equation, preferably the simpler one: use y = 9x to get . Therefore, (D) is correct.Page 119

  7. A

    Difficulty: Medium

    Category: Modeling Real-Life Situations with Inequalities

    Strategic Advice: Translating English into math, set up one inequality for Francine’s number of sales calls and another for the dollar amount of sales in terms of her goal.

    Getting to the Answer: Since Francine can make up to 15 sales calls per week and there are three categories of the results of her efforts, you can write the inequality p + n + u ≤ 15. Eliminate (B). For the monetary goal, that is more than $20,000, so this inequality is 12,000p + 8,000n > 20,000. Note that the unsuccessful sales calls are not included in this inequality since they do not generate any revenue. (A) is correct.

  8. B

    Difficulty: Medium

    Category: Modeling Real-Life Situations with Inequalities

    Getting to the Answer: The amount Luis spends on each item is that item’s price times the quantity he buys. The total amount must be less than or equal to $25. Thus, you can write the inequality 1.25p + 2.50n + 4.00m + 1(5.75) ≤ 25.00. Since the question asks for the number of markers, isolate m on one side of the inequality: 4m ≤ 25 − 1.25p − 2.5n − 5.75. Therefore, . (B) is correct.

  9. B

    Difficulty: Hard

    Category: Linear Inequalities

    Getting to the Answer: Examine each of the roman numeral choices individually, determine which one or ones must be true, then select the correct choice. Subtract 1 from all terms in the given inequality to get −a − 1 < b < a − 1, so that b stands alone. 

    I. Ignoring the middle term, b, the given inequality tells you that −a < a. The only way this can be true is if a is a positive number, so statement I must be true. If you have a hard time seeing this, pick a negative number for a. For example, if a = −1, then the inequality would say −(−1) < −1, which is 1 < −1, which is false. Note that if a = 0, then the inequality becomes 0 < 0, which is also false. Because statement I must be true, you can eliminate (C).

    II. Recall that the absolute value of any number is its distance from 0 on the number line, which is its value without a sign. If b is positive or 0, then, since b < a − 1, the value of b, which is the same as |b|, must also be less than a. However, if b is negative, the relationship is not directly clear. Pick some numbers that satisfy the original inequality, such as a = 4 and b = −2. For these numbers, −a − 1 = −5, |b| = 2, a − 1 = 3. Thus, −5 < 2 < 3, so −a − 1 < |b| < a − 1, which means that a must be more than 1 greater than |b|. Because a must be positive (as determined by statement I) and because a must be more than 1 greater than b (from the original inequality), the value of −a − 1 must be less than b. Thus, statement II must be true. Eliminate (A). If the logic here is difficult, plug in more numbers for a and b to find a pattern.

    III. Since a must be positive and any positive value of b is less than a − 1, there is no way that b > a + 1. Statement III is not true, so eliminate (D).

    Because I and II must be true, and III cannot be true, (B) is correct.

  10. 3

    Difficulty: Medium

    Category: Linear Inequalities

    Strategic Advice: Isolate x in each of the two inequalities to determine a range for x.

    Getting to the Answer: Expand the first inequality to get 3x − 3 + 5 > 11, so 3x > 9 and x > 3. Multiply the second inequality by −1, so that 5x − 18 ≤ 12. This simplifies to x ≤ 6. Combine the two results: 3 < x ≤ 6. There are 3 allowable integer values for x: 4, 5, and 6. Grid in 3.