

Mathematicians and Their World
SOME ARE LONERS, SOME ARE ICONOCLASTS, AND SOME ARE gregarious self-promoters. There is just one feature that defines great mathematicians—an inborn genius, a talent like that of an elite athlete or a gifted musician or exceptional artist. The profiles in this section show the range of mathematicians’ talents and why these astonishingly creative people chose their field.
They include the rural uneducated Indian genius Srinivasa Ramanujan, who died at age 32 at the turn of the 20th century. Somehow, he intuited deep mathematical truths.
James Gleick, writing about Ramanujan, quotes a mathematician who says, “He seems to have functioned in a way unlike anybody else we know of. He had such a feel for things that they just flowed out of his brain.”
Then there is Andrew Wiles, who solved the notorious Fermat’s Last Theorem while working in seclusion for seven years in his attic office in his Princeton home. “No computer was necessary, and no telephone was present to intrude on the absolute silence,” I wrote. He told no one what he was doing. One of his colleagues explained why: “If he said he was working on Fermat’s Last Theorem, people would look askance. And if you start telling people who are experts, you end up collaborating with them. He wanted to do it on his own.”
John Horton Conway, one of Wiles’s colleagues at Princeton, loves games and puzzles and wants to learn odd facts, like the digits of pi extended to 1,000 places or the name and location of every visible star.
He became famous in 1969, when he was working at the University of Cambridge, desperately seeking recognition, and took up a hard problem.
“Basically, I said goodbye to my wife, kissed the kids and stuck myself in the front room.” To his own amazement, he solved the problem in 12 hours.
The list, and the stories, go on, each personality offering a different view into the world of mathematics and why it drew each of them. The chapter ends with an article by George Johnson on one other type of personality drawn to the field. Mathematicians call them the math cranks.
And they pose a dilemma as they send out lengthy missives to well-known mathematicians, demanding a hearing, someone to look over what they say is pathbreaking work.
“Who is going to stroll through pages and pages of stuff that is very hard to understand when you don’t have to do it?” one mathematician told Johnson. “From the point of view of these guys, we are arrogant, unwilling to reconsider ideas. And why shouldn’t they expect a responsible scientist to look carefully at some new idea that might be important?
“You can’t just say Ramanujan was a genius and these other guys were cranks. With a superficial look, there is hardly any visible difference. There is not always a sharp line between eccentric mathematicians and intelligent but maybe obsessed amateurs.”
Paul Erdos, 83, a Wayfarer in Math’s Vanguard, Is Dead
Paul Erdos, a legendary mathematician who was so devoted to his subject that he lived as a mathematical pilgrim with no home and no job, died on Friday in Warsaw. He was 83.
The cause of death was a heart attack, according to an e-mail message sent out this weekend by Dr. Miki Simonovits, a mathematician at the Hungarian Academy of Sciences, who was a close friend.
Dr. Erdos (pronounced AIR-dosh) was attending a mathematics meeting in Warsaw when he died, Dr. Simonovits reported.
The news, only now reaching the world’s mathematicians, has come as a blow. Dr. Ronald L. Graham, the director of the information sciences research center at A.T.&T. Laboratories, said, “I’m getting e-mail messages from around the world, saying, ‘Tell me it isn’t so.’”
Never, mathematicians say, has there been an individual like Paul Erdos. He was one of the century’s greatest mathematicians, who posed and solved thorny problems in number theory and other areas and founded the field of discrete mathematics, which is the foundation of computer science. He was also one of the most prolific mathematicians in history, with more than 1,500 papers to his name. And, his friends say, he was also one of the most unusual.
Dr. Erdos “is on the short list for our century,” said Dr. Joel H. Spencer, a mathematician at New York University’s Courant Institute of Mathematical Sciences.
Dr. Graham said, “He’s among the top 10.”
Dr. Ernst Straus, who worked with both Albert Einstein and Dr. Erdos, wrote a tribute to Dr. Erdos shortly before his own death in 1983. He said of Dr. Erdos: “In our century, in which mathematics is so strongly dominated by ‘theory doctors,’ he has remained the prince of problem solvers and the absolute monarch of problem posers.” Dr. Erdos, he continued, is “the Euler of our time,” referring to the great 18th-century mathematician, Leonhard Euler, whose name is spoken with awe in mathematical circles.
Stooped and slight, often wearing socks and sandals, Dr. Erdos stripped himself of all the quotidian burdens of daily life: finding a place to live, driving a car, paying income taxes, buying groceries, writing checks. “Property is nuisance,” he said.
Concentrating fully on mathematics, Dr. Erdos traveled from meeting to meeting, carrying a half-empty suitcase and staying with mathematicians wherever he went. His colleagues took care of him, lending him money, feeding him, buying him clothes and even doing his taxes. In return, he showered them with ideas and challenges—with problems to be solved and brilliant ways of attacking them.
Dr. László Babai of the University of Chicago, in a tribute written to celebrate Dr. Erdos’s 80th birthday, said Dr. Erdos’s friends “care for him fondly, repaying in small ways for the light he brings into their homes and offices.”
Mathematicians like to brag about their connections to Dr. Erdos by citing their “Erdos number.” A person’s Erdos number is 1 if he or she has published a paper with Dr. Erdos. It is 2 if he or she has published with someone who has published with Erdos, and so on. At last count, Dr. Erdos had 458 collaborators, Dr. Graham said. An additional 4,500 mathematicians had an Erdos number of 2, Dr. Graham added. He said so many mathematicians were still at work on problems they had begun with Dr. Erdos that another 50 to 100 papers with Dr. Erdos’s name on them were expected to be published after his death.
Dr. Graham, whose Erdos number is 1, handled Dr. Erdos’s money for him, setting aside an “Erdos room” in his house for the chore. He said Dr. Erdos had given away most of the money he earned from lecturing at mathematics conferences, donating it to help students or as prizes for solving problems he had posed. Dr. Erdos left behind only $25,000 when he died, Dr. Graham said, and he plans to confer with other mathematicians about how to give it away to help mathematics.
Dr. Graham said Dr. Erdos’s “driving force was his desire to understand and to know.” He added, “You could think of it as Erdos’s magnificent obsession. It determined everything in his life.”
Dr. Spencer, of New York University, who also has an Erdos number of 1, said, “He was always searching for mathematical truths.” He added: “Erdos had an ability to inspire. He would take people who already had talent, that already had some success, and just take them to an entirely new level. His world of mathematics became the world we all entered.”
Born in Hungary in 1913, Dr. Erdos was a cosseted mathematical prodigy. At age 3, Dr. Graham said, Dr. Erdos discovered negative numbers for himself when he subtracted 250 degrees from 100 degrees and came up with 150 degrees below zero. A few years later, he amused himself by solving problems he had invented, like how long would it take for a train to travel to the sun.
Dr. Erdos had two older sisters who died of scarlet fever a few days before he was born, so his mother became very protective of him. His parents, who were mathematics teachers, took him out of public school after just a few years, Dr. Graham said, and taught him at home with the help of a German governess. And, Dr. Graham said, Dr. Erdos’s mother coddled him. “Erdos had never buttered his own toast until he was 21 years old,” Dr. Graham said. He never married and left no immediate survivors.
When Dr. Erdos was 20, he made his mark as a mathematician, discovering an elegant proof for a famous theorem in number theory. The theorem, Chebyshev’s theorem, says that for each number greater than 1, there is always at least one prime number between it and its double. A prime number is one that has no divisors other than itself and 1.
Although his research spanned a variety of areas of mathematics, Dr. Erdos kept up his interest in number theory for the rest of his life, posing and solving problems that were often simple to state but notoriously difficult to solve and that, like Chebyshev’s theorem, involved relationships between numbers. “He liked to say that if you can state a problem in mathematics that’s unsolved and over 100 years old, it is probably a problem in number theory,” Dr. Graham said.
Dr. Erdos, like many mathematicians, believed that mathematical truths are discovered, not invented. And he had an evocative way of conveying that notion. He spoke of a Great Book in the sky, maintained by God, that contained the most elegant proofs of every mathematical problem. He used to joke about what he might find if he could just have a glimpse of that book.
He would also muse about the perfect death. It would occur just after a lecture, when he had just finished presenting a proof and a cantankerous member of the audience would have raised a hand to ask, “What about the general case?” In response, Dr. Erdos used to say, he would reply, “I think I’ll leave that to the next generation,” and fall over dead.
Dr. Erdos did not quite achieve his vision of the perfect death, Dr. Graham said, but he came close.
“He died with his boots on, in hand-to-hand combat with one more problem,” Dr. Graham said. “It was the way he wanted to go.”
September 24, 1996
Journeys to the Distant Fields of Prime

Four hundred people packed into an auditorium at U.C.L.A. in January to listen to a public lecture on prime numbers, one of the rare occasions that the topic has drawn a standing-room-only audience.
Another 35 people watched on a video screen in a classroom next door. Eighty people were turned away.
The speaker, Terence Tao, a professor of mathematics at the university, promised “a whirlwind tour, the equivalent to going through Paris and just seeing the Eiffel Tower and the Arc de Triomphe.”
His words were polite, unassuming and tinged with the accent of Australia, his homeland. Even though prime numbers have been studied for 2,000 years, “There’s still a lot that needs to be done,” Dr. Tao said. “And it’s still a very exciting field.”
After Dr. Tao finished his one-hour talk, which was broadcast live on the Internet, several students came down to the front and asked for autographs.
Dr. Tao has drawn attention and curiosity throughout his life for his prodigious abilities. By age 2, he had learned to read. At 9, he attended college math classes. At 20, he finished his Ph.D.
Now 31, he has grown from prodigy to one of the world’s top mathematicians, tackling an unusually broad range of problems, including ones involving prime numbers and the compression of images. Last summer, he won a Fields Medal, often considered the Nobel Prize of mathematics, and a MacArthur Fellowship, the “genius” award that comes with a half-million dollars and no strings.
“He’s wonderful,” said Charles Fefferman of Princeton University, himself a former child prodigy and a Fields Medalist. “He’s as good as they come. There are a few in a generation, and he’s one of the few.”
Colleagues have teasingly called Dr. Tao a rock star and the Mozart of Math. Two museums in Australia have requested his photograph for their permanent exhibits. And he was a finalist for the 2007 Australian of the Year award.
“You start getting famous for being famous,” Dr. Tao said. “The Paris Hilton effect.”
Not that any of that has noticeably affected him. His campus office is adorned with a poster of Ranma ½, a Japanese comic book series. As he walks the halls of the math building, he might be wearing an Adidas sweatshirt, blue jeans and scruffy sneakers, looking much like one of his graduate students. He said he did not know how he would spend the MacArthur money, though he mentioned the mortgage on the house that he and his wife, Laura, an engineer at the NASA Jet Propulsion Laboratory, bought last year.
After a childhood in Adelaide, Australia, and graduate school at Princeton, Dr. Tao has settled into sunny Southern California.
“I love it a lot,” he said. But not necessarily for what the area offers.
“It’s sort of the absence of things I like,” he said. No snow to shovel, for instance.
A deluge of media attention following his Fields Medal last summer has slowed to a trickle, and Dr. Tao said he was happy that his fame might be fleeting so that he could again concentrate on math.
One area of his research—compressed sensing—could have real-world use. Digital cameras use millions of sensors to record an image, and then a computer chip in the camera compresses the data.
“Compressed sensing is a different strategy,” Dr. Tao said. “You also compress the data, but you try to do it in a very dumb way, one that doesn’t require much computer power at the sensor end.”
With Emmanuel Candès, a professor of applied and computational mathematics at the California Institute of Technology, Dr. Tao showed that even if most of the information were immediately discarded, the use of powerful algorithms could still reconstruct the original image.
By useful coincidence, Dr. Tao’s son, William, and Dr. Candès’s son attended the same preschool, so dropping off their children turned into useful work time.
“We’d meet each other every morning at preschool,” Dr. Tao said, “and we’d catch up on what we had done.”
The military is interested in using the work for reconnaissance: blanket a battlefield with simple, cheap cameras that might each record a single pixel of data. Each camera would transmit the data to a central computer that, using the mathematical technique developed by Dr. Tao and Dr. Candès, would construct a comprehensive view. Engineers at Rice University have made a prototype of just such a camera.
Dr. Tao’s best-known mathematical work involves prime numbers—positive whole numbers that can be divided evenly only by themselves and 1. The first few prime numbers are 2, 3, 5, 7, 11 and 13 (1 is excluded).
As numbers get larger, prime numbers become sparser, but the Greek mathematician Euclid proved sometime around 300 B.C. that there is nonetheless an infinite number of primes.
Many questions about prime numbers continue to elude answers. Euclid also believed that there was an infinite number of “twin primes”—pairs of prime numbers separated by 2, like 3 and 5 or 11 and 13—but he was unable to prove his conjecture. Nor has anyone else in the succeeding 2,300 years.
A larger unknown question is whether hidden patterns exist in the sequence of prime numbers or whether they appear randomly.
In 2004, Dr. Tao, along with Ben Green, a mathematician now at the University of Cambridge in England, solved a problem related to the Twin Prime Conjecture by looking at prime number progressions—series of numbers equally spaced. (For example, 3, 7 and 11 constitute a progression of prime numbers with a spacing of 4; the next number in the sequence, 15, is not prime.) Dr. Tao and Dr. Green proved that it is always possible to find, somewhere in the infinity of integers, a progression of any length of equally spaced prime numbers.
“Terry has a style that very few have,” Dr. Fefferman said. “When he solves the problem, you think to yourself, ‘This is so obvious and why didn’t I see it? Why didn’t the 100 distinguished people who thought about this before not think of it?’”
Dr. Tao’s proficiency with numbers appeared at a very young age. “I always liked numbers,” he said.
A 2-year-old Terry Tao used toy blocks to show older children how to count. He was quick with language and used the blocks to spell words like “dog” and “cat.”
“He probably was quietly learning these things from watching Sesame Street,” said his father, Dr. Billy Tao, a pediatrician who immigrated to Australia from Hong Kong in 1972. “We basically used Sesame Street as a babysitter.”
The blocks had been bought as toys, not learning tools. “You expect them to throw them around,” said the elder Dr. Tao, whose accent swings between Australian and Chinese.
Terry’s parents placed him in a private school when he was 3½. They pulled him out six weeks later because he was not ready to spend that much time in a classroom, and the teacher was not ready to teach someone like him.
At age 5, he was enrolled in a public school, and his parents, administrators and teachers set up an individualized program for him. He proceeded through each subject at his own pace, quickly accelerating through several grades in math and science while remaining closer to his age group in other subjects. In English classes, for instance, he became flustered when he had to write essays.
“I never really got the hang of that,” he said. “These very vague, undefined questions. I always liked situations where there were very clear rules of what to do.”
Assigned to write a story about what was going on at home, Terry went from room to room and made detailed lists of the contents.
When he was 7½, he began attending math classes at the local high school.
Billy Tao knew the trajectories of child prodigies like Jay Luo, who graduated with a mathematics degree from Boise State University in 1982 at the age of 12, but who has since vanished from the world of mathematics.
“I initially thought Terry would be just like one of them, to graduate as early as possible,” he said. But after talking to experts on education for gifted children, he changed his mind.
“To get a degree at a young age, to be a record-breaker, means nothing,” he said. “I had a pyramid model of knowledge, that is, a very broad base and then the pyramid can go higher. If you just very quickly move up like a column, then you’re more likely to wobble at the top and then collapse.”
Billy Tao also arranged for math professors to mentor Terry.
A couple of years later, Terry was taking university-level math and physics classes. He excelled in international math competitions. His parents decided not to push him into college full time, so he split his time between high school and Flinders University, the local university in Adelaide. He finally enrolled as a fulltime college student at Flinders when he was 14, two years after he would have graduated had his parents pushed him only according to his academic abilities.
The Taos had different challenges in raising their other two sons, although all three excelled in math. Trevor, two years younger than Terry, is autistic with top-level chess skills and the musical savant gift to play back on the piano a musical piece—even one played by an entire orchestra—after hearing it just once. He completed a Ph.D. in mathematics and now works for the Defence Science and Technology Organisation in Australia.
The youngest, Nigel, told his father that he was “not another Terry,” and his parents let him learn at a less accelerated pace. Nigel, with degrees in economics, math and computer science, now works as a computer engineer for Google Australia.
“All along, we tend to emphasize the joy of learning,” Billy Tao said. “The fun is doing something, not winning something.”
Terry completed his undergraduate degree in two years, earned a master’s degree a year after that, then moved to Princeton for his doctoral studies. While he said he never felt out of place in a class of much older students, Princeton was where he finally felt he fit among a group of peers. He was still younger, but was not necessarily the brightest student all the time.
His attitude toward math also matured. Until then, math had been competitions, problem sets, exams. “That’s more like a sprint,” he said.
Dr. Tao recalled that as a child, “I remember having this vague idea that what mathematicians did was that, some authority, someone gave them problems to solve and they just sort of solved them.”
In the real academic world, “Math research is more like a marathon,” he said.
As a parent and a professor, Dr. Tao now has to think about how to teach math in addition to learning it.
An evening snack provided him an opportunity to question his son, who is 4. If there are 10 cookies, how many does each of the five people in the living room get?
William asked his father to tell him. “I don’t know how many,” Dr. Tao replied. “You tell me.”
With a little more prodding, William divided the cookies into five stacks of two each.
Dr. Tao said a future project would be to try to teach more non-mathematicians how to think mathematically—a skill that would be useful in everyday tasks like comparing mortgages.
“I believe you can teach this to almost anybody,” he said.
But for now, his research is where his focus is.
“In many ways, my work is my hobby,” he said. “I always wanted to learn another language, but that’s not going to happen for a while. Those things can wait.”
March 13, 2007
Highest Honor in Mathematics Is Refused
Grigori Perelman, a reclusive Russian mathematician who solved a key piece in a century-old puzzle known as the Poincaré conjecture, was one of four mathematicians awarded the Fields Medal today.
But Dr. Perelman refused to accept the medal, as he has other honors, and he did not attend the ceremonies at the International Congress of Mathematicians in Madrid.
“I regret that Dr. Perelman has declined to accept the medal,” Sir John M. Ball, president of the International Mathematical Union, said during the ceremonies.
The Fields Medal, often described as mathematics’ equivalent to the Nobel Prize, is given every four years, and several can be awarded at once. Besides Dr. Perelman, three professors of mathematics were awarded Fields Medals this year: Andrei Okounkov of Princeton; Terence Tao of University of California, Los Angeles; and Wendelin Werner of the University of Paris-Sud in Orsay.
Dr. Perelman, 40, is known not only for his work on the Poincaré conjecture, among the most heralded unsolved math problems, but also because he has declined previous mathematical prizes and has turned down job offers from Princeton, Stanford and other universities. He has said he wants no part of $1 million that the Clay Mathematics Institute in Cambridge, Mass. has offered for the first published proof of the conjecture.
In June, Dr. Ball traveled to St. Petersburg, Russia, where Dr. Perelman lives, for two days in hopes of persuading him to go to Madrid and accept the medal.
“He was very polite and cordial, and open and direct,” Dr. Ball said in an interview.
But he was also adamant. “The reasons center around his feeling of isolation from the mathematical community,” Dr. Ball said of Dr. Perelman’s refusal, “and in consequence his not wanting to be a figurehead for it or wanting to represent it.”
Dr. Ball added, “I don’t think he meant it as an insult. He’s a very polite person. There was never a cross word.”
Despite Dr. Perelman’s refusal, he is still officially a Fields Medalist. “He has a say whether he accepts it, but we have awarded it,” Dr. Ball said.
Beginning in 2002, Dr. Perelman, then at the Steklov Institute of Mathematics of the Russian Academy of Sciences in St. Petersburg, published a series of papers on the Internet and gave lectures at several American universities describing how he had overcome a roadblock in the proof of the Poincaré conjecture.
The conjecture, devised by Henri Poincaré in 1904, essentially says that the only shape that has no holes and fits within a finite space is a sphere. That is certainly true looking at two-dimensional surfaces in the everyday three-dimensional world, but the conjecture says the same is true for three-dimensional surfaces embedded in four dimensions.
Dr. Perelman solved a difficult problem that other mathematicians had encountered when trying to prove the conjecture, using a technique called Ricci flow that smoothes out bumps in a surface and transforms it into a simpler form.
Dr. Okounkov, born in 1969 in Moscow, was recognized for work that tied together different fields of mathematics that had seemed unrelated. “This is the striking feature of Okounkov’s work, finding unexpected links,” said Enrico Arbarello, a professor of geometry at the University of Rome in Italy.
Dr. Okounkov’s work has found use in describing the changing surfaces of melting crystals. The boundary between melted and non-melted is created randomly, but the random process inevitably produces a border in the shape of a heart.
Dr. Tao, a native of Australia and one of the youngest Fields Medal winners ever at age 31, has worked in several different fields, producing significant advances in the understanding of prime numbers, techniques that might lead to simplifying the equations of Einstein’s theory of general relativity and the equations of quantum mechanics that describe how light bounces around in a fiber optic cable.
Dr. Werner, born in Germany in 1968, has also worked at the intersection of mathematics and physics, describing phenomena like percolation and shapes produced by the random paths of Brownian motion.
The medal was conceived by John Charles Fields, a Canadian mathematician, “in recognition of work already done and as an encouragement for further achievements on the part of the recipient.”
Since 1936, when the medal was first awarded, judges have interpreted the terms of Dr. Fields’s trust fund to mean that the award should usually be limited to mathematicians 40 years old or younger.
August 22, 2006
Scientist at Work: John H. Conway; At Home in the Elusive World of Mathematics
Dr. John H. Conway sits down at his computer and gets ready to log on. But before the computer allows him to begin work, it quickly spews out 10 randomly selected dates from the past and the future, dates like 3/15/2005 or 4/29/1803. Dr. Conway has to mentally calculate what day of the week each would be before his computer lets him open a file and get to work.
It is a game he has rigged up to play with himself. “I think I’m the fastest person in the world at this,” he says. His record is 15.92 seconds to calculate all 10 days. But, he modestly says, it is not that hard. “It’s the kind of thing autistic kids do.”
Dr. Conway is unlike any other mathematician at Princeton University, and even in the field at large he stands out for the originality of his work on a mix of disparate and difficult subjects, including number theory, knot theory, the theory of quadratic forms and the theory of games.
And in a profession where quirky skills, like being a lightning calculator, are not unexpected, he is also remarkable for his odd and unusual interests and abilities. He is obsessed with games and puzzles, and has a thirst to learn unusual facts, like the digits of pi extended to 1,000 places or the name and location of every star in the heavens. “It took me a year to learn that,” the 55-year-old mathematician said, explaining that the sky changes each night. But, at the same time, he has made significant mathematical discoveries of both theoretical and practical importance.
Dr. Ronald L. Graham, adjunct director for the information science division at A.T.&T. Bell Laboratories in Murray Hill, N.J., described Dr. Conway as “one of the most original mathematicians” around. “He’s definitely world class, yet he has this kind of childlike enthusiasm,” Dr. Graham said. “He’s confident enough to work on any crazy thing he wants to.” He added that Dr. Conway was entitled to veer off the beaten path. “He’s certainly achieved enough,” Dr. Graham said.
But Dr. Conway has so many peculiar interests and quirks, and he can so easily be made to sound eccentric, that his deep love of mathematics and the natural world can be lost in the fluff. In a way, he is oblivious to the routines and customs of ordinary life. He recently bought a pair of shoes, after wearing only sandals, even in winter, since 1969. Three weeks ago, he went to a barber for the first time in 30 years—he had been wearing his hair in a ponytail and periodically hacking it off at the ends.
Yet though indifferent to fashion or fads, Dr. Conway is intensely aware of nature, and wants to know it deeply and intimately. He is infinitely curious and observant, seeing nature not only in a spider web or the details of a daffodil but in mathematics. Part of his way of getting to know the world is through an eclectic mixture of problems, ranging from the logic of quantum mechanics to the best way to pack eight-dimensional spheres, to devising an esthetically pleasing design for an artist who is going to plant a mountain with trees and wants to be sure that the design remains intact when trees are removed as the forest matures.
Dr. Conway was born and reared in Liverpool, England, the son of a laboratory assistant at the Liverpool Institute for Boys, a school that was attended by Paul McCartney and John Lennon of the Beatles. His talents in mathematics were always apparent. “My mother used to say she found me reciting the powers of 2 when I was 4 years old,” he said. “I don’t know if it’s true and I don’t know how far I got. It may be just one of those motherly stories.” When he went to school, he said, “I was universally good at everything; I was the top or second or third in my class in every subject.”
Yet mathematics always had a special appeal for Dr. Conway, and not just because he was good at calculations.
“This Other World”
“What turned me on was this mysterious relationship between things,” he said. “There is this wonderful world of logic and connections that is very difficult to see. I can see trees and cats and people, but there is this other world and it’s very, very powerful.”
He explained that mathematical logic, the sort of reasoning that ties abstract ideas together, fascinated him and made him wonder how real it all was. Were abstract mathematical constructions illusions or reality? Were they a creation of the human mind or were they describing the essence of reality? Or, is mathematics discovered or invented?
Dr. Conway, along with many other mathematicians, believes that mathematics is discovered because, over and over again, abstract mathematical ideas, found purely by thought and reasoning, have turned out to provide exact descriptions of the physical world.
For example, he has been doing research with Dr. Neil Sloane of Bell Labs on sphere packing, which involves figuring the best way to pack spheres into a particular space. The work would seem to be an abstract and useless exercise. But it turns out that the eight-dimensional sphere packing problem is exactly what is needed to find the best way to transmit data, like the dimensions of a sound wave made by a human voice, along telephone lines. The idea is to take every eight data points, which name a point in eight-dimensional space, round them off, and transmit the rounded point. The rounded point is the center of the nearest eight-dimensional sphere.
Book on Sphere Packing
“I find it really lovely that this purely geometrical thing that I’m interested in is actually useful to quite practical people,” Dr. Conway said.
Dr. Conway and Dr. Sloane recently wrote a book about their sphere packing work, which was reviewed in the journal Advances in Mathematics by Dr. Gian-Carlo Rota of the Massachusetts Institute of Technology.
It went, in part, like this: “This is the best survey of the best work in the best fields of combinatorics written by the best people. It will make the best reading by the best students interested in the best mathematics that is now going on.” Dr. Conway calls it the “best” review.
Dr. Conway got his start as a renowned mathematician in 1969, when he was a faculty member at Cambridge University, hoping desperately to make a mark on the world. He was married and had four little girls. “I knew that I was a good mathematician, but the world didn’t,” he said. “I was getting very depressed because I hadn’t lived up to the promise.”
Another mathematician announced that he had discovered a new mathematical object, a highly symmetrical creation, in 24-dimensional space. By studying this, mathematicians hoped to find a new group, the concept they use to understand symmetry. But the discovery was preliminary and required working out the rules and symmetries to establish the group. Dr. Conway decided to make the attempt.
“I had this great plan,” he recalled. “We were very poor at the time and I was doing lots of teaching to make ends meet. My plan was that I would work on it on Wednesday evenings from six until midnight and on Saturdays from 12 noon until midnight. I told my wife that this was very important.”
Surprisingly Quick Solution
He started work on a Saturday. “It was very funny,” he said. “Basically, I said goodbye to my wife, kissed the kids and stuck myself in the front room.” Twelve and a half hours later he emerged, to his own astonishment, with the task completed.
That was the turning point in Dr. Conway’s career. “It catapulted me into the jet set,” he said. Soon he was traveling around the world, much in demand to describe his work. “I remember flying to New York to give a 20-minute lecture and then flying back again,” he said.
The discovery “had a great psychological effect,” Dr. Conway said. “It cured this depression and it also totally removed my ambition. I’ve been totally successful, but at some level I couldn’t care less.” He used to dream of being like most of his colleagues who “think of very deep things,” he said. But with his momentous proof of the existence of what has come to be called the Conway Group, “that sort of removed that feeling.” He added, “I decided that I might as well enjoy myself instead.”
So what does he do to enjoy himself? For one, he indulges in his fascination with symmetries. He studies the petals of a daffodil, figuring out why there have to be an alternation of three true petals and three leaflike petals called setals. “I feel like I understand it,” he said. “It gives me a nice feeling of being in tune with the world. It’s a feeling of harmony.”
A Deep Look at Pi
Or he decides to memorize the first 1,000 digits of pi. This was a project that his second wife, Dr. Larissa Conway, a Russian-born mathematician with whom Dr. Conway has two little boys, instigated when one day she needed the value of pi and knew it only as 3.14.
“I taught her the first 100 places, but she wanted more,” Dr. Conway said. “I discovered I didn’t know any more,” he said, so he and his wife made it a project to memorize 100 digits a day. The two would practice together during their regular routines.
“It was really rather sweet,” Dr. Conway said. “We used to go on a walk on Sundays to the village and one person would recite 20 digits as we walked along, like a little poem, then hand it over to the next person and they would take over for the next 20 places.”
He went on: “As I’m getting older, I’m finding that rather than having a deep understanding of lots and lots of problems, I like just knowing lots and lots of things.
“I don’t mean facts—I mean things. Knowing what a daffodil looks like or the 633d digit of pi. Whatever it is. Some are show-offy. Most have no earthly use. I just like the feeling, and it is of some use to me professionally.”
Asked how he actually does research, Dr. Conway describes a life of the mind that to most experimental scientists would seem unbearably frustrating. “What happens most of the time is nothing,” he said. “You just can’t have ideas often.” When he does not know what to try to prove, he says, he will just start enumerating something. “I might try and list all the polyhedra with nice properties,” he said. “With any luck, I’ll see something.”
It is a method that gets him through the dry days, he said and one that eludes most young researchers. “One of the terrible things that happen to bright young graduate students is they’ll come in at 9 a.m. and write ‘theorem’ at the top of their paper. Then they might write ‘proof.’ Then they sit there staring at this thing. They get depressed, they go home at 5. They come in the next day and start again.” The problem, he explained, is that “there’s no way of proving a theorem to order. If someone comes up with a gun and says, ‘Prove this theorem,’ well, then, you’re just dead.”
Just an Ordinary Day
A typical day for Dr. Conway starts at about 8 a.m. when he goes to a local coffee shop and reads a newspaper over a breakfast of oatmeal. Then he turns on his computer, works out 10 dates and reads his e-mail. “There might be a math problem in the e-mail that might make me think,” he explained. He will often have very long telephone conversations with Dr. Sloane about the work they are doing together or he might go to Bell Labs to work. He is teaching two classes this semester.
“The point is, I don’t work,” Dr. Conway said. “I mean, honestly, if you or your readers saw what I actually did, they’d be disgusted. They’d say, ‘Good money is being paid out to support these people.’”
Dr. Conway said he used to feel guilty about the way he worked. “Then it suddenly dawned on me that that’s the way I produce good stuff—by lying around, doing nothing, thinking about what I like,” he said. And, he added, “I’ve been a lot more successful since I stopped feeling guilty.”
October 12, 1993
Claude Shannon, B. 1916—Bit Player
Halfway through the last century, information became a thing. It became a commodity, a force—a quantity to be measured and analyzed. It’s what our world runs on. Information is the gold and the fuel. We measure it in bits. That’s largely because of Claude Shannon.
Shannon is the father of information theory, an actual science devoted to messages and signals and communication and computing. The advent of information theory can be pretty well pinpointed: July 1948, the Bell System Technical Journal, his landmark paper titled simply “A Mathematical Theory of Communication.” Before that, no such theory existed. Suddenly, there it was, almost full grown.
To treat information scientifically, engineers needed to answer the kinds of questions they were asking about matter and energy: How much? How fast? For fundamental particles, an irreducible unit of measure, Shannon proposed the word “bits”—as shorthand (suitably compressed) for “binary digits.” A bit is a choice. On or off. Yes or no. One or zero. Shannon saw that these pairs are all the same. Information is fungible: smoke signals and semaphores, telegraph and television, all channels carrying bits.
Back then, the main technologies for sending and storing information were analog, not digital, so this was far from obvious. Phonograph records embodied sound waves in vinyl, and Shannon’s telephone-company employers trafficked mostly in wavy signals, too. Yet some interesting communications channels were not continuous but discrete: the telegraph and teletype.
Mainly, though, Shannon was thinking of electrical circuits. The marriage of on-off to yes-no meant that circuits could carry out something akin to logic. They could not only transmit bits; they could manipulate them. Not coincidentally, in that same year Bell Labs was preparing to announce a new invention: the transistor. “It is almost certain,” Scientific American declared bravely in 1952, “that ‘bit’ will become common parlance in the field of information, as ‘horsepower’ is in the motor field.” Sure enough, bits led to bytes and, inexorably, to kilobytes, megabytes, gigabytes and terabytes.
All that still rests on the theoretical foundation laid by this playful mathematician and electrical engineer. Shannon was born in rural Michigan in 1916, the son of a language teacher and a probate judge. He was an early and enthusiastic tinkerer in the new American style. Thomas Edison was his hero. Once he built a crude telegraph using a half-mile of barbed wire between his house and a friend’s.
Nor did he stop playing just because he grew up. At Bell Labs, and then as a professor at the Massachusetts Institute of Technology, he amused colleagues by building juggling machines, unicycles, chess-playing computers and robotic turtles. He left a body of work comprising more than a hundred technical papers along the lines of “Reliable Circuits Using Less Reliable Relays,” as well as others, not quite so influential, like “Scientific Aspects of Juggling” and “The Fourth-Dimensional Twist, or a Modest Proposal in Aid of the American Driver in England.” He was also the author of “A Rubric on Rubik Cubics,” which can be sung to the tune of “Ta-ra-ra-boom-de-ay.”
When modern theorists worry about compressing data, maximizing bandwidth and coping with noise, they use the tools Shannon provided. They also keep in mind a paradox he emphasized from the very beginning—one that is either lovely or perverse, depending on your point of view. Information, in its new scientific sense, is utterly divorced from meaning. Chaotic systems, and strings of random numbers, altogether meaningless, are dense with information.
The medium, it turns out, is not the message. Words, sounds, pictures or gibberish—it’s still just bits.
December 30, 2001
An Isolated Genius Is Given His Due
In some ways, mathematicians are finally beginning to penetrate the mind of Srinivasa Ramanujan.
One hundred years have passed since Ramanujan was born in the small city of Kumbakonam in southern India. When he died 32 years later, he left a strange, raw legacy, about 4,000 formulas written on the pages of three notebooks and some scrap paper.
Some of the power and originality of Ramanujan’s mathematics was understood a few years before his death. His contemporaries saw from the theorems scrawled across his pages that he possessed a genius for calculating the hidden laws and relationships that govern the wilderness of numbers.
But Ramanujan was uneducated in standard mathematics and isolated by geography for most of his productive life. Often his formulas seemed as obscure as they were elegant. He worked in a place of his own and a way of his own, drawing his formulas and theorems from a mental landscape that remained far from the frontier of mathematics as it was seen in his day.
Now his work is flowing into mathematics and science more deeply than could have been imagined a generation ago. Computers, with special programs to manipulate algebraic quantities, have made it possible for more ordinary mathematicians to pick up the trail of his thought. And modern physics, from the superstring theory of cosmology to the statistical mechanics of complicated molecular systems, finds itself turning more and more often to the pure findings of number theory and complex analysis—the worlds of Ramanujan.
So researchers are intensifying a process of forensic mathematics, or mathematical archeology—poring over the rough pages, trying to understand the formulas and prove them. As they learn more of why Ramanujan chose particular paths, they sense a foundation that has not yet been revealed.
“When he pulled extraordinary objects out of the air, they weren’t just curiosities but they were the right things,” said Jonathan M. Borwein of Dalhousie University in Halifax, Nova Scotia, one of many mathematicians who has lately found himself turning to Ramanujan’s formulas. “They are elusive evidence of a theory that’s lurking around somewhere that he never made explicit.”
The trail is hard to follow. Out of necessity and then perhaps out of habit, Ramanujan worked in a style that awes and frustrates modern mathematicians. He used a slate, jotting down formulas, erasing them with his elbow, jotting down more, and then recording a result in his precious notebook only when it had reached final form.
The intermediate results, the links of the chain, are lost. Unlike mainstream mathematicians, he felt no need to prove that a result was true. His legacy is simply a set of discoveries.
“A Feel for Things”
“He seems to have functioned in a way unlike anybody else we know of,” Dr. Borwein said. “He had such a feel for things that they just flowed out of his brain. Perhaps he didn’t see them in any way that’s translatable. It’s like watching somebody at a feast you haven’t been invited to.”
So mathematicians have spent years—often valuable and productive years—proving theorems that Ramanujan knew to be true. Deriving the formulas has often been more illuminating than the formulas themselves. Whole new subdisciplines within mathematics have blossomed around ideas that Ramanujan put forward in a peculiar, stark isolation.
With the special excuse of his centennial year, mathematicians are gathering to discuss the implications of Ramanujan’s work at meetings in the United States and India. They have far more raw material to work with than ever before, because the last decade has brought a new effort to find and organize the pages that make up his legacy.
A University of Illinois mathematician, Bruce Berndt, has spent years editing the notebooks, tracking down sources and relationships and, above all, proving as many of the unproved theorems as possible. A mathematician at Pennsylvania State University, George Andrews, has been performing the same task with the so-called Lost Notebook, 130 pages of scrap paper from the last year of Ramanujan’s life.
“The work of that one year, while he was dying, was the equivalent of a lifetime of work for a very great mathematician,” said Richard Askey of the University of Wisconsin, who has collaborated with Dr. Andrews in trying to understand some of Ramanujan’s work.
“What he accomplished was unbelievable,” Dr. Askey said. “If it were in a novel, nobody would believe it.”
Ramanujan might have died in complete obscurity if he had not written a series of desperate, bold letters to English mathematicians in 1912 and 1913. By then he was 25 years old, working as a $30-a-year clerk after several years of unemployment, unwilling to put aside his slate and formulas.
His family was Hindu, high-caste but poor. His father and grandfather before him worked as clerks for cloth merchants. Ramanujan was lucky enough to have a fairly good high school education in Kumbakonam, and he began his creative exploration of mathematics after discovering the few outdated and second-rank textbooks in the library there.
“An Unknown Hindu Clerk”
His intellect stood out clearly, but in college at Madras, about 150 miles north of his birthplace, he failed again and again to pass examinations in other subjects. In mathematics itself, he had no teacher. He worked, as the English mathematician Godfrey Harold Hardy later said, “in practically complete ignorance of modern European mathematics.”
Hardy was not the first mathematician to receive a letter from this “unknown Hindu clerk,” as he recalled—“at the best, a half-educated Indian.” But he was the first to understand what the letter contained.
Ramanujan’s letters said, in effect, I know the following … and I also know this … and, by the way, I have discovered this. He offered a carefully chosen selection of his theorems. Most were in the form of identities—statements that some familiar quantity, like pi, was equal to some unfamiliar quantity, or that two unfamiliar quantities were equal.
Hardy examined them with bewilderment. A few struck chords of recognition, he said later; he thought he had proved similar statements himself. Some he thought he could prove if he tried—and he succeeded, although with surprising difficulty.
Other theorems were already known. Still others, however, “defeated me completely,” Hardy said in an essay years later.
Journey to Cambridge
“I had never seen anything in the least like them before,” he said. “A single look at them is enough to show that they could only be written down by a mathematician of the highest class. They must be true because, if they were not true, no one would have had the imagination to invent them.”
Furthermore, Hardy could tell that Ramanujan was holding some things back, offering specific examples of theorems for which he surely must have discovered more general versions. He arranged an invitation to Cambridge University, and in 1913 Ramanujan arrived, leaving his wife behind. He stayed for nearly six years.
The two men collaborated often. Hardy remembered a slight man, of medium height, with eyes through which some light seemed to shine. Ramanujan remained a strict vegetarian, cooking all his own food in his rooms, and when he fell mysteriously ill in 1917, Hardy thought his vegetarianism contributed to his failing health.
Shared Fascination
Years later, Hardy took some pains to dispel the idea, perhaps a by-product of subtle English racism, that Ramanujan was some sort of Asian curiosity—either an “inspired idiot” or “some mysterious manifestation of the immemorial wisdom of the East.” On the contrary, in Hardy’s eyes he was a deliberate rationalist, often shrewd, and not nearly so religious as his dietary habits made him appear.
They shared a fascination with numbers as almost living things, or characters in a story. They thought about round numbers, defined as numbers with only small factors, like 300, 22 × 3 × 52. They worked on the question of how common such numbers are, in strict mathematical terms, and on many problems more difficult to put into words.
“A Very Interesting Number”
One day after Ramanujan fell ill, Hardy visited him in a taxicab and remarked that the cab’s number had been rather uninteresting—1729, or 7 × 13 × 19. “No, it is a very interesting number,” Ramanujan responded, as Hardy later told the story. “It is the smallest number expressible as a sum of two cubes in two different ways.” (It is the sum of 1 × 1 × 1 and 12 × 12 × 12, and it is also the sum of 9 × 9 × 9 and 10 × 10 × 10.) Hardy understood and appreciated Ramanujan more than any of his contemporaries. But even he could not see beyond the blinkers of his time and place. To him, Ramanujan’s story was ultimately a tragedy—of inadequate education and of genius unguided. When he finally came to assess the younger mathematician’s work and its likely influence on the future of his subject, he expressed disappointment.
“It has not the simplicity and the inevitableness of the very greatest work,” Hardy wrote in 1927. “It would be greater if it were less strange.”
Few mathematicians accept that assessment today, as strangeness comes into the light and Hardy recedes into Ramanujan’s greater shadow.
“Hardy thought it was a shame that Ramanujan wasn’t born a hundred years earlier,” Dr. Askey said. That was the great age of formulas, the era of ground-laying work by such mathematicians as Euler and Gauss. “My comment is that it’s a shame Ramanujan wasn’t born a hundred years later,” he said. “We’re trying to do problems in several variables now—the problems are harder, and it would be marvelous to have somebody with his intuition to help get started.”
Not that his intuition was infallible. Ramanujan made some errors, once claiming to have found a formula for the approximate number of primes less than any given number. No such formula exists. He was too optimistic, and it was the optimism of an earlier time; by the 19th century, mathematicians had learned that some problems could never be solved, but Ramanujan’s isolation shielded him from their doubts as much as from their knowledge.
In 1919, increasingly ill, having entered and left a nursing home and several sanitariums, Ramanujan returned to India. He continued to work feverishly, fighting the pain of his mysterious ailment, writing on whatever paper he could find. The next April, at the age of 32, he died.
Papers Discovered in 1976
The work of his last year, 130 unlabeled pages, came to rest at the library of Trinity College, Cambridge, where they lay in a box, along with assorted bills and letters, until Dr. Andrews of Pennsylvania State University found them in 1976. This was the Lost Notebook.
“It’s a bizarre term to use for something that was in the major library of the major college in England,” Dr. Borwein said, “but in terms of people appreciating its contents, it was certainly true.”
Dr. Andrews found that Ramanujan had cleared a path that mathematicians had not succeeded in matching in the intervening half century. Many discoveries concerned a family of identities he called mock theta functions—“simple assertions in arithmetic,” as Dr. Andrews put it, although “their implications are quite profound.”
Seeds of “Ramanujan’s Garden”
Such mathematics has helped drive one of the major new conceptions of theoretical physics, superstring theory, as the physicist Freeman Dyson told a Ramanujan conference last month. “As pure mathematics, it is as beautiful as any of the other flowers that grew from seeds that ripened in Ramanujan’s garden,” he said.
Another identity was used last year to enable a computer to calculate millions of digits of pi. It converges on the exact value with far greater efficiency than any previous method. Yet, as always, Ramanujan had merely asserted his discovery; only later did Dr. Borwein and his brother, Peter B. Borwein, prove rigorously that those millions of digits really were pi.
The applications of Ramanujan’s magical-seeming formulas make mathematicians think that he was mining a deep vein of theory, the full outlines of which are not yet known. But many prefer not to dwell on just how Ramanujan was able to think as he did.
Hardy looked at Ramanujan’s origins and saw a crippling neglect by an inadequate educational system cut off from European society. Still, as mathematicians realize now, Ramanujan had a decent high school, a handful of books and the traditions of a culture that allowed him to aspire to a life as a scholar.
Those looking for lessons in his brief, rich life sometimes note that now, one century later, much of the planet lacks that much.
“Ramanujan is important not just as a mathematician but because of what he tells us that the human mind can do,” Dr. Askey said. “Someone with his ability is so rare and so precious that we can’t afford to lose them. A genius can arise anywhere in the world.”
A Mysterious Formula for Pi
Mathematicians find many of Ramanujan’s formulas to be both beautiful and obscure. To their surprise, the formula above provides an extremely rapid way to calculate the value of pi, an age-old preoccupation. Only last year, a computer scientist used a version of Ramanujan’s formula to calculate pi to 17 million places. Only after this success were mathematicians able to prove why Ramanujan’s insight was correct.
July 14, 1987
Scientist at Work: Andrew Wiles; Math Whiz Who Battled 350-Year-Old Problem
Dr. Andrew Wiles is a quiet, diffident man with a shy smile. Yet he summoned the courage to invest seven years working in secret on the world’s most famous math problem, a formidable challenge that has baffled the best mathematicians for more than 300 years.
Last week, despite all odds, he was able to announce that he had solved it. It was a feat that, if sustained, will catapult the 40-year-old mathematics professor at Princeton University into the ranks of his subject’s giants, assuring that the pinnacle of his career had been reached and making him, instantaneously, a sort of icon.
The problem, known as Fermat’s Last Theorem—and familiarly as F.L.T. to its adherents—dates to 1637. The illustrious French mathematician Pierre de Fermat scribbled it in the margin of a book, adding that he had a marvelous proof that, unfortunately, would not fit in the margin. Great and not-so-great mathematicians have been trying to prove it ever since.
Dr. Wiles’s proposed proof could still turn out to have a subtle flaw, some mathematicians warn. His 200-page manuscript has not yet been published. And it will take time for experts to check it fully. But unlike the many previous claims, all of which turned out to be flawed, Dr. Wiles’s work has been hailed and by the leaders of the field.
Already, in Princeton, a town where people routinely drive visitors past the house where Albert Einstein lived, Dr. Wiles’s celebrity has begun. Dr. Simon Kochen, chairman of Princeton’s mathematics department, said he was receiving congratulations even from strangers.
Dr. Wiles has been all but impossible to find since he announced his proof at Cambridge in England last week, so the thousands of telephone calls, computer messages, and faxes from around the world have rained in on others, like Dr. Kochen and other members of Princeton’s mathematics department instead.
“I don’t know what it is,” Dr. Kochen said. After all, he said, mathematics is practiced by a few thousand professionals and it often seems to be of little interest to the outside world. But with Dr. Wiles’s proof, he added: “It’s like the human spirit still lives. It’s like listening to one of the late Beethoven quartets. It’s like we live now and this happened during our lifetimes.”
So why Dr. Wiles? And why now? Dr. Wiles, in an interview on Sunday at his office and at his house a few blocks away, explained that finding the proof was a matter of being in the right place at the right time. And it was a matter of being obsessed with the problem.
Fermat’s Last Theorem is deceptively simple to state. It says that there are no positive whole numbers that solve the equation xn + yn = zn when n is greater than 2. When n is 2, solutions are easy to find. For example, 32 + 42 = 52. But that, by Fermat’s Last Theorem, is the end of the line. There are no solutions for when n is 3 or any greater number.
Dr. Wiles says he has been fascinated by Fermat’s Last Theorem since childhood. It was the theorem that drew him into mathematics.
A Boyhood Dream
The son of a theologian at Oxford University in England, Andrew Wiles first came across Fermat’s Last Theorem when he was 10 years old and saw it in a book his town’s public library. He has forgotten the book’s title and author, but he vividly remembers its effect. It made him want to be a mathematician and it made him want to solve the problem.
“I spent much of my teenage years trying to prove it,” Dr. Wiles recalled. “It was always in the back of my mind.” But once he became a professional mathematician, he said, he realized that there was a lot more to it than simply working on the problem with a teenager’s enthusiasm.
The problem had foiled mathematicians from Fermat’s day onward. Fermat himself proved that it was true when the n’s, called the exponents in the equations, were 4. A hundred years later, in 1780, the mathematical genius Leonhard Euler proved the theorem was true for the exponent 3, and over the next 50 years others proved it true for the cases of 5, 7 and 13. But Fermat’s majestic theorem refers to an infinity of numbers. The one by one approach would never prove it.
The turning point came about a decade ago when Dr. Gerhard Frey of the University of the Saarlands in Germany suggested that Fermat’s Last Theorem would follow automatically if another, seemingly unrelated, proposition were true. That proposition, known as the Taniyama conjecture, dealt with mathematical objects known as elliptical curves, a class of cubic equations that had been intensively studied by Fermat himself, although not in connection with his theorem.
Then, seven years ago, Dr. Kenneth Ribet, a mathematician at the University of California at Berkeley, formalized Dr. Frey’s suggestion, showing that if a crucial part of the Taniyama conjecture could be proved, Fermat’s Last Theorem would be true.
Dr. Ribet said that the Taniyama conjecture was part of a sort of grand unified theory of mathematics. “The Taniyama conjecture is part of the vast Langlands philosophy,” Dr. Ribet said. He explained that Dr. Robert Langlands, a professor at the Institute for Advanced Study in Princeton, suggested years ago that two apparently disparate fields of mathematics were actually one and the same.
The fields are algebra, which deals with equations, and analysis, which, like calculus, deals with how mathematical structures vary around the neighborhood of a point on a surface. The Langlands philosophy, Dr. Ribet said, “is a deep, far-reaching vision in mathematics.” The Taniyama conjecture, he added, “is a special case of what Langlands suspected.”
Dr. Ribet’s proof linking the conjecture to Fermat’s Last Theorem fired Dr. Wiles’s imagination. It said, essentially, that you could associate an elliptic curve with a solution to the Fermat equations. If you could just show that the elliptic curve could not exist, then you could show that neither could solutions to the Fermat equations.
“I knew when I heard about Frey and Ribet’s result that the landscape had changed,” Dr. Wiles said. “There is no question that what they did completely changed the problem for me psychologically. Hitherto, Fermat’s Last Theorem was like other problems in number theory. It would go on for thousands of years and never be solved and mathematics would hardly notice.”
But, Dr. Wiles added: “What Frey and Ribet did was make Fermat’s Last Theorem a consequence of a problem that mathematics could not ignore. Too much depended on it. A whole architecture of future problems depended on it. For me, that meant that the problem was going to be solved. And once I had that confidence, I couldn’t let go.”
The very day that Dr. Wiles heard about Dr. Ribet’s result, he dedicated his life to using it to prove Fermat’s Last Theorem.
He worked at home on his secret project, sitting in a barren attic office on the third floor of his Tudor-style house. No computer was necessary, and no telephone was present to intrude on the absolute silence.
“If he said he was working on Fermat’s Last Theorem, people would look askance,” Dr. Kochen said. “And if you start telling people who are experts, you end up collaborating with them. He wanted to do it on his own.” Only one person in the Princeton mathematics department knew what was going on. That was Dr. Nicholas Katz, who agreed to serve as sort of a sounding board for Dr. Wiles. But Dr. Katz was sworn to secrecy.
“I made progress in the first few years,” Dr. Wiles said. “I developed a coherent strategy.” Then, he said, two years ago, he had a pivotal idea that reduced the problem to a calculation. The calculation was “one that was similar to one others had tried and hadn’t achieved, but it was a problem that was doable,” he said.
Dr. Wiles worked feverishly on it. “Basically, I restricted myself to my work and my family,” Dr. Wiles said. “I don’t think I ever stopped working on it. It was on my mind all the time. Once you’re really desperate to find the answer to something, you can’t let go,” he said.
Eventually, Dr. Wiles said, he had solved all but one critical case for his proof. The final barrier fell about six weeks ago when Dr. Wiles was reading a paper by Dr. Barry Mazur, a mathematician at Harvard University. “I can’t even remember why I was looking at it,” Dr. Wiles said. But he took notice of the mention of a certain construction. “It was immediately clear to me that I should use this construction and that I would solve this last problem I had.”
The construction, as it happened, was not new. It dates to the 19th century. But Dr. Wiles had not heard of it before. As soon as he saw it, Dr. Wiles said, “I knew I had it.” He could complete his seven-year quest and the proof of Fermat’s Last Theorem.
Dr. Wiles presented his work in three lectures that he gave last week at a small mathematics meeting at Cambridge University in England.
Unlike many scientists who trumpet every tiny advance in papers and press release, Dr. Wiles gave no hint of the bombshell he was about to detonate over the world of mathematics. The audience was not even certain he would mention Fermat, though some had their suspicions from the drift of the argument. Between lectures, e-mail messages started flashing between England and the United States as mathematicians speculated on his target.
One reported, “Andrew gave his first talk today. He did not announce a proof of Taniyama-Weil but he is moving in that direction and he still has two more lectures. He is still being very secretive about the final result.”
Dr. Kochen, waiting back in Princeton, was tense with anticipation. He and another Princeton mathematician, Dr. John Conway, could talk of nothing but whether the proof would be accepted by the handful of mathematicians who understood the field.
“Tuesday night, neither of us could sleep,” Dr. Kochen said. Around 5:30 Wednesday morning, Dr. Conway unlocked the door to Fine Hall, the brown tower that houses the mathematics department, went into his office, and turned on his computer. The first message arrived at 5:53 a.m. from Dr. John McKay of Concordia University in Montreal, who was attending the meeting. “F.L.T. proved by Wiles,” it said.
Not until the end of the third and last lecture had Dr. Wiles cited the proof in which his argument culminated.
Dr. Wiles arrived home on Friday. On Sunday, elated but tired, he said that he had spent his time with his wife and young daughters. “I’ve been to the swings,” he said, and to a party given by friends. He has tried to absorb the consequences of his achievement.
It is also a release to have completed the proof. “I haven’t let go of this problem for nearly seven years. It’s gone on on a day-to-day basis. I’ve almost forgotten the experience of getting up and thinking about something else,” he said.
Yet the release is accompanied by a bittersweet feeling at seeing this most famous of all mathematics problems vanish.
There is a certain sadness in solving the Last Theorem, Dr. Wiles said. “All number theorists, deep down, feel that,” he said. “For many of us, his problem drew us in and we always considered it something you dream about but never actually do.” Now, he said, “There is a sense of loss, actually.”
June 29, 1993
Scientist at Work: Leonard Adleman; Hitting the High Spots of Computer Theory
There is nothing to look at in Dr. Leonard Adleman’s office at the University of Southern California, no clue that the office is even occupied. There are no pictures of his wife or of his three daughters, no cartoons or mementos—just a computer, two chairs, a desk and a blackboard. And that is fine with Dr. Adleman. For although he is an active faculty member at the university, although he is a devoted husband and father, his is a life of the mind.
It is a life that is nourished by deep philosophical questions and the overarching beauty of mathematics. It is a life that involves days, weeks, months of pure thought, alone in his equally barren office at his home in Northridge, Calif., 30 miles from the campus. And it is a life that has led Dr. Adleman to play a central role in some of the most surprising, and provocative, discoveries in theoretical computer science.
Dr. Adleman is perhaps best known for a project that he only reluctantly got involved in. He says now that he failed to understand its significance at the time. The discovery, which he made with two other researchers, was of an entirely new sort of encryption system that changed investigators’ thinking about what it meant for a code to be unbreakable.
After that, Dr. Adleman invented a new way of demonstrating that a number was prime, based on a probabilistic mathematical proof that challenged the notion, dating to the ancient Greeks, that a proof must offer utter certainty.
It was one of Dr. Adleman’s students who invented the first computer virus, and Dr. Adleman who coined the term “computer virus.”
And, in one of the few disappointments of his career, he hit upon an idea for slowing the loss of the white blood cells in people with AIDS, based on an analysis of graphs of the decline of immune system cells in those infected by the human immunodeficiency virus. Although Dr. Adleman is still sure he was right, he failed to communicate his enthusiasm to the medical community.
Last month, in a pioneering experiment that astonished computer scientists, Dr. Adleman combined his knowledge of two fields and published a paper showing that DNA, the biological material, could be used as a computer, solving a math problem with a laboratory experiment.
Dr. David Jefferson, a computer scientist at the University of California at Los Angeles, said he was “incredibly impressed” by Dr. Adleman’s accomplishments, especially because Dr. Adleman works alone most of the time. “It is rare to make contributions in several fields and work alone,” Dr. Jefferson said.
Yet despite his successes in a field on the borderline between mathematics and computer science that seems made for him, Dr. Adleman, 48, floundered for years trying to decide what he wanted to do with his life. When he finally fell into mathematics and theoretical computer science it happened almost by chance.
Dr. Adleman grew up in San Francisco. His father was an appliance salesman and his mother a bank teller. He did not have any great ambitions for himself and, in fact, never even thought about becoming a mathematician. It was not until he took a high school course in Shakespeare that he even realized the beauty of ideas. “I was an incredibly naive and immature kid,” he said, but when he took that class, and, in particular, when he read Hamlet, the teacher, “opened my eyes to the fact that one could see things more deeply than the purely superficial.” He enrolled at the University of California at Berkeley only because his Shakespeare teacher suggested it.
At Berkeley, he at first declared he was going to be a chemist, inspired by years of watching Mr. Wizard on television, and then changed his mind and said he would be a doctor, inspired by his Kappa Nu fraternity brothers who wanted to be doctors. Finally, he settled on a mathematics major. “I had gone through a zillion things and finally the only thing that was left where I could get out in a reasonable time was mathematics,” he said.
Dr. Adleman graduated in five years, in 1968, “wondering what I wanted to do with my life.” He took a job as a computer programmer at the Bank of America, and soon after applied to medical school. He was accepted but changed his mind and did not enroll. Then, he said, “I decided maybe I should be a physicist,” so he began taking classes at San Francisco State College while working at the bank.
Once again, Dr. Adleman lost interest. “I didn’t like doing experiments, I liked thinking about things,” he said. So he simply stopped going to class. Afraid that incompletes turning to F’s would hurt him later, he took a book on computer science out of the college library and never returned it, figuring that the college would not send out a transcript if a student had an unpaid library fine. “I’ve felt guilty ever since,” he said. “Maybe I should send the college an anonymous gift.”
A New Way to Foil Electronic Spies
Dr. Leonard Adleman’s approach to encryption, shown in the following graphic, used “keys” in the form of mathematical formulas that are easy to compute in one direction, but believed to be impossible for an outsider to reverse.
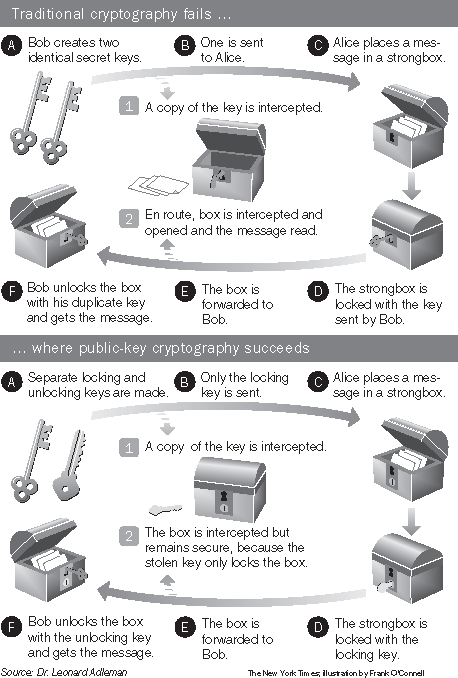
Finally, he returned to Berkeley, with the aim of getting a Ph.D. in computer science. He had two motivations. One was practical. “I thought that getting a Ph.D. in computer science would at least further my career,” he said.
The second was more romantic. Dr. Adleman had read a column in Scientific American by Martin Gardner on one of the most profound mathematical discoveries of the 20th century, a theorem by Kurt Gödel, and was overwhelmed by the deep philosophical implications. Gödel had shown that there are some truths that can never be verified and some untrue statements that can never be proved false. He showed that no matter how mathematicians design a logical system of axioms, there always will be some statements that are outside the system and beyond the reach of its logic.
“I thought, ‘Wow. This is so neat,’” Dr. Adleman said. “There were several things I found neat—black holes, general relativity. I thought that for once in my life, I want to really understand one of these deep results.” When he went to graduate school this time, he decided, he would come away understanding Gödel’s result at a level beyond the superficial.
But while in graduate school, something else happened to Dr. Adleman. He finally understood the true nature and compelling beauty of mathematics. He discovered, he said, that mathematics “is less related to accounting than it is to philosophy.”
“People think of mathematics as some kind of practical art,” Dr. Adleman said. But, he added, “the point when you become a mathematician is where you somehow see through this and see the beauty and power of mathematics.”
Dr. Adleman got his Ph.D. in 1976 and immediately landed a job as an assistant professor of mathematics at the Massachusetts Institute of Technology. (His father advised him to stay with Bank of America, where at least they had a good retirement plan.)
One day, soon after he arrived, he walked into the next-door office of Dr. Ronald Rivest. Dr. Rivest was gripped by an article that had just appeared in an electrical engineering journal, IEEE Transactions on Information Theory. The paper, by Dr. Martin Hellman, a computer scientist at Stanford, and his student Whitfield Diffie, described an idea for a new sort of encryption system. All previous schemes had relied on secret “keys”—formulas for scrambling messages and unscrambling them. Anyone who had a key to encrypt could also decrypt by simply reversing the encryption instructions. But Mr. Diffie and Dr. Hellman had a revolutionary idea. They proposed using one-way functions—mathematical formulas that are easy to compute one way but impossible to reverse unless you have some special knowledge of how they were constructed. You could make your encryption key public so anyone could send you an encoded message. But only you would have the key to decoding it.
Dr. Rivest announced that he would find such a one-way function and create a public key cryptosystem. Although it seemed clear that the idea should work, finding a one-way function that was truly one-way was not so easy. Dr. Adleman greeted the idea with a polite yawn, thinking it impractical and not very interesting. Nonetheless, Dr. Rivest and another colleague, Dr. Adi Shamir, now at the Weizman Institute of Science in Israel, were truly excited by the concept.
“They would talk endlessly about it and because we were all together so much, we would discuss it,” Dr. Adleman said. Soon Dr. Rivest and Dr. Shamir were trying to invent an unbreakable public key system. Dr. Adleman’s role was to test each proposed system by trying to break it. Dr. Rivest and Dr. Shamir invented 42 coding systems and each time Dr. Adleman broke the code.
Late one night, following a Passover dinner, Dr. Rivest called Dr. Adleman with the 43d idea, this one based on a difficult factoring problem. Dr. Adleman said he thought this time the code really was unbreakable because of the mathematics involved. The number would take years or even centuries of computing to factor. The makers of the code could decrypt it because they had constructed the large number and so they knew its factors.
Dr. Rivest stayed up all night working on a manuscript describing the code and the next morning he handed it to Dr. Adleman. He had listed the paper’s authors, in alphabetical order, as Adleman, Rivest, Shamir.
“I told Ron, ‘Take my name off the paper. It’s your work,’” Dr. Adleman said. But Dr. Rivest insisted.
“I thought, ‘Well, it’s going to be the least important paper I’ve ever been on, but in a few years I will need so many lines on my vita to get tenure,’” Dr. Adleman recalled. “And, on the other hand, I did do a substantial amount of intellectual work breaking the codes 1 through 42. So the reasonable thing to do is be the third author.”
Mr. Gardner wrote about the code, now called R.S.A. for the names of its authors, in his column. To Dr. Adleman’s astonishment, the investigators and their code were instantly famous. Thousands of people wrote to them. “It just shocked us,” Dr. Adleman said. The National Security Agency, which until then had been essentially the only place where encryption was studied and had kept its work secret, complained that the publication of seemingly unbreakable codes like R.S.A. could endanger national security.
“People kept telling me I was going to get rich,” Dr. Adleman recalled, so he went out and bought a new car, a red Toyota, a hopeful investment since he was making just $13,000 a year at M.I.T.
The three investigators assigned the patent for their code to M.I.T. and in 1983 formed a company, R.S.A. Data Security Inc. of Redwood City, Calif., to make R.S.A. computer chips. “I was the president, Ron was chairman of the board, and Adi was treasurer,” Dr. Adleman said. He still owns stock in the company, but, he said, after he “drove the company into the ground,” he no longer is an officer. The R.S.A. chips are widely used, but the privately held company has yet to make anyone rich.
Although he was intellectually stimulated at M.I.T., Dr. Adleman longed for California and he wanted to marry and have a family. So, in 1980, he took a job at the University of Southern California and left for Los Angeles. Three years later, he met his future wife, Lori Bruce, at a singles dance. “It was love at first sight,” Dr. Adleman said, and the two were married six weeks later.
The first computer virus was born on Nov. 11, 1983, when one of Dr. Adleman’s students, Fred Cohen, told Dr. Adleman he had a new idea for a computer security threat and asked if he could use the university’s computers to try it out. “The second he described it to me, I thought there was no point in doing the experiment,” Dr. Adleman said. “It was obvious it would work.”
Dr. Adleman named the program a computer virus and had long, agonizing discussions with Mr. Cohen about the ethics of its creation. Finally, they decided that it was best to publicize the viruses to warn people that such programs could be made. Mr. Cohen set his virus loose and it quickly spread throughout the university’s computers. Mr. Cohen wrote his Ph.D. thesis on computer viruses.
Dr. Adleman’s mode of working is to find something that intrigues him and to dig in. He does not read mathematics journals, he says, because he does not want to be influenced by other people’s ideas.
When he decided, recently, to see if he could use DNA, the genetic material, as a computer, he spent a year in a molecular biology lab learning the techniques and what experiments were possible. He wanted to translate a mathematical problem into the language of biology and then exploit the incredible efficiency and speed of biological reactions to solve it. He spent six months thinking of the perfect problem to ask DNA to solve before deciding upon a problem involving finding a path connecting seven cities. And once he found the problem, it was like the computer virus. The actual experiment was superfluous. It was obvious that it would work, he said. But he did the experiment anyway, to enhance his chances of getting the paper published. His paper describing the work appeared in the journal Science on Nov. 11.
Asked what it is like to simply sit and think for six months, Dr. Adleman responded: “That’s what a mathematician always does. Mathematicians are trained and inclined to sit and think. A mathematician can sit and think intensely about a problem for 12 hours a day, six months straight, with perhaps just a pencil and paper.” The only prop he himself needs, he said, is a blackboard to stare at.
December 13, 1994
Dr. Kurt Gödel, 71, Mathematician
Dr. Kurt Gödel, regarded by some mathematicians as the world’s leading logician, died yesterday at the Medical Center in Princeton, N.J. He was 71 years old and lived at 145 Linden Lake in Princeton.
Dr. Gödel formulated Gödel’s Theorem, which became a hallmark of 20th-century mathematics and generated tremendous strides in mathematical logic and the foundations of modern mathematics.
The theorem states that in any rigidly logical mathematical system there are propositions or questions that cannot be proved or disproved on the basis of the axioms within that system and that, consequently, it is not certain that the basic axioms of mathematics will not raise contradictions.
The scholar had formulated the theorem in 1931 while he was teaching at the University of Vienna. He was also a member of the Institute for Advanced Study in Princeton in 1933 and 1935 and from 1938 to 1952. He became a professor there in 1953 and retired in 1976 as professor emeritus.
“More Than a Monument”
In 1951, Dr. Gödel was the co-recipient of the first Albert Einstein Award for achievement in the natural sciences. The award has been termed the highest of its kind in the United States. His work in mathematical logic, according to the awards committee composed of distinguished scientists, constitutes “one of the greatest contributions to the sciences in recent times.”
In presenting the award, Dr. John von Neumann of the institute said, “Kurt Gödel’s achievement in modern logic is singular and monumental—indeed it is more than a monument, it is a landmark which will remain visible far in space and time.”
Gödel “was the first man to demonstrate that certain mathematical theorems can neither be proved nor disproved with the accepted, rigorous methods of mathematics. In other words, he demonstrated the existence of undecidable mathematical propositions. He proved, furthermore, that a very important specific proposition belonged to this class of undecidable problems—the question as to whether mathematics is free of inner contradictions. The subject of logic will never be the same.”
The logician won many honorary doctorates from major institutes. A citation from Harvard described him as the “discoverer of the most significant mathematical truth of this century, incomprehensible to laymen, revolutionary for philosophers and logicians.”
January 15, 1978
Genius or Gibberish? The Strange World of the Math Crank
The letter, dated Christmas Day 1998 and addressed to a professor at the Niels Bohr Institute in Copenhagen, began portentously: “Nowadays, we seek to comprehend our comprehensions and call that comprehensiveness knowledge in the mistaken belief that as a science it is immortal. Such omniscience diffuses like Helium-3 into the penetralia mentis of omnipotent impotency within any God-head such that any caveat actor is saved….”
Within a few sentences, the writer was holding forth on Heisenberg’s Uncertainty Principle and “the concept of nothing” as the empty set, before launching into speculations involving number theory: “It’s enough to make me conjecture that infinity’s prime and Riemann’s Zeta function accounts for fractional charge subatomically just for the Higg’s boson with an involucral matrix of ogdoad parity as midwife!”
The letter was typed single-spaced with the tiniest of margins and embellished with hand-drawn diagrams and colored annotations. Copies were sent to a list that included the linguist Noam Chomsky, the physicists John Archibald Wheeler, David Deutsch and Stephen Hawking, and the mathematician John Casti.
“It has all the hallmarks of a crank,” said Dr. Casti, who is affiliated with the Technical University of Vienna and the Santa Fe Institute in New Mexico. “It’s amazing all the stuff you can get onto a single piece of paper.”
But was it not just possible that couched in the obscure mix of mathematics, physics and Egyptian mysticism (“ogdoad parity” refers to four pairs of gods with names like Darkness, Absence and Endlessness), there lay an important insight?
Didn’t two Cambridge University mathematicians dismiss the great self-taught Indian number theorist Srinvasa Ramanujan as a crackpot when he sent them long eccentric letters from India early in this century? Only their colleague G. H. Hardy had the foresight to recognize Ramanujan as a genius. And didn’t the great German mathematician Carl Friedrich Gauss foolishly throw away unread a groundbreaking paper from his young Norwegian colleague Niels Heinrik Abel, calling it “another of those monstrosities”?
Dr. Casti was not too worried about the possibility. Though the stories of Ramanujan and Abel may linger in the backs of mathematicians’ minds as they aim the latest unsolicited epistle toward the wastebasket, most become quickly jaded.
“After several hundred of these things you get into that mode,” said Dr. Ian Stewart, a mathematician at Warwick University in England. “It has to do with your self-preservation.
“The writers of these letters range from pretty good amateur mathematicians who have made a mistake somewhere or skipped over an important step to people who are completely mad,” he said. “You get very strange mail in 17 different fonts and 14 colors and with an idiosyncratic grammar.” Many of the correspondents are intelligent, well-meaning, indefatigable souls who, in their untrained way, share the fascination mathematicians feel for the invisible world of numbers. And many are simply cranks.
The equipment necessary for discovering a subatomic particle in your own home costs too much and would never fit inside a basement laboratory or even a very large backyard. But with nothing more than a pencil and paper, and maybe a compass and straightedge, even an amateur can explore the mathematical nether world, stumbling across important new truths.
That, anyway, is the dream or obsession that drives would-be Ramanujans all over the world to send mathematicians letter after letter crammed full of diagrams and equations promising answers to unsolved problems ranging from squaring the circle or proving (in a simpler way) Fermat’s Last Theorem to revealing, through the wonders of mathematics, the meaning of life.
Physicists get their share of mail from amateurs attempting to reconcile quantum mechanics and general relativity or to show that Einstein was wrong. But the greater ease with which one can speculate about numbers has caused the mathematical crank to become enshrined in academic folklore. The phenomenon is even documented in a book called Mathematical Cranks (Mathematical Association of America, 1992), by Dr. Underwood Dudley, a mathematician at DePauw University in Indiana.
“I’ve been at this for a decade and still can’t pin down exactly what it is that makes a crank a crank,” said Dr. Dudley, who has met a few in person. “They are usually men, old men,” he said. “All are humorless. None of them are fat,” a characteristic he attributes to their obsessive personalities. “It’s like obscenity—you can tell a crank when you see one.”
With recent films like Good Will Hunting and Pi giving mathematics a romantic sheen and popular new biographies romanticizing the lives of the mathematicians Paul Erdos and John Nash, the flow of crank mail will only increase, predicted Dr. John Allen Paulos, a mathematician at Temple University in Philadelphia.
Add millennial anxiety, including the Y2K problem, Dr. Paulos speculated, and the time he and his colleagues spend opening and discarding letters peppered with strange symbols and grand claims is bound to increase. “Popular mathematics used to be an oxymoron,” he said, “but happily that’s not true anymore.” The celebrity, though, has its price.
“It’s a real dilemma,” said Dr. Reuben Hersh, professor emeritus of mathematics at the University of New Mexico in Albuquerque. “Who is going to stroll through pages and pages of stuff that is very hard to understand when you don’t have to do it? From the point of view of these guys, we are arrogant, unwilling to reconsider ideas. And why shouldn’t they expect a responsible scientist to look carefully at some new idea that might be important?
“You can’t just say Ramanujan was a genius and these other guys were cranks,” Dr. Hersh said. “With a superficial look, there is hardly any visible difference. There is not always a sharp line between eccentric mathematicians and intelligent but maybe obsessed amateurs.”
Until recently much of the mail contained supposed proofs of Fermat’s Last Theorem. But since Dr. Andrew Wiles of Princeton University recently proved this famous puzzle and number theory, Dr. Paulos said, the focus has shifted to disproving Dr. Wiles. Another favorite diversion is Goldbach’s Conjecture, which holds that all even numbers are the sum of two primes. Though no one has found a counter example, this would-be theorem remains unproven, unless the solution has been crumpled up in a math department wastebasket somewhere.
Mathematicians are especially impatient with letters claiming to have solved one of the three classical problems of Greek mathematics: trisecting an angle (dividing it into three equal parts), doubling a cube, or squaring a circle (constructing a square with the exact same area as a circle) using only a compass and an unmarked straightedge. The reason for imposing these restrictions was to see how much mathematics could be derived from two basic concepts, the line and the circle.
Solving the three classic problems this way has been proved impossible. In 1882, for example, the German mathematician Ferdinand Lindemann established that trying to square the circle was hopeless. The reason is that pi, the ratio of the circumference of a circle to its diameter, is not only irrational (its infinitely long string of decimal places never repeats) but transcendental (it is not a solution of any polynomial equation with whole number coefficients).
Amateur attempts at squaring the circle generally amount to rounding off pi to a close approximation like 22/7 or 355/113. At best the result is a square that is very close but never exactly the size of the circle.
“These people don’t know what impossible means,” Dr. Dudley lamented. “They think it just means very hard.”
Or the writers do not believe the impossibility proofs. “They start by saying they know there is a theorem that says it is impossible but they’ve done it anyway, so there must be something wrong with the theorem,” Dr. Stewart said. “They are operating in a conceptual vacuum. It leads to strange things.”
One of the rules mathematicians soon learn is never to answer a possible crank. The mail often comes with cover letters assuring that the work has been “endorsed” by a certain Harvard or Stanford professor, say, or even a member of the United States Senate. The attached letters are usually no more than polite brushoffs: “Thank you for your interesting letter….” A mathematician who makes a perfunctory reply can count on its being stapled to the next round of mailings.
Dr. Hersh replied to a letter from an amateur mathematician in India. “This guy wrote very well,” he said, “in a good expository style. His penmanship was fine. What he said made enough sense that I thought I would try to explain and straighten him out.”
Over several years, Dr. Hersh realized that his correspondent had come to believe that the whole edifice of mathematics was about to crumble because of a dreadful mistake made centuries ago. Dr. Hersh finally wrote back in exasperation: “I’ve done all I can for you. I can’t do anymore.” The writer answered that he did not consider himself Dr. Hersh’s student but rather his opponent in a debate.
Surprisingly, the rise of the Internet has not increased the amount of mathematical crank mail. Most of the letters still come typewritten, often on what appear to be manual typewriters. “Cranks are always about one level of technology behind,” Dr. Dudley said. Dr. Casti said he imagined some of his correspondents as “penniless guys in cold-water flats.” They save paper and postage by single-spacing and often type on both sides of the page.
Sometimes, just sometimes, a mathematician finds that it pays to answer an unsolicited letter, one that does not have what Dr. Stewart calls “the strange fairy dusting of lunacy.” He once received a clearly written letter from a man in China who believed he had trisected the angle. “I knew it had to be a fallacy, but it gave me a spur to try and see where it was wrong,” Dr. Stewart said. “There were three points in the diagram that looked as if they were on a straight line, but actually were not.” Dr. Stewart wrote back and received a reply thanking him for pointing out the error. “It is the only time I’ve had any success convincing someone like this that they were wrong,” he said.
Several years ago Dr. Stewart heard from a man—in India again—who had found a new, simpler proof for an obscure, pointless theorem in number theory written by Ramanujan and a collaborator. According to the Ramanujan-Nagell theorem, the only numbers one can square and add 7 to, yielding an answer that is a power of 2, are 1, 3, 5, 11 and 181. For example, squaring 3 and adding 7 gives 16, which is the fourth power (the square of the square) of 2.
Dr. Stewart was surprised to realize that the proof was correct, but it was badly typed on strange paper and cast in an idiosyncratic style that would have given any journal editor the impression that the writer was a crank. Dr. Stewart advised the writer to find an Indian number theorist who could teach him how to present a proper paper. Several years later the result was published, and soon after came another publication from the same man. “It is worth reading these things occasionally,” Dr. Stewart said.
But only occasionally, Dr. Dudley advised. “There is always that chance of success, but it is so small,” he said. “I’ve gone through enough reams of crank stuff to know that the probability is close to zero.”
February 9, 1999