Click here to download a PDF of BC Calculus Practice Test 2.
Section I of this examination contains 45 multiple-choice questions. Fill in only the ovals for numbers 1 through 45 on your answer sheet.
CALCULATORS MAY NOT BE USED IN THIS PART OF THE EXAMINATION.
Indicate all of your answers to the multiple-choice questions on the answer sheet. No credit will be given for anything written in this exam booklet, but you may use the booklet for notes or scratch work. After you have decided which of the suggested answers is best, completely fill in the corresponding oval on the answer sheet. Give only one answer to each question. If you change an answer, be sure that the previous mark is erased completely. Here is a sample question and answer.
Sample Question
Chicago is a
(A) state
(B) city
(C) country
(D) continent
(E) village
Sample Answer
Use your time effectively, working as quickly as you can without losing accuracy. Do not spend too much time on any one question. Go on to other questions and come back to the ones you have not answered if you have time. It is not expected that everyone will know the answers to all the multiple-choice questions.
Many candidates wonder whether or not to guess the answers to questions about which they are not certain. Multiple choice scores are based on the number of questions answered correctly. Points are not deducted for incorrect answers, and no points are awarded for unanswered questions. Because points are not deducted for incorrect answers, you are encouraged to answer all multiple-choice questions. On any questions you do not know the answer to, you should eliminate as many choices as you can, and then select the best answer among the remaining choices.
CALCULUS BC
SECTION I, Part A
Time—55 Minutes
Number of questions—28
A CALCULATOR MAY NOT BE USED ON THIS PART OF THE EXAMINATION
Directions: Solve each of the following problems, using the available space for scratchwork. After examining the form of the choices, decide which is the best of the choices given and fill in the corresponding oval on the answer sheet. No credit will be given for anything written in the test book. Do not spend too much time on any one problem.
In this test: Unless otherwise specified, the domain of a function f is assumed to be the set of all real numbers x for which f(x) is a real number.
1. What is the slope of the line tangent to the curve x2 + 2xy + 3y2 = 2 when y = 1?
| (A) | – |
| (B) | – |
| (C) | –1 |
| (D) | 0 |
| (E) | |
| (A) | –e |
| (B) |  |
| (C) | 0 |
| (D) |  |
| (E) | e |
3. If, for t > 0, x = t2 and y = cos(t2), then 
=
| (A) | cos(t2) |
| (B) | –sin(t2) |
| (C) | –sin(2t) |
| (D) | sin(t2) |
| (E) | cos(2t) |
4. The function f(x) = 4x3 – 8x2 + 1 on the interval [–1, 1] has an absolute minimum at x =
| (A) | –11 |
| (B) | –1 |
| (C) | 0 |
| (D) | 1 |
| (E) |  |
| (A) |  |
| (B) |  |
| (C) |  |
| (D) |  |
| (E) |  |
| (A) | 2x sin 2x |
| (B) | 2x cos2 x |
| (C) | x sin 2x |
| (D) | 2x sin2 x + x2 cos2 x |
| (E) | 2x sin2 x + x2 sin 2x |
7. The line normal to the curve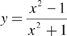 
at x = 2 has slope
| (A) |  |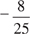
| (B) |  |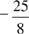
| (C) | 1 |
| (D) |  |
| (E) | –1 |
8. If f and g are differentiable functions and h(x) = f(x)eg(x), then h′(x) =
| (A) | f′(x)eg′(x) |
| (B) | f′(x)eg(x) + f(x)eg′(x) |
| (C) | eg(x)[f′(x) + f(x)g′(x)] |
| (D) | eg(x)[f′(x) + 1] |
| (E) | eg′(x)[f′(x) + g′(x)] |
The graph of y = f(x) is shown above. Which of the following could be the graph of y = f′(x) ?
| (A) |  |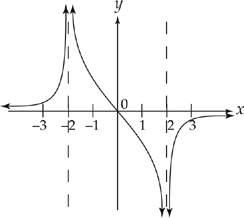
| (B) |  |
| (C) |  |
| (D) |  |
| (E) |  |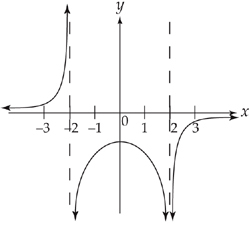
| (A) |  |
| (B) |  |
| (C) | e4 + 2e2 + e |
| (D) |  |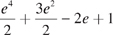
| (E) |  |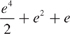
| (A) |  |
| (B) |  |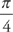
| (C) |  |
| (D) | π |
| (E) | Divergent |
12. What is the equation of the line tangent to the graph of y = sin2 x at ?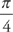
| (A) |  |
| (B) |  |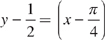
| (C) |  |
| (D) |  |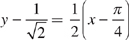
| (E) |  |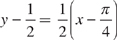
13. If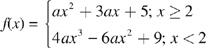 
, find the value of a that makes f(x) continuous for all real values of x.
| (A) | –1 |
| (B) | 0 |
| (C) | 1 |
| (D) | 2 |
| (E) | 4 |
| (A) |  |
| (B) |  |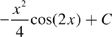
| (C) |  |
| (D) |  |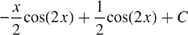
| (E) |  |
15. If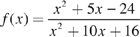 
, then 
is
| (A) | 0 |
| (B) | 1 |
| (C) |  |
| (D) |  |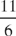
| (E) | Nonexistent |
16. What is the approximation of the value of e3 obtained by using the fourth-degree Taylor polynomial about x = 0 for ex?
| (A) |  |
| (B) |  |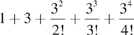
| (C) |  |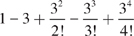
| (D) |  |
| (E) |  |
17. A rock is thrown straight upward with an initial velocity of 50 m/s from a point 100 m above the ground. If the acceleration of the rock at any time t is a = –10 m/s2, what is the maximum height of the rock (in meters)?
| (A) | 125 |
| (B) | 150 |
| (C) | 175 |
| (D) | 200 |
| (E) | 225 |
18. The sum of the infinite geometric series 
is
| (A) | –6 |
| (B) | –3 |
| (C) | 0 |
| (D) |  |
| (E) |  |
19. What are all values of x for which the series 
converges?
| (A) | –2 ≤ x ≤ 8 |
| (B) | –2 < x ≤ 8 |
| (C) | –2 ≤ x < 8 |
| (D) | –5 ≤ x ≤ 5 |
| (E) | –5 ≤ x < 5 |
20. Find the area inside one loop of the curve r = sin 2θ.
| (A) | |
| (B) |  |
| (C) | |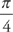
| (D) | |
| (E) | π |
21. The average value of sec2 x on the interval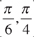 
is
| (A) |  |
| (B) |  |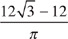
| (C) |  |
| (D) |  |
| (E) |  |
22. Find the length of the arc of the curve defined by x = t2 and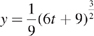 
from t = 0 to t = 2.
| (A) | 8 |
| (B) | 10 |
| (C) | 12 |
| (D) | 14 |
| (E) | 16 |
23. The function f is given by f(x) = x4 + 4x3. On which of the following intervals is f decreasing?
| (A) | (–3, 0) |
| (B) | (0, ∞) |
| (C) | (–3, ∞) |
| (D) | (–∞, –3) |
| (E) | (–∞, 0) |
24. Which of the following series converge(s)?
| I. |  |
| II. |  |
| III. |  |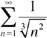
| (A) | I only |
| (B) | II only |
| (C) | I and II |
| (D) | I and III |
| (E) | I, II, and III |
25. Given the differential equation 
, where z(0) = 50, what is 
?
| (A) | 400 |
| (B) | 200 |
| (C) | 100 |
| (D) | 50 |
| (E) | 4 |
The slope field shown above corresponds to which of the following differential equations?
| (A) |  |
| (B) |  |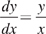
| (C) |  |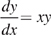
| (D) |  |
| (E) |  |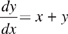
27. The value of c that satisfies the mean value theorem for derivatives on the interval [0, 5] for the function f(x) = x3 – 6x is
| (A) |  |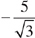
| (B) | 0 |
| (C) | 1 |
| (D) |  |
| (E) |  |
The graph of f is shown in the figure above. If 
, for what positive value of x does g(x) have a minimum?
| (A) | 0 |
| (B) | 1 |
| (C) | 2 |
| (D) | 3 |
| (E) | 4 |
END OF PART A, SECTION I
IF YOU FINISH BEFORE TIME IS CALLED, YOU MAY CHECK YOUR WORK ON PART A ONLY.
DO NOT GO ON TO PART B UNTIL YOU ARE TOLD TO DO SO.
SECTION I, Part B
Time—50 Minutes
Number of questions—17
A GRAPHING CALCULATOR IS REQUIRED FOR SOME QUESTIONS ON THIS PART OF THE EXAMINATION
Directions: Solve each of the following problems, using the available space for scratchwork. After examining the form of the choices, decide which is the best of the choices given and fill in the corresponding oval on the answer sheet. No credit will be given for anything written in the test book. Do not spend too much time on any one problem.
In this test:
(1) The exact numerical value of the correct answer does not always appear among the choices given. When this happens, select from among the choices the number that best approximates the exact numerical value.
(2) Unless otherwise specified, the domain of a function f is assumed to be the set of all real numbers x for which f(x) is a real number.
29. If f(x) is the function given by f(x) = e3x + 1, at what value of x is the slope of the tangent line to f(x) equal to 2?
| (A) | –.135 |
| (B) | 0 |
| (C) | .231 |
| (D) | –.366 |
| (E) | .693 |
30. If y = (sin x)ex, then, when defined, y′ =
| (A) | (sin x)ex (cos x) |
| (B) | (cos x)ex |
| (C) | ex(cot x + ln(sin x)) |
| (D) | ex(sin x)ex (cot x + ln(sin x)) |
| (E) | ex(sin x)ex (cot x) |
31. The side of a square is increasing at a constant rate of 0.4 cm/sec. In terms of the perimeter, P, what is the rate of change of the area of the square, in cm2/sec?
| (A) | 0.05P |
| (B) | 0.2P |
| (C) | 0.4P |
| (D) | 6.4P |
| (E) | 51.2P |
32. If f is a vector-valued function defined by f(t) = (sin 2t, sin2 t), then f″(t) =
| (A) | (–4 sin 2t, 2 cos 2t) |
| (B) | (–sin 2t, –cos2 t) |
| (C) | (4 sin 2t, cos2 t) |
| (D) | (4 sin 2t, –2 cos 2t) |
| (E) | (2 cos 2t, –4 sin 2t) |
33. The height of a mass hanging from a spring at time t seconds, where t > 0, is given by h(t) = 12 – 4 cos(2t). In the first two seconds, how many times is the velocity of the mass equal to 0?
| (A) | 0 |
| (B) | 1 |
| (C) | 2 |
| (D) | 3 |
| (E) | 4 |
| (A) | 2 |
| (B) |  |
| (C) |  |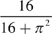
| (D) | |
| (E) | Nonexistent |
35. What is the trapezoidal approximation of 
using n = 4 subintervals?
| (A) | 6.407 |
| (B) | 13.565 |
| (C) | 19.972 |
| (D) | 27.879 |
| (E) | 34.944 |
36. Given x2y + x2 = y2 + 1, find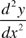 
at (1,1).
| (A) | 36 |
| (B) | 12 |
| (C) | –4 |
| (D) | –12 |
| (E) | –36 |
37. If 
and 
, then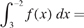 
| (A) | a + b |
| (B) | a – 2b |
| (C) | a – b |
| (D) | b – a |
| (E) | 2b – a |
| (A) | 5 cos 5x – 2 cos 2x |
| (B) | 5 sin 5x – 2 sin 2x |
| (C) | cos 5x – cos 2x |
| (D) | sin 5x – sin 2x |
| (E) |  |
39. Using the Taylor series about x = 0 for sin x, approximate sin(0.4) to four decimal places.
| (A) |  |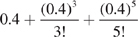
| (B) |  |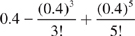
| (C) |  |
| (D) |  |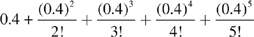
| (E) |  |
40. Let R be the region in the first quadrant between the graphs of y = e–x, y = sin x, and the y-axis. The volume of the solid that results when R is revolved about the x-axis is
| (A) | –0.888 |
| (B) | –0.869 |
| (C) | 0.277 |
| (D) | 0.869 |
| (E) | 0.888 |
41. Use Euler’s Method, with h = 0.2 to estimate y(3), if = 2y – 4x and y(2) = 6.
| (A) | 9.684 |
| (B) | 10.442 |
| (C) | 12.378 |
| (D) | 12.756 |
| (E) | 18.426 |
| (A) | tan4 x + C |
| (B) |  |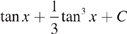
| (C) | tan2 x + C |
| (D) |  |
| (E) | sec2 x tan2 x + C |
43. Let 
; 0 < x < π. If 
, then f(1) =
| (A) | –1.861 |
| (B) | –0.480 |
| (C) | 0.134 |
| (D) | 0.524 |
| (E) | 1.521 |
| (A) |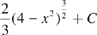 
|
| (B) |  |
| (C) |  |
| (D) |  |
| (E) |  |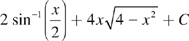
45. A force of 250 N is required to stretch a spring 5 m from rest. Using Hooke’s law, F = kx, how much work, in Joules, is required to stretch the spring 7 m from rest?
| (A) | 14.286 |
| (B) | 71.429 |
| (C) | 245 |
| (D) | 490 |
| (E) | 1225 |
STOP
END OF PART B, SECTION I
IF YOU FINISH BEFORE TIME IS CALLED, YOU MAY CHECK YOUR WORK ON PART B ONLY.
DO NOT GO ON TO SECTION II UNTIL YOU ARE TOLD TO DO SO.
SECTION II
GENERAL INSTRUCTIONS
You may wish to look over the problems before starting to work on them, since it is not expected that everyone will be able to complete all parts of all problems. All problems are given equal weight, but the parts of a particular problem are not necessarily given equal weight.
A GRAPHING CALCULATOR IS REQUIRED FOR SOME PROBLEMS OR PARTS OF PROBLEMS ON THIS SECTION OF THE EXAMINATION.
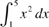may not be written as fnInt (X2, X, 1, 5).SECTION II, PART A
Time—30 minutes
Number of problems—2
A graphing calculator is required for some problems or parts of problems.
During the timed portion for Part A, you may work only on the problems in Part A.
On Part A, you are permitted to use your calculator to solve an equation, find the derivative of a function at a point, or calculate the value of a definite integral. However, you must clearly indicate the setup of your problem, namely the equation, function, or integral you are using. If you use other built-in features or programs, you must show the mathematical steps necessary to produce your results.
1. An object moving along a curve in the xy-plane has its position given by (x(t), y(t)) at time t seconds, 0 ≤ t ≤ 1, with 
= 8t cos t units/sec and 
= 8t sin t units/sec.
At time t = 0, the object is located at (5, 11).
| (a) | Find the speed of the object at time t = 1. |
| (b) | Find the length of the arc described by the curve’s position from time t = 0 to time t = 1. |
| (c) | Find the location of the object at time t = . |
A baseball diamond is a square with each side 90 feet in length. A player runs from second base to third base at a rate of 18 ft/sec.
| (a) | At what rate is the player’s distance from first base, A, changing when his distance from third base, D, is 22.5 feet? |
| (b) | At what rate is angle a increasing when D is 22.5 feet? |
| (c) | At what rate is the area of the trapezoidal region, formed by line segments A, B, C, and D, changing when D is 22.5 feet? |
SECTION II, PART B
Time—1 hour
Number of problems—4
No calculator is allowed for these problems.
During the timed portion for Part B, you may continue to work on the problems in Part A without the use of any calculator.
3. Consider the equation x2 – 2xy + 4y2 = 64.
| (a) | Write an expression for the slope of the curve at any point (x,y). |
| (b) | Find the equation of the tangent lines to the curve at the point x = 2. |
| (c) | Find  at (0,4). |
Three trains, A, B, and C each travel on a straight track for 0 ≤ t ≤ 16 hours. The graphs above, which consist of line segments, show the velocities, in kilometers per hour, of trains A and B. The velocity of C is given by v(t) = 8t – 0.25t2.
(Indicate units of measure for all answers.)
| (a) | Find the velocities of A and C at time t = 6 hours. |
| (b) | Find the accelerations of B and C at time t = 6 hours. |
| (c) | Find the positive difference between the total distance that A traveled and the total distance that B traveled in 16 hours. |
| (d) | Find the total distance that C traveled in 16 hours. |
5. Let y be the function satisfying f′(x) = x(1 – f(x)); f(0) = 10.
| (a) | Use Euler’s Method, starting at x = 0, with step size of 0.5 to approximate f(x) at x = 1. |
| (b) | Find an exact solution for f(x) when x = 1. |
| (c) | Evaluate  |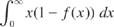
6. Given f(x) = tan–1(x) and 
, for |x| ≤ 1.
| (a) | Find the fifth-degree Taylor polynomial and general expression for g(x) about x = 0. |
| (b) | Given that 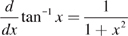 , for |x| ≤ 1, use the result of part (a) to find the fifth-degree Taylor polynomial and general expression for f(x) about x = 0. |
| (c) | Use the fifth-degree Taylor polynomial to estimate  |
STOP
END OF EXAM
