Keimpe Algra

Aristotle’s Conception of Place and its Reception in the Hellenistic Period

1 Introduction

At first sight the discussion of place in Aristotle Phys. 4, 1–5 may seem patchy and its style at times crabbed. On closer view, however, its contents acquire coherence through the conscious and explicit application of what has been labelled Aristotle’s ‘dialectical method’. It is no coincidence that the discussion of place has been one of the key examples in G.E.L. Owen’s classic study of this method,4 for Aristotle is more explicit than he usually is in outlining his procedure:

We must try to make our inquiry in such a way that the ‘what-it-is’ is provided, the aporiai are solved, the apparent facts about place are accounted for, and, finally, so that the reason for the difficulty and for the problems around it are clear (Arist. Phys. 4, 211a7–11).

Aristotle practices what he preaches: he provides the ‘what it is’ in the form of a definition or account (“the first immobile boundary of what contains”, Phys. 4, 212a20); he solves the aporiai (at least for this, his own, conception of place, Phys. 4, 212b22–29); he accounts for the apparent facts (at least for those apparent properties that genuinely apply to place, i. e. the set of properties specified at 210b33 ff.); and he provides us with an explanation of the difficulty of the subject, in the following passage:

Place seems to be something profound and difficult to grasp, both because the notions of matter and form present themselves together with it (παρεμφαίνεσθαι), and because of the fact that change of position of a moving body occurs within a surrounding body which is at rest; for [from this] it appears to be possible that there is an extension in between which is something other than the magnitudes which move. Air, too, contributes to this suggestion, by appearing to be incorporeal; place seems to be not only the limits of the vessel, but also that which is in between, which is considered as being void (Arist. Phys. 4, 212a7–30).

The problem seems to be, in other words, that the phenomena are unclear to the extent that in everyday thinking and speaking various conceptions of place – including the most important rival conception of place as three-dimensional extension (“that which is in between, which is considered as being void”) – readily come to mind and may be used promiscuously. In fact, and as we shall see, the corpus Aristotelicum itself does not always stick to what in Physics 4 comes out as the correct account. Nevertheless it is here, in Physics 4, that the various conceptions of place are disentangled and examined, and that we are told which one can be coherently maintained.

This is not to say that everything is clear and convincing, and it should come as no surprise that the conception with which Aristotle eventually comes up, place as the first immobile surface of what surrounds (τὸ τοῦ περιέχοντος πέρας 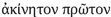, 212a20), had a mixed reception in later ancient and medieval thought. It was dutifully reported and defended in such Aristotelian scholastic texts as the commentaries of Alexander of Aphrodisias (of which only fragments remain) and Themistius, and even in the exegetical part of the sixth century commentary of Philoponus.5 Yet, in a separate excursus, nowadays known as the Corollary on Place, this same Philoponus launched some devastating objections against the Aristotelian conception of place. The formidable Simplicius was no less critical, although he came up with a different alternative theory. Some points made in these later ancient texts resurfaced in the stock quaestiones of the medieval commentary tradition.6

The subject of the present paper is the equally mixed reception of Aristotle’s accounts of place and void in the Hellenistic period. Engagement with Aristotle’s theory in this period appears to have come in at least two stages. The first concerns the interpretation of the theory of Phys. 4, 1– 5 by Aristotle’s earliest successors. I will discuss these early reactions, mostly on the basis of the evidence provided by Simplicius (in particular in the Corollary on Place which rounds off his commentary on Phys. 4, 1–5), in sections 3 (Eudemus), 4 (Theophrastus) and 5 (Strato) of this paper. The second stage appears to have started in the first century BC – after the resurfacing of the corpus Aristotelicum – and to have taken the form of a debate between Peripatetics and Stoics on place and on the Stoic conception of an extra-cosmic void. Here again it is Simplicius who offers part of the evidence – on the Peripatetic Xenarchus of Seleucia and on anti-Stoic arguments assembled by Alexander of Aphrodisias – whereas other relevant information is provided by Cleomedes in his first or second century AD handbook of Stoic cosmology. This debate will be the subject of my section 6. Finally, the sceptical accounts of place in Sextus Empiricus’ work may complement our picture of the late Hellenistic situation, in so far as they as well basically oppose the Stoic and the Aristotelian positions. They will be discussed in section 7.

I will start out, however, with a systematic discussion (section 2) of four rather problematic aspects of Aristotle’s account, each of which seems to have left its traces not only among the commentaries of late antiquity, but also in the discussions within the Hellenistic Peripatos (section 2). This will force me, in passing, to come to terms with Ben Morison’s challenging ‘revisionist’ interpretations of Aristotle’s theory of place (his book On Location now being the most extensive in-depth study of Phys. 4, 1–5). According to Morison people have been too quick to criticize the theory, which he thinks was actually quite good.⁷ In a separate article he also discusses the evidence on Theophrastus, claiming, contrary to what has thus far been the mainstream interpretation, that Theophrastus defended Aristotle’s conception of place unrestrictedly.⁸ Although my conclusion will be that this interpretation, though sympathetically charitable, gets insufficient support from Aristotle’s text and from the evidence on the early Peripatetic reactions to it, I am convinced that the challenge of engaging with Morison’s arguments will help us to sharpen our view both of the problems and arguments involved and of the nature and limitations of the evidence.

2 Problems in Aristotle’s account

Aristotle’s conception of place is rooted in some sensible ways of using the conception of place in ordinary speaking and thinking. When we say that a fish is swimming ‘in the water’ or that I am ‘in Athens’, we are speaking of a thing’s surroundings as its place. However, in so far as Aristotle’s account makes a point of transforming such general ways of speaking into a more technical philosophical conception by specifying a thing’s place in the proper sense as “the first immobile surface of the surrounding body”, it can be seen to run into difficulties on at least two accounts. To begin with, it is not clear why this particular conception should be favoured over other conceptions as the only correct one, more particularly why the most commonly accepted rival conception – place as an independent three-dimensional extension – should be discarded. Secondly, specifying place as a first surrounding surface brings in problems of its own. For one thing, such a surrounding surface disappears once the emplaced body has been removed (so that it is not stable in the required sense of something that can be left behind and re-filled); for another, such a surface is the surface of a mobile substance (so it is not clear how it can be immobile, as it should be, also according to Aristotle); and finally, some bodies, such as the outer sphere of the heavens, do not have a surrounding surface (so that not every physical substance is in a place, as should be the case). Let us examine each of these objections – numbered as (i)-(iv) – in succession.

 

(i) Aristotle’s arguments against the conception of place as a three-dimensional extension are problematic. The arguments used by Aristotle to eliminate the most important rival conception (place as an underlying three-dimensional extension) are unsatisfactory.9 The text at issue is very unclear; and the arguments it contains do not appear to hit the mark. The two main arguments used in the fourth chapter of Phys. 4 (211b19–29) seem to be these:

• (1) On this conception of place, there would be an infinity of places in the same spot ( 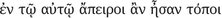, 211b20–21) for in a continuous emplaced body we can distinguish an infinity of parts which will all have their own places, so that we have an infinity of juxtaposed (and, we may presume, in fact also overlapping) three-dimensional places “in the same spot”; and
• (2) On this conception of place, place will be moving (ἅμα δὲ καὶ ὁ τóπος 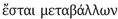, 211b23).

Elsewhere in the same book, in the course of his discussion of void in chapter 8, Aristotle uses a different route to reach the absurd conclusion of an infinity (or at least: an indefinite number) of places “in the same spot”. This time he seems to be thinking of a doubling of three-dimensional extensions which can go on ad infinitum:

• (3) “What will be the difference between the body of the cube and the void and place which are equal to it? And if two things can behave like this, why cannot any number of things coincide?” (216b9–11).

It is not too difficult to see, however, that (2) and (3) are not straightforwardly convincing in that they simply presuppose Aristotle’s apparent conviction that there is only one kind of three-dimensional extension, viz. the extension of substances themselves. Elsewhere Aristotle argues that it is the phenomenon of things moving through air that has caused the mistaken supposition that things can move through mere extension, for air seems to be incorporeal. But, or so he claims, there is no extension apart from the extension of substances: “what is in between a place is whatever body it may be, but not the extension of a body” (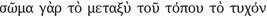 , , 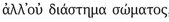Phys. 4, 212b26 – 27).

This brings us to objection (1). It is defended by Ben Morison:

Aristotle was right to argue that on the diastēma theory of place a particular mass of water, for example, will have an infinity of internal places ‘hanging around’, whereas in truth the parts of the water are not the right sort of item to have a place.10

There are two claims involved here. The last claim, about parts of a continuous substance not being the sort of things to be emplaced, may be true on Aristotle’s own principles. And indeed, even Philoponus – as we know, an adherent of the diastēma view – subscribes to the Aristotelian idea that only substances have places.¹¹ But this very example shows that the claim that randomly defined parts of continuous substances all have places of their own is in itself not a necessary concomitant of the conception of place as an independent three-dimensional extension. Moreover, even if we assume, with Aristotle, that it is, there is still no compelling reason to find it intrinsically problematic. On the contrary, one might argue that it is rather the Aristotelian view, with its denial that the parts of continuous substances have places of their own, that goes against common intuitions on the way the concepts of place and position are to be used.

Morison’s first claim, that Aristotle is right about the infinity of internal places ‘hanging around’, is more puzzling. For it is simply not true that the conception of place as a three-dimensional extension involves an actual infinity of overlapping or nested places. Even from an Aristotelian point of view the diastēma view would involve – if we take it to involve a view about the emplacement of parts at all – that the (only potentially infinite number of) parts of a continuous substance, however specified, would occupy (a potential infinity of) correspondingly specified parts of one and the same absolute extension, not that an actual infinity of places co-exist or ‘hang around’.

What are the reasons for Aristotle’s odd claims, and what is the reason why they were accepted by at least part of the subsequent Aristotelian tradition? We may note that the rival conception of place as a self-subsistent three-dimensional extension could not be easily integrated within an Aristotelian ontology: being self-subsistent, such a place or space could not be considered as an accident, i. e. a quantity; but neither could it be seen as a substance in the sense of a synolon of form and matter. Philoponus acknowledges this in his Corollary on Place but argues that we should then conclude that, in the face of the strong arguments in favour of the existence of space as a three-dimensional extension, there is something wrong with the ontological premise that a quantity cannot subsist by itself (Ph. 578, 5–579, 17). Note, incidentally, that in Phys. 4 Aristotle does not explicitly make the point about the ontological inconceivability of place as a self-subsistent extension, although the point may be implied in the aporia in chapter 1 which claims that it is unclear what genus we should ascribe to place: it has three dimensions but is not a body (209a4–6).

At the beginning of the sixth century it was the weakness of Aristotle’s arguments against the rival conception of place as an independent extension that triggered the excursus on place in Philoponus’ commentary which is nowadays known as the Corollary on Place. As we shall see (sections 5 and 6 below, pp. 38–47), he was anticipated in this respect by the Hellenistic Peripatetics Strato of Lampsacus and Xenarchus of Seleucia. For many Aristotelians, however, the view that place was a separate extension apparently could not be true and that may have helped them to swallow Aristotle’s rather limp refutation of this view in Physics 4. Thus, we have no evidence that Eudemus or Theophrastus did not follow Aristotle on this point of his account. And as we shall see (section 7 below, pp. 47– 51), Sextus Empiricus’ (partly parallel) accounts of place in PH 3, 119–135 and M 10, 1–36 show us that also the late Hellenistic arsenal of sceptical arguments from which Sextus could draw still used versions of Aristotle’s arguments against the conception of place as a three-dimensional extension, although now directed against the Stoics.

 

(ii) Place and the explanation of motion. A second problem emerges once we realize that Aristotle’s theory of place appears to be primarily a theory of the location of static bodies. Or perhaps we should say that it appears to work only in cases where we actually do find a more or less stable surrounding surface, whereas it is not easy to use his conception of place to describe the trajectory of bodies in motion. Nevertheless the explanation of motion, or change in general, is explicitly adduced as the raison d’être for introducing the subject within the context of the Physics in the first place.12 Why would the theory count as defective in this respect? But also: why would it be that many Aristotelians did not see this? Let us have a look at the difficulties first. Using Aristotle’s conception of place, we should describe a body in motion as traversing an infinity of instantaneous two-dimensional places. In his Corollary on Place Philoponus takes Aristotle to task for the element of two-dimensionality:

If place is the boundary of the container and is not some different extension between the boundaries over and above the bodies that come to be in it, then clearly during my motion from Athens to Thebes the parts of air that yield up their own place to me (for motion is a change of places and a continuous exchange) yield up nothing but surfaces. But when surfaces alone are put together, even an infinite number of them, coinciding with each other they make the whole no bigger. So how can the moving body move forwards? (Phlp. Ph. 567, 12–18).13

It is perhaps no coincidence that in contexts like these, where we are describing the trajectory of a moving body, Aristotle sometimes consciously or unconsciously resorted to the very concept of place as a three-dimensional extension which he had rejected for theoretical reasons.¹⁴

[…] the celestial element is eternal and the spatial path (topos) through which it moves is endless, though always complete, while the terrestrial bodies each have their distinct and limited regions (topous) (Arist. Meteor. 339a25ff.).

Richard Sorabji focuses on another problem by pointing out that the surrounding surfaces in such a process are instantaneous.¹⁵ Hence, a boat moving through water should be taken to traverse a series of instantaneous limits, so that it could never return to a place, for once a place is left it no longer exists. This may not count as an odd result, if we recall the explicit claim that “place is together with the object, for the limits are together with what is limited” (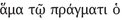. 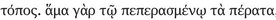, Phys. 4, 212a29–30). But it does appear to be an odd result, if we take account of a different requirement also introduced by Aristotle, namely that place should be something that can be left behind: “the place where the thing is can be left by it, and is therefore separable from it” (Phys. 4, 211a3).¹⁶ So the problem is simply this: in what sense does Aristotle’s theory allow me speak of the place I occupied this morning while standing in the garden, or of the place where I will be tonight while having dinner, if the relevant surrounding surfaces no longer exist or not yet?

It may be worth our while to have a look at the way in which Ben Morison’s charitable interpretation of Aristotle deals with this problem. The central point of his interpretation is that, according to Aristotle, place as the surface or limit “of what surrounds” can in practice be specified in various ways and that in the end “Aristotle identifies the proper place of x at time t as the inner limit of the universe at which it is in contact with x at t” (my italics).¹⁷ In other words, “the surroundings (to periechon) to which a body must be related in order to say where it is are the whole universe” (again, italics mine).¹⁸ This allows him to dodge the objection about instantaneous places by claiming that place as a limit is something which the universe can assume and re-assume: “shapes, sizes, limits, colours etc. are assumed – sometimes instantaneously – and they can be re-assumed. Our interpretation is safe from this objection”.¹⁹ Perhaps this does offer a way to make sense of the notion of (returning to) the ‘same’ place. However, the point remains that also on this interpretation places are hardly things that can be identified once they have been left, or before they are reached.

As we will see, the evidence suggests that Eudemus of Rhodes was sensitive to this problem. But apparently for him, as well as for the other ‘mainstream’ followers of Aristotle, this problem did not count as fatal. A possible reason for this may have been that being able to indicate the location of static substances was precisely what most Aristotelians expected from the theory of place, even within the context of a theory of locomotion. After all, Aristotle and Aristotelians were used to analysing changes, including locomotion, first and foremost in terms of their starting point and end point. See, for example, the analysis of change in Phys. 1 (esp. chapter 1, 5) as a process occurring between opposites. Within such a general descriptive framework Aristotle’s conception of place sufficed to describe the situation at the outset as well as the situation at the end of a process of locomotion. So even those, like Eudemus, who noted that the account of place as a two-dimensional surrounding surface involved some counter-intuitive aspects, may have found it workable for all practical purposes in the context of Aristotelian physics.

 

(iii) The required immobility of place. Even if, for the reasons indicated, these first two general objections may have carried little weight within the context of Aristotelian physics, there were some other problems which remained pressing also within an Aristotelian context and which accordingly left their traces more widely in the commentary tradition. First, there is the problem of saving the required immobility of place. At some point in the middle of his account in chapter 4 of Phys. 4 Aristotle adds the requirement that place should be immobile (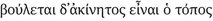 , 212a18), so he qualifies his definition of place accordingly: it is not just the surface of the surrounding body, but the immobile surface of the surrounding body. In the same context he adds that a thing located in a mobile place is in a vessel rather than in a place. So a vessel is a mobile place and a place is an immobile vessel. But how can this distinction be applied in practice? After all, in most circumstances a thing’s surroundings consist of mobile substances. Even in the case of the layers of the elements we see that water and air are mobile and in fact moving, and the same goes for fire, and for the aether of the heavenly bodies. So, where are immobile places to be found?20

Aristotle adds to the difficulty by providing a rather obscure example: a boat in a river (presumably what he has in mind is a boat flowing along with the river). He claims that in such a case the boat is in the flowing water as in a vessel (with respect to which it does not move), whereas its immobile place is “the whole river” (with respect to which it does move):

Just as a vessel is a mobile place, so place is an immobile vessel. That is why, when something is in motion inside a moving object (imagine a boat on a river), it uses its surroundings as a vessel rather than as a place. But place is meant to be immobile. For that reason the whole river is rather the place (), because taken as a whole it is immobile (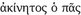) (Arist. Phys. 4, 212a14–20).

This passage was much debated by ancient and medieval commentators and various solutions were devised.²¹ Some commentators took the claim about “the whole river” being the place to refer to the immobile river banks (as opposed to the mobile, flowing water). But that would be to violate one of the criteria for place which Aristotle had set up himself, viz. that it should be contiguous (prōton peras) and of the same size (“neither larger nor smaller”, 211a2). In order to save both the contiguity and the immobility of Aristotelian place (qua surface of the surrounding body) some later medieval commentators introduced a distinction between material place (the actual surface of the immediately surrounding body, which may be mobile) and formal place (the surrounding surface, considered in abstracto, and with its immobility defined in terms of its location in relation to the outer sphere of the heavens).

Ben Morison presents what at first sight may seem to be a variant of this theory.²² His interpretation of Aristotle’s definition resembles the medieval conception of formal place in that it rescues the immobility of the surrounding surface by specifying it in a particular way. The difference is that whereas the medieval commentators specified the relevant surface as the surface of the immediately containing substance, but taken in abstracto, Morison specifies it as the containing surface taken as the surface of a larger surrounding entity, or of a group of entities, and in the end even as the surface of the surrounding cosmos as a whole. Thus, in the example of the boat being moored in, or moving through, a flowing river, the boat’s (immobile) place, i.e. the surrounding surface, should not be specified as the surface of the surrounding (flowing) water, but as the surface of the (immobile) river as a whole, and in the end even as the inner surface of the whole universe surrounding the boat. And the universe as a whole is immobile – as Morison points out – not in the sense that it is naturally designed for motion but will in fact not move, but in the sense that, although nothing prevents it from moving, it is impossible for it to move, because there is nothing outside it.²³ This, in the end, is what guarantees the required immobility of the surrounding surface. So the problem of the immobility of place disappears: we can always specify the surrounding surface as the surface of the immobile surrounding cosmos as a whole.

This is an ingenious solution, which at first sight seems to have in its favour that it makes use of the familiar Aristotelian practice of specification by means of qua-locutions. But there is a drawback: we do not easily derive it from what Aristotle actually says. He does not use the relevant qua locutions in this context. He does not speak of the surface of the surrounding river qua surface of the surrounding universe. Instead, he simply uses the crude formula “the whole river is rather the place”. At this point Morison, appears to support his interpretation by offering a different translation of the words 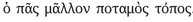. He takes them to mean: “rather the whole river is a place”, i. e. one of the possible ways of identifying the surrounding surface, next, for example to the identification of this surface as the limit of the surrounding universe.²⁴ On this reading, in other words, the eventual identification of the surrounding surface as the surface of the surrounding immobile universe is thus at least implied.

Apart from the fact that this does not appear to be the most natural way to interpret the Greek of this particular passage,²⁵ the role here accorded to the immobility of the cosmos as a whole seems questionable for other reasons as well. First of all, it is true that Aristotle does speak of the universe as a koinos topos, so in a sense each thing can be said to be ‘in the universe’ as in a place. But in so far as I can see, he does not anywhere connect this device to the issue of the immobility of a thing’s proper place (idios topos).²⁶ Secondly, it may well be asked whether the immobility of the cosmos as a whole is the kind of immobility we are looking for. We are discussing intra-cosmic motion and rest, so we need an immobile reference point within the cosmos which allows us to determine whether a particular body is moving or at rest. This is what Aristotle makes clear in the passage immediately following on the river example and the statement of the immobility requirement.²⁷ For there he goes on to talk about the centre of the world and the inner limit of the sphere of the heavens as ‘above’ and ‘below’ in the basic, or ‘absolute’ sense, because they are both at rest. It is with respect to these two items that we can determine the natural rest or natural motion of the elements. As we will see, Eudemus explicitly works out this line of thought by specifying that we define immobile places with reference to the heavenly sphere which is immobile in the relevant, intra-cosmic, sense. For all we know, he did not speak of the immobility of the cosmos as a whole.

And indeed we may well ask how relevant the immobility of the cosmos as a whole would be in this connection. Imagine a situation where the cosmos is surrounded by an infinite empty space and where – as imagined by the Stoic Cleomedes and in medieval thought experiments – it moves or is moved so that it exhibits a rectilinear translation through this space.28 Would that change the way in which we define mobile versus immobile substances within the cosmos? Wouldn’t we still regard the centre and the periphery as fixed reference points for determining intra-cosmic motion and rest? Conversely, of what use would the immobility of the cosmos as a whole be, for the purpose of locating things within the cosmos, if we imagine the cosmos as containing no fixed elements, but consisting of substances which all move helter-skelter all the time? It appears, in other words, that the search for immobile places would in principle not be thwarted by any supposed motion of the cosmos as a whole, whereas it would indeed be thwarted if we had no immobile reference points within the cosmos. So perhaps we should not look as far as the immobility of the universe to secure the required immobility of places.

On the basis of these considerations, I do not think it very likely that Aristotle’s claim that “the whole river is rather the place” refers to the surrounding surface of the boat-sized hole in the cosmos. One would rather expect it to refer to the surface of the surrounding river, taken in abstracto, i.e. as a geographic entity, following the interpretation of the earlier mentioned medieval commentators (an interpretation which has been taken up some time ago in a slightly different way by Myles Burnyeat).²⁹ This surface, we may surmise, derives its immobility from the immobility of the river qua geographical entity, which has a fixed position on the immobile earth, which in its turn has a fixed position with respect to the heavenly spheres. Nevertheless, even this solution remains problematic in that (a) the roughshod phrase “the whole river is the place” needs translation and unpacking and (b) the solution still presupposes a distinction between the surface qua surface of the surrounding water and the surface qua the surface of the surrounding immobile river as a geographical entity – a distinction which is not provided in the context of these particular passages, nor indeed elsewhere in Phys. 4. Consequently, we need not be surprised, pace Morison , that the problem of the immobility of place remained on the agenda in the later ancient and medieval commentary traditions, starting, as I believe, with Theophrastus.30

 

(iv) The location of the heavens. The text of the first part of chapter 5 of Phys. 4, which deals with the subject of the emplacement of the (outer sphere of the) heavens (212b8–21) is extremely condensed and difficult. Aristotle appears to claim that the ouranos is not in a place as a whole, but that it has places for its parts in so far as they move and contain each other (hence, they somehow act as each other’s places). The fact alone that Aristotle designates his subject as “the ouranos” does not make matters easier. After all, in Aristotle, even in this single context, the word ouranos can refer either to (1) the whole cosmos (as a synonym of ‘the universe’ or to pan), or to (2) the outer sphere of the heavens, or to (3) the heavens as a whole. Interpretations of what Aristotle is saying (and especially of what he means by “the parts” of the ouranos) naturally differ according as one opts for (1), (2) or (3). The translation of Waterfield and Bostock (1996), for example, opts for (1) and takes the whole of 212a31-b22 to be about the (place of the) universe. Hussey (1983) 119 rather assumes that Aristotle is moving between the various senses of ouranos, as indeed does Philoponus in the various sections of his commentary.31 Simplicius (Ph. 594, 35–37) actually complains that “it is clear that he was calling either the whole universe or the whole of that which revolves ‘the heavens’, but he created much unclarity in the passage before us by saying sometimes ‘the heavens’ and sometimes ‘the universe’”.

But perhaps we should leave the problem of the lack of clarity in the presentation for what it is and move on to the underlying conceptual problems. Whether ouranos refers to the outer sphere, or to the heavens as a whole, or to the cosmos as a whole, the cosmos is said not to be in a place. Is there a problem here at all? One might say that these three entities all do indeed lack a container, so that from an Aristotelian point of view it is hardly a problem that they are not in a place. If only those things are in a place which have surroundings, then these three objects do not need to be emplaced. However, this would be to ignore two further problems. One is that it is surely counter-intuitive that a particular part or parts of the cosmos are not in a place. This is not an anachronistic or un-Aristotelian worry, for Aristotle himself appears to have subscribed to the first premise of Zeno’s paradox, viz. that all things that exist are, if not in a place, then at least ‘somewhere’ or ‘in something’ (see also 208a39: “the idea that existing things are somewhere is universally accepted”). So why not the heavens? We do have a problem here, it seems. Another problem is that the havens do in fact exhibit a form of locomotion, for the spheres rotate. How can we have a form of locomotion without change of place? Aristotle’s solution in the obscure first part of ch. 5 seems to come down to the claim that

• (a) ouranos, in whatever meaning of the word he has in mind here, is indeed in place, but only in virtue of its parts; and that
• (b) the kind of locomotion involved is rotation and that this means that its parts exchange places without the ouranos as a whole doing so.

So we have a location for the ouranos, not as a whole but in virtue of its parts; and we have a form of locomotion, in the proper sense of changing places, not for the ouranos as a whole, but for its parts. How are we to interpret this?

The commentary tradition comes up with two ways in which this could be worked out, each of them equally unsatisfactory. One option is to take this passage to be about the heavens as a whole, in which case the reference to “the parts” and their respective motions is taken to be to the nested spheres. The problem with this is that it does not leave us with a place which can serve as the measure of rotation; for during its rotation each inner sphere remains in the same outer sphere. Secondly we are left with the outermost sphere, which on this interpretation should still be taken not to be in a place at all, for it has nothing to surround it from outside, unless we take it to be located, exceptionally, not in a concave surrounding surface, but in the convex surface of the inner sphere of Saturn, as some commentators appear to have suggested.32 Another option is to take the whole passage to be about the outer sphere alone, and to take the reference to the parts to be to the continuous parts of this outer sphere itself. The problem with his interpretation is that on Aristotle’s own line of thought, and as we noted above in subsection (i), the parts of a continuous whole are not in a place properly speaking. Moreover, in the process of the rotation of the outer sphere these parts do not in fact change place relatively to each other, so they can hardly constitute the places that measure the rotation of the sphere.

A possible way out for an Aristotelian would be to claim that locomotion is restricted to the kind of rectilinear motion that we witness in the sublunary world, and that only substances in that region are the sort of things that need places to explain their motions. In that case the fact that the outer sphere and the heavens as a whole are not in place, although they rotate, could be seen as no longer problematic because rotation would no longer be treated as a subspecies of locomotion, but as a separate species of change (next to locomotion, qualitative change etc.) in its own right, one which does not require a place to start from, nor a place to move into. This appears to have been the option chosen by Alexander of Aphrodisias.³³ However, as Simplicius notes in the first part of his Corollary, there are passages where Aristotle emphatically does claim that rotation is in fact one of the subspecies of locomotion or kinēsis kata topon.34 So at the very least one does get the impression that Aristotle had not thought this through sufficiently.

In his Corollary on Place Philoponus has this to say on the subject:

Hence, when they try to explain how the sphere of fixed stars could move in place when it is not in place, they throw everything into confusion rather than saying anything clear and persuasive. For they cannot deny that the sphere moves in place, because they cannot even make up a story about what {other} kind of motion it would have. However, they cannot explain what is the place in respect of which it moves, but like people playing dice they throw out first one account, then another, and through them all they destroy their original assumptions and agreements. For by concealing the weakness of his account with obscurity, Aristotle licensed those who want to change their stories however they wish (Phlp. Ph. 565, 12–21).

But we need not resort to the critical Philoponus. Even Ben Morison has to conclude that it is “a problem which is recognized and tackled by Aristotle, but unsatisfactorily”.35

3 Eudemus of Rhodes

Simplicius starts his Corollary on Place, which is appended to his discussion of Phys. 4, 1–5, by remarking that Aristotle’s account “contains many difficulties and offered many lines of examination to those who came after him”. In the next few sections (3, 4, 5) I want to examine to what extent this judgement applies to the first two generations of Aristotelian philosophers: Eudemus of Rhodes, Theophrastus of Eresus and Strato of Lampsacus. I will not provide an exhaustive discussion of the evidence on these philosophers, nor will I discuss everything they thought and wrote about place, space and void. but I will focus instead on those fragments that can be linked to any of the four problems that were discussed in the previous section. Let us start with Eudemus.

Eudemus of Rhodes returned to his home island in 323, when after the death of Alexander the Great, Aristotle had to leave Athens and Theophrastus became scholarch. In Rhodes he started a small-scale philosophical school of his own. Gottschalk describes him as a “worthy professor, battling to instil the rudiments of Aristotelian philosophy into an undistinguished group of students”.36 His Physics appears to have been based on a course of lectures covering the same subjects as Aristotle’s Physics and in the same order, except that it contained nothing corresponding to what we now know as book 7.³⁷ It has been argued, accordingly, that his selection of material from Aristotle anticipated the selection later supposedly made by Andronicus of Rhodes.³⁸ He seems to have been virtually unknown in the Hellenistic era, but some of his works, among which the Physics circulated during the renaissance of Aristotelianism in the first two centuries AD. Eudemus was much quoted by Alexander of Aphrodisias and through him his ideas reached the later commentators, although also Simplicius appears to have read his Physics for himself (in fr. 44 Wehrli he claims that he is unable to find a quotation provided by Alexander in Eudemus’ own text). I think the work can be characterized as a shortened paraphrase (it seems to have comprised four or five books) with some additions and clarifications, perhaps comparable to what we later find in Themistius (whose work was also freely used by later commentators).

What remains of Eudemus’ discussion of place in his Physics is in a sense a mixed bag. Some fragments are merely a paraphrasing explanation of what is in Aristotle, comparable to what we find in the later exegetical paraphrase by Themistius. Other fragments try to clarify and articulate Aristotle’s account at various points, and in doing so they may go beyond what is in Aristotle and introduce slight modifications. An example of such a slight modification can be found in a passage in which Simplicius tells us that both Theophrastus and Eudemus took the immobility of place to be among the initial axiōmata of the theory.39 Aristotle, we may recall, did not include the immobility requirement in his axiōmata, i.e. in his revised list of basic phainomena at the beginning of chapter 4, but added it only later on, at the end of the chapter (212a14 – 21). The modification proposed by Theophrastus and Eudemus does not affect the core of Aristotle’s theory, but straightens out his argument and makes for a clearer presentation.⁴⁰

The immobility of place recurs as a theme in another fragment which may be taken to offer an example of a further articulation of what is in Aristotle. On closer view, the problem of immobility involves two sub-questions: (a) with respect to what should we specify the immobility of place, and (b) can we find places as surrounding surfaces that are indeed immobile in the required sense? Eudemus focuses on the first question. He answers it by saying that we determine immobile places in relation to the heavens:

Having said that place must be the limit, in so far as it surrounds, of the surrounding body which was immobile, he [scil. Eudemus] added: “For that which moves is like a vessel, and that is why we determine places in relation to the heavens. For they do not change place, except in their parts” (Simp. Ph. 595, 5–8; part of Eudemus fr. 80 Wehrli).

In doing so he may well have taken his exegetical cue from the passage in Aristotle which immediately follows on the final definition of place as the “first immobile limit of what contains”. For Aristotle goes on to claim that this definition takes account of a number of basic phenomena, starting out by saying that

We can now see the reason why the centre of the world and the inner limit of the heavenly revolution are taken to give us ‘above’ and ‘below’ in the most basic sense. It is because of their constancy: the centre is absolutely stable and the limit of the rotation always stays in the same state (Arist. Phys. 4, 212a21–24).

As Hussey remarks in his commentary, “the present section shows that there are natural places, kept immobile by the permanent structure of the world”.41 If I am correct, Eudemus used this brief reference in Aristotle to immobile natural places to specify the immobility of all places in terms of their relations to the heavens as a fixed point of reference. This means that he answered our sub-question (a), thus adding in a welcome clarification of Aristotle’s conception of immobile places, and showing that immobility is not defined in terms of the immobile universe, but in terms of the immobile heavenly sphere.⁴² The evidence does not tell us whether he also wrote about our sub-question (b).

More interesting, perhaps, for our purpose is a fragment which adds another reason for the difficulty of the subject (i. e. to the reasons already outlined by Aristotle in 212a7–14, a passage quoted above, p. 11):

Eudemus says that a further cause of the difficulty of the problem of place is that [the notion of] place is not easy to grasp, because it altogether escapes us when the body in it is removed, and it is not possible to apprehend it in itself, but, if at all, in combination with something else, like the sounds of the so-called consonants. For with ‘a’ added, the sound of ‘b’ and ‘c’ becomes clear (Simp. Ph. 523, 22–28; Eudemus fr. 73 Wehrli).

Here, I believe, Eudemus wittingly or unwittingly puts his finger on what is primarily an additional weakness of Aristotle’s theory, rather than of any theory of place. After all, in the very passage on which Eudemus is here commenting Aristotle claims that one of the difficulties surrounding our theorizing about place is that we may take the container which remains at rest to be place-as-extension, because it seems “possible that there is an extension between the limits which is not the same as the magnitude of the moving bodies” (212a7– 30, quoted above, p. 11). In other words, the rival conception does allow us to think of place as something existing in its own right. For Aristotle’s own conception, however, this is different. As we saw in the previous section, this conception usually does not allow us to specify a place that an object has left behind or to specify the place where an object is going to end up before it has moved over. That this objection was still ‘around’ later in the Hellenistic period can be inferred from the critique of the Peripatetic theory of place in Sextus Empiricus PH 3, 131, which no doubt has earlier collections of sceptical arguments as its proximate source,43 but which may ultimately be traced back to doubts raised within the Peripatetic tradition of the kind here presented by Eudemus:

But if this is what place is, then the same thing will both exist and not exist. When a body is about to come into being at a certain place, then in so far as nothing can come into being in what does not exist, the place must pre-exist in order that in this way the body may come into being in it. And for this reason the place will exist before the body which is in the place comes into being in it. But in so far as a place is effected when the surface of what includes encloses what is included, a place cannot subsist before the body comes to be in it; and for that reason it will not then exist (S.E. PH 3, 131).

It appears, then, that surrounding surfaces are not just items that can only with much difficulty, if at all, be given the required immobility, they are also items that are hardly stable enough to serve the purpose places should serve, viz. being a container that can be filled and then left behind. As I have argued in the previous section, this is not a worry that stems from considerations that are external to Aristotle’s own theory, but rather one that follows from his own requirement that place should be “left behind by each object and be separable” (211a2–3), a requirement that is more easily satisfied by the rival conception of place as a three-dimensional extension. Of course it is also satisfied by vessels. But not by non-permanent Aristotelian places. Here we may be hitting upon a central problem. One might say that Aristotle’s attempt to locate bodies primarily in terms of their surroundings is in principle a reasonable one – working out, as we saw, common sense ways of thinking and speaking, like ‘a fish is swimming in the water’ or ‘I am in Athens’. But the problem seems to be his reification of place as a surrounding something. Perhaps, in other words, the comparison with the vessel was not such a good idea after all.

4 Theophrastus of Eresus: frs. 146 and 149 FHSG revisited

This brings us to Theophrastus. His Physics appears to have differed from its Eu-demean counterpart in several ways. For one thing, there appears to have been no systematic correspondence between the order of its books and the books of Aristotle’s Physics.44 For another, Theophrastus’ text appears to have had rather different aims: where Eudemus basically provided an exegetical paraphrase, probably for scholastic use, Theophrastus appears to have felt free to raise puzzles, to improve Aristotle’s theory on some points, and to disagree with him on others.⁴⁵ Nevertheless, we have no reason to see his work otherwise than as aiming to continue Aristotle’s project, while following Aristotle in broad outlines.

The two most relevant fragments dealing with place are both to be found in Simplicius Corollary on Place. The first (fr. 146 FHSG) lists a set of five aporiai that concern Aristotle’s account. Although, as we shall see, these aporiai can be seen as objections that are to be taken seriously, we are not told whether Theophrastus thought them decisive. The second fragment (fr. 149 FHSG) offers what looks like a cautious suggestion, but it is not immediately clear what this suggestion amounts to and to what extent it constitutes an alternative to Aristotle’s account. Some earlier scholars, such as Jammer and Sambursky, claimed that together these fragments show that Theophrastus actually rejected Aristotle’s concept of place and replaced it with a concept of place as a relation, more or less resembling the concept that would be defended by Leibniz later on.46 Sorabji provided an alternative interpretation of these fragments as indicating that Theophrastus rejected Aristotle’s theory of natural places.⁴⁷ I myself have argued elsewhere that the two fragments under discussion here appear to fit in with the overall picture of Theophrastus which I have just sketched: that his critical attitude merely shows itself in his leaving the aporiai of our first fragment unanswered, without the implication that Aristotle’s theory should therefore be rejected; and that the second fragment presents us with hardly more than the suggestion that perhaps we should not regard place as something that is really there (i. e. the surface of a surrounding body), but rather as a concept we use to denote the order and position of things.⁴⁸ It is likely, in my view, that it is precisely in virtue of this cautious way of presenting this suggestion that, contrary to what has been suggested by Sorabji,⁴⁹ Theophrastus’ ideas about place did not become widely known. Outside the context of these few passages in Simplicius, we find no references to them.

Recently Ben Morison has come up with an even more watered-down interpretation of these two fragments. In his view the aporiai in the first fragment (fr. 146) should not be read as serious objections at all, but as harmless puzzles or “clarificatory devices”.⁵⁰ In his view all of them “can be given Aristotelian answers”,⁵¹ although he admits that in most cases no straightforward answers are forthcoming either from Aristotle’s own texts or from what remains of Theophrastus’ Physics. He then goes on to interpret our second fragment (fr. 149) as in fact supporting or actually restating Aristotle’s theory. So Aristotle’s theory of place – which, we may recall, Morison sees as basically unobjectionable –comes out shining and unscathed: Theophrastus signals no serious problems and offers no alternative view, cautious or otherwise.

Given these divergent assessments, and especially against the background of Morison’s challenging new interpretation, a fresh look may be appropriate. First the aporiai of fr. 146 FHSG. The text, as noted, comes from Simplicius’ Corollary on Place. In the first few pages of this Corollary Simplicius lists a number of objections against Aristotle’s conception of place. After having asked “where then is place, i. e. which things are properly in place? For both air and water are in motion, while individual bodies in general are in flux in the air or water”, he goes on as follows:

One should know that in his Physics Theophrastus too raises difficulties (ἀπορεῖ) of the following sort against Aristotle’s account of place:

(1) a body will be in a surface;

(2) place will be in motion;

(3) not every body will be in a place; for the sphere of the fixed stars will not;

(4) if the spheres are taken together, the heaven as a whole will also not be in a place;

(5) things in a place will no longer be in a place if, without their changing themselves at all (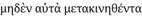), their surroundings are removed (Simp. Ph. 604, 5–11; Theophrastus. fr. 146 FHSG).

Let us first review the five aporiai once again, with a view to their potential strength.

Aporia 1 (a body will be in a surface): the most natural way to take this aporia is as an application of Aristotle’s own requirement that places should be equal (i.e. “neither larger nor smaller”, Phys. 4, 211a2) to the emplaced body. For how can a surface be equal in size to a body? Morison thinks the problem can be easily solved: “the size of the surface should be calculated in this instance as being not its area, but the volume it encloses”.52 And his everyday example of my coat being ‘my size’ meaning that it encloses me snugly has a certain prima facie appeal. One may still wonder, however, whether this does not involve a certain amount of cheating with the notion of ‘size’. Moreover, one might object that taking the size of the place in the sense of the volume that is being enclosed, and then saying that this is equal to the size of the emplaced body, amounts to surreptitiously introducing the notion of place as a three-dimensional extension. For if the size of the place is the volume that is being enclosed, and place is different from what is emplaced, then one might well conclude that place is an enclosed volume independent of the enclosed body, and in that sense equal in size to it.

One may doubt, in other words, whether this Aristotelian answer really works. It should be granted, however, that this aporia may not be the most damaging one. Perhaps the best way to solve it would be to say that the original requirement that place should be neither larger nor smaller should be reformulated in more neutral and less obviously quantitative terms, e. g. by claiming that place should be ‘exactly fitting’ – for this would accommodate both the concept of place as an extension (being exactly covered by what is emplaced) and the concept of place as a surrounding surface (exactly surrounding the emplaced).

Aporia 2 (place will be moving): here as elsewhere the future tense (estai) indicates that we are dealing with a supposed consequence of Aristotle’s conception of place. It is an undesirable consequence, we may suppose, because both according to Aristotle and according to Theophrastus himself (as we can infer from some other fragments) place should be immobile.⁵³ But why would this be a consequence of Aristotle’s account or theory? I have argued earlier that this is because Aristotle defines place as a surrounding surface, and surrounding surfaces are the surfaces of mobile substances.⁵⁴ So strictly speaking – i.e. without invoking the “surfaces taken in abstracto” of the later medieval commentators (on which see section 2 above, p. 20) – there are no immobile surrounding surfaces. Theophrastus would thus be the first to have signalled the problem of the immobility of place as a problem which specifically arises for the Aristotelian theory, in which, as we saw, he would be followed by many others.

Now we saw that Ben Morison claimed that Aristotle actually solved the problem of immobility by taking place to be the surface of the whole surrounding cosmos, for this cosmos is immobile in the relevant sense. So how, on his view, can Theophrastus present moving places as a consequence of Aristotle’s conception of place? The answer is that Morison prefers to connect this aporia with a fragment (to which I briefly referred in the previous section on Eudemus) which suggests that both Eudemus and Theophrastus claimed that the immobility requirement should not have been added to the definition, as it is in Aristotle (at the end of chapter 4), but to the axiōmata of the theory (as given at the beginning of chapter 4).⁵⁵ The solution to the aporia is then supposed to be easy: add the requirement to the axiomata, as proposed by Theophrastus himself, and all is fine. But this is odd. The point made by Theophrastus and Eudemus about relegating the required immobility of place to the axiōmata appears to be one of presentation only. Why would Theophrastus claim that adding the requirement of immobility to the definition rather than to the starting points would have the effect that place – presumably: any place – will as a matter of fact be moving? On balance, I do not think this interpretation offers a convincing way to read, and then defuse, the second aporia.

Aporiai 3 and 4 (outer sphere and heavens as a whole are not in a place): I have already discussed this problem as one of the ‘classic’ problems associated with Aristotle’s theory in the later tradition. Morison, as we saw, recognizes that “Theophrastus has put his finger on a problem which needs spelling out explicitly – a problem which is recognized and tackled by Aristotle himself, but unsatisfactorily”.⁵⁶ He also claims that “nothing indicates that Theophrastus meant this to be a devastating objection”,⁵⁷ to which I agree, and adds that all aporiai, including this one, can in the end be given an Aristotelian solution, which is something I find it harder to go along with, for no satisfactory solution emerges from the tradition.

Aporia 5 (take away the surroundings, and a thing is no longer in a place, without it changing or moving itself in any way): I agree with Morison against Sorabji that this aporia is not about a situation where a thing’s surroundings are in motion, but rather about the situation where a thing’s surroundings are removed altogether. According to Morison this is “an unexpected consequence of Aristotle’s theory, but not a weakness”.⁵⁸ For he locates the paradoxical aspect in the fact that such a body appears both to have moved (for it is no longer in its former place) and not to have moved (for it has not exhibited any motion itself). And then, he argues, the solution is easy: you should not say ‘x has moved from place p if x is no longer in place p’, but rather ‘x has moved from place p if x is no longer in place p but in place q’.

Against this we should first note that the words 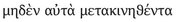could equally well be translated as “having changed in no way” rather than “not having been moved themselves”, so that the issue would not be about things paradoxically having moved and not having moved at the same time, but rather about their suddenly finding themselves without a location, without having themselves changed in any way. But even if we adopt Morison’s translation, we should acknowledge that the way in which the aporia is phrased shows that the focus is not on the paradox identified by Morison (i. e. it is not primarily about moving and not moving), but that the unexpected consequence is rather (once again voiced in the future tense) that “these things will no longer be in a place” (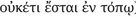). And this is arguably not just an unexpected consequence, but also a weird one. We are dealing with a thought experiment about emplaced things, i. e. substances within the cosmos. If we take away their immediate surroundings and (counterfactually) create a void around them, these substances suddenly find themselves without a location. Yet, we know that all cosmic substances are supposed to have a location and indeed we can still indicate where these substances are with respect to the remaining elements of the cosmos, even if their direct surroundings are no longer there. I am not saying that Theophrastus thought this to be “evidence that Aristotle’s account of place is doomed”.⁵⁹ After all there is no evidence, as we saw, that he rejected the theory in the end. But examples such as this could very well have led him to the cautious suggestion (presented in the second fragment) that perhaps place is not a surrounding thing, but rather a term we use to indicate a thing’s relation to its surroundings. For that would be a way to circumvent this fifth aporia.

But this would be to jump to conclusions concerning the meaning of our second fragment. Let me first take stock of this discussion of the five aporiai. I grant Ben Morison that the first one is perhaps rather innocuous, although some work is needed to get it out of the way, and although later commentators did in fact repeat it (see e.g. Phlp. Ph. 563, 31–564,3). But the solutions Morison offers for aporiai 2 and 5 do not appear to address the problems that are at stake, and he does not even offer a solution for numbers 3 and 4, but merely suggest that a proper Aristotelian solution can be found even though, as we have seen, no satisfactory solution appears to have been offered within the Aristotelian tradition. All in all, then, I think we are entitled to conclude that even if (as I agree) Theophrastus somehow did not think of these aporiai as fatal to Aristotle’s theory, he did signal them as problematic aspects of that theory.

Let us now move on to our second fragment:

Also Theophrastus seems to have subscribed to this view in his Physics, in a passage where he continues his account in an aporetic fashion (Morison: as one who in an impasse tries to advance the argument) and says: “Perhaps place (topos) is not a substance (ousia tis) in its own right (kath’ heauton), but we speak of it because bodies have an order (taxis) and position (thesis) in conformity with their natures and powers, and similarly also in the case of animals and plants and in general of things with a differentiated structure, whether animate or inanimate, if they have a nature that exhibits form. For in these too there is an order and position of parts in relation to the whole substance. And this is why each of them is said to be in its own place through having its own proper order. For each part of the body too would desire and demand its own place and position” (Simp. Ph. 639, 13–22; Theophrastus fr. 149 FHSG).

On my own earlier reading of this fragment, it offers, as a cautious suggestion, an alternative way of conceiving place.60 Ben Morison, by contrast, argues that the fragment contains nothing that could not be endorsed by Aristotle too, and that it thus testifies to Theophrastus’ complete orthodoxy in so far as the theory of place is concerned.⁶¹ In order to take things further, I think three questions in particular need to be answered:

• (1) How does this passage fit into the overall structure of Simplicius’ overview in the Corollary on Place? More particularly, what do the words “this view” in the first line refer to?
• (2) What are the implications of Simplicius’ claim that Theophrastus “continues his account in an aporetic fashion” – or, if we prefer Morison’s translation, which seems equally possible: “as one who in an impasse tries to advance the argument”?
• (3) What is the nature of the view set out by Theophrastus in the actual quotation?

As for question (1), it is clear from the larger context that Simplicius sees Theophrastus as anticipating some aspects of the theory of place of his teacher Damascius. But only some aspects, since Damascius’ theory is presented as “novel”.62 So which aspects does he have in mind? I think he makes this quite clear in the diaeresis of conceptions of place which he offers at Ph. 641, 23 ff., at the end of his Corollary. This diaeresis is based on the various ways in which different theories of place can be seen to work out what Simplicius describes as the koinē en-noia, or common conception, of place:

But now it should be added to the above that there is a common conception of the whole of place which says that it is the determination of the position of each distinct thing among entities. This determination is either made in terms of (i) the receptacle ( χήν) or (ii) the container/what surrounds (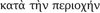), or (iii) the ordering of the position of each distinct thing among entities ( 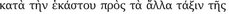θέσεως) (Simp. Ph. 641, 23–27).

He then goes on to assign Aristotle’s conception of place as a subspecies to type (ii) (642, 11–13), whereas he assigns Damascius’ view to type (iii):

This, I said, was the view of Damascius, the head of our school, and it was vouched for by Theophrastus and Iamblichus (Simp. Ph. 642, 17–19).

This final diaeresis of conceptions of place thus offers a classification according to what me might call the morphology of place: other aspects (such as the dynamic or non-dynamic character of place) are left unconsidered, which is why we can find Theophrastus lumped together with two in other respects very different Neoplatonic theories (those of Iamblichus and Damascius). This classification shows that Simplicius reads Theophrastus’ suggestion as at least an anticipation of the view of place primarily in terms of taxis and thesis, rather than as an elaboration of the Aristotelian view of place as a containing something. Of course we cannot exclude that he is mistaken, but he almost certainly had direct access to the text of Theophrastus’ Physics,63 and should accordingly be considered capable of seeing this quotation against the background of its larger Theophrastean context. We have no prima facie reason to distrust his evidence.

As for question (2), Morison rightly, in my view, explains that “being in an aporia” here means that you are considering a question, and that you have considered and rejected all the answers which strike you as possible, but end up having nowhere to go. “Advancing the argument” then means: bringing in a new set of possible answers. So we have a conception X of place as an ousia which somehow leads to puzzlement, and then we are given an alternative Y. But, in this case, what is X and what is Y? On the view I defended earlier, Theophrastus, having considered a number of puzzles surrounding Aristotle’s conception of place as in some relevant sense a thing, ventures to suggest the alternative view that place is a concept which has no clear denotation, but is used to indicate a body’s relation (taxis and thesis) to its surroundings. So, on this interpretation, X is the Aristotelian view and Y is an alternative conception. Yet it is an alternative which, in so far as it still defines place in terms of surroundings, might be thought to be acceptable to an Aristotelian as well. Hence the presentation of Y as a cautious, but apparently not immediately implausible, suggestion.

Morison, by contrast, argues that it is Y that actually presents us with Aristotle’s considered view, and that X, the conception that induced the aporia, must be a non-Aristotelian conception of place, for example the view which takes place to be an independently existing extension.⁶⁴ But if this is the nature of the contrast between X and Y – a clearly non-Aristotelian view being replaced by the Aristotelian view – one wonders where the puzzlement on X comes from (the conception of place as an independent extension, for example, had been rejected by Aristotle in no uncertain terms, even if we may regard his arguments as inconclusive) and why Y is being introduced so tentatively with the words “perhaps place is not an ousia, but etc.”. Doesn’t this rather suggest that X and Y are not radically opposed alternatives, but rather two views which might both seem to fit within the overall framework of Aristotelian physics?

This brings me to (3). It might be argued that conception X, which Theophrastus here presents as the problematic one, cannot be the Aristotelian one, for Aristotle does not in fact hold that place is a substance (ousia). In a strict sense this is true. But there are two less strict senses in which Aristotelian places may be said to be substances. First of all, place qua surface falls under one of the senses of ousia outlined in ch. 8 of Metaphysics Γ. The third class of entities which may be called substance is there defined as follows:

The parts which are present in such things [i. e. simple bodies, substances in the primary sense] limiting them and marking them as individuals, and by whose destruction the whole is destroyed, as the body is by the destruction of the plane, as some say, and the plane by the destruction of the line (Arist. Metaph. 1071b17–20).

Secondly, Aristotle more than once uses a reference to a thing’s surrounding substance as a kind of shorthand for a proper reference to a thing’s place. Think of the phrase “the whole river is the place”, or of the way in which the beginning of chapter 2 of Phys. 4 tells us that we are in the world, in the air and on the earth, thus claiming that these are all somehow our places. It appears to me, in other words, that the label ousia can in a broad sense be applied to Aristotelian places. At any rate I do not see that it would be better suited to capture the three-dimensional extension of the opposite party, which, of course, is not an ousia in any Aristotelian sense at all.

Aristotle defines places in terms of surroundings rather than in terms of an underlying extension, and our fragment shows us that Theophrastus does so too. So in that sense the suggestion made in our fragment is not completely alien to Aristotle’s thought; indeed it may count as a sensible elaboration. But it is an elaboration, not something which is already explicitly present in Aristotle’s text. For, as noted before, in Aristotle we do seem to witness a certain reification of place: place is a surface and as such a surrounding something – the vessel analogy suggests as much – and hardly an epiphenomenon, as Morison seems to suggest.65 And this arguably led to precisely the kind of problems signalled in Theophrastus’ aporiai: as a surrounding something place may be thought to move, viz. along with the substance of which it is the surface (the subject of the second aporia); and bodies without a surrounding something – the outer sphere of the heavens, the heavens as a whole, intra-cosmic substances of which the surroundings are thought away – paradoxically have no place (the subject of the last three aporiai). The fact that our fragment can thus be seen to cohere with the aporiai of the other fragment we discussed is one reason to take it as a cautious suggestion that one might consider an alternative view, rather than a straightforward endorsement of Aristotle. Other reasons are, as we saw, that this seems to be the way in which Simplicius, who could see the fragment in context, read it; that it makes sense of the way in which Simplicius classifies the relevant position in his final diaeresis, viz. as a position different from the position held by Aristotle; and that it makes better sense of the tentative way in which Theophrastus introduces his suggestion.

5 Strato of Lampsacus

Strato of Lampsacus became head of the Peripatetic school in Athens after Theophrastus (c. 287 BCE) and kept that position until his death (269 BCE). That he showed serious interest in the problems of space and void is shown by the catalogue of his works, preserved by Diogenes Laërtius 5, 59 (= fr. 1 Sharples),66 which informs us of the fact that he wrote a 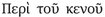(On the Void). The evidence suggests that in his conceptions of place and void he departed from Aristotle in three important respects:

• (1) he replaced Aristotle’s conception of place as a surrounding surface by the rival conception of place as a three-dimensional extension; unfortunately we do not have his arguments, but we may be sure that he was not convinced by Aristotle’s arguments against this view and we may presume that he was also influenced by the puzzles raised by the first generation of Peripatetics, i.e. by Eudemus and Theophrastus;
• (2) he seems to have disambiguated the use of the term kenon: where Aristotle uses it both to denote space as a three-dimensional extension and to denote an actual void or empty pockets in substances, Strato seems to have preferred topos as a term to refer to occupied space and reserved kenon to refer to an actual void;⁶⁷
• (3) although he followed Aristotle in believing that the cosmos is a plenum which as such does not contain stretches of actual void between substances (and does not need them to explain the locomotion of substances), he did support the notion of void interstices or empty pockets or pores within individual substances as a notion required to account for particular physical processes, such as compression and the propagation of light through diaphanous substances.

I cannot here discuss the complex evidence concerning Strato’s theory of micro-voids (items (2) and (3) in the survey above),68 but will only add some observations on his conception of place (i.e. on (1). According to Simplicius, in the diaeresis with which he begins his Corollary, Aristotle’s conception of place as two-dimensionally extended, i.e. as a containing surface, was supported by “the whole Peripatos” (Ph. 601, 20)). A few lines further on this same Simplicius shows that this view should be taken cum grano salis, when he adds Strato as an adherent of the rival view that place is three-dimensionally extended:

Some say that it is three-dimensional. Of these some said that it is absolutely homogeneous and sometimes remains without any body, like the followers of Democritus and Epicurus, some that it is an interval, always containing body and fitted to each (ἐπιτήδειον πρὸς ἕκαστον), like the well-known Platonists and Strato of Lampsacus (Simp. Ph. 601, 23 – 24; Strato fr. 27a Sharples).

Who the Platonists here mentioned are supposed to be – and why Plato himself figures elsewhere in this diaeresis among those who think of place as completely dimensionless – remains a bit puzzling, but the reference is probably to Platonists who took their cue from the spatial characterizations of the receptacle in the Timaeus.69 Perhaps we find a glimpse of such a conception in the section on the physics of Antiochus of Ascalon in Cicero Acad. 1, 27 which, after having claimed that matter can be infinitely divided, makes the same claim for the intervalla over which bodies move, i.e. presumably: space.70 We should also note that the conception of place as “an interval always containing body”, but “empty in its own right”, was also defended by Simplicius’ near-contemporary Philoponus, with whose commentary on the Physics Simplicius was familiar.71

However this may be, that Strato of Lampsacus defended the conception of place or space here ascribed to him is borne out by several other pieces of evidence. First, there is a text which has often been neglected by those who studied Strato’s theory of space and void, probably because it originally did not belong to any of the purely physical treatises (it is not cited by Simplicius in a physical context) and because Wehrli, rightly, printed it among the logical fragments. It is a fragment from Strato’s book 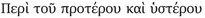(On prior and Posterior) preserved by Simplicius in his commentary on the Categories. We are dealing with a summary by Simplicius of Strato’s listing of a number of ways in which one thing can be ‘prior’ to another – ways which according to Simplicius can all be subsumed under the five forms of being ‘prior’ recognized by Aristotle (Cat. 14a26). Although the text is not a literal quotation, we may be sure that the examples are by Strato. After all, Simplicius’ point is precisely that these examples all fit into the Aristotelian scheme. One among the various ways in which one thing can be prior to another is ‘being prior by nature’:

As prior by nature, as [i.e. in Aristotle’s terms] not admitting of a reversal of the order of being/of the ontological priority (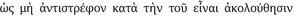 [we may regard Strato’s example of] that which is capable of existing when the other does not exist, like place (topos) in relation to body and body to colour (Simp. Cat. 432, 1 ff.; Strato fr. 15 Sharples).

In the same context place is also adduced as an example of yet another kind of priority: it is also said to be prior to time in so far as it does not participate in coming to be and passing away. Together these claims make clear that we are dealing with the un-Aristotelian conception of place as a self-subsistent three-dimensional extension. The view that there is such a place or space and that it is ontologically prior is listed by Aristotle among the general phainomena from which his investigation in Phys. 4 takes its start. He takes it to be implied by He siod’s talk about the role of chaos in the cosmogony (Phys. 4, 208 b34–209a3), but he himself of course in the end rejects it:

If place were such, its power would be astonishing, and prior to all other things (πρóτερα πάντων). For that without which nothing else is, but which can itself exist without all other things, is necessarily prior to everything else. For place [in that case] is not destroyed when the things in it are destroyed (Arist. Phys. 4, 208b34–209a3).

Our passage in Simplicius, though not a verbatim quotation, suggests that Strato was prepared to turn the tables on Aristotle on precisely this point, and to defend the very ontological priority of space which Aristotle thought would be ‘astonishing’.

Strato’s heterodox conception of place was not only reflected in the commentaries of Simplicius, but also in the doxographical tradition. Stobaeus, in a passage which appears to combine materials from Aët. 1, 18 and 1, 19 in Diels’ reconstruction, offers the following doxographical information:

Strato said that there is no void outside the world, but that it can come to be within it – and he said that space/place (topos) is the extension between container and contained (τὸ με 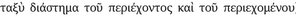) (Stob. Ecl. 1, 18, 1b, p. 156, 4–6 Wachsmuth).

We seem to be dealing with a slightly garbled version of the formula Aristotle uses to describe the conception of place as a three-dimensional extension, as one of the four possible candidates for being place: τὸ μεταξὺ τῶν ἐσχάτων (Phys. 4, 211b8). In Aristotle the context makes clear that the ἔσχατα he has in mind are the . In the doxographical context of Aëtius the addition of the superfluous words καὶ τοῦ περιεχομένου was probably triggered by the co-occurrence of the terms ‘surrounder’ (περιέχον) and ‘surrounded’ (περιεχομένον) in the previous doxa (on Aristotle: τὸ ἔσχατον τοῦ περιέχοντος 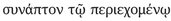).72

This testimony also refers to Strato’s conception of the void. As we noted, Strato claimed that we need to conclude that there is such a thing as pocket-voids within substances (in order to explain particular phenomena such as the propagation of light through transparent substances such as water), but that separate stretches of void are not required as a natural feature of the world. The world is a plenum of substances, and Strato subscribes to the Aristotelian theory of antiperistasis: bodies replace each other without needing an empty place to move into first. In other words, the fact that bodies need a separate place or space to move through does not imply that they need an empty place to move into:

If you drop a pebble into a vessel filled with water and invert the vessel, blocking the exit at the mouth, the pebble moves to the mouth of the vessel as water moves around into the place of the pebble. The same thing happens with swimmers, and fish, and so on (Simp. Ph. 659, 20ff.; Strato fr. 29 Sharples).

This means that Strato here basically follows Aristotle:

Nor do we need the void to explain change of place, since it is possible for things to make way for one another without there being any separate extension besides the moving bodies. It is as easy to see this in the case of rotation of continuous objects as it is in the rotation of liquids (Arist. Phys. 4, 213a28 – 32).

However, he obviously does not share Aristotle’s conclusion that such an independent extension is non-existent. In fact, his commitment to the existence of pocket voids, combined with his commitment to an underlying independent three-dimensional extension, even allowed Strato to leave open the possibility of such an extended intra-cosmic and ‘inter-substance’ void being produced artificially. Hence, presumably, the claim that “it can come to be within it” in Stobaeus’ testimony.73 In addition, both Simplicius and Stobaeus explicitly claim that Strato denied the possibility of an extra-cosmic void. For him – as, many centuries later for Philoponus – space as extension was finite and completely co-extensive with the equally finite cosmos. As a critic of Aristotle he was to be outdone in this respect by Xenarchus of Seleucia, two centuries later.

It is unfortunate that on some important issues (his rejection of Aristotle’s conception of place, but also of the idea of an extra-cosmic void) we only know Strato’s tenets, not his arguments. However, the evidence leaves no doubt that he accepted the main rival conception rejected by Aristotle and refused to follow Aristotle’s own theory of place.

6 Xenarchus of Seleucia, Cleomedes, Alexander of Aphrodisias

In the late Hellenistic period, when Stoic cosmology had gained a certain dominance, Aristotelians had to take their stand vis-à-vis the Stoic conception of space, and of the extra-cosmic void in particular. In principle they could do this on the basis of the arguments offered by Aristotle in chapters 6 to 9 of Physics 4 and at the end of the first book of the On the Heavens. In Phys. 4, 6–9 Aristotle offers a range of arguments to show that the void does not and cannot exist. Some of these arguments simply conceptualize the void as an independent three-dimensional extension, so that there is some overlap between this discussion and the critique of the conception of place as extension in the first sections of Phys. 4. For our purpose the most relevant arguments are two that concern the ontology of such an extension (whether occupied or empty):

• (1) There is basically only one type of extension, and that is the extension of substances (cf. 216b9–10: “what will be the difference between the body of the cube and the void and place which are equal to it?”)
• (2) Places (on the basis of Aristotle’s own theory, that is) are always the places of emplaced substances (cf. 216a23–26: “Some people think that without a void, separated off in its own right, there could be no change of place. But this is no different from claiming that there is such a thing as place in isolation from what occupies it, and I have already argued that this is impossible”).

So the conception of the void used (and rejected) by Aristotle is basically the conception of place as an independent three-dimensional extension. And in fact, once conceived as independent, such an extension can also be conceived as empty, i.e. as void. The first of the arguments just quoted argues against this conception by claiming that extension is always the extension of some substance, the second makes a similar claim for places: they are always the places of something. This means that places and emplaced bodies are actually correlatives: just as every body is in a place, so every place is the place of a particular body. It is clear from our examination of Strato that he rejected argument (1) in so far as he was prepared to accord ontological priority to space; but we may presume that he also rejected (2). After all, he did not regard an empty place as a conceptual impossibility, even if he also maintained that the cosmos is as a matter of fact structured in such a way that such an empty place will not naturally occur. In the case of the first century BC Peripatetic Xenarchus of Seleucia, however, we have evidence of an explicit rejection of (2).

According to his pupil Strabo, the Peripatetic Xenarchus of Seleucia taught in Alexandria, Athens and, finally, Rome, and was befriended by Arius (probably Arius Didymus) and by the emperor Augustus.74 In his commentary on Aristotle’s On the Heavens Simplicius provides us with a whole list of arguments from Xe narchus’ work On the Fifth Substance in which he criticized Aristotle’s claims (Cael. 1, 2) concerning the existence of a fifth element with a circular natural motion.75 These arguments show that he was prepared to dissent from Aristotle on crucial points. Elsewhere in the same commentary Simplicius quotes Alexander of Aphrodisias reporting an equally heterodox argument of Xenarchus concerning the void (possibly, but not necessarily, also deriving from On the Fifth Substance). It concerns Aristotle’s argument that there neither is nor can be void, space or time beyond the heavens (Cael. 1, 9, 279a12–18).

The relevant passage in Simplicius (Cael. 285, 27–286, 15 Heiberg) shows that Alexander quoted it as a possible counterargument in the context of his own polemical discussion of the Stoic conception of an extra-cosmic void, which adduced three arguments:

• (1) the existence of an infinite void implies the existence of an infinite body which can fill up this void, which, even on the Stoics’ own presuppositions, is absurd;
• (2) why wouldn’t the cosmos move through such a void rather than be stable?
• (3) the cosmos could move in all directions at once and hence be dispersed into the void; god, qua fire, could not keep it together.

Arguments (2) and (3), which deal with the alleged cosmological consequences of the existence of an extra-cosmic void, are clearly polemical and ad hominem against the Stoics, for they disregard Aristotle’s own point that void would actually preclude motion (Phys. 4, 214b28–33). Argument (1) is the interesting one in the context of our present investigation, for it regards the nature of the void and the way it relates to body. Moreover, unlike arguments (2) and (3), it takes its cue from Aristotle, namely from a passage in the first book of On the Heavens:

It is obvious that there is neither place, nor void, nor time outside the heavens, since it has been demonstrated that there neither is, nor can be body there (Arist. Cael. 1, 279a16–18).

This Aristotelian argument (implicitly) presents body and void as correlatives, and Alexander follows suit. He gives an additional anti-Stoic twist to it by focusing on the presumed infinity of such a supposed extra-cosmic void:

Alexander says […] that if the void is infinite, as Chrysippus claims, and if they say the void is that extension which, while being capable of containing a body, does not actually contain it, and if it is necessary in the case of relatives that if the one exists, the other exists as well, it follows that if there is that which can contain, there is, or can be, also that which can be contained. However they themselves say that there is no body that can be contained by the infinite void, and indeed such a body does not exist. Therefore that which can contain it does not exist either (Simp. Cael. 285, 27–286, 2).

Cleomedes, who wrote his Stoicizing cosmology in the first or second century AD, shows us that the Stoics were aware of Peripatetic criticisms of this kind and that they responded to them. He records versions of all three arguments.⁷³ He also gives us the Stoic responses. His responses to (2) and (3) need not concern us here.76 Against argument (1), that void (as container) and body (as contained) are correlatives, he claims that “vessel of a body” (ἀγγεῖον σώματος) may be taken in two ways: as ‘the container of the contained’ (in which case, we may add, we are indeed dealing with correlatives) and as ‘that which can contain’, which may, as a matter of fact be empty.⁷⁷ He addresses the issue of infinity in a separate argument: void and the cosmic body are not only not correlatives, but the one is by its own nature (or rather: in its own conception (ἐπίνοια)) infinite and the other by its own nature finite; moreover, he claims (now arguing from a strictly Stoic point of view), there cannot exist a hexis which keeps an infinite body together (so we do have an argument against the conception of an infinite body, whereas we do not have such an argument against the conception of an infinite void).⁷⁸

Back to Alexander. Immediately after the passage just quoted he inserts a counter-argument by Xenarchus of Seleucia which is comparable to, though also slightly different from, the first half of this Stoic defence offered by Cleomedes,⁷⁹ thus showing that this kind of debate between Peripatetics and Stoics was already going on in the first century BC:

Xenarchus changed ‘that which can contain’ (τὸ οἷóν τε δέξασθαι) into ‘the container’ (τὸ δεκτικóν), thus trying to solve the problem arising for this position in virtue of the relativity 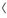of the concepts. However, this change did not really help. For the ‘container’ is nothing else but ‘that which can contain’, and as such it remains a relative (Simp. Cael. 286, 2–6).

It is unfortunate that we do not have more information about the context in which Xenarchus’ argument was embedded. It is clear, however, that by distinguishing between (a) the void as what can contain a body, in the sense that, given the nature of things, it may in fact at some point contain a body, and (b) the void as simply in its own nature capable of receiving a body, even if it never will, Xenarchus rejected Aristotle’s conception of place and body as correlatives and thus defused this particular Peripatetic argument against the Stoic position.

Paul Moraux made the stronger claim that Xenarchus even tried to defend the Chrysippean conception of an infinite extra-cosmic void.80 It is true that the formula which Xenarchus corrects in the above passage – “that which can contain” (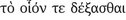) – resembles the Wortlaut of the preserved Stoic (Chrysippean) definition of place and void (which speak of τὸ οἷóν τε κατέχεσθαι 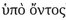),⁸¹ and has no counterpart in the ninth chapter of the first book of Aristotle’s On the Heavens. But even if we are dealing with a conscious adaptation, this does not in itself show the extent of Xenarchus’ commitment to the Chrysippean view. For all we know, he may merely have wanted to show that the arguments of contemporary Aristotelians, who based themselves on Arist. Cael. 1, 9, were unsuccessful against the Chrysippean conception of the void, in that they failed to show that such a void was inconceivable. True, his conviction that an independent extra-cosmic extension is conceivable means that he must have remained unconvinced by Aristotle’s arguments against the conception of place as extension and that it is extremely unlikely that he was committed to Aristotle’s own conception of place. But we still cannot exclude the possibility that he himself subscribed to the Stratonian conception of a finite space. After all, although other fragments do indeed show traces of Stoicizing tendencies in Xenarchus’ work,⁸² there is no reason to assume that he accepted the idea of a periodical conflagration, which for the Stoics was the physical raison d’être of the extra-cosmic void. On the other hand, he may not have needed such physical or cosmological arguments. He may have gone beyond Strato in taking the full consequences of the rejection of the correlativity of body and void, and in accepting the type of argument provided by Cleomedes, according to which space or void, once conceived as independent, is in its own nature (or in its own conception) infinite. However this may be, in the context of our present survey the interesting thing is that the evidence on Xenarchus offers us a second example, next to Strato, of a straightforwardly heterodox conception of place within the Hellenistic Peripatos.

7 Sextus Empiricus and Hellenistic discussions ofplace

We may take the evidence on Xenarchus, and the arguments of Alexander and Cleomedes as signs of a growing polemical interaction between the Peripatos and the Stoics, starting from the renaissance of Aristotelian philosophy in the first century BC, when the main texts of the corpus Aristotelicum became widely available again.83 Two aspects of this interaction seem to be noteworthy, although the fragmentary nature of our sources sets severe limits to the security of our conclusions.

First of all, the interaction appears to be late: there are no signs that the early Peripatetics – Eudemus, Theophrastus, Strato – reacted to the Stoic conceptions of place, space or void. This is not surprising. When Strato became scholarch in the early eighties of the third century, Chrysippus was still to be born. And it was arguably Chrysippus who worked out the Stoic theory of space in all its details.84 Conversely, there are no signs that Chrysippus knew or reacted to Aristotle’ theory of place, and in general the evidence suggests that by the mid third century Aristotle’s physical works were no longer widely known and studied. Chrysippus appears to have been a lively polemicist, but he appears to have directed his arrows primarily at what was by now becoming the most important rival school: the Garden.⁸⁵

Secondly, it also appears that the interaction was rather one-sided. We have evidence of Peripatetic attacks of the Stoic position in the fragments of Xenarchus and Alexander, and in the treatise of Cleomedes. On the Stoic side we only find Cleomedes’ reaction to these attacks; no evidence of original anti-Aristotelian polemics. Of course this general picture may in principle be merely an artefact of the lacunose transmission of the primary evidence. But it may also have something to do with the fact that the Aristotelians now had texts like the Physics and the De Caelo at their disposal, in which Aristotle himself had argued against the most important rival conception of place as an independent extension, whereas the Stoics could find no similar anti-Aristotelian ammunition in the works of their own founding fathers.

This general picture appears to be confirmed by the evidence from Sextus Empiricus. In the partly parallel chapters devoted to the subject of place in PH 3, 119–135 and M 10, 1–36 he presents arguments against the two main conceptions of place that were around at the time: the Stoic view of place (topos) as a three-dimensional container (hypodektikos), and the Aristotelian view of place as a surrounding surface (periektikos).⁸⁶ One might perhaps expect him to use Stoic arguments against the Peripatetics and vice versa, but this is not what we encounter. His proximate source or sources are probably collections of arguments put together by earlier sceptics: at M 10, 20 the anti-Stoic arguments are introduced as “coming from the sceptical tradition” ( ). But his ultimate sources for both the critique of the Stoic conception and the critique of its Aristotelian counterpart appear to belong to the Aristotelian tradition.

In the account of PH 3 we find the arguments against the Stoic conception of place as a three-dimensional container at PH 3, 124–130. They revolve around the alleged impossibility of conceiving a diastēma – the central term in the Stoic definitions of spatial concepts – independent of the dimensions of the emplaced body: “how do they conceive of it as an extension?” (πῶς καὶ λέγουσιν αὐτὸν εἶναι διάστημα, 3,125). Three arguments are given.

According to the first argument (3, 125–126), the word diastēma (‘extension’) must refer either to just one dimension or to the three of them (αἱ τρεῖς διαστάσεις). In the former case place will not be equal to the emplaced body; moreover, place will, absurdly, be part of the emplaced. In the latter case we must conclude that, since there is no void space available in the place at issue (for qua place it is occupied) nor any body other than the body occupying the place, there is only the emplaced body with its three dimensions (and additional ‘resistance’ or antitypia). This means that the body (the only three-dimensionally extended entity available) will be its own place. This argument of course ignores the fact that for the Stoics “that which is capable of being occupied by body” (τὸ οἷóν 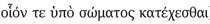) – i. e. the formula which constitutes the common denominator of topos, chōra and kenon – was conceived as independent of the emplaced body.⁸⁷

The second argument (3, 127–128) is based on the assumption that the dimensions cannot be twofold (ἐπεὶ διπλαῖ αἱ διαστάσεις οὐ θεωροῦνται), i.e. that we cannot have two overlapping ‘sets’ of three dimensions. As a result, either the dimensions of the body or those of place or the void (with which place is here identified for the sake of the argument) will turn out to be non-existent, or else the two will coincide, and body will be void. Both conclusions are absurd, so there is no separate dimension of place (τóπου διάστασις), hence no place.

The third argument (3, 129) starts out from the definition of the void as “that which can receive body”, arguing that on the approach of body void must either remain (in which case void and plenum are the same), or move away, or perish (in the latter two cases void will be body, for the capacities to move and to perish are typical of bodies). All three possibilities are said to be untenable, although we may note that the Stoics would have no problem with opting for the first possibility. For precisely qua “that which can receive body”, the void will remain what it is, also when occupied by body, although it will no longer be labelled ‘void’.

These criticisms of the Stoic position are presented as traditional, or at least as shared by others.⁸⁸ As noted above, the sceptical tradition may have been their proximate source. Ultimately, however, they appear to be of Peripatetic origin. In the end they all revolve around the supposed inconceivability of an independent three-dimensional diastēma. In this respect they appear to go back to Aristotle’s discussion and rejection of the conception of place as a three-dimensional extension in Phys. 4. In Sextus’ first two arguments we find the traces of Aristotle’s argument that “what is in between a place is whatever body it may be, but not the extension of a body” (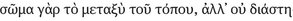 - 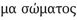, Phys. 4, 212b26–27), and of his assumption that “if there are two such things, why shouldn’t there be an infinity of them in the same spot?” (καὶ εἰ δύο τοιαῦτα, διὰ τί οὐ καὶ ὁποσαοῦν ἐν 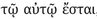, 216b10 – 11), so that “there will be many places together” (πολλοὶ τóποι ἔσονται, 211b24). But also the third argument provided by Sextus, claiming that the void should either remain, or move away or perish at the approach of a body can to some extent be traced back to Aristotle: at Phys. 4, 216a26 ff. the option of a moving void is rejected whereas the option of a remaining void runs up against the difficulty of how to distinguish its dimensions from the dimensions of the occupying body.

The anti-Stoic arguments offered in the parallel section in M 10, 19–23 are comparable in nature and need not detain us further here. Instead, we may turn to the anti-Peripatetic arguments as offered in PH 3, 131–133 and M 10, 24–36. It is striking that, whereas the arguments against the Stoics are written from a non-Stoic perspective, i. e. from the point of view of an Aristotelian ontology, the anti-Peripatetic arguments focus on difficulties within Aristotle’s theory itself. Indeed we encounter three of the difficulties that had been identified by Eudemus and Theophrastus.

We may note, to begin with, that the arguments against the Peripatetic conception of place offered at PH 3, 131–133 revolve around the fact that this place is ontologically dependent on there being both an emplaced body and a surrounding body, which means that such a place cannot be conceived of as pre-existing, and hence can play no role in the description of locomotion. A first argument (quoted above, in section 3 of this paper) claims that, when conceived in advance as the final destination of a particular moving body, such a place would necessarily both be (i. e. as a pre-existent place awaiting the body which is to fill it) and not be (i. e. because it does not exist as a surrounding surface prior to the advent of the body) at the same time. A second argument claims that if such a place exists, it must be either created or uncreated; yet both possibilities turn out to be inconceivable, given the specific requirement that place should somehow be moulded around the emplaced body. Hence it cannot exist. As suggested in section 3 of this paper, this problem was already signalled by Eudemus.

Furthermore, M 10, 24–36 offers us two other arguments that appear to go back to the discussions within the early Peripatos. At M 10, 24 Sextus claims that place as a surrounding limit would have to be either corporeal or incorporeal, but that both options lead to difficulties – the former because place would be a body and hence itself in place, the latter because place would then turn out to be a surface (ἔσται ἑκάστου 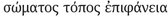). This, Sextus adds, is absurd, since a surface is not dimensionally equal to the emplaced body – an objection which appears to have been raised by Theophrastus as well, in fr. 146 FHSG. And at M 10, 35–36 Sextus points to the absurd consequence that on the Aristotelian view the heavens are not in a surrounding place –which he glosses by saying that they are, absurdly, “in themselves”, or that they are their own place. As we saw, also this problem of the emplacement of the heavens was taken up by Theophrastus in fr. 146 FHSG.

The sceptical nature of the proximate sources used by Sextus shows itself in the often typically dilemmatic surface structure of the arguments (“if place exists, it must be either corporeal or incorporeal etc.”). But the underlying philosophical arguments appear to be mainly Peripatetic in origin, and this may be taken to emphasize that the philosophical debate between Aristotelians and Stoics on place in the late Hellenistic and early Imperial period was largely determined by the Peripatetics and by their exploration of the arguments in Aristotle’s Physics and On the Heavens. The arguments directed against Aristotle’s own conception of place, on the other hand, also show that some of the problems and objections concerning Aristotle’s conception of place, as they had been conceived within the early Hellenistic Peripatos, were still around in the first two centuries AD, presumably also within the Aristotelian tradition itself, and that they had as yet not lost their force.

8 Conclusions

There is clearly a lot that we do not know about the early Hellenistic discussions of Aristotle’s theory of place: to what extent precisely was Theophrastus committed to Aristotle’s theory and how seriously did he think his aporiai compromised Aristotle’s position? What were Strato’s reasons for rejecting Aristotle’s theory? However, the evidence does provide us with sufficient indications, first, that Aristotle’s theory was thought to contain some serious weaknesses; secondly, that Eudemus (to a lesser extent) and Theophrastus (to a greater extent) were responsive to these weaknesses; and thirdly, that Strato and Xenarchus defended a different theory altogether. I do not think that Morison’s ingenious interpretation of Aristotle and his equally ingenious new interpretation of the evidence on Theophrastus give us strong reasons to change this picture of the early Peripatetic reception of Aristotle’s theory. In fact, for all we can see, Morison’s interpretation finds no support in the (admittedly: scanty) evidence on Aristotle’s earliest followers.

These early discussions influenced the later tradition in so far as they were transmitted by people like Alexander of Aphrodisias and taken up again in the large commentaries of Simplicius and Philoponus. There are two strands visible within this tradition. The orthodox followers of Aristotle such as Eudemus and Alexander, and the sources of the anti-Stoic arguments in Cleomedes and Sextus accepted Aristotle’s ontological claim that there is only one type of extension in the physical world, viz. the extension of substances, and they stuck to he principle of the correlativity of body and place. In doing so the later Hellenistic Peripatetics came up with arguments against the Stoic conception of place and void which were ineffective in so far as they disregarded the fact that the Stoics were committed to a different ontology which allowed them to posit space as a self-subsistent incorporeal and to deny the correlativity of body and void. At the same time these orthodox Peripatetics had to discuss, and find their way around, the kind of problems inherent in Aristotle’s theory of place that had been signalled by Eudemus and Theophrastus. We find traces of both their anti-Stoic attacks and their internal discussion of Aristotle’s theory in Sextus’ sceptical discussion of place in PH 3 and M 10.

The other strand – the minority one – starts with Strato, continues with Xenarchus and may arguably be said to lead up to Philoponus’ conception of space as defended in his Corollary on Place. These philosophers had to posit the ontological independence of place or space, as Strato explicitly did, and to reject the correlativity of body and place, as was explicitly done by Xenarchus in his defence of the possibility of an extra-cosmic void. As a physical theory of place this theory was much more effective than its orthodox Aristotelian counterpart and it managed to steer free of all the problems raised for Aristotle’s theory by Theophrastus and others. The drawback was that for Aristotelians it remained unclear what then the ontological status of this supposedly absolute space had to be. Philoponus makes clear that, faced with this dilemma, we have to opt for the physically preferable option and be prepared to revise our ontology in any way that is necessary. But the majority of ancient Aristotelians made the opposite choice, sticking to the Aristotelian substance ontology and being thus prepared to live with a physically less than perfect theory of place.