If absolute value sounds familiar, but you can’t quite remember it, this chapter can help. It provides a comprehensive review of the math terms and rules you haven’t had to think about since high school.
Before we get started talking about the Quantitative section of the GMAT, let’s take a moment to talk about the Integrated Reasoning section. The Integrated Reasoning section tests a blend of math and critical reasoning (verbal) skills. However, some questions test math skills. The good news is that those math skills are the same math skills that the GMAT has been testing for years. So, while we’ll be mostly discussing the Quantitative section in this and the following chapters, you’ll want to remember that you’ll also use the math reviewed in these chapters to answer some of the Integrated Reasoning questions.
The 31 math questions on the GMAT come in two different formats. About half of the questions are regular Problem Solving questions of the type you’re familiar with from countless other standardized tests, such as the SAT. The other half of the questions, mixed in among the regular Problem Solving questions, are of a type unique to the GMAT: they’re called Data Sufficiency questions, and they ask you to determine whether you can answer a math question based on two pieces of information. We’ve devoted two entire chapters to Data Sufficiency.
But whether the question falls into the category of Problem Solving or Data Sufficiency, GMAT questions test your general knowledge of three subjects:
Arithmetic
Basic algebra
Basic geometry
The good news is that you won’t need to know calculus, trigonometry, or any complicated geometry. The bad news is that the specialized, business-type math you’re probably good at isn’t tested, either. There will be no questions on computing the profit on three ticks of a particular bond sale, no questions about amortizing a loan, no need to calculate the bottom line of a small business.
For the most part, what you’ll find on the GMAT is a kind of math that you haven’t had to think about in years: junior high school and high school math. Because most people who apply to business school have been out of college for several years, high school math may seem a bit like ancient history. In the next few chapters, we’ll give you a fast review of the important concepts, and we’ll show you some powerful techniques for cracking the system.
Because it’s probably been a long time since you’ve needed to reduce fractions or remember how many degrees there are in a quadrilateral, the first thing to do is review the information tested on the GMAT by going through our math review. Along the way, you’ll learn some valuable test-taking skills that will allow you to take advantage of some of the inherent weaknesses of standardized testing.
When you’ve finished the math review, you should read our chapter on Data Sufficiency and then take our diagnostic math test. Based on your approximate score on our diagnostic, you can then practice working through problems at, or just above, your scoring range. By becoming familiar with the general level of difficulty of these problems and the number of steps required to solve them, you can increase your score on the real GMAT.
Although we can show you which mathematical principles are most important for the GMAT, this book cannot take the place of a basic foundation in math. We find that most people, even if they don’t remember much of high school math, pick it up again quickly. Our drills and examples will refresh your memory if you’ve gotten rusty, but if you have serious difficulties with the following chapters, you should consider a more thorough review, like Math Workout for the GMAT, from The Princeton Review. This book will enable you to see where you need the most work. Always keep in mind, though, that if your purpose is to raise your GMAT score, it’s a waste of time to learn math that won’t be tested.
One form of extra help you won’t be allowed during the Quantitative section of the GMAT is a calculator. (For the Integrated Reasoning section, there’s an onscreen calculator.) All calculations for the Quant section must be done the old fashioned way—by hand. To get used to this, you should retire your calculator (especially during practice tests) until after you have finished with your real GMAT.
Try the following problem:
How many even integers are between 17 and 27 ?
 9
9
 7
7
 5
5
 4
4
 3
3
This is an easy GMAT question. Even so, if you don’t know what an integer is, the question is impossible to answer. Before moving on to arithmetic, you should make sure you’re familiar with some basic terms and concepts. This material isn’t difficult, but you must know it cold. (The answer, by the way, is (C).)
Integers are the numbers we think of when we think of numbers. Integers are sometimes called whole or natural numbers. They can be negative or positive. They do not include fractions. The positive integers are:
1, 2, 3, 4, 5, etc.
The negative integers are:
–1, –2, –3, –4, –5, etc.
Zero (0) is also an integer. It is the only number that is neither positive nor negative. Sometimes the GMAT will reference a number that is not positive, or non-negative. That is another way to refer to zero as a potential part of a question.
Positive integers get greater as they move away from 0; negative integers get lesser. Look at this number line:
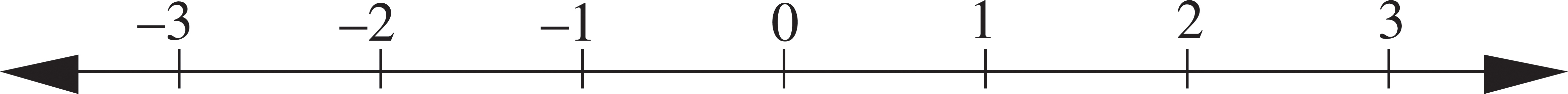
2 is greater than 1, but –2 is less than –1.
Positive numbers lie to the right of zero on the number line. Negative numbers lie to the left of zero on the number line.
There are three rules regarding the multiplication of positive and negative numbers:
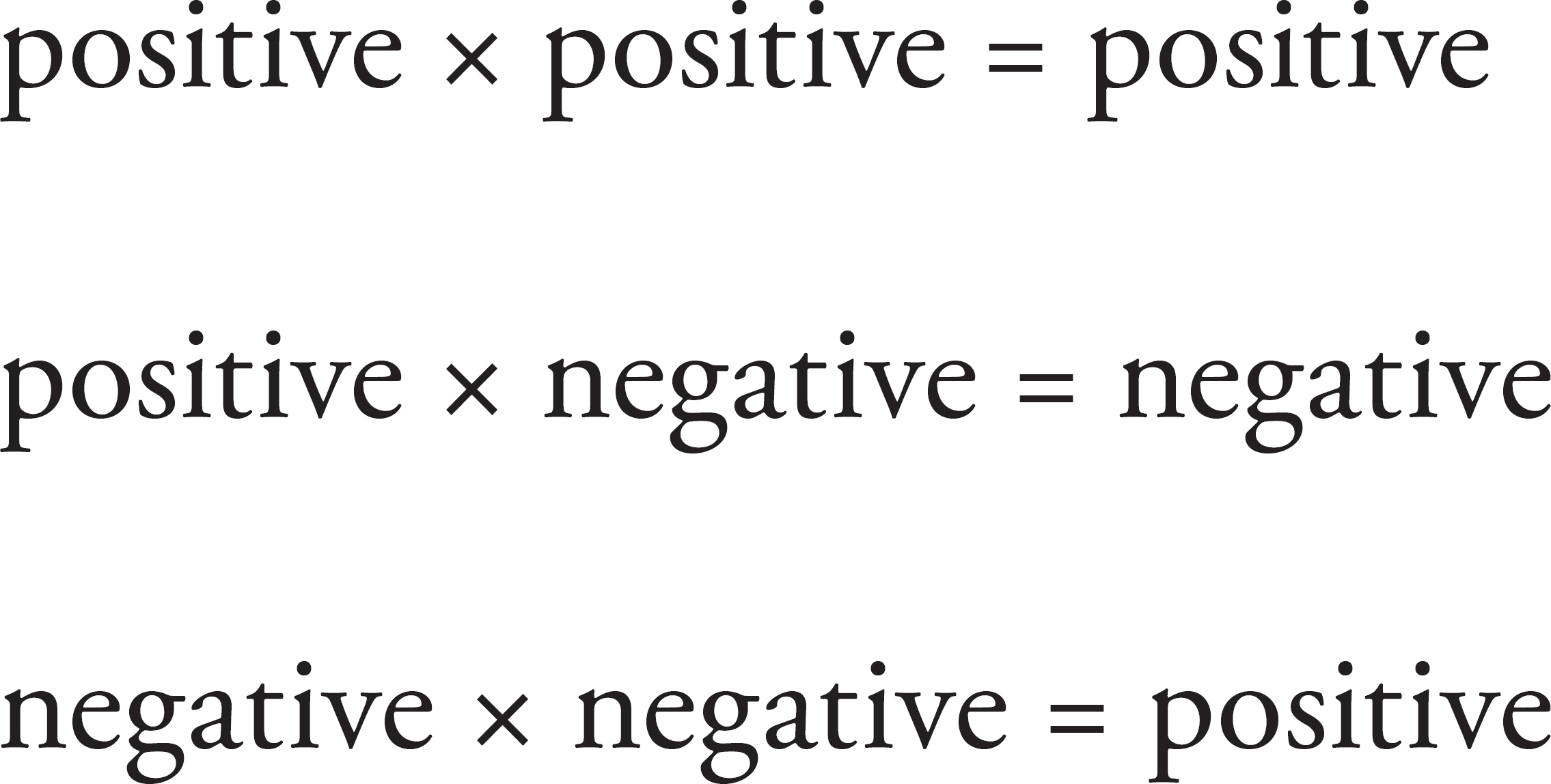
If you add a positive number and a negative number, subtract the number with the negative sign in front of it from the positive number.
4 + (–3) = 1
If you add two negative numbers, you add them as if they were positive, then put a negative sign in front of the sum.
–3 + –5 = –8
There are ten digits:
0, 1, 2, 3, 4, 5, 6, 7, 8, 9
All integers are made up of digits. In the integer 246, there are three digits: 2, 4, and 6. Each of the digits has a different name:
6 is called the units (or ones) digit.
4 is called the tens digit.
2 is called the hundreds digit.
A number with decimal places is also composed of digits, although it is not an integer. In the decimal 27.63, there are four digits:
2 is the tens digit.
7 is the units digit.
6 is the tenths digit.
3 is the hundredths digit.
If an integer cannot be divided evenly by another integer, the integer that is left over at the end of division is called the remainder. Thus, remainders must be integers.

Even numbers are integers that can be divided evenly by 2, leaving no remainder. Here are some examples:
–6, –4, –2, 0, 2, 4, 6, etc.
Any integer, no matter how large, is even if its last digit is divisible by 2. Thus 777,772 is even.
Odd numbers are integers that cannot be divided evenly by 2. Put another way, odd integers have a remainder of 1 when they are divided by 2. Here are some examples:
–5, –3, –1, 1, 3, 5, etc.
Any integer, no matter how large, is odd if its last digit is not divisible by 2. Thus 222,227 is odd.
There are several rules that always hold true for even and odd numbers:
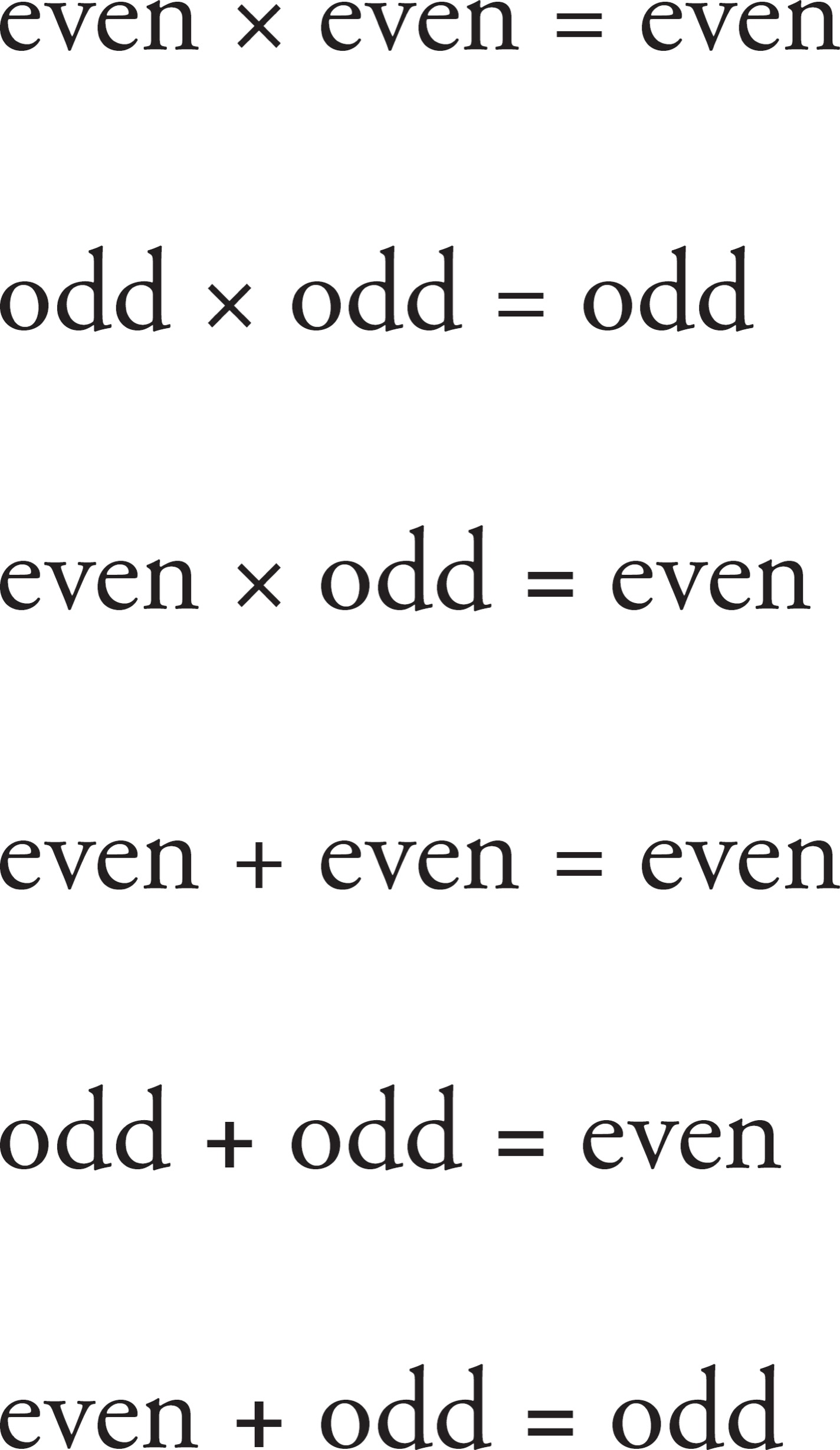
It isn’t necessary to memorize these, but you must know that the relationships always hold true. The individual rules can be derived in a second. If you need to know even × even, just try 2 × 2. The answer in this case is even, as even × even always will be.
Consecutive integers are integers listed in order of increasing value without any integers missing in between. For example, –3, –2, –1, 0, 1, 2, 3 are consecutive integers. Only integers can be consecutive.
Some consecutive even integers: –2, 0, 2, 4, 6, 8, etc.
Some consecutive odd integers: –3, –1, 1, 3, 5, etc.
If two numbers are distinct, they cannot be equal. For example, if x and y are distinct, then they must have different values.
A prime number is a positive integer that is divisible only by two numbers: itself and 1. Thus 2, 3, 5, 7, 11, 13 are all prime numbers. The number 2 is both the least and the only even prime number. Neither 0 nor 1 is a prime number. All prime numbers are positive.
If there is no remainder when integer x is divided by integer y, then x is said to be divisible by y. Put another way, divisible means you can evenly divide the greater number by the lesser number with no remainder. For example, 10 is divisible by 5.
An integer is divisible by 2 if its units digit is divisible by 2. Thus, 772 is divisible by 2.
An integer is divisible by 3 if the sum of its digits is divisible by 3. We can instantly tell that 216 is divisible by 3, because the sum of the digits (2 + 1 + 6) is divisible by 3.
An integer is divisible by 4 if the number formed by its last two digits is divisible by 4. 3,028 is divisible by 4, because 28 is divisible by 4.
An integer is divisible by 5 if its final digit is either 0 or 5. Thus, 60, 85, and 15 are all divisible by 5.
An integer is divisible by 6 if it is divisible by both 2 and 3, the factors of 6. Thus, 318 is divisible by 6 because it is even, and the sum of 3 + 1 + 8 is divisible by 3.
Division by zero is undefined. The test writers won’t ever put a zero in the denominator. If you’re working out a problem and you find yourself with a zero in the denominator of a fraction, you’ve done something wrong. By the way, a 0 in the numerator is fine. Any fraction with a 0 on the top is 0.

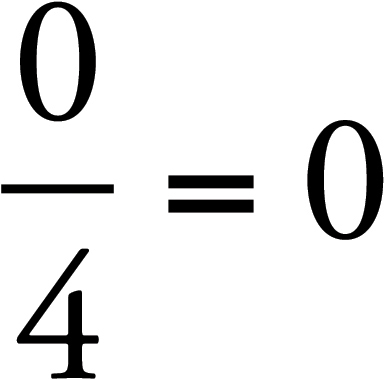

An integer, x, is a factor of another integer, y, if y is divisible by x. So, in other words, y = nx, where y, n, and x are all integers. For example, 3 is a factor of 15 because 15 = (3)(5). All the factors of 15 are 1, 3, 5, and 15.
The multiples of an integer, y, are all numbers 0, ±1y, ±2y, ±3y…, etc. For example, 15 is a multiple of 3 (3 × 5); 12 is also a multiple of 3 (3 × 4). When you think about it, most numbers have only a few factors, but an infinite number of multiples. The memory device you may have learned in school is “factors are few; multiples are many.”
Every integer greater than 1 is both its own greatest factor and least positive multiple.
If an integer, x, is divisible by two integers, n and m, then x is a common multiple of n and m. For example, 30 is a common multiple of 5 and 6. The least common multiple of two integers is called the least common multiple. For our example, 30 is also the least common multiple of 5 and 6.
The most straightforward way to find a least common multiple is to simply start listing the positive multiples of both integers. When you find a number that is on both lists, that number is the least common multiple.
For example, here’s how you find the least common multiple of 4 and 6.
Multiples of 4: 4, 8, 12, 16, 20,…
Multiples of 6: 6, 12,…
Since 12 is on both lists, 12 is the least common multiple of 4 and 6.
If two integers, n and m, are both divisible by an integer, x, then x is a common factor of n and m. For example, 6 is a common factor of both 12 and 18. The greatest factor that two numbers have in common is referred to as the greatest common factor. For our example, 6 is also the greatest common factor of 12 and 18.
The most straightforward way to find a greatest common factor is to simply list the factors of both numbers. Then you just need to find the greatest number that is on both lists.
For example, here’s how you find the greatest common factor of 12 and 18.
Factors of 12: 1, 2, 3, 4, 6, 12
Factors of 18: 1, 2, 3, 6, 9, 18
Since 6 is the greatest number on both lists, 6 is the greatest common factor of 12 and 18.
If an integer, x, that is a factor of an integer, y, is also prime, then x is called a prime factor of y. For example, 3 and 5 are prime factors of 15.
To find the prime factors of an integer, use a factor tree:

All positive integers greater than 1 have unique prime factorizations, a fact that the GMAT frequently tests. So, it doesn’t matter which pair of factors you start with when you use the factor tree.

The prime factorization of 12 is 2 × 2 × 3.
The absolute value of a number is the distance between that number and 0 on the number line. The absolute value of 6 is expressed as  .
.

x and y are integers less than 60 such that x is equal to the sum of the squares of two distinct prime numbers, and y is a multiple of 17. Which of the following could be the value of x – y ?
 –19
–19
 –7
–7
 0
0
 4
4
 9
9
During the test, you’ll be able to see test instructions by clicking on the “Help” button at the bottom of the screen. However, to avoid wasting time reading these during the test, read our version of the instructions for Problem Solving questions now:
Problem Solving Directions: Solve each problem and choose the best of the answer choices provided.
Numbers: This test uses only real numbers; no imaginary numbers are used or implied.
Diagrams: All problem solving diagrams are drawn as accurately as possible UNLESS it is specifically noted that a diagram is “not drawn to scale.” All diagrams are in a plane unless stated otherwise.
Without a review of the basic terms and rules of math tested on the GMAT, you won’t be able to begin to do the problems.
Study the vocabulary and rules in the preceding chapter to get this stuff back into your head. If you find that you need an even more comprehensive review, consider getting Math Workout for the GMAT or Math Smart, both published by The Princeton Review.