The barnacle-encrusted lump had been sitting under the portico of the Athens archaeological museum for several months before anyone noticed anything unusual. A year and a half before, in October 1900, some sponge-divers from Rhodes had been blown off course by a storm and dropped anchor off the almost uninhabited island of Antikythera. Sixty metres below was a shelf that had not been recorded on any chart. When the winds had died down, they decided to look for sponges before sailing for home. The first diver had been in the water for no more than a few minutes when he tugged violently on his rope and was pulled to the surface. Evidently suffering from hallucinations caused by nitrogen narcosis, he claimed to have seen a mass of human remains. A second diver went to investigate. He, too, saw a dark mound of wreckage and then, protruding from the mound, a beautiful bronze arm.
Two thousand years earlier, in about 80 BC, a large and elderly ship carrying bronze and marble statues had sunk with all its crew and cargo. It had probably been sailing to Syracuse or Rome when the wind gods capsized it and locked up its treasures in the sea. After its rediscovery, the arm eventually found its way to Athens. An expedition was mounted and, with the help of the sponge-divers, the contents of the wreck were salvaged.
The Antikythera wreck produced so many spectacular finds – a bronze lyre, a marble bull, a philosopher’s head, a Hercules, a bowl of blue glass similar to one found at Alesia in Gaul – that no one paid much attention to the formless bits of debris that were dumped under the portico or deposited in cardboard boxes. It was not until one of the lumps fell apart that the museum director spotted something that was not only strange but, according to everything that was known about the ancient world, impossible: embedded in the calcified wood was what appeared to be a gear wheel with tiny bronze teeth less than two millimetres long. The first inspections showed a series of bronze plates enclosed in a wooden case. Since then, increasingly sophisticated techniques have been brought to bear on the object known as the Antikythera Mechanism. Recently, a few more bits and pieces were discovered in a storeroom packed into boxes labelled ‘Antikythera’, and with the help of computer-gaming software and an eight-ton X-ray machine, most of the miraculous mechanism has now been brought back to life.
It took the form of a rectangular case about the size of a small shoebox. Some of the names of months engraved on its bronze plates suggest that it was manufactured in Corinth, presumably before that city was destroyed by the Romans in 146 BC. The device was practically an antique when it was taken on board the ill-fated vessel in c. 80 BC. There were two dials on the front and one on the back. A side-crank moved pointers on the dials and allowed the user to calculate the dates of solar and lunar eclipses, the phases of the Moon, the dates of the four Panhellenic Games, and probably also the positions of the sun and the five planets, and the rising and setting of certain stars. There was a choice of two calendars – Egyptian and Metonic.18 The calculations were based on astronomical observations made by the Babylonians over the course of several centuries. It had epicyclic gearing (gears whose bearings are attached to other gears), which is otherwise unknown until the Middle Ages. This would have made it possible to multiply fractions and to replicate the apparently erratic motion of the moon across the sky. Despite the infinitesimal complexity of its workings, it would have been no more difficult to operate than an iPhone, though it must have taken some careful studying of the manual – thousands of Greek characters minutely engraved on the dials – to comprehend phrases such as ‘spiral subdivisions 235’.
The exact purpose of the Antikythera Mechanism is still unknown. It may have been a scientific toy and an elegant illustration of micro-engineering (it was designed to be easily dismantled and reassembled).
As a calendar or an almanac, it was unnecessarily but delightfully precise. It would have been the perfect gift for an astronomer who wanted to hold the workings of the heavens in his hands or a geographer who wanted to find out exactly where he was on the earth. Whatever its purpose, it seems an almost alien presence in that world of brute force and basic machinery, and this was the most startling revelation of all: no one could possibly have known from the written record that such a thing had ever existed. And yet, unless the sponge-divers had been unbelievably lucky, there was more than one hand-held computer travelling about the Mediterranean in the second and first centuries BC.
That shapeless lump of wood and the glittering microcosm it contained are a rude reminder that only fragments of ancient wisdom have survived. A scholar who enters a Classics reading room with its beautifully published texts prepared by priestly editors is witnessing an illusion: behind the orderly accumulation of knowledge is a cavernous museum of many floors, devastated by war or natural catastrophe, most of its shelves and cabinets either empty or strewn with illegible clumps of charred parchment.
Inventions and discoveries were not instantly transmitted to the rest of the world. They could lie dormant in libraries for hundreds of years and then disappear for ever when the library was burned to the ground or when a librarian replaced an old text in the belief that it was out of date. Even the existence of a scientific instrument was no guarantee that the principles of its construction would be deduced and understood. In 263 BC, in the early stages of the war on the Carthaginians, Roman legions captured the Sicilian town of Catania. Among the trophies that were carried back to Rome was a curious invention called the horologium solarium. The sun cast the shadow of an upright stick onto a flat surface marked with lines and, in this way, indicated the hours of the day. The device was set up on a column facing the Senate House in Rome. For ninety-nine years, it gave the citizens of Rome the wrong time of day until finally someone realized what people on the Mediterranean coast of Gaul had known two centuries before: that the height of the sun and the angle of its shadow vary with latitude. Unless the earth shifted on its axis, a sundial made for Catania would never be accurate four degrees further north in Rome.
Since the Middle Ages, scientific wisdom has accumulated like interest in a savings account, but in the days of the ancient Celts, whole tribes and empires vanished within a few decades or were reduced to relict populations with no means of reproduction. The Celts believed that, one day, the sky would fall in and destroy the earth. This belief reflected their experience. Their own scientists and intellectuals, the Druids, were hunted almost to extinction: their last significant appearance in Roman history is as a band of screeching, foul-mouthed fanatics on a coast in North Wales. In some periods and domains, regression was the norm, and it would not be surprising if a future historian, confused by the backward counting of years BC, reversed the chronological data in order to produce a more logical sequence.
![]()
It was almost three centuries before the Roman conquest of Gaul that one of the greatest expeditions in the ancient world took place, and over a thousand years would pass before anything similar was attempted. One day in the mid-320s, an ocean-going vessel rounded the rocky headland, passed under the beacon-tower and the temple of Artemis, and came to rest among the other ships in what is now the Vieux Port of Marseille. Massalia, founded three hundred years before, had become one of the most powerful cities in the Mediterranean. Aristotle had recently praised its enlightened oligarchy and its council of six hundred senators. Its houses stretched over the hills behind the port, where vines and olive trees had been planted. Ramparts kept out the Ligurian tribes who lurked in the forested ravines of the hinterland, but there were safe and well-travelled routes up the Rhone Valley leading to the lands of the wealthy, wine-addicted Celtic tribes. In the crowds that walked along the quays, there were Greek-speaking Celts and Gaulish-speaking Greeks. The warehouses and taverns were thronged with merchants and pirates who had seen a world many times bigger than the world of Homer’s Odyssey. But even the most imaginative and cosmopolitan Massaliot would have found it hard to match the tales of the traveller who returned that day to his native city.
The traveller’s name was Pytheas. He may have been commissioned by the senate or by a guild of merchants to prospect new trading routes, or perhaps, as a boy growing up in Massalia, he had contracted the incurable disease of curiosity. He would have read the sixth-century Periplus that described the coasts from Massalia to the Sacred Promontory, ‘where the starry light declines’, and the sea routes that led to the wintry lands under the Great Bear from where the tin and the amber came. Mediterranean city-states treated their explorers as secret agents and kept their logbooks under lock and key, but leaks were unavoidable, especially in a large port, and mariners are notoriously loquacious. Pytheas would certainly have heard of his fellow Massaliot, Euthymenes, who, in the early 500s, had sailed through the Pillars of Hercules, turned south and followed the coast for many weeks until he saw crocodiles and hippopotami swimming in the fresh water of a great river that flowed far out to sea. Down at the harbour, he would have talked to sailors who knew how to steer a steady course over long distances and whose knowledge of winds, constellations, tides and currents was only then being translated into mathematical equations.
Pytheas was conversant with the very latest developments in scientific theory. He may even have corresponded with Aristotle. He knew that the celestial pole around which all the stars revolved was an empty patch of sky that could be located from three stars in the constellations of the Little Bear and the Dragon. (There was no star at the pole in the third century BC.) Before leaving home, he set up a gnomon marked off into one hundred and twenty sections – a system which, in contradiction of the accepted chronology, suggests knowledge of the Babylonian division of the circle into 360. On the longest day of the year, the shadow cast by the noonday sun was 414/5 sections long. This indicates a latitude of 43.2° – one-tenth of a degree south of the harbour of Marseille, where the reading is supposed to have been taken. It was a remarkably precise figure and perhaps completely accurate: 43.2° is the latitude of Cap Croisette, where only the raggedy gnomons of telegraph poles spoil the view, and where there were fewer obstructions and disturbances than in Massalia. For the first time in recorded history, a man defined his position on the earth with an exact coordinate. When Pytheas stood on that windy headland on a sunny day in June measuring the length of the sun’s shadow, he had already embarked on his voyage of discovery.
He sailed through the Pillars of Hercules – or, if the Carthaginian blockade was in force, took the overland route along the rivers of Aquitanian Gaul – to the Atlantic coast. He passed through Corbilo at the mouth of the Loire. The mud of the estuary was already swallowing the port. Long before the Romans arrived, it disappeared, and nothing now remains of it, except perhaps a sandbank called the Banc de Bilho. From Corbilo, he sailed along the granite coast of a peninsula called Ouexisame in the Celtic tongue; its inhabitants were the Osismi – ‘the People at the End of the World’. The name has survived in ‘Ouessant’ (Ushant), the island off Finistère which marks the western extremity of Gaul. In February 1959, a few miles along the coast at Lampaul-Ploudalmézeau, a man who had been collecting seaweed for his vegetable patch noticed a gold coin glinting among his lettuces. It had been minted in the Mediterranean city of Cyrene in about 320 BC – a sign of how far the Greek trading empire extended, unless it came from the treasure chest of Pytheas himself. (Cyrenean gold coins would have made better bartering tokens than the drab, bronze coins of Massalia.)
North of Ouexisame, there was no sight of land for a day or two, until the stormy promontories of Belerion (Land’s End and the Lizard). This was the southernmost tip of the semi-mythical island or islands called Prettanike. Pytheas sailed along the busy coast among the coracles and canoes and larger vessels from the Atlantic lanes, to the headland at the other end of southern Britain: Kantion (Kent). According to one source, he then walked all the way through Britain. The natives lived in houses made of reed or logs, and threshed their wheat indoors because of the rainy climate. They made a beverage from honey and grain, which they drank with a dismal potage of millet, roots and herbs, having very little meat or fruit.
As he went, Pytheas calculated his latitude from the elevation of the mid-winter sun. In one place, it rose four peches (about eight degrees) above the horizon, which implies a latitude between the Peak District and the Yorkshire Dales. The next reading showed a solar elevation of only three peches (somewhere near the Moray Firth in Sutherland). Here, in the middle of a fourth-century BC winter, the history of Britain begins – not with Caesar’s summer raiding parties three hundred years later, but with the first identifiable visitor, a scientific traveller with a name, a place of birth and geographical coordinates, muddying his Mediterranean shoes on the soil of an island whose very existence was in doubt.
He reached the northernmost part of Prettanike at a place called Orka – possibly Duncansby Head, which looks over to the Orkneys. He had now gone far beyond even the imaginings of Homer. He set sail again, perhaps in a native boat. Six days out from Orka was an island called Thoule (the Faroes or Iceland), and a place where the sun kept watch all night in summer, barely rising from its bed. Further still, he came to a region that was neither land nor sea but a mixture of all the elements ‘on which one can neither walk nor sail’. In the fog banks and pack ice of the Arctic, he saw the earth in its troubled infancy or its confused old age.
He returned through the amber-rich Baltic and probably followed the river Dnieper to the Black Sea. In effect, Pytheas circumnavigated Europe. In the warmth and bustle of Massalia, or perhaps in a villa on Cap Croisette, he wrote up his journal. It was known by the title Peri tou okeanou (‘On the Ocean’), and it became one of the most famous books of the ancient world. No copy has ever been found – though some palimpsestic remnant may yet be hiding in a monastery – and one of the greatest voyages of discovery ever made is known only from brief, generally hostile references in a handful of Greek and Latin texts. The main source is the Geography of Strabo (7 BC), the geographer whose name means ‘one who can’t see straight’. The fantastic tales of that impudent Greek from southern Gaul made him burn with envy. How could Pytheas have talked to people who lived six days north of Britannia and thus beyond the limits of the habitable world? It was well known in 7 BC that nothing north of Ierne (Ireland) supported human life; Ierne itself was the home of ‘complete savages [who] lead a miserable existence because of the cold’. The exotic place names – Orka, Thoule, the Bed of the Sun – were obviously invented by Pytheas to give his incredible account an air of truth . . .
But even Strabo grudgingly admitted that there might be something to Pytheas’s scientific observations: ‘If judged by the science of the celestial phenomena and by mathematical theory, he might possibly seem to have made adequate use of the facts.’ This was, after all, the great contribution of Pytheas: whatever the official reason for his expedition, he had been collecting the priceless gems of evidence that would make it possible to construct a map of the world.
![]()
18. The Voyage of Pytheas
A scientifically produced map of the world in the fourth century BC seems as unlikely as the Antikythera Mechanism. If the warped, amoebic continents of medieval maps represent the sum of geographical wisdom, what formless fictions must have lived in the minds of the ancients? And yet, though precise measurements were the rarest of rare commodities, the principles were well established. Eighty years after the voyage of Pytheas, the chief librarian of the Library of Alexandria, Eratosthenes of Cyrene, made a momentous calculation. He had been told that at noon on the longest day of the year the sun at Syene (Aswan) shone directly down a well as though pouring its light carefully into the shaft without spilling a drop. A stick planted upright in the earth at Syene cast no shadow, whereas at Alexandria, eight hundred and fifty kilometres to the north, at exactly the same time, there was a very noticeable shadow. Given the distance between Syene and Alexandria (five thousand stadia) and the angle of the shadow to the sun’s rays (about one-fiftieth of a circle or just over seven degrees), Eratosthenes concluded that the circumference of the earth must be 250,000 stadia (5000 x 50).
This is the earliest record of a rational mind embracing the whole earth. Inevitably, Eratosthenes’ calculations were the approximate caresses of a lover who was unable to express the precision of his desire. He assumed that the earth was a perfect sphere, that Alexandria lay due north of Syene, and that Syene lay directly on the tropic (where the summer solstice sun is directly overhead at noon). It is impossible to say how close he came to the mathematical truth: the exact value of the stadion he used is unknown; the error was somewhere between two and thirty per cent of the actual figure. But the essential point was the application of theory to the cosmos: equipped with nothing but a stick, a distance measurement and simple geometry, Eratosthenes had established the basis of a world map.
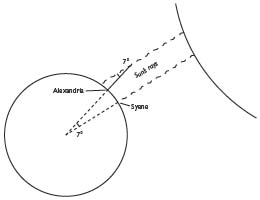
19. Eratosthenes’ experiment
Ancient accounts usually attributed discoveries to heroic individuals when in fact they probably dawned in different places and at different times. When Eratosthenes scratched the cosmic truth into the wax of a writing tablet, several vast realities of terrestrial existence were already widely known or suspected: the world was not flat, and since the sun was very large and very distant, it was mechanically absurd to assume that it orbited the earth. In the 320s BC, Pytheas knew that latitude could be calculated from the shadow of a gnomon, from the length of the longest day, or from the rising and setting of a star. When he walked through Britain, he felt the curve of the earth beneath his feet, and when he sailed on the ocean, he knew that the swelling tides were in some manner caused by the moon.
The information was available, and so were the means of fashioning it into imaginable forms. By modern standards, it was a motley collection of data. In the Library of Alexandria, Eratosthenes would have found a few precise readings from gnomons and water-clocks, astronomical observations made by Babylonian astrologers, measurements of distance provided by armies, camel-trains, the mensores (land-surveyors) of Alexander the Great, and the betamists (professional walkers) and ‘rope-stretchers’ who re-surveyed Egypt to re-establish land boundaries after each major flooding of the Nile. Ships putting in at Alexandria were searched and any scrolls found on board were confiscated; copies were made and the originals placed in the library, marked ‘from the ships’. This wily acquisitions policy must have produced a fine collection of sailors’ periploi, which listed river mouths and headlands, and gave distances in days of sailing.
Most of this geographical knowledge survives only as a muddle of rumour and misreporting in later Roman texts, but the early descriptions of the oikoumene (the inhabited earth) show that certain key coordinates had been identified – Rhodes, the Pillars of Hercules, Byzantion, Borysthenes, etc. When Pytheas sailed from Massalia, these points of reference were already being used to organize a conception of the earth that had once seemed a prerogative of the gods. Accurate depictions of the coastlines and continents were still many centuries away, but a semblance of the oikoumene could now be held within a human mind, thanks to one of the great inventions of the ancient world: the division of the terrestrial sphere into zones of latitude called klimata.
20. The oikoumene
The numbers on the left (hours and minutes) give the length of the longest day in 300 BC at that latitude. (The decimal degrees are those of the modern coordinate system.) The commonest intervals between klimata were half an hour; smaller divisions were also made. The latitude lines are derived primarily from Dicaearchus, Eratosthenes and Timosthenes. See also heren.
Determining latitude is fairly simple. Schoolchildren supplied with sticks and protractors regularly perform the exercise on sunny days. Determining longitude is far trickier and sometimes thought to have been beyond the capability of the ancient Greeks.
The erratic path of an ancient meridian or line of longitude can be seen towards the right of the map. The six places from Borysthenes (the mouth of the Dnieper) to Meroe (in the Sudan) were considered by Eratosthenes, for the sake of argument, to lie on the same north–south line. In reality, they occupy a time zone that covers almost six degrees of longitude: noon occurs at Rhodes twenty-two minutes later than at Meroe. For a sailor, the error would be catastrophic. The usual solution was ‘latitude sailing’: head north or south until the latitude of the destination is reached, then sail west or east until land is sighted. The same form of navigation was used by Columbus and Vasco da Gama almost two thousand years later. Once it became possible to calculate longitude as well as latitude, a ship could steer a direct, diagonal course along a rhumb line,19 holding to the same bearing throughout the voyage, instead of laboriously following two sides of the triangle. But with no landmarks, a surface that never stood still and no chance of repeating the experiment, it was impossible to determine longitude at sea with any degree of accuracy until the invention of a reliable marine chronometer in the late eighteenth century.
The inconveniences of ocean sailing greatly magnify the problem of longitude, which is not quite as intractable as it seems. On land, various forms of triangulation can be used to create a network of coordinates. The gridlines of Greek colonies on the Gaulish coast and parts of the Celtic system of Mediolana were, in a sense, accurate maps of miniature worlds. On a national or global scale, something more manageable was required, but according to the written record, not even a theoretical solution existed until about 150 BC. It was then that the Greek astronomer Hipparchos suggested that meridians east and west of a zero longitude (Rhodes) could be established by recording the local times at which a lunar eclipse was observed.
Here again, the sand in the hour-glass seems to flow in the wrong direction. If no means of determining longitude was devised before Hipparchos, how did Hannibal, for instance, manage to navigate his way along the Heraklean diagonal, and how was he able to rejoin the solar path after being forced to deviate from it? How could the pathways of the Celts have been anything but a wonderful mirage? A clue can be found, surprisingly, in Pliny the Elder’s credulous encyclopedia of wonders, Historia Naturalis (c. AD 78). In a chapter which is bizarre even by Pliny’s standards, he describes two ancient ‘experiments’, the purpose and result of which escaped him almost entirely. He knew only that they had something to do with the curvature of the earth, which ‘discovers and hides some things to some, and others to others’.
Someone had evidently tried to explain to Pliny the problem of longitude. The first example describes a fictitious phenomenon reminiscent of the puzzle with which brains befuddled by jetlag often have to wrestle: one of Alexander’s high-speed couriers could run 1200 stadia (about 200 kilometres) in nine hours, but only when he was heading west with the sun; the return journey took him six hours longer, despite the fact that it was downhill all the way. The other ‘experiment’ was just as confusing and equally impossible as described by Pliny. ‘High beacon-towers’ were erected by Hannibal in Africa and Spain. The beacons were lit at the sixth hour of day (noon). At the same moment, their light was seen in Asia at the third hour of night (9 pm).
This may be the only surviving indication of the kind of rapid, long-distance triangulations that enabled the Carthaginian general to follow the path of the sun. Hannibal and his astrologers performed this geodetic feat in 218 BC, perhaps with the aid of an early form of theodolite called the dioptra – a surveyor’s rod with sights at both ends through which the relative positions of the beacons could be calculated (see here). By then, the process may have been well established. A semi-instinctive form of rhumb-line sailing may have been adapted for use on land so that armies could navigate the continents like armadas.
There is even some unnoticed evidence of a longitude experiment further back in time, using astronomical rather than terrestrial measurements. In 331 BC, a lunar eclipse was observed at Arbela (Arbil, in Iraq), eleven days before the battle of Arbela at which Alexander defeated Darius III. The eclipse was recorded in at least three different places three thousand kilometres apart. All these places – Arbela, Syracuse and Carthage – lie in the same klima or latitude zone, as do two of the scientific capitals of the ancient world – Rhodes and Athens (fig. 20). Rhodes had recently become part of Alexander’s empire. Like Hannibal, Alexander had an urgent need for accurate maps and global positioning techniques, and perhaps it was at Rhodes that his geometers and surveyors coordinated the world’s first international scientific experiment.
Ultimately, the precision of these measurements depended on time-keeping. Most ancient records of eclipses give the times to the nearest hour or half hour. Since sunlight passes over the Mediterranean at about twenty-two kilometres a minute, the margin of error is enormous. Timing to the nearest minute was practically impossible, which is why ancient Greek and Latin have no word for ‘minute’. The material evidence is barely enough to fill a small cardboard box. Fragments of four water-clocks have survived from Egypt and Greece. They appear to have been capable of measuring time to within ten minutes in a twelve-hour period, though more refined readings were apparently attainable: some of the day lengths reported by Pliny include thirds, fifths and ninths of an hour, and even, in one case, a thirtieth (two minutes). This would still have produced a very erratic meridian. The great advance in time-keeping came much later, in eleventh-century Spain, when an Arab engineer invented a water-clock with epicyclic gearing (here). But historical chronologies are changing all the time. As we now know, the same technology was already performing its clockwork miracles in the second century BC. Someone on board a ship sailing west in the Aegean may have known exactly where he was before Poseidon reached up and confiscated the magical mechanism near the island of Antikythera.
![]()
The measuring and mapping of the world coincided with the first great age of European exploration. Discoverers of distant places were usually said to have been blown off course and to have been led by the gods to lands ruled by monsters or by women of unimaginable beauty and sexual appetite. But some of those supposedly accidental voyages were so long and successful that the adventurers must have been prepared, both materially and scientifically. When Eudoxus of Kyzikos set off to reach India by circumnavigating Africa in the late second century BC, he had a well-fed, motivated crew which included doctors, craftsmen and ‘flute girls’.
Not all those navigators arrived on uncultivated shores inhabited by Stone Age tribes. In the traditional European view of exploration, the bold adventurer is always more intelligent than the natives. While this was certainly true in the case of Hanno the Carthaginian, who reached equatorial West Africa in the early fifth century BC and captured three wild and hairy ‘women’ of the ‘Gorillai’ tribe, there is no reason to suppose that it was always the case. The people of Belerion on the south coast of England had been civilized by their contact with foreigners, and Pytheas was able to take his latitude readings throughout Britain without suffering the fate of some eighteenth-century French cartographers who were savagely attacked by suspicious natives. Pytheas’s measurements of the elevation of the mid-winter sun are revealing in more than one respect. In order to take his readings at locations several degrees of latitude apart, he would have had to remain in Britain for over a year, and quite possibly several years, if the modest British sun failed to show itself in late December. Perhaps he really did endure two British winters, or perhaps the land of astronomically aligned stone temples was able to provide him with the information.
When the Gaulish tribes began to bisect their domains with parallels and meridians, the world was already shedding its mythical aura. The fabled Isles of the Blessed, the Elysium of demi-gods and heroes, were increasingly associated with real islands thought to lie somewhere in the Atlantic, a long way west of the inhabited world. There were sailors who claimed to have seen them: perhaps they had sighted or made landfall on the Canaries, Madeira or Cape Verde.
With the Greek klimata as a guide, it is not hard to mount a virtual expedition, and the directions are easy to follow. Logically, to reach the Elysian Fields, one would sail due west on the latitude of Delphi, the omphalos of the earth, and hold to this bearing, riding the rays of the setting sun, to the place where Zeus released one of the two eagles or crows that met at Delphi. After ten thousand stadia (the distance given to the Roman general Sertorius by some Iberian mariners), the ship would come to a group of islands in the mid-Atlantic three and a half hours of daylight west of Delphi. In 1749, on the island of Corvo in the Azores, a rainstorm washed a black pot out of the foundations of a house. It contained a hoard of ancient coins. The coins have disappeared, and the finding will never be authenticated. But some of them were sent to Lisbon, and drawings were published in a scholarly journal in 1778. Most of the coins were clearly Carthaginian; two others came from the Greek city of Cyrene. They were dated to the late third century BC. The fact that the coins were discovered on the island furthest from Europe was thought to be particularly incredible, but an expedition to the edge of the world would hardly have sailed for home when there was still land to the west.
![]()
As migrants, traders and mercenaries, the Celts, too, belonged to this age of exploration. It was only later that a shadow fell over the shrinking world: when the Romans landed in Britain in 55 BC, in the region called Cantium, there was no trace of Pytheas’s expedition six generations before. ‘Persistent enquiries’ by Caesar produced little useful information and a good deal of misinformation. Tin, he was assured by a local informant, ‘is found in the midland regions’ (‘in mediterraneis regionibus’), whereas Pytheas, along with countless Carthaginian, Greek and Celtic traders, had known that it came from Belerion in the south-west. Caesar asked about the islands where night was said to last for thirty days at the time of the winter solstice, but no one was able to confirm their existence. ‘Accurate measurements’ with water-clocks established the fact that the days were longer than in Gaul, which was hardly an original finding, and a spell of unusually good weather – or a misreading of Pytheas’s account of the effects of the Gulf Stream – produced the surprising observation that ‘the climate is more temperate than in Gaul’.
21. Caesar’s movements in Gaul and Britain, 58–51 BC
In Gaul and in Britain, Caesar was entirely dependent on local information, which is why it took him so long to discover the most convenient crossing of the Channel. At any given moment, his mental horizons were those of the visible world. His descriptions of particular sites are accurate enough for his battles to be re-enacted and his tactics analysed, but otherwise, his vision was as foggy as the Oceanus Britannicus. To reach Gaul from the Alps at the start of each campaigning season, he usually travelled in a north-westerly direction, which would have enabled him to believe that his skewed conception (shown in fig. 22) was more or less correct. Luck shielded him from the effects of ignorance. In the seventh year of the war, he decided not to return to the Roman part of Gaul, in part because of ‘the difficult roads leading over the obstacle of the Cévennes’. Any trader could have told him that, from his camp near the river Allier, there was no need to cross the Cévennes. Instead, believing himself to be cut off from Italy, he marched towards the Rhine and set the scene for the final defeat of the Gauls.
22. Caesar’s conception of the Celtic lands
Based on De Bello Gallico (58–51 BC).
One hundred and fifty years after Caesar, in AD 98, the Roman historian Tacitus decided to describe the geography of Britain using ‘ascertained fact’ instead of guesswork. The fleet commanded by his father-in-law, Agricola, had circumnavigated the island, and he was able to describe it as a double-headed axe topped with a huge and shapeless tract of land (Caledonia) tapering to a wedge. To the east, the coast ran parallel to Germania. To the west, if one crossed the island of Hibernia (Ireland), one came to Hispania (Spain), which explained why the Silures of South Wales had ‘swarthy complexions and curly hair’. Tacitus had often heard Agricola say that he could have conquered Ireland with a single legion and trapped the rebellious Britons between Spain and Gaul. But Ireland was never invaded, and the history of exploration was deprived of one of its finest tableaux – a Roman general standing on the Dingle Peninsula, peering at the Atlantic horizon in the hope of catching sight of the Iberian coast.
In the Highlands of Scotland, ‘where earth and nature end’, Agricola is supposed to have said to his troops, ‘We do not have our enemy’s knowledge of the country.’ This would have been something of an understatement. A more accurate assessment of the Roman position is provided, in Tacitus’s account, by the British leader, Calgacus, pointing at the Romans: ‘a scanty band, dismayed by their ignorance, staring blankly at the unfamiliar sky, sea and forests around them.’
23. Strabo’s conception of the Celtic lands
Based on Strabo’s Geography (c. 7 BC). Massilia was the Roman name of Massalia (Marseille).
Long before Agricola imagined himself reaching Spain by way of Ireland, traders, fishermen and adventurers had been sailing the unmapped seas, following the coasts of Spain and Gaul or, by observing the stars, the flight of birds and clouds rising over warm land, steering a diagonal course from northern Spain across the Bay of Biscay. They rounded the Armorican Peninsula (Brittany) and put in at international ports on the south coast of Britain – Mount Batten in Plymouth Sound, Poole Harbour and Hengistbury Head in Dorset. Before any Roman had set foot in Britain, the great navy of the Celtic Veneti tribe, ‘whose knowledge and experience of all things nautical are second to none’, according to Caesar, patrolled the Atlantic seaboard in their high-prowed ships with stretched-skin sails. They levied a tax on all who sailed in their territorial waters, which implies not only registration procedures and written records but also accurate knowledge of tides, currents and sea lanes.
The information was still there, but it was locked away in separate disciplines and professions, where it might as well have existed in different dimensions. A Roman surveyor in Agricola’s army would scarcely have recognized the mental geography of his commander. No trader who had followed the rivers of Gaul would have believed, as Strabo did, that the Seine had its source in the Alps. Maps did exist: Tacitus, Pliny and Strabo were obviously thinking of particular maps when they described Britain as ‘a double-headed axe’, Italy as ‘an oak-leaf’ and Spain as ‘an ox-hide’, but they were describing the maps themselves rather than the reality they depicted, as though expecting the painting of a pudding to serve as a recipe.
The essential problem was practical rather than theoretical. In order to obtain accurate working measurements using the technology of his time, Eratosthenes would have had to assemble a team of intelligent, well-educated observers and station them at more or less regular intervals over a large part of the world. The observers would have been thoroughly trained in the sciences of measuring, surveying and astronomy. They would have had at their disposal a reliable communications system for transmitting the data. So that their results could be cross-checked and coordinated, they would have remained in position like parish priests for many years. The map-making expeditions of the Cassini family in the eighteenth century used ninety-five geometers to cover most of the area of modern France, but they had telescopes, magnetic compasses and sextants: at the very least, an expedition which lacked such technological refinements would have had one fixed observer for each triangulation point.
Ideally, the scientific body that collated the results would have had access to older, precisely dated records of eclipses. The organization would have been centralized or able to meet at regular intervals in a central location. It would have adhered to a strict code of scientific conduct that prevented it from allowing the temporary prestige of a tribe or a city from influencing the eternal truth of the survey. It would have operated in a period of relative political stability, or been able to call upon military protection. Above all, unlike the Cassini expeditions, it would have enjoyed an uninterrupted source of funding. With all this, a wonderfully accurate map of vast regions might have been created. For someone in Eratosthenes’ position, such magnificent efficiency would have been the dream of a madman.