The rules of trigonometry tested on the Math Level 1 Subject Test are much more limited than those tested on the Math Level 2 Subject Test. Trigonometry on the Math Level 1 Subject Test is confined to right triangles and the most basic relationships between the sine, cosine, and tangent functions. If you’re taking the Math Level 1, that’s the only material from this chapter you need to know. If you plan to take the Math Level 2, then this entire chapter is your domain; rule it wisely.
Here are some trigonometric terms that appear on the Math Subject Tests. Make sure you’re familiar with them. If the meaning of any of these vocabulary words keeps slipping your mind, add that word to your flash cards.
The basis of trigonometry is the relationship between the parts of a right triangle. When you know the measure of one of the acute angles in a right triangle, you know all the angles in that triangle. For example, if you know that a right triangle contains a 20° angle, then you know all three angles—the right triangle must have a 90° angle, and because there are 180° in a triangle, the third angle must measure 70°. You don’t know the lengths in the triangle, but you know its shape and its proportions.
A right triangle that contains a 20° angle can have only one shape, though it can be any size. The same is true for a right triangle containing any other acute angle. That’s the fundamental idea of trigonometry. Once you know the measure of an acute angle in a right triangle, you know that triangle’s proportions.
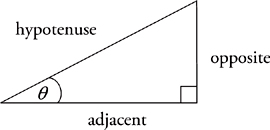
The three basic functions in trigonometry—the sine, cosine, and tangent—are ways of expressing proportions in a right triangle (that’s the ratio of one side to another). They may sound familiar to you. Or maybe you’ve heard of a little phrase called SOHCAHTOA?
Let’s break it down.
The sine of an angle is the ratio of the opposite side to the hypotenuse. The sine function of an angle θ is abbreviated sin θ.
The cosine of an angle is the ratio of the adjacent side to the hypotenuse. The cosine function of an angle θ is abbreviated cos θ.
The tangent of an angle is the ratio of the opposite side to the adjacent side. The tangent function of an angle θ is abbreviated tan θ.
These three functions form the basis of everything else in trigonometry. All of the more complicated functions and rules in trigonometry can be derived from the information contained in SOHCAHTOA.
Tables of sine, cosine, and tangent values are programmed into your calculator—that’s what the “sin,” “cos,” and “tan” keys do.
• If you press one of the three trigonometric function keys and then enter an angle measure, your calculator will give you the function (sine, cosine, or tangent) of that angle. Just make sure that your calculator is in degree mode. This operation is written:
sin 30° = 0.5 cos 30° = 0.866 tan 30° = 0.577
• Your calculator can also take a trig function value and tell you what angle would produce that value. Press the “2nd” key, then press “sin,” “cos,” or “tan,” then enter the decimal or fraction you’re given, and your calculator will give you the measure of that angle. This is called taking an inverse function, and it’s written: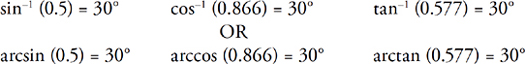
The expressions “sin−1 (0.5)” and “arcsin (0.5)” have the same meaning. Both mean “the angle whose sine is 0.5.” While ordinary trig functions take angle measures and output ratios, inverse trig functions take ratios and produce the corresponding angle measures; they work in reverse.
On the Math Level 1 Subject Test, the three basic trigonometric functions always occur in right triangles—particularly the Pythagorean triplets from Chapter 5.
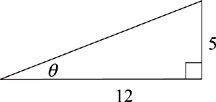
Use the definitions of the sine, cosine, and tangent to fill in the requested quantities in the following triangles. The answers to these drills can be found in Chapter 12.
1. sin θ = _____________
cos θ = _____________
tan θ = _____________
2. sin θ = _____________
cos θ = _____________
tan θ = _____________
3. sin θ = _____________
cos θ = _____________
tan θ = _____________
4. sin θ = _____________
cos θ = _____________
tan θ = _____________
The preceding examples have all involved figuring out the values of trigonometric functions from lengths in a right triangle. Slightly more difficult trigonometry questions may require you to go the other way and figure out lengths or measures of angles using trigonometry. For example:
x = _____________
Because we’re dealing with the hypotenuse and the side that is opposite the angle, the best definition to use is sine.
sin =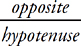 
sin 35° = 
5(sin 35°) = x
5(0.5736) = x
2.8679 = x
BC of ∆ABC therefore has a length of 2.87.
You can use a similar technique to find the measure of an unknown angle in a right triangle. For example:
x = ________________________
In triangle DEF, you know DF and DF. EF is the side that is opposite the angle we’re looking for, and DF is the side that is adjacent to that same angle. So the best definition to use is tangent.
tan =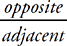 
tan x = 
tan x = 
tan x = 0.5
To solve for x, take the inverse tangent of both sides of the equation. On the left side, that just gives you x. The result is the angle whose tangent is 0.5.
tan−1 (tan x) = tan−1(0.5)
x = 26.57°
The measure of ∠D is therefore 26.57°.
Use the techniques you’ve just reviewed to complete the following triangles. The answers to these drills can be found in Chapter 12.
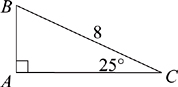
1. AB = _____________
CA = _____________
∠B = _____________
2. EF = _____________
FD = _____________
∠D = _____________
3. HJ = _____________
JK = _____________
∠J = _____________
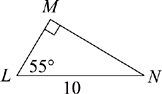
4. LM = _____________
MN = _____________
∠N = _____________
5. TR = _____________
∠S = _____________
∠T = _____________
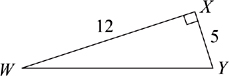
6. YW = _____________
∠W = _____________
∠Y = _____________
Some Math Subject Test questions will ask you to do algebra with trigonometric functions. These questions usually involve using the SOHCAHTOA definitions of sine, cosine, and tangent. Often, the way to simplify equations that are mostly made up of trigonometric functions is to express the functions as follows:
Writing trig functions this way can simplify trig equations, as the following example shows:
Working with trig functions this way lets you simplify expressions. The equation above is actually a commonly used trigonometric identity. You should memorize this, as it can often be used to simplify equations.
= tan x
Here’s the breakdown of another frequently used trigonometric identity:
That last step may seem a little baffling, but it’s really simple. This equation is based on a right triangle, in which O and A are legs of the triangle, and H is the hypotenuse. Consequently you know that O2 + A2 = H2. That’s just the Pythagorean theorem. That’s what lets you do the last step, in which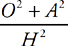 
= 1. This completes the second commonly used identity that you should memorize.
sin2 θ + cos 2 θ = 1
In addition to memorizing these two identities, you should practice working algebraically with trig functions in general. Some questions may require you to use the SOHCAHTOA definitions of the trig functions; others may require you to use the two identities you’ve just reviewed. Take a look at these examples:
35. If sin x = 0.707, then what is the value of (sin x) • (cos x) • (tan x) ?
(A) 1.0
(B) 0.707
(C) 0.5
(D) 0.4
(E) 0.207
Here’s How to Crack It
This is a tricky question. To solve it, simplify that complicated trigonometric expression. Writing in the SOHCAHTOA definitions works just fine, but in this case it’s even faster to use one of those identities.
Now it’s a simpler matter to answer the question. If sin x = 0.707, then sin2 x = 0.5. The answer is (C).
36. If sin a = 0.4, and 1 − cos2 a = x, then what is the value of x ?
(A) 0.8
(B) 0.6
(C) 0.44
(D) 0.24
(E) 0.16
Here’s How to Crack It
Here again, the trick to the question is simplifying the complicated trig expression. Since sin2 θ + cos2 θ= 1, you can rearrange any of those terms to rephrase it. Using the second trig identity, you can quickly take these steps:
1 − cos2 a = x
sin2 a = x
(0.4)2 = x
x = 0.16
And that’s the answer. (E) is correct.
Using the SOHCAHTOA definitions and the two trigonometric identities reviewed in this section, simplify trigonometric expressions to answer the following sample questions.
Try the following practice questions. The answers to these drills can be found in Chapter 12.
25. (1 − sin x)(1 + sin x) =
(A) cos x
(B) sin x
(C) tan x
(D) cos2 x
(E) sin2 x
31. 
=
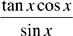(A) 
(B)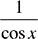 
(C) 1
(D) cos2 x
(E) tan x
39. 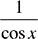−(sin x)(tan x) =
(A) cos x
(B) sin x
(C) tan x
(D) cos2 x
(E) sin2 x
42. 
=
(A) 1 − cos x
(B) 1 − sin x
(C) tan x + 1
(D) cos2 x
(E) sin2 x
On the Math Level 2 Subject Test, you may run into the other three trigonometric functions—the cosecant, secant, and cotangent. These functions are abbreviated cscθ, secθ, and cotθ, respectively, and they are simply the reciprocals of the three basic trigonometric functions you’ve already reviewed.
You can also express these functions in terms of the sides of a right triangle—just by flipping over the SOHCAHTOA definitions of the three basic functions.
These three functions generally show up in algebra-style questions, which require you to simplify complex expressions containing trig functions. The goal is usually to get an expression into the simplest form possible, one that contains no fractions. Such questions are like algebra-style questions involving the three basic trig functions; the only difference is that the addition of three more functions increases the number of possible forms an expression can take. For example:
The entire expression (cos x)(cot x) + (sin2 x csc x) is therefore equivalent to a single trig function, the cosecant of x. That’s generally the way algebraic trigonometry questions work on the Math Level 2 Subject Test.
Simplify each of these expressions to a single trigonometric function. Keep an eye out for the trigonometric identities reviewed on this page; they’ll still come in handy. The answers to these drills can be found in Chapter 12.
19. sec2 x − 1 =
(A) sin x cos x
(B) sec2 x
(C) cos2 x
(D) sin2 x
(E) tan2 x
23. 
=
(A) cos x
(B) sin x
(C) tan x
(D) sec x
(E) csc x
24. sin x + (cos x)(cot x) =
(A) csc x
(B) sec x
(C) cot x
(D) tan x
(E) sin x
There are two common ways to represent trigonometric functions graphically—on the unit circle, or on the coordinate plane (you’ll get a good look at both methods in the coming pages). Both of these graphing approaches are ways of showing the repetitive nature of trigonometric functions. All of the trig functions (sine, cosine, and the rest) are called periodic functions. That simply means that they cycle repeatedly through the same values.
This is the unit circle. It looks a little like the coordinate plane; in fact, it is the coordinate plane, or at least a piece of it. The circle is called the unit circle because it has a radius of 1 (a single unit). This is convenient because it makes trigonometric values easy to figure out. The radius touching any point on the unit circle is the hypotenuse of a right triangle. The length of the horizontal leg of the triangle is the cosine (which is therefore the x-coordinate) and the length of the vertical leg is the sine (which is the y-coordinate). It works out this way because sine = opposite ÷ hypotenuse, and cosine = adjacent ÷ hypotenuse; and here the hypotenuse is 1, so the sine is simply the length of the opposite side, and the cosine simply the length of the adjacent side.
Suppose you wanted to show the sine and cosine of a 30° angle. That angle would appear on the unit circle as a radius drawn at a 30° angle to the positive x-axis (above). The x-coordinate of the point where the radius intercepts the circle is 0.866, which is the value of cos 30°. The y-coordinate of that point is 0.5, which is the value of sin 30°.
Now take a look at the sine and cosine of a 150° angle. As you can see, it looks just like the 30° angle, flipped over the y-axis. Its y-value is the same—sin 150° = 0.5—but its x-value is now negative. The cosine of 150° is −0.866.
Here, you see the sine and cosine of a 210° angle. Once again, this looks just like the 30° angle, but this time flipped over the x- and y-axes. The sine of 210° is −0.5; the cosine of 210° is −0.866.
This is the sine and cosine of a 330° angle. Like the previous angles, the 330° angle has a sine and cosine equivalent in magnitude to those of the 30° angle. In the case of the 330° angle, the sine is negative and the cosine positive. So, sin 330° = −0.5 and cos 330° = 0.866. Notice that a 330° angle is equivalent to an angle of −30°.
Following these angles around the unit circle gives us some useful information about the sine and cosine functions.
• Sine is positive between 0° and 180° and negative between 180° and 360°. At 0°, 180°, and 360°, sine is zero. At 90°, sine is 1. At 270°, sine is −1.
• Cosine is positive between 0° and 90° and between 270° and 360°. (You could also say that cosine is positive between −90° and 90°.) Cosine is negative between 90° and 270°. At 90° and 270°, cosine is zero. At 0° and 360°, cosine is 1. At 180°, cosine is −1.
When these angles are sketched on the unit circle, sine is positive in quadrants I and II, and cosine is positive in quadrants I and IV. There’s another important piece of information you can get from the unit circle. The biggest value that can be produced by a sine or cosine function is 1. The smallest value that can be produced by a sine or cosine function is −1.
Following the tangent function around the unit circle also yields useful information.
The sine of 45° is 
, or 0.707, and the cosine of 45° is also , or 0.707. Since the tangent is the ratio of the sine to the cosine, that means that the tangent of 45° is 1.
The tangent of 135° is −1. Here the sine is positive, but the cosine is negative.
The tangent of 225° is 1. Here the sine and cosine are both negative.
The tangent of 315° is −1. Here the sine is negative, and the cosine is positive.
This is the pattern that the tangent function always follows. It’s positive in quadrants I and III and negative in quadrants II and IV.
• Tangent is positive between 0° and 90° and between 180° and 270°.
• Tangent is negative between 90° and 180° and between 270° and 360°.
The unit circle is extremely useful for identifying equivalent angles (like 270° and −90°), and also for seeing other correspondences between angles, like the similarity between the 45° angle and the 135° angle, which are mirror images of one another on the unit circle.
A good way to remember where sine, cosine, and tangent are positive is to write the words of the phrase All Students Take Calculus in quadrants I, II, III, and IV, respectively, on the coordinate plane. The first letter of each word (A S T C) tells you which functions are positive in that quadrant. So All three functions are positive in quadrant I, the Sine function is positive in quadrant II, the Tangent function is positive in quadrant III, and the Cosine function is positive in quadrant IV.
Make simple sketches of the unit circle to answer the following questions about angle equivalencies. The answers to these drills can be found in Chapter 12.
18. If sin 135° = sin x, then x could equal
(A) −225°
(B) −45°
(C) 225°
(D) 315°
(E) 360°
21. If cos 60° = cos n, then n could be
(A) 30°
(B) 120°
(C) 240°
(D) 300°
(E) 360°
26. If sin 30° = cos t, then t could be
(A) −30°
(B) 60°
(C) 90°
(D) 120°
(E) 240°
30. If tan 45° = tan x, then which of the following could be x ?
(A) −45°
(B) 135°
(C) 225°
(D) 315°
(E) 360°
36. If 0° ≤ θ ≤ 360° and (sin θ)(cos θ) < 0, which of the following gives the possible values of θ ?
(A) 0° ≤ θ ≤ 180°
(B) 0° ≤ θ ≤ 180° or 270° ≤ θ ≤ 360°
(C) 0° < θ < 90° or 180° < θ < 270°
(D) 90° < θ < 180° or 270° < θ < 360°
(E) 0° < θ < 180° or 270° < θ < 360°
On the Math Level 2 Subject Test, you may run into an alternate means of measuring angles. This alternate system measures angles in radians rather than degrees. One degree is defined as 
of a full circle. One radian, on the other hand, is the measure of an angle that intercepts an arc exactly as long as the circle’s radius. Since the circumference of a circle is 2π times the radius, the circumference is about 6.28 times as long as the radius, and there are about 6.28 radians in a full circle.
Because a number like 6.28 isn’t easy to work with, angle measurements in radians are usually given in multiples or fractions of π. For example, there are exactly 2π radians in a full circle. There are π radians in a semicircle. There are 
radians in a right angle. Because 2π radians and 360° both describe a full circle, you can relate degrees and radians with the following proportion:
To convert degrees to radians, just plug the number of degrees into the proportion and solve for radians. The same technique works in reverse for converting radians to degrees. The figures on the next page show what the unit circle looks like in radians, compared to the unit circle in degrees.
By referring to these unit circles and using the proportion given on this page, fill in the following chart of radian−degree equivalencies. The answers to these drills can be found in Chapter 12.
| Degrees | Radians |
| 30° | |
| 45° | |
 |
|
|
|
| 120° | |
 |
|
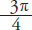| 150° | |
| π | |
 |
|
| 240° | |
 |
|
| 300° | |
| 315° |  |
| 330° | |
| 2π |
In a unit-circle diagram, the x-axis and y-axis represent the horizontal and vertical components of an angle, just as they do on the coordinate plane. The angle itself is represented by the angle between a certain radius and the positive x-axis. Any trigonometric function can be represented on a unit-circle diagram.
When a single trigonometric function is graphed, however, the axes take on different meanings. The x-axis represents the value of the angle; this axis is usually marked in radians. The y-axis represents a specific trigonometric function of that angle. For example, here is the coordinate plane graph of the sine function.
Compare this graph to the unit circle on this page. A quick comparison will show you that both graphs present the same information. At an angle of zero, the sine is zero; at a quarter circle ( radians, or 90°), the sine is 1; and so on.
Here is the graph of the cosine function.
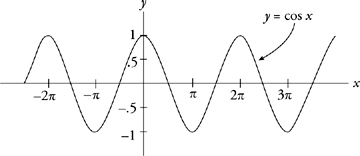
Notice that the cosine curve is identical to the sine curve, only shifted to the left by radians, or 90°. The cosine function also has a period of 2π radians.
Finally, here is the graph of the tangent function.
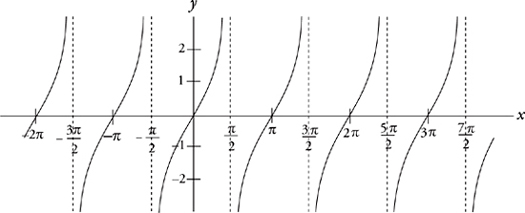
This function, obviously, is very different from the others. First, the tangent function has no upper or lower limit, unlike the sine and cosine functions, which produce values no higher than 1 or lower than −1. Second, the tangent function has asymptotes. These are values on the x-axis at which the tangent function does not exist; they are represented by vertical dotted lines. Finally, the tangent function has a period of π radians.
The Undefined Tangent
It’s easy to see why the tangent function’s graph has asymptotes, if you recall the definition of the tangent.
tan θ = 
A fraction is undefined whenever its denominator equals zero. At any value where the cosine function equals zero, therefore, the tangent function is undefined—it doesn’t exist. As you can see by comparing the cosine and tangent graphs, the tangent has an asymptote wherever the cosine function equals zero.
It’s important to be able to recognize the graphs of the three basic trigonometric functions. You’ll find more information about these functions and their graphs in the following chapter on functions.
The rules of trigonometry are based on the right triangle, as you’ve seen in the preceding sections. Right triangles are not, however, the only places you can use trigonometric functions. There are a couple of powerful rules relating angles and lengths that you can use in any triangle. These are rules that only come up on the Math Level 2 Subject Test, and there are only two basic laws you need to know—the Law of Sines and the Law of Cosines.
The Law of Sines can be used to complete the dimensions of a triangle about which you have partial information. This is what the law says:
Let’s take a look at an example.
∠B = _____________
AB = _____________
AC = _____________
In this triangle, you know only two angles and one side. Immediately, you can fill in the third angle, knowing that there are 180° in a triangle. Then, you can fill in the missing sides using the Law of Sines. Write out the proportions of the Law of Sines, filling in the values you know.
At this point, you can set up two individual proportions and solve them individually for b and c, respectively.
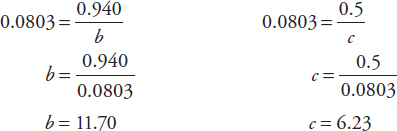
The length of AB is therefore 6.23, and the length of AC is 11.70. Now you know every dimension of triangle ABC.
The Law of Sines can be used in any triangle if you know
• two sides and one of their opposite angles (this gives you two different possible triangles)
• two angles and any side
When you don’t have the information necessary to use the Law of Sines, you may be able to use the Law of Cosines instead. The Law of Cosines is another way of using trigonometric functions to complete partial information about a triangle’s dimensions.
c2 = a2 + b2 −2ab cos C
The Law of Cosines is a way of completing the dimensions of any triangle. You’ll notice that it looks a bit like the Pythagorean theorem. That’s basically what it is, with a term added to the end to compensate for non-right angles. If you use the Law of Cosines on a right triangle, the “2ab cos C” term becomes zero, and the law becomes the Pythagorean theorem. The Law of Cosines can be used to fill in unknown dimensions of a triangle when you know any three of the quantities in the formula.
c = ______________ ∠A = ______________ ∠B = ______________
In this triangle, you know only two sides and an angle—the angle between the known sides. That is, you know a, b, and C. In order to find the length of the third side, c, just fill the values you know into the Law of Cosines, and solve.
c2 = a2 + b2 − 2ab cos C
c2 = (10)2 + (12)2 − 2(10)(12) cos 45°
c2 = 100 + 144 − 240(0.707)
c2 = 74.3
c2 = 8.62
The length of AB is therefore 8.62. Now that you know the lengths of all three sides, just use the Law of Sines to find the values of the unknown angles, or re-arrange the Law of Cosines to put the other unknown angles in the C position, and solve to find the measures of the unknown angles.
The Law of Cosines can be used in any triangle if you know
• all three sides
• two sides and the angle between them
In the following practice exercises, use the Law of Sines and the Law of Cosines to complete the dimensions of these non-right triangles. The answers to these drills can be found in Chapter 12.
1. a = ______________ ∠B = ______________ ∠C = ______________
2. ∠A = ______________ ∠B = ______________ ∠C = ______________
3. c = ______________ ∠B = ______________ ∠C = ______________
Polar coordinates are another way of describing the position of a point in the coordinate plane. In the previous figure, the position of point P can be described in two ways. In standard rectangular coordinates, you would count across from the origin to get an x-coordinate and up from the origin to get a y-coordinate. (Remember: These x and y distances can be regarded as legs of a right triangle. The hypotenuse of the triangle is the distance between the point and the origin.) Rectangular coordinates consist of a horizontal distance and a vertical distance, and take the form (x, y). In rectangular coordinates, point P would be described as (5
, 5).
Polar coordinates consist of the distance, r, between a point and the origin, and the angle, θ, between that segment and the positive x-axis. Polar coordinates thus take the form (r, θ). The angle θ can be expressed in degrees, but is more often expressed in radians. In polar coordinates, therefore, P could be described as (10, 30°) or 
.
As you saw in the unit circle, there’s more than one way to express any angle. For any angle, there is an infinite number of equivalent angles that can be produced by adding or subtracting 360° (or 2π, if you’re working in radians) any number of times. Therefore, there is an infinite number of equivalent polar coordinates for any point. Point P, at (10, 30°), can also be expressed as (10, 390°), or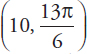 
. You can continually produce equivalent expressions by adding or subtracting 360° (or 2π).
There’s still another way to produce equivalent polar coordinates. The distance from the origin—the r in (r, θ)—can be negative. This means that once you’ve found the angle at which the hypotenuse must extend, a negative distance extends in the opposite direction, 180° away from the angle. Therefore, you can also create equivalent coordinates by increasing or decreasing the angle by 180° and flipping the sign on the distance. The point P(10, 30°) or could also be expressed as (−10, 210°) or 
Other equivalent coordinates can be generated by pairing equivalent angles with these negative distances.
Converting rectangular coordinates to polar coordinates and vice versa is simple. You just use the trigonometry techniques reviewed in this chapter.
Given a point (r, θ) in polar form, you can find its rectangular coordinates by drawing a right triangle such as the following:
From this picture, using SOHCAHTOA and the Pythagorean theorem, you can see the following relationships:
cos θ = 
; sin θ =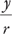 
; tan θ = 
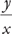; x2 + y2 = r2; θ = tan−1 
Try the following practice questions about polar coordinates. The answers to these drills can be found in Chapter 12.
39. Which of the following rectangular coordinate pairs is equivalent to the polar coordinates 
?
(A) (0.5, 1.7)
(B) (2.6, 5.2)
(C) (3.0, 5.2)
(D) (4.2, 4.8)
(E) (5.2, 15.6)
42. The point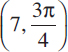 
in polar coordinates is how far from the x-axis?
(A) 3.67
(B) 4.95
(C) 5.25
(D) 6.68
(E) 16.71
45. The points A, B, and C in polar coordinates define which of the following?
(A) A point
(B) A line
(C) A plane
(D) A three-dimensional space
(E) None of these
, cos = 
, and tan = 
. Tan is also equal to 
. ETS will test these with trigonometric identity questions.
, sec θ = 
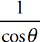, and cot θ = 
.
.
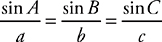; and the Law of Cosines says that c2 = a2 + b2 − 2ab cos C.; sin θ = 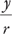; tan θ = 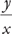; x2 + y2 = r2; and θ tan−1
.
