In this chapter, you learn to find the perimeter, area, and volume of geometric figures.
Perimeter
The perimeter of a simple, closed plane figure is a one-dimensional concept and is the distance around the figure. The perimeter is always measured in units of length such as inches, feet, centimeters, or meters. What length of fence is needed to enclose a yard? What length of decorative border is needed for a mirror? Situations such as these call for measuring the perimeter.
As an example, find the perimeter of the following figure.
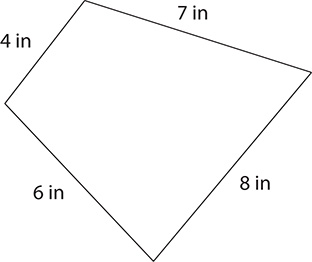
To find the perimeter, add the lengths of the four sides:
Perimeter = 4 in + 7 in + 8 in + 6 in = 25 in
Problem
Find the perimeter of the figure shown.
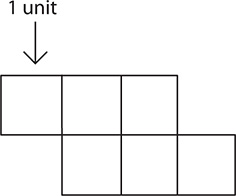
Solution
The perimeter is the distance around the figure.
Step 1. Label the lengths of each side.
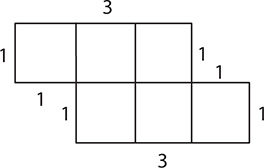
Step 2. Sum the lengths of the sides to get the perimeter.
Perimeter = 3 + 1 + 1 + 1 + 3 + 1 + 1 + 1 = 12 units
Perimeter of Special Shapes
Some polygons have characteristics that enable you to deduce formulas for their perimeters.
A rectangle has opposite sides that are congruent. If the length is denoted by l and the width by w, then the formula for the perimeter P is P = 2l + 2w.
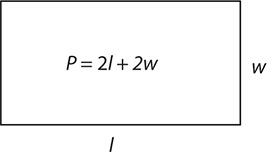
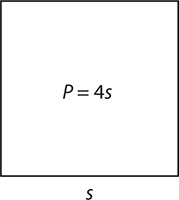
A square has four congruent sides. If the side lengths are denoted by s, then the formula for the perimeter is P = 4s.
Several other figures often encountered in the study of geometry include the following.
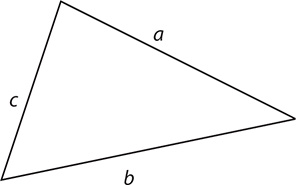
Recall from Chapter 15 that a triangle is a simple, closed plane figure with three sides. The perimeter of a triangle is the sum of its sides; that is, P = a + b + c.
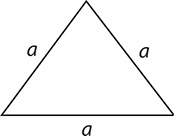
The perimeter of an equilateral triangle with sides each of length a is P = 3a.
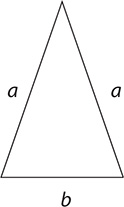
The perimeter of an isosceles triangle with congruent sides of length a and third side of length b is P = 2a + b.
A circle is a unique figure. The perimeter of a circle is given the special name of circumference, C. It has no straight sides. The formula for C was discovered long ago after much thought and experimentation. It is simply C = πd = 2πr, where d is the length of the diameter of the circle and r is the length of its radius.
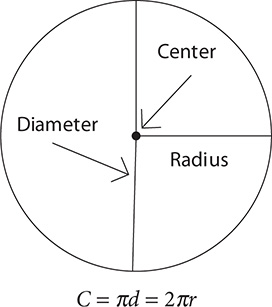
Problem
Find the indicated perimeter or circumference.
a. Find the perimeter of a square that measures 3.5 cm on each side.
b. Find the perimeter of an isosceles triangle whose congruent sides are each 10 ft and the other side 6 ft.
c. Find the circumference of a circle whose radius is 20 cm. Use 3.14 to approximate π.
d. The circumference of the Earth is about 25,000 mi. What is the approximate diameter of the Earth? Use π ≈ 3.14.
Solution
a. Find the perimeter of a square that measures 3.5 cm on each side.
Step 1. Sketch the figure.
Step 2. Use the formula for the perimeter of a square.
P = 4s
Step 3. Apply the formula to the figure and compute the perimeter.
P = 4(3.5 cm) = 14 cm
b. Find the perimeter of an isosceles triangle whose congruent sides are each 10 ft and the other side 6 ft.
Step 1. Sketch the figure.
Step 2. Use the formula for the perimeter of an isosceles triangle with congruent sides of length a and third side of length b.
P = 2a + b
Step 3. Apply the formula to the figure and compute the perimeter.
P = 2(l0ft) + 6 ft = 20 ft + 6 ft = 26 ft
c. Find the circumference of a circle whose radius is 20 cm. Use 3.14 to approximate π.
Step 1. Sketch the figure.
Step 2. Use the formula for the circumference of a circle.
C = 2πr
Step 3. Apply the formula to the figure and compute the circumference.
C = 2π(20 cm) ≈ 2(3.14)(20 cm) = 125.6 cm
d. The circumference of the Earth is about 25,000 mi. What is the approximate diameter of the Earth? Use π ≈ 3.14.
Step 1. Select the appropriate formula for the problem. The Earth is approximately a sphere, so the circumference is approximately that of a circle.
C = πd
Step 2. Apply the formula to the problem.
25,000 mi = πd ≈ (3.14)d
Step 3. Solve for d.
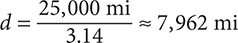
Area
The area of a closed plane figure is a two-dimensional concept. It is the amount of surface enclosed by the boundary of the figure. For instance, in Figure 16.1, there are (2 in)(4 in) = 8(in)(in) = 8 in2 of area enclosed by the rectangular figure shown.
Area is always measured in square units such as square inches (in2), square feet (ft2), square miles (mi2), and square meters (m2). Regardless of the shape of the figure, the area units are always square units.
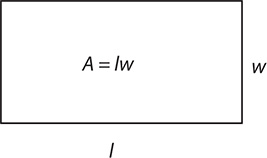
As with perimeter, special figures have special formulas for finding the area enclosed by the figure. The formula for the area of a rectangle is A = lw, where A is the area and l and w are the length and width of the sides of the rectangle.
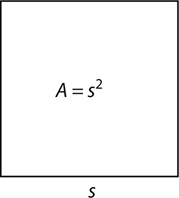
The area of a square is A = s2.
The area of a triangle is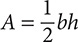 
, where the base, b, can be any side of the triangle and the height, h, for that base is the perpendicular distance from the opposite vertex to that base (or an extension of it).
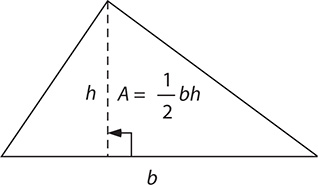
Like the circumference, the area of a circle involves the number π and is completely determined by the length of the radius. The formula for the area of a circle is A = πr2, where r is the length of the radius.
Problem
Find the indicated area.
a. Find the area of a rectangle whose length is 6 in and width 4 in.
b. Find the area of a circle whose diameter is 24 cm.
c. Find the area of a triangle whose base measures 8 ft and height (to that base) measures 7 ft.
Solution
a. Find the area of a rectangle whose length is 6 in and width 4 in.
Step 1. Sketch the figure.
Step 2. Choose the appropriate area formula.
A = lw
Step 3. Apply the formula to the figure and compute the area.
A = (6 in)(4 in) = 24 in2
b. Find the area of a circle whose diameter is 24 cm.
Step 1. Sketch the figure.
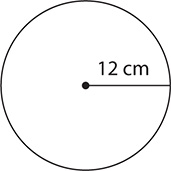
Note: The diameter is 24 cm, so the radius 
.
Step 2. Choose the appropriate area formula.
A = πr2
Step 3. Apply the formula to the figure and compute the area.
A = π(12 cm)2 ≈ (3.14)(144 cm2) = 452.16 cm2
c. Find the area of a triangle whose base measures 8 ft and height (to that base) measures 7 ft.
Step 1. Sketch the figure.
Step 2. Choose the appropriate area formula.
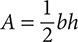
Step 3. Apply the formula to the figure and compute the area.
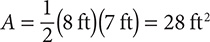
Surface Area
The surface area of a solid three-dimensional figure is the area of the outside surface of the figure. As such, the surface area is a two-dimensional measure and has square units. If the figure is a rectangular prism (a box), as shown in Figure 16.2, then the surface area is the sum of the areas of all the faces.
Problem
Find the surface area of a box that has dimensions l = 8 in, w = 6 in, and h = 5 in.
Solution
Step 1. Sketch the figure.
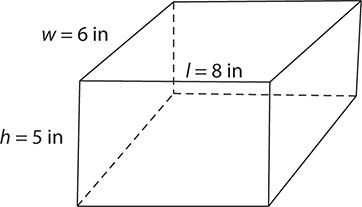
Step 2. Use the length and height to find the area of the front and rear faces.
Area of the front and rear faces = 2(8 in)(5 in) = 80 in2
Step 3. Use the height and width to find the area of the two side faces.
Area of the two side faces = 2(5 in)(6 in) = 60 in2
Step 4. Use the length and width to find the area of the top and bottom faces.
Area of the top and bottom faces = 2(6 in)(8 in) = 96 in2
Step 5. Add the areas to get the final surface area.
Surface area = 80 in2 + 60 in2 + 96 in2 = 236 in2
Volume
Volume is a three-dimensional concept and is measured in cubic units. It is a measure of the space or capacity inside a three-dimensional closed figure such as a can, a cereal box, a room of a house, or a soccer ball. These types of measurements are very important to manufacturers of goods.
As with perimeter and area, special figures have special volume formulas. A rectangular prism (box) is a three-dimensional figure all of whose faces are rectangles. It has three characteristic measurements: length l, width w, and height h. The volume formula is V = lwh.
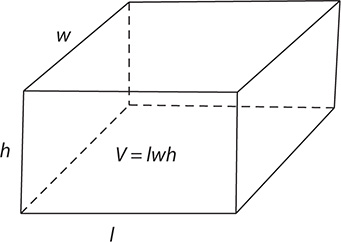
A cylinder, such as a can, has a circular base and top; consequently, the number π enters into the volume formula. The volume formula for a cylinder is V = πr2h, where r is the radius of the base circle and h is the height of the cylinder.
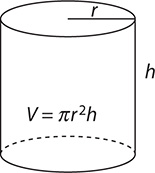
Notice that in both of the previous formulas, V = (lw)h and V = (πτ2)/h, the product in parentheses is the area of the base of the respective figures. If you let B = the area of the base, then both formulas are the same, V = Bh. In fact, any prism or any cylinder has the same volume formula, V = Bh, where B is the area of the base and h is the height. This is the preferred format of these particular formulas in most of the current textbooks.
The following figures are triangular prisms, and the V = Bh formula applies to them also. In this instance, the base areas are the areas of triangles. Notice that a prism does not have to rest on its “base.”
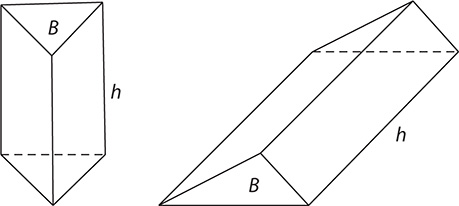
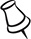The volume, V, of a prism or cylinder that has a base of area B and height h is
V = Bh
A sphere has volume formula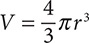 
, where r is the radius of the sphere. This formula can be derived with the tools of calculus.
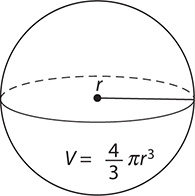
Problem
Find the volume as indicated.
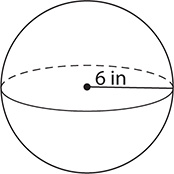
a. Find the volume of a sphere of radius 6 in.
b. The living room in a house has dimensions: l = 30 ft, w = 20 ft, and h = 8 ft. The owner of the house wants to know what volume of air the air conditioner will have to cool. This information will determine the size of air conditioner the owner will purchase. Find the volume of the living room.
c. Find the volume of a soda can that has a base radius of 2 in and a height of 5 in.
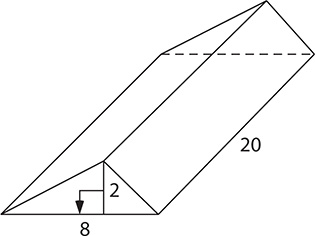
d. Find the volume of the triangular prism shown.
Solution
a. Find the volume of a sphere of radius 6 in.
Step 1. Sketch the figure.
Step 2. Select the appropriate formula.
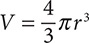
Step 3. Apply the formula to the problem and compute the volume.
b. The living room in a house has dimensions: l = 30 ft, w = 20 ft, and h = 8 ft. The owner of the house wants to know what volume of air the air conditioner will have to cool. This information will determine the size of air conditioner the owner will purchase. Find the volume of the living room.
Step 1. Sketch the figure.
Step 2. Select the appropriate formula.
V = Bh = (lw)h
Step 3. Apply the formula to the problem and compute the volume.
V = [(30 ft)(20 ft)]8 ft = [600 ft2]8ft = 4,800 ft3
c. Find the volume of a soda can that has a base radius of 2 in and a height of 5 in.
Step 1. Sketch the figure.
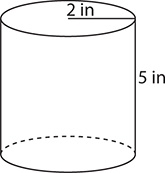
Step 2. Select the appropriate formula.
V = Bh =(πr2)h
Step 3. Apply the formula to the problem and compute the volume.
V = [π(2 in)2]5 in ≈ [3.14(4 in2)]5 in = 62.8 in3
d. Find the volume of the triangular prism shown.
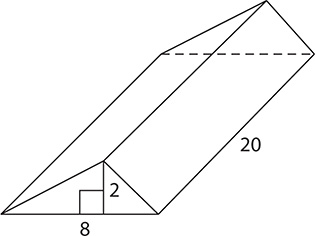
Step 1. Select the appropriate formula.
V = Bh
Step 2. Apply the formula to the problem and compute the volume.
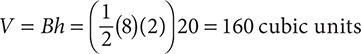
The exercises are designed to give you experience with different formulas and actually calculating perimeters, areas, and volumes. Some of these formulas you will remember from now on. Others will dim with time. The important thing is to remember there are such formulas and where to find them when needed. If you are comfortable with using them, you can put them to use to solve problems that might arise.
Exercise 16
1. The Mercedes-Benz Superdome in New Orleans is a hemisphere that has a diameter of 680 ft. What is the approximate circumference of the hemisphere?
2. How many 12 in by 12 in tiles are needed to cover a floor that is 20 ft by 30 ft?
3. A rectangular garden is 10 ft by 12 ft. A rectangular sidewalk 1 ft wide is built around the outside of the garden. What is the area of the complete garden region?
4. A rectangle and a square have equal areas. If the rectangle is 4 ft by 9 ft, how long is a side, s, of the square?
5. A lot is 21 ft by 30 ft. To support a fence, a post is needed at each corner and every 3 ft in between. How many posts are needed?
6. What is the area of the bottom surface of a circular swimming pool of diameter 20 ft?
7. What is the approximate volume of air trapped in the hemispherical top of the Mercedes-Benz Superdome in New Orleans? (See problem 1.)
8. A silo has a height of 100 ft and a base radius of 20 ft.
a. How much grain (in ft 3) will the silo hold?
b. If a bushel of wheat takes up 4 ft 3, how many bushels of wheat will the silo hold?
c. If a bushel of wheat sells for $2, what is the value of a silo full of wheat?
9. Find the area of a triangle whose base measures 21 in and whose height measured to that base is 14 in.
10. Find the volume of the prism shown.
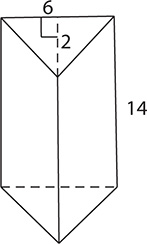
11. Find the surface area of a rectangular prism that has dimensions l = 30 ft, w = 20 ft, and h = 8 ft.