In this chapter, you learn about the Pythagorean theorem.
Right Triangle Concepts
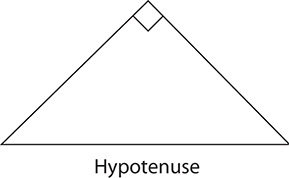
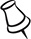
A right triangle is a triangle with exactly one right (90°) angle. The longest side of the right triangle is opposite the 90° angle and is called the hypotenuse. The word hypotenuse derives from the Greek hypo- (“under”) and tein-ein (“to stretch”). This interpretation was probably derived from the fact that the Pythagoreans, followers of the Greek mathematician Pythagoras, and other early geometers drew most of their triangles with the hypotenuse down (“under”) as in Figure 17.1.
Pythagorean Theorem
The three sides of a right triangle have a special relationship, named after Pythagoras.
Pythagorean Theorem
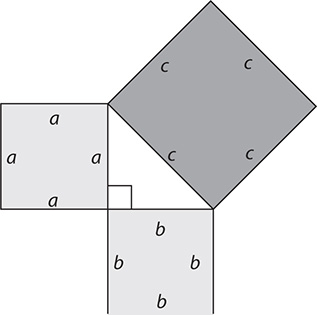
If a right triangle has legs of lengths a and b and hypotenuse of length c, then a2 + b2 = c2
This theorem is probably the most well-known theorem of mathematics and also one of the most used. Its discovery led not only to a great advance in geometry but also to the introduction of the completely unknown, at the time, set of irrational numbers.
The theorem was originally stated geometrically as the square on the hypotenuse is equal to the sum of the squares on the two legs, as depicted in Figure 17.2.
This formula is used so much today in algebra, calculus, physics, engineering, and many other disciplines that we tend to forget that it is a geometry theorem.
The example problems and the exercise problems are varied to illustrate the wide use of the theorem.
Problem
Use the Pythagorean theorem to solve as indicated. Use your calculator and round answers, as needed.
a. Find the length of the diagonal of a rectangular garden that has dimensions of 30 ft by 40 ft.
b. Televisions are sized by the length of the diagonal of the face. Find the “size” of a portable TV whose rectangular face measures 27 in by 21 in.
c. A 25 ft ladder is placed against a wall with the base of the ladder 12 ft from the wall on level ground. How high up on the wall does the ladder reach?
d. Two people want to carry a tall mirror that is 9 by 9 ft square through a doorway that measures 3 ft by 8 ft. Will the mirror fit through the doorway?
e. Find the area of the triangle shown.
Solution
a. Find the length of the diagonal of a rectangular garden that has dimensions of 30 ft by 40 ft.
Step 1. Sketch the figure.
Step 2. From the figure, you can see that the diagonal is the hypotenuse of a right triangle having legs of 30 ft and 40 ft.
Step 3. Substitute the given values into the Pythagorean theorem, omitting the units for convenience.
Step 4. Solve for c.
To obtain c, you must find the square root of 2,500. Thus, you have 
Step 5. State the answer.
The length of the diagonal of the garden is 50 ft.
b. Televisions are sized by the length of the diagonal of the face. Find the “size” of a portable TV whose rectangular face measures 27 in by 21 in.
Step 1. Sketch the figure.
Step 2. From the figure, you can see that the diagonal is the hypotenuse of a right triangle having legs of 27 in and 21 in.
Step 3. Substitute these values into the Pythagorean theorem, omitting the units for convenience.
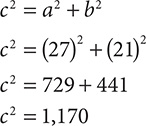
Step 4. Solve for c.
To obtain c, you must find the square root of 1,170. Thus, you have 
Step 5. State the answer.
The size of the TV is 34 in (rounded to the nearest whole number).
c. A 25 ft ladder is placed against a wall with the base of the ladder 12 ft from the wall on level ground. How high up on the wall does the ladder reach?
Step 1. Sketch the figure.
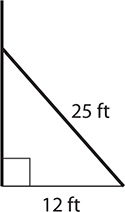
Step 2. The ladder, the ground, and the wall form a right triangle with the ladder as the hypotenuse.
Step 3. Let h = the distance up the wall the ladder reaches and substitute the numbers into the Pythagorean theorem.
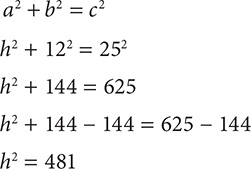
Step 4. Solve for h.
To obtain h, you must find the square root of 481. Thus, you have 
Step 5. Answer the question.
The ladder reaches approximately 22 ft up the wall.
d. Two people want to carry a tall mirror that is a 9 by 9 ft square through a doorway that measures 3 ft by 8 ft. Will the mirror fit through the doorway?
Step 1. Let m = length of the diagonal of the doorway. Sketch the figure.
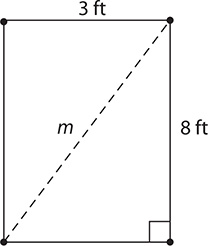
Step 2. Analyze the problem.
Because the doorway is only 8 ft high, the only way to get the mirror through the doorway is on a slant. Therefore, determine whether the length of the diagonal m of the doorway is greater than 9 ft, the length of an edge of the mirror.
Step 3. A right triangle is formed with m as the hypotenuse. Substitute the numbers into the Pythagorean theorem.
c2 = a2 + b2
m2 = 32 + 82 = 9 + 64 = 73
Step 4. Solve for m.
To obtain m, you must find the square root of 73. Thus, you have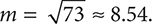 
.
Step 5. Answer the question.
The mirror will not fit through the doorway because the length of the diagonal of the doorway is about 8.54 ft, which is less than 9 ft, the length of an edge of the mirror.
e. Find the area of the triangle shown.
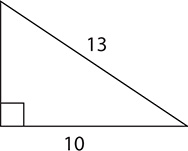
The height must be known in order to use the area formula for the triangle.
Step 1. Sketch the figure. Let h be the measure of the height.
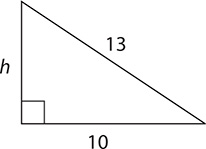
Step 2. Substitute into the Pythagorean theorem.
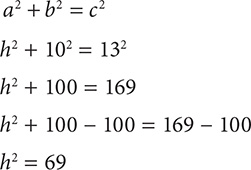
Step 3. Solve for h.
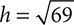(because 69 is not a perfect square, leave the answer in the radical form until the last calculation)
Step 4. Substitute the base and height into the area formula for the triangle.
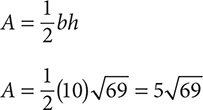
Step 5. Answer the question.
The area of the triangle is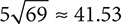 
square units.
Converse of the Pythagorean Theorem
Triangles whose sides satisfy the Pythagorean theorem are right triangles. Thus, you have the following converse statement.
Converse of the Pythagorean Theorem
If a triangle has sides of lengths a, b, and c and a2 + b2 = c2, then the triangle is a right triangle and c is the length of the hypotenuse.
Problem
Is the triangle with sides of 8, 14, and 17 units a right triangle?
Solution
Step 1. Because 17 is the longest side, check whether the numbers satisfy the Pythagorean theorem.
Step 2. Answer the question.
The triangle is not a right triangle.
Exercise 17
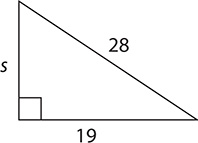
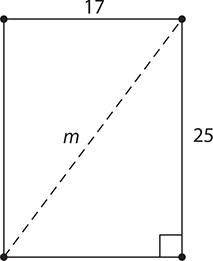
In 1-4, solve for the unknown side. Use your calculator and round, as needed.
5. Is the triangle whose sides are 11, 15, and 19 units a right triangle?
6. Find the area of the region in the semicircle and outside the right triangle. (Hint: Recall the area formula for a circle.)
7. If a person in Texas travels 7 miles north, then 3 miles east, and finally 3 miles south, how far is the person from the starting point?
8. Find the perimeter of the square.
9. Is the triangle whose sides measure and 4 units a right triangle?
10. A ladder 4.0 m long is placed against a wall with the base of the ladder 0.8 m from the wall on level ground. How high up on the wall does the ladder reach?