These are very cursory descriptions of the two views. But it is enough to see that the two embody quite different conceptions of explanation. Nor is it just a matter of choosing which to pursue, since they are joined to distinct metaphysical pictures. In practice the two conceptions meet; for in real life explanations, failure of deductivity is the norm. Duhem predicts this. But proponents of the D-N model can account for the practical facts as well. They attribute the failure of deductivity not to the lack of unity in nature, but to the failings of each particular theory we have to hand.
The difference between the two conceptions with respect to van Fraassen's challenge may be obscured by this practical convergence. We sometimes mistakenly assume that individual explanations, on either account, will look the same. Van Fraassen himself seems to suppose this; for he requires that the empirical substructures provided by a theory should be isomorphic to the true structures of the phenomena. But Duhem says that there can be at best a rough match. If Duhem is right, there will be no wealth of truly deductive explanations no matter how well developed a scientific discipline we look to.
Duhem sides with the thinkers who say 'A physical theory is an abstract system whose aim is to
summarize and to classify
logically a group of experimental laws without aiming to explain these laws', where 'to explain (explicate,
explicare) is to strip reality of the appearances covering it like a veil, in order to see the bare reality itself.'
8 In an effort to remain metaphysically neutral, we might take an account of explanation which is more general than either Duhem's or the D-N story: to explain a collection of phenomenological laws is to give a physical theory of them, a physical theory in Duhem's sense, one that summarizes the laws and logically classifies them; only now we remain neutral as to whether we are also called upon to explain in the deeper sense of stripping away appearances. This is the general kind of account I have been supposing throughout this essay.
There is no doubt that we can explain in this sense.
end p.96
Physical theories abound, and we do not have to look to the future completion of science to argue that they are fairly successful at summarizing and organizing; that is what they patently do now. But this minimal, and non-question-begging, sense of explanation does not meet van Fraassen's challenge. There is nothing about successful organization that requires truth. The stripped down characterization will not do. We need the full paraphernalia of the D-N account to get the necessary connection between truth and explanation. But going beyond the stripped down view to the full metaphysics involved in a D-N account is just the issue in question.
There is still more to Laudan's criticism. Laudan himself has written a beautiful piece against inference to the best explanation.
9 The crux of his argument is this: it is a poor form of inference that repeatedly generates false conclusions. He remarks on case after case in the history of science where we now know our best explanations were false. Laudan argues that this problem plagues theoretical laws and theoretical entities equally. Of my view he says,
What I want to know, is what epistemic difference there is between the evidence we can have for a theoretical law (which you admit to be non-robust) and the evidence we can have for a theoretical entity—such that we are warranted in concluding that, say electrons and protons exist, but that we are not entitled to conclude that theoretical laws are probably true. It seems to me that the two are probably on an equal footing epistemically.
Laudan's favourite example is the electromagnetic aether, which 'had all sorts of independent sources of support for it collected over a century and a half'. He asks, 'Did the enviable successes of one- and two-fluid theories of electricity show that there really was an electrical fluid?'
10
I have two remarks, the first very brief. Although the electromagnetic aether is one striking example, I think these cases are much rarer than Laundan does. So we have a historical dispute. The second remark bears on the first. I have been arguing that we must be committed to the existence of the cause if we are to accept a given causal account.
end p.97
The same is not true for counting a theoretical explanation good. The two claims get intertwined when we address the nontrivial and difficult question, when do we have reasonable grounds for counting a causal account acceptable? The fact that the causal hypotheses are part of a generally satisfactory explanatory theory is not enough, since success at organizing, predicting, and classifying is never an argument for truth. Here, as I have been stressing, the idea of direct experimental testing is crucial. Consider the example of the laser company, Spectra Physics, mentioned in the Introduction to these essays. Engineers at Spectra Physics construct their lasers with the aid of the quantum theory of radiation, non-linear optics, and the like; and they calculate their performance characteristics. But that will not satisfy their customers. To guarantee that they will get the effects they claim, they use up a quarter of a million dollars' worth of lasers every few months in test runs.
I think there is no general theory, other than Mill's methods, for what we are doing in experimental testing; we manipulate the cause and look to see if the effects change in the appropriate manner. For specific causal claims there are different detailed methodologies. Ian Hacking, in 'Experimentation and Scientific Realism', gives a long example of the use of Stanford's Peggy II to test for parity violations in weak neutral currents. There he makes a striking claim:
The experimentalist does not believe in electrons because, in the words retrieved from medieval science by Duhem, they 'save the phenomena'. On the contrary, we believe in them because we use them to
create new phenomena, such as the phenomenon of parity violation in weak neutral current interactions.
11 I agree with Hacking that when we can manipulate our theoretical entities in fine and detailed ways to intervene in other processes, then we have the best evidence possible for our claims about what they can and cannot do; and theoretical entities that have been warranted by well-tested causal claims like that are seldom discarded in the progress of science.
end p.98
4. Conclusion
I believe in theoretical entities. But not in theoretical laws. Often when I have tried to explain my views on theoretical laws, I have met with a standard realist response: 'How could a law explain if it weren't true?' Van Fraassen and Duhem teach us to retort, 'How could it explain if it were true?' What is it about explanation that guarantees truth? I think there is no plausible answer to this question when one law explains another. But when we reason about theoretical entities the situation is different. The reasoning is causal, and to accept the explanation is to admit the cause. There is water in the barrel of my lemon tree, or I have no explanation for its ailment, and if there are no electrons in the cloud chamber, I do not know why the tracks are there.
end p.99
Essay 6 For Phenomenological Laws
Abstract: Conventional accounts like the deductive-nomological model suppose that high-level theories couched in abstract language encompass or imply more low-level laws expressed in more concrete language (here called phenomenological laws). The truth of phenomenological laws is then supposed to provide evidence for the truth of the theory. This chapter argues that, to the contrary, approximations are required to arrive at phenomenological laws and generally these approximations improve on theory and are not dictated by the facts. Examples include an amplifier model, exponential decay and the Lamb shift (Based on work with Jon Nordby).
Nancy Cartwright
0. Introduction
A long tradition distinguishes fundamental from phenomenological laws, and favours the fundamental. Fundamental laws are true in themselves; phenomenological laws hold only on account of more fundamental ones. This view embodies an extreme realism about the fundamental laws of basic explanatory theories. Not only are they true (or would be if we had the right ones), but they are, in a sense, more true than the phenomenological laws that they explain. I urge just the reverse. I do so not because the fundamental laws are about unobservable entities and processes, but rather because of the nature of theoretical explanation itself. As I have often urged in earlier essays, like Pierre Duhem, I think that the basic laws and equations of our fundamental theories organize and classify our knowledge in an elegant and efficient manner, a manner that allows us to make very precise calculations and predictions. The great explanatory and predictive powers of our theories lies in their fundamental laws. Nevertheless the content of our scientific knowledge is expressed in the phenomenological laws.
Suppose that some fundamental laws are used to explain a phenomenological law. The ultra-realist thinks that the phenomenological law is true because of the more fundamental laws. One elementary account of this is that the fundamental laws make the phenomenological laws true. The truth of the phenomenological laws derives from the truth of the fundamental laws in a quite literal sense—something like a causal relation exists between them. This is the view of the seventeenth-century mechanical philosophy of Robert Boyle and Robert Hooke. When God wrote the Book of Nature, he inscribed the fundamental laws of mechanics and he laid down the initial distribution of matter in the universe. Whatever phenomenological laws would be true fell out as a consequence. But this is not only the view of
end p.100
the seventeenth-century mechanical philosophy. It is a view that lies at the heart of a lot of current-day philosophy of science—particularly certain kinds of reductionism—and I think it is in part responsible for the widespread appeal of the deductive-nomological model of explanation, though certainly it is not a view that the original proponents of the D-N model, such as Hempel, and Grünbaum, and Nagel, would ever have considered. I used to hold something like this view myself, and I used it in my classes to help students adopt the D-N model. I tried to explain the view with two stories of creation.
Imagine that God is about to write the Book of Nature with Saint Peter as his assistant. He might proceed in the way that the mechanical philosophy supposed. He himself decided what the fundamental laws of mechanics were to be and how matter was to be distributed in space. Then he left to Saint Peter the laborious but unimaginative task of calculating what phenomenological laws would evolve in such a universe. This is a story that gives content to the reductionist view that the laws of mechanics are fundamental and all the rest are epi-phenomenal.
On the other hand, God may have had a special concern for what regularities would obtain in nature. There were to be no distinctions among laws: God himself would dictate each and every one of them—not only the laws of mechanics, but also the laws of chemical bonding, of cell physiology, of small group interactions, and so on. In this second story Saint Peter's task is far more demanding. To Saint Peter was left the difficult and delicate job of finding some possible arrangement of matter at the start that would allow all the different laws to work together throughout history without inconsistency. On this account all the laws are true at once, and none are more fundamental than the rest.
The different roles of God and Saint Peter are essential here: they make sense of the idea that, among a whole collection of laws every one of which is supposed to be true, some are more basic or more true than others. For the seventeenth-century mechanical philosophy, God and the Book of Nature were legitimate devices for thinking of laws and the relations among them. But for most of us nowadays these stories are
end p.101
mere metaphors. For a long time I used the metaphors, and hunted for some non-metaphorical analyses. I now think that it cannot be done. Without God and the Book of Nature there is no sense to be made of the idea that one law derives from another in nature, that the fundamental laws are basic and that the others hold literally 'on account of' the fundamental ones.
Here the D-N model of explanation might seem to help. In order to explain and defend our reductionist views, we look for some quasi-causal relations among laws in nature. When we fail to find any reasonable way to characterize these relations in nature, we transfer our attention to language. The deductive relations that are supposed to hold between the laws of a scientific explanation act as a formal-mode stand-in for the causal relations we fail to find in the material world. But the D-N model itself is no argument for realism once we have stripped away the questionable metaphysics. So long as we think that deductive relations among statements of law mirror the order of responsibility among the laws themselves, we can see why explanatory success should argue for the truth of the explaining laws. Without the metaphysics, the fact that a handful of elegant equations can organize a lot of complex information about a host of phenomenological laws is no argument for the truth of those equations. As I urged in the last essay, we need some story about what the connection between the fundamental equations and the more complicated laws is supposed to be. There we saw that Adolf Grünbaum has outlined such a story. His outline I think coincides with the views of many contemporary realists. Grünbaum's view eschews metaphysics and should be acceptable to any modern empiricist. Recall that Grünbaum says:
It is crucial to realize that while (a more comprehensive law)
G entails (a less comprehensive law)
L logically, thereby providing an explanation of
L, G is not the 'cause' of
L. More specifically, laws are explained
not by showing the regularities they affirm to be products of the operation of
causes but rather by recognizing their truth to be special cases of more comprehensive truths.
1 end p.102
I call this kind of account of the relationship between fundamental and phenomenological laws a generic-specific account. It holds that in any particular set of circumstances the fundamental explanatory laws and the phenomenological laws that they explain both make the same claims. Phenomenological laws are what the fundamental laws amount to in the circumstances at hand. But the fundamental laws are superior because they state the facts in a more general way so as to make claims about a variety of different circumstances as well.
The generic-specific account is nicely supported by the deductive-nomological model of explanation: when fundamental laws explain a phenomenological law, the phenomenological law is deduced from the more fundamental in conjunction with a description of the circumstances in which the phenomenological law obtains. The deduction shows just what claims the fundamental laws make in the circumstances described.
But explanations are seldom in fact deductive, so the generic-specific account gains little support from actual explanatory practice. Wesley Salmon
2 and Richard Jeffrey,
3 and now many others, have argued persuasively that explanations are not arguments. But their views seem to bear most directly on the explanations of single events; and many philosophers still expect that the kind of explanations that we are concerned with here, where one law is derived from others more fundamental, will still follow the D-N form. One reason that the D-N account often seems adequate for these cases is that it starts looking at explanations only after a lot of scientific work has already been done. It ignores the fact that explanations in physics generally begin with a model. The calculation of the small signals properties of amplifiers, which I discuss in the
next section, is an example.
4 We first
end p.103
decide which model to use—perhaps the T-model, perhaps the hybrid-π model. Only then can we write down the equations with which we will begin our derivation.
Which model is the right one? Each has certain advantages and disadvantages. The T-model approach to calculating the midband properties of the CE stage is direct and simple, but if we need to know how the CE stage changes when the bias conditions change, we would need to know how all the parameters in the transistor circuit vary with bias. It is incredibly difficult to produce these results in a T-model. The T-model also lacks generality, for it requires a new analysis for each change in configuration, whereas the hybrid-π model approach is most useful in the systematic analysis of networks. This is generally the situation when we have to bring theory to bear on a real physical system like an amplifier. For different purposes, different models with different incompatible laws are best, and there is no single model which just suits the circumstances. The facts of the situation do not pick out one right model to use.
I will discuss models at length in the next few essays. Here I want to lay aside my worries about models, and think about how derivations proceed once a model has been chosen. Proponents of the D-N view tend to think that at least then the generic-specific account holds good. But this view is patently mistaken when one looks at real derivations in physics or engineering. It is never strict deduction that takes you from the fundamental equations at the beginning to the phenomenological laws at the end. Instead we require a variety of different approximations. In any field of physics there are at most a handful of rigorous solutions, and those usually for highly artificial situations. Engineering is worse.
Proponents of the generic-specific account are apt to think that the use of approximations is no real objection to their view. They have a story to tell about approximations: the process of deriving an approximate solution parallels a D-N explanation. One begins with some general equations which we hold to be exact, and a description of the situation to which they apply. Often it is difficult, if not impossible, to solve these equations rigorously, so we rely on our description of the situation to suggest approximating procedures.
end p.104
In these cases the approximate solution is just a stand-in. We suppose that the rigorous solution gives the better results; but because of the calculational difficulties, we must satisfy ourselves with some approximation to it.
Sometimes this story is not far off. Approximations occasionally work just like this. Consider, for example, the equation used to determine the equivalent air speed,
V
E , of a plane (where
P
Tis total pressure,
P
0is ambient pressure, ρ
Sis sea level density, and
M is a Mach number):
- (6.1)
-

The value for
V
Edetermined by this equation is close to the plane's true speed.
Approximations occur in connection with (
6.1) in two distinct but typical ways. First, the second term,
is significant only as the speed of the plane approaches Mach One. If
M < 0.5, the second term is discarded because the result for
V
Egiven by
will differ insignificantly from the result given by (
6.1). Given this insignificant variation, we can approximate
V
Eby using
- (6.2)
-
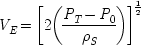
for
M < 0.5. Secondly, (
6.1) is already an approximation, and not an exact equation. The term
has other terms in the denominator. It is a Taylor series expansion. The next term in the expansion is
M6/1600, so we get
end p.105
in the denominator, and so on. For Mach numbers less than one, the error that results from ignoring this third term is less than one per cent, so we truncate and use only two terms.
Why does the plane travel with a velocity,
V, roughly equal to
Because of equation (
6.1). In fact the plane is really travelling at a speed equal to
But since
M is less than 0.5, we do not notice the difference. Here the derivation of the plane's speed parallels a covering law account. We assume that equation (
6.1) is a true law that covers the situation the plane is in. Each step away from equation (
6.1) takes us a little further from the true speed. But each step we take is justified by the facts of the case, and if we are careful, we will not go too far wrong. The final result will be close enough to the true answer.
This is a neat picture, but it is not all that typical. Most cases abound with problems for the generic-specific account. Two seem to me especially damaging: (1) practical approximations usually improve on the accuracy of our fundamental laws. Generally the doctored results are far more accurate than the rigorous outcomes which are strictly implied by the laws with which we begin. (2) On the generic-specific account the steps of the derivation are supposed to show how the fundamental laws make the same claims as the phenomenological laws, given the facts of the situation. But seldom are the facts enough to justify the derivation. Where approximations are called for, even a complete knowledge of the circumstances may not provide the additional premises necessary to deduce the phenomenological laws from the fundamental equations that explain them. Choices must be
end p.106
made which are not dictated by the facts. I have already mentioned that this is so with the choice of models. But it is also the case with approximation procedures: the choice is constrained, but not dictated by the facts, and different choices give rise to different, incompatible results. The generic-specific account fails because the content of the phenomenological laws we derive is not contained in the fundamental laws which explain them.
These two problems are taken up in turn in the next two sections. A lot of the argumentation, especially in Section
2, is taken from a paper written jointly by Jon Nordby and me, 'How Approximations Take Us Away from Theory and Towards the Truth'.
5 This paper also owes a debt to Nordby's 'Two Kinds of Approximations in the Practice of Science'.
6
1. Approximations That Improve on Laws
On the generic-specific account, any approximation detracts from truth. But it is hard to find examples of this at the level where approximations connect theory with reality, and that is where the generic-specific account must work if it is to ensure that the fundamental laws are true in the real world. Generally at this level approximations take us away from theory and each step away from theory moves closer towards the truth. I illustrate with two examples from the joint paper with Jon Nordby.
1.1. An Amplifier Model
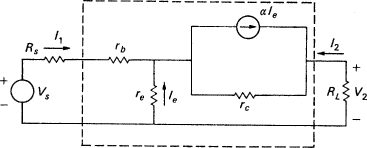
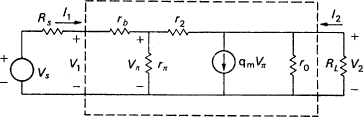
Consider an amplifier constructed according to Figure
6.1. As I mentioned earlier, there are two ways to calculate the small signal properties of this amplifier, the T-model and the hybrid-π model. The first substitutes a circuit model for the transistor and analyses the resulting network. The second characterizes the transistor as a set of two-port parameters and calculates the small signal properties of the amplifier
end p.107
in terms of these parameters. The two models are shown in Figures
6.2 and
6.3 inside the dotted area.
The application of these transistor models in specific situations gives a first rough approximation of transistor parameters at low frequencies. These parameters can be theoretically estimated without having to make any measurements on the actual circuit. But the theoretical estimates are often grossly inaccurate due to specific causal features present in the actual circuit, but missing from the models.
end p.108
One can imagine handling this problem by constructing a larger, more complex model that includes the missing causal features. But such a model would have to be highly specific to the circuit in question and would thus have no general applicability.
Instead a different procedure is followed. Measurements of the relevant parameters are made on the actual circuit under study, and then the measured values rather than the theoretically predicted values are used for further calculations in the original models. To illustrate, consider an actual amplifier, built and tested such that
I
E =1 ma;
R
L= 2.7

15 = 2.3 k ohm;
R
1= 1 k ohm. β = 162 and
R
S= 1 k ohm and assume
r
b= 50 ohms. The theoretical expectation of midband gain is
- (6.3)
-
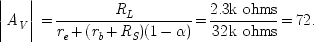
The actual measured midband gain for this amplifier with an output voltage at
f = 2 kHz and source voltage at 1.8 mv and 2 kHz, is
- (6.4)
-
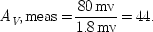
This result is not even close to what theory predicts. This fact is explained causally by considering two features of the situation: first, the inaccuracy of the transistor model due to some undiagnosed combination of causal factors; and second, a specifically diagnosed omission—the omission of equivalent series resistance in the bypass capacitor. The first inaccuracy involves the value assigned to r
eby theory. In theory, r
e= kT/qI
E , which is approximately 25.9/I
E . The actual measurements indicate that the constant of proportionality for this type of transistor is 30 mv, so r
e= 30/I
E , not 25.9/I
E .
Secondly, the series resistance is omitted from the ideal capacitor. But real electrolytic capacitors are not ideal. There is leakage of current in the electrolyte, and this can be modelled by a resistance in series with the capacitor. This series resistance is often between 1 and 10 ohms, sometimes as high as 25 ohms. It is fairly constant at low frequency but
end p.109
increases with increased frequency and also with increased temperature. In this specific case, the series resistance,
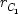
, is measured as 12 ohms.
We must now modify (
6.3) to account for these features, given our measured result in (
6.4) of
A
V , meas. = 44:
- (6.5)
-

Solving this equation gives us a predicted midband gain of 47.5, which is sufficiently close to the measured midband gain for most purposes.
Let us now look back to see that this procedure is very different from what the generic-specific account supposes. We start with a general abstract equation, (
6.3); make some approximations; and end with (
6.5), which gives rise to detailed phenomenological predictions. Thus superficially it may look like a D-N explanation of the facts predicted. But unlike the covering laws of D-N explanations, (
6.3) as it stands is not an equation that really describes the circuits to which it is applied. (
6.3) is refined by accounting for the specific causal features of each individual situation to form an equation like (
6.5). (
6.5) gives rise to accurate predictions, whereas a rigorous solution to (
6.3) would be dramatically mistaken.
But one might object: isn't the circuit model, with no resistance added in, just an idealization? And what harm is that? I agree that the circuit model is a very good example of one thing we typically mean by the term
idealization. But how can that help the defender of fundamental laws? Most philosophers have made their peace with idealizations: after all, we have been using them in mathematical physics for well over two thousand years. Aristotle in proving that the rainbow is no greater than a semi-circle in
Meterologica III. 5 not only treats the sun as a point, but in a blatant falsehood puts the sun and the reflecting medium (and hence the rainbow itself) the same distance from the observer. Today we still make the same kinds of idealizations in our celestial theories.
7 Nevertheless, we have managed to discover
end p.110
or setting γ ≡ 2π
g2(ω
eg ) 𝓓 (ω
eg ),
and finally,
But in moving so quickly we lose the Lamb shift—a small displacement in the energy levels discovered by Willis Lamb and R. C. Retherford in 1947. It will pay to do the integrals in the opposite order. In this case, we note that
c
e (
t) is itself slowly varying compared to the rapid oscillations from the exponential, and so it can be factored out of the
t′ integral, and the upper limit of that integral can be extended to infinity. Notice that the extension of the
t′ limit is very similar to the Markov approximation already described, and the rationale is similar. We get
or, setting γ ≡ 2π
g2(ω
eg )𝓓(ω
eg ) and
end p.116
(𝓟(
x) = principal part of
x.)
Here Δω is the Lamb shift. The second method, which results in a Lamb shift as well as the line-broadening γ, is what is usually now called 'the Weisskopf-Wigner' method.
We can try to be more formal and avoid approximation altogether. The obvious way to proceed is to evaluate the Laplace transform, which turns out to be
then
To solve this equation, the integrand must be defined on the first and second Riemann sheets. The method is described clearly in Goldberger and Watson's text on collision theory.
12
The primary contribution will come from a simple pole Δ

such that
This term will give us the exponential we want:
But this is not an exact solution. As we distort the contour on the Riemann sheets, we cross other poles which we have not yet considered. We have also neglected the integral around the final contour itself. Goldberger and Watson calculate that this last integral contributes a term proportional to

. They expect that the other poles will add only
end p.117
negligible contributions as well, so that the exact answer will be a close approximation to the exponential law we are seeking; a close approximation, but still only an approximation. If a pure exponential law is to be derived, we had better take our approximations as improvements on the initial Schroedinger equation, and not departures from the truth.
Is there no experimental test that tells which is right? Is decay really exponential, or is the theory correct in predicting departures from the exponential law when
t gets large? There was a rash of tests of the experimental decay law in the middle 1970s, spurred by E. T. Jaynes's 'neo-classical' theory of the interaction of matter with the electromagnetic field, a theory that did surprisingly well at treating what before had been thought to be pure quantum phenomena. But these tests were primarily concerned with Jaynes's claim that decay rates would depend on the occupation level of the initial state. The experiments had no bearing on the question of very long time decay behaviour. This is indeed a very difficult question to test. Rolf Winter
13 has experimented on the decay of Mn
56 up to 34 half-lives, and D. K. Butt and A. R. Wilson on the alpha decay of radon for over 40 half-lives.
14 But, as Winter remarks, these lengths of time, which for ordinary purposes are quite long, are not relevant to the differences I have been discussing, since 'for the radioactive decay of Mn
56. . . non-exponential effects should not occur before roughly 200 half-lives. In this example, as with all the usual radioactive decay materials, nothing observable should be left long before the end of the exponential region'.
15 In short, as we read in a 1977 review article by A. Pais, 'experimental situations in which such deviations play a role have not been found to date.'
16 The times before the differences emerge are just too long.
end p.118
2. Approximations not Dictated by the Facts
Again, I will illustrate with two examples. The examples show how the correct approximation procedure can be undetermined by the facts. Both are cases in which the very same procedure, justified by exactly the same factual claims, gives different results depending on when we apply it: the same approximation applied at different points in the derivation yields two different incompatible predictions. I think this is typical of derivation throughout physics; but in order to avoid spending too much space laying out the details of different cases, I will illustrate with two related phenomena: (a) the Lamb shift in the excited state of a single two-level atom; and (b) the Lamb shift in the ground state of the atom.
2.1. The Lamb Shift in the Excited State
Consider again spontaneous emission from a two-level atom. The traditional way of treating exponential decay derives from the classic paper of V. Weisskopf and Eugene Wigner in 1930, which I described in the
previous section. In its present form the Weisskopf-Wigner method makes three important approximations: (1) the rotating wave approximation; (2) the replacement of a sum by an integral over the modes of the electromagnetic field and the factoring out of terms that vary slowly in the frequency; and (3) factoring out a slowly-varying term from the time integral and extending the limit on the integral to infinity. I will discuss the first approximation below when we come to consider the level shift in the ground state. The second and third are familiar from the last section. Here I want to concentrate on how they affect the Lamb shift in the excited state.
Both approximations are justified by appealing to the physical characteristics of the atom-field pair. The second approximation is reasonable because the modes of the field are supposed to form a near continuum; that is, there is a very large number of very closely spaced modes. This allows us to replace the sum by an integral. The integral is over a product of the coupling constant as a function of the frequency, ω, and a term of the form exp(−iωt). The coupling
end p.119
constant depends on the interaction potential for the atom and the field, and it is supposed to be relatively constant in ω compared to the rapidly oscillating exponential. Hence it can be factored outside the integral with little loss of accuracy. The third approximation is similarly justified by the circumstances.
What is important is that, although each procedure is separately rationalized by appealing to facts about the atom and the field, it makes a difference in what order they are applied. This is just what we saw in the last section. If we begin with the third approximation, and perform the t integral before we use the second approximation to evaluate the sum over the modes, we predict a Lamb shift in the excited state. If we do the approximations and take the integrals in the reverse order—which is essentially what Weisskopf and Wigner did in their original paper—we lose the Lamb shift. The facts that we cite justify both procedures, but the facts do not tell us in what order to apply them. There is nothing about the physical situation that indicates which order is right other than the fact to be derived: a Lamb shift is observed, so we had best do first (3), then (2). Given all the facts with which we start about the atom and the field and about their interactions, the Lamb shift for the excited state fits the fundamental quantum equations. But we do not derive it from them.
One may object that we are not really using the same approximation in different orders; for, applied at different points, the same technique does not produce the same approximation. True, the coefficients in t and in ω are slowly varying, and factoring them out of the integrals results in only a small error. But the exact size of the error depends on the order in which the integrations are taken. The order that takes first the t integral and then the ω integral is clearly preferable, because it reduces the error.
Two remarks are to be made about this objection. Both have to do with how approximations work in practice. First, in this case it looks practicable to try to calculate and to compare the amounts of error introduced by the two approximations. But often it is practically impossible to decide which of two procedures will lead to more accurate
end p.120

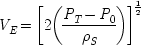

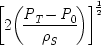

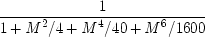
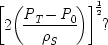


 15 = 2.3 k ohm; R
1= 1 k ohm. β = 162 and R
S= 1 k ohm and assume r
b= 50 ohms. The theoretical expectation of midband gain is
15 = 2.3 k ohm; R
1= 1 k ohm. β = 162 and R
S= 1 k ohm and assume r
b= 50 ohms. The theoretical expectation of midband gain is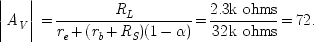
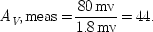
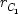 , is measured as 12 ohms.
, is measured as 12 ohms.

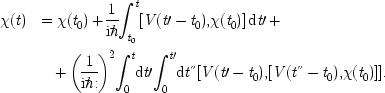
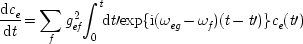
 , for E
ethe energy of the excited state, E
gthe energy of the de-excited; ω
fis the frequency of the fth mode of the field; and g
efis the coupling constant between the excited state and the fth mode. c
eis the amplitude in the excited state, no photons present.)
, for E
ethe energy of the excited state, E
gthe energy of the de-excited; ω
fis the frequency of the fth mode of the field; and g
efis the coupling constant between the excited state and the fth mode. c
eis the amplitude in the excited state, no photons present.)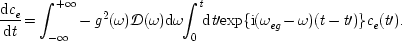

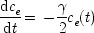
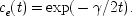


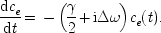

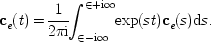
 such that
such that

 . They expect that the other poles will add only
. They expect that the other poles will add only  rather than
rather than
 . This additional imaginary term, iω, appears when the integrals are done in the reverse order, and it is this term that represents the Lamb shift. But what difference does this term make? In the most immediate application, for calculating decay probabilities, it is completely irrelevant, for the probability is obtained by multiplying together the amplitude
. This additional imaginary term, iω, appears when the integrals are done in the reverse order, and it is this term that represents the Lamb shift. But what difference does this term make? In the most immediate application, for calculating decay probabilities, it is completely irrelevant, for the probability is obtained by multiplying together the amplitude
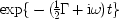 , and its complex conjugate
, and its complex conjugate
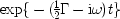 , in which case the imaginary part disappears and we are left with the well-known probability for exponential decay, exp(−Γt).
, in which case the imaginary part disappears and we are left with the well-known probability for exponential decay, exp(−Γt).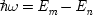 , for energies levels E
mand E
nof the atom; ω
kis a mode frequency of the field). Energy conserving transitions vary as exp{±i(ω−ω
k )t}. For optical frequencies ω
kis large. Thus for ordinary times of observation the exp{±i(ω+ω
k )t} terms oscillate rapidly, and will average approximately to zero. The approximation is called a 'rotating-wave' approximation because it retains only terms in which the atom and field waves 'rotate together'.
, for energies levels E
mand E
nof the atom; ω
kis a mode frequency of the field). Energy conserving transitions vary as exp{±i(ω−ω
k )t}. For optical frequencies ω
kis large. Thus for ordinary times of observation the exp{±i(ω+ω
k )t} terms oscillate rapidly, and will average approximately to zero. The approximation is called a 'rotating-wave' approximation because it retains only terms in which the atom and field waves 'rotate together'.