2. Regularities and Rules: A Genealogical Account
The account I shall sketch below, in the hope of capturing some of Wittgenstein’s central ideas, should solve the two problems above, by answering the question posed at the outset: What is it for a proposition to be a proposition of mathematics? The account has a genealogical character: what elucidates the nature of such propositions is their origin—and, because of this origin, they can be put to a certain use. If convincing, the account should also be able to give more than a hint as to how Wittgenstein deflects the skeptical doubt about (the necessity of) mathematical truths.
The account consists of two components, each of them deserving to be called ‘naturalist’. (I do not have a definition of naturalism; my hope is that in the end it will be unquestionable that the label fits.) The first component has to do with certain methodological aspects Wittgenstein deploys when reflecting on mathematics (unsurprisingly, it is the same methodology he uses in Philosophical Investigations, and everywhere else in his later philosophy.) The second element concentrates on the abovementioned relation between the mathematical statements and ‘experience’. The term of art Wittgenstein introduces here is quite suggestive; he talks about ‘hardening’ of empirical propositions into rules in RFM, VI-22. I will examine this ‘hardening’ process, and of some help here will be a legal analogy, suggested by Wittgenstein himself in RFM I-116, between (the rules of) mathematics and (the laws of) jurisprudence. The key-idea is that “custom is a source of law”, a locution common in legal theory.23 This passage from Raz is illustrative for my train of thought in sect. 2.2 and 3:
Of course, the word ‘law’ designates, among other things, rules or norms, and the concept of a rule probably emerged from a concept of a law which did not separate natural law from customary practices, nor either of them from a normative law.
(My emphasis)24
2.1 Methodology
A central (but neglected) aspect of Wittgenstein’s philosophy of mathematics is his strategy to treat the concepts and notions typically considered mathematical technicalities from the perspective of their role in ordinary, everyday language:
An important problem arises from the subject itself: How can I—or anyone who is not a mathematician—talk about this? What right has a philosopher to talk about mathematics? […] I can as a philosopher talk about mathematics because I will only deal with puzzles which arise from the words of our ordinary everyday language, such as ‘proof’, ‘number’, ‘series’, ‘order’, etc. Knowing our everyday language—this is one reason why I can talk about them.25
This perspective legitimizes the authority of his entire enterprise (once again). But it also makes possible to apply the method he introduced in the well-known family resemblance passages in the Investigations §65– 71. Thus, when analyzing ordinary language notions, a philosopher’s concern is to “look and see”, not to ‘think’26—so it is similar to that of a naturalist painter: not to distort.27 And what Wittgenstein notices when he looks into the employment of such natural language concepts is a challenge to what has been called ‘intellectualism’: Language users are unable to identify comprehensive, essentialist definitions of many concepts (and ‘game’ is the famous example here; not accidentally, ‘number’ is another). This is usually taken as an attack on essentialism, but some commentators suggested that this is only a secondary goal for Wittgenstein here,28 if at all.29 In fact, what he wants is to ask “a question about a human being”,30 lending itself to an unequivocal, and philosophically illuminating anti-intellectualist answer, that “everyone admits”: when we use the word ‘game’, are we able to give a definition of it? The answer is ‘no’, the definition—of ‘game’, ‘number’, etc.—eludes us; despite this, we are typically able to (learn to) apply these concepts correctly.
Then, it is not surprising that both RFM and LFM are pervaded with the same kind of question, ‘about a human being’. The key-move is, once again, to “look at what happens”31 when we deal with arithmetic, and to ask questions about human abilities and practices, answerable by drawing on what is in front of our eyes—as opposed to ‘hard’ mathematical (calculational, technical) questions. During a discussion with Turing, recounted in the LFM, Wittgenstein emphasized this methodological point, about the kind of questions he poses:
I did not ask, ‘How many numerals are there?’ This is immensely important. I asked a question about a human being, namely, ‘How many numerals did you learn to write down?’32
So, what does Wittgenstein see when he looks at arithmetic from this perspective? What first stands out is how seriously we take it, how central and important it is for us. Arithmetical calculations are not esoteric matters, technicalities debated among experts; nor are they mere hobbies, unserious pursuits. They are deeply embedded in our life, and ubiquitous.33
For what we call ‘counting’ is an important part of our life’s activities. Counting and calculating are not—e.g. simply a pastime. Counting (and that means: counting like this) is a technique that is employed daily in the most various operations of our lives. And that is why we learn to count as we do: with endless practice, with merciless exactitude; that is why it is inexorably insisted that we shall all say ‘two’ after ‘one’, ‘three’ after ‘two’ and so on.34
The other salient aspect is the silence, peace, the muted and deep agreement among people when dealing with mathematical issues. In this sense—to avoid contradicting the remark above—even disinterest and neglect: “We acknowledge it [a mathematical proposition] by turning our back on it”.35 We are also reminded that
We have all of us worked out certain multiplications. And actually there are no disagreements about the result of a multiplication—so that we don’t know what to believe because we always have a headache, or all the people get different results. This hasn’t happened; that is immensely important.36
That there is hardly ever any discrepancy between ways of counting, and when there is we are able to clear it up usually, is of immense importance.37
Both these evident aspects—seriousness (‘merciless exactitude’) and nonlitigiousness (‘no disagreements’)—will be relevant in the discussion below.
2.2 Hardening
Going back to our ‘prior’ question, let us address it from the methodological perspective sketched above. To summarize what I will argue next: According to Wittgenstein, if we look at the propositions of mathematics, we see no intrinsic feature of them, something about their content, that makes them necessary.38 Instead, what qualifies a proposition as a ‘mathematical’ (necessary) one is a certain extrinsic feature, an “attitude”39 we have toward it (and toward the technique, or procedure, establishing it). To flesh out a more complete story, we need first to recall the proposal from LFM, 95 already cited above:
Mathematical propositions are […] propositions dependent on experience but made independent of it.
This echoes points made in several previous lectures. In Lecture IV, we are told that a mathematical proposition is:
Independent of experience because nothing which happens will ever make us call it [25 × 25 = 625] false or give it up.40
Dependent on experience because you wouldn’t use this calculation if things were different. The proof of it is only called a proof because it gives results which are useful in experience.41
Moreover,
All the calculi in mathematics have been invented to suit experience and then made independent of experience.42
[25 × 25 = 625] was first introduced because of experience. But now we have made it independent of experience; it is a rule of expression for talking about our experiences.43
We also need to recall here RFM IV-21, where Wittgenstein explicitly connects the descriptive (law-like regularities, holding in ‘experience’) with the normative (rules):
The twofold character of the mathematical proposition—as law and as rule.
(‘Law’ here has a descriptive sense, while ‘rule’ has a normative meaning. This brings us back to the duality44 I signaled above, and this notion will help solve the first problem, the truth-value assignment). On the descriptive side, there are no special difficulties. We all know that some things happen in a regular, law-like fashion while others just do not. It is an empirical regularity that pebbles always fall downwards when left unsupported in the air—and also that they don’t suddenly disintegrate or multiply when laid down on the ground to be counted. Similarly, it is a psychological regularity that a vast majority of people behave in (roughly) the same way when experiencing similar circumstances (e.g., when hurt, when surprised), and even most surely so when they receive the same kind of persistent, exacting, ‘merciless’ training—in counting pebbles, for instance. Here we should note that ‘training’ is, in many of Wittgenstein’s employments e.g., in PI §5, a translation of the German word ‘Abrichtung’, used only when one talks about animals! Some of these regular reactions may have an innate basis, and many are induced by training-abrichtung; either way, “everyone admits” that such regularities exist. Now, what about the normative side?
A good way to explain the emergence of normativity is through a little story. Once upon a time there was a kingdom where people knew how to count up to 1000, but they had not yet invented multiplication.45 (Moreover, these people never cared about concepts such as ‘necessity’ and ‘possibility’; we can even speculate that this describes a period of the actual human history.) But one day, the Queen laid down 25 batches of 25 pebbles each on the shiny floor of her palace, and kept counting them carefully every morning:

Figure 7.1
The Queen’s pebbles
She reached 625 almost always, 626 once, and 624 on a couple of occasions. She then decided to ask the royal court to do the counting too. All of them reached 625, even the King; one duke, however, got 619. (The Queen noticed that he was a bit tipsy when he counted, so she decided to dismiss this result.) Another (sober) subject got 626, and the Bishop got 624. She then used her royal prerogatives and ordered everyone (aristocrats, merchants and peasants) to arrange pebbles on the ground and start counting. An overwhelming majority, of the order of tens of thousands, reported 625, but three people, working independently, found the result to be exactly 601. This coincidence caught the Queen’s attention— how can this be?! It turned out that these people understood the order ‘put 25 batches of 25 pebbles each on the ground and count them’ in this way46 (Figure 7.2):
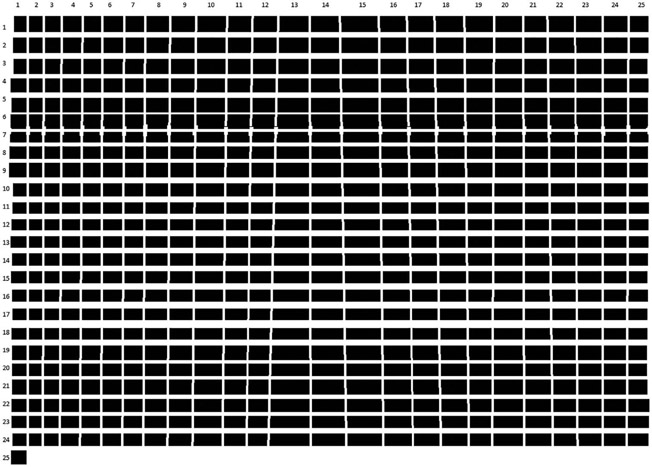
Figure 7.2
An arrangement of 25 batches of 25 pebbles each, i.e., 24 horizontal batches and one vertical.
However, when told that their procedure was not the same as the others, they did it again and two of them got 625. One of them still insisted that what he did was right, and so the result is 601. Yet, in the end, he gave up when the Queen, too tired to argue, threatened to exile him. After taking all the reports into account, the Queen and virtually everyone else came to believe that the result was the ‘peak’ value of 625 pebbles.
The point of this story is to illustrate how certain (arithmetically-sounding) beliefs—expressed as descriptive propositions, e.g.,’25 batches of 25 pebbles each is 625 pebbles’—may emerge. They have the following characteristics:
- (i) are ‘dependent on experience’47 (verifiable empirically, quickly convergent, stable) and, in the end, peacefully accepted by (virtually) everyone.
- (ii) it is considered important to settle them, and thus the (extremely few) dissenters are taken seriously and silenced if necessary.
On this royal road to normativity the next thing to note is that beliefs like these form a set of propositions which serves, in the hands of a more practically-inclined community (or Queen), as the pool of candidates to fulfill the role of basic rules needed to implement social uniformity— once the community finds desirable to ensure it (e.g., to avoid protracted legal negotiations, to facilitate commerce, etc.). Propositions like the one above—recall, originally dependent on experience, (almost) universally accepted as true—are now made independent of experience. They are made so by the community, most likely gradually and not by a completely transparent and explicit decision.
‘Independence’ means that no empirical facts, or procedures will be recognized (explicitly, by the community) as capable of challenging these propositions. Thus, one may say that such an empirical proposition has become harder to overthrow. But this is not strong enough; in fact, it has become meaningless to even try to do so—to make a proposition independent of experience is to change its status, to look at it in a different light: not as a (descriptive) statement, but as a (normative) rule. A monetary analogy may be useful to understand what happens here. If I give you a 1$ bill (currently in circulation) and tomorrow you give me another 1$ bill (in circulation), this is (i) a neutral, monetary transaction, and (ii) a non-commercial one: we just exchange money, neutrally, and without selling or buying anything. But, if in a second transaction, tomorrow you give me a 1$ bill issued in 1869 (i.e., not in circulation) for a current 1$ bill I gave you today, this transaction is not neutral; it is not even a monetary one. Moreover, there is no definite amount of current dollars I should give you to make it so. This is not a monetary transaction because I give you money, but you give me an object (an old piece of paper, that once had the role of money). So, we don’t exchange money for money, exchange which, if it were one current dollar for another current dollar, it would have been ‘monetary’ (and ‘neutral’—because, if you give me two current dollars tomorrow, neutrality is lost, since this amounts to lending and borrowing, at an astonishing interest rate!) This leads to (ii). Our second transaction above is, after all, a commercial one. In essence, I buy from you (i.e., give you money for) an object, an old piece of paper; and, as it happens, it is a pretty bad transaction for you, since a 1$ bill issued in 1869 would cost a collector today significantly more than one current dollar! Regardless, we have now entered the commercial realm.
To return to the analogy with the propositions of mathematics, they are much like the dollar bills issued in 1869. They look like empirical/descriptive, truth-valuable ones (dollars in use), but are not so. This old bill per se will not buy you anything today (say, if you put it in a vending machine). The lack of purchase power stands for the impossibility to refute a mathematical proposition by invoking empirical matters. The question “how many empirical facts do I need to show you in order to renounce a mathematical proposition?” has no sensible answer, just as “how many current dollars should I ask someone who wants to exchange , monetarily and neutrally, a 1$ bill not in use?” does not have one. (Again, the latter question is not: “How many current dollars should I ask a collector to pay me for the very old 1$ bill I found in my great-grand father’s attic?”)
Making rules involves instituting restrictions about what people are allowed to do, i.e., the techniques and procedures they employ. If an individual insists on getting recognition for the technique leading from “25 groups of 25 objects each” to “601 objects”, the community has to react by taking exclusionary measures against that individual. As Figure 7.2 shows, what he did “can be made out to accord with the rule”, as PI §201 warns us. He can’t be proved wrong by pointing out that he counted some pebbles twice, since there are situations when we do this: we say that the equation x2 – 6x + 9 = 0 has two roots, so we count the root ‘+3’ twice (LFM, 156); we also talk about the Christian Trinity, etc.
Thus, before instituting the rules the community was inclined to take such punitive measures as a matter of course (the Queen thought that some of the reported results should be dismissed.). But after this happened such measures are taken with merciless regularity; the community has to take them, as it sees itself entitled to do so, since everyone was warned in advance, and trained in arithmetic, abrichtung-style. While social exclusion-type measures are taken both before and after setting the rules, they have a different nature in the two cases: de facto and de jure.
RFM VI-22, perhaps the most important paragraph in all of Wittgenstein’s later philosophy of mathematics, gives us the ‘hardening’ notion:
The justification of the proposition 25 × 25 = 625 is, naturally, that if anyone has been trained in such-and-such a way, then under normal circumstances he gets 625 as the result of multiplying 25 by 25. But the arithmetical proposition does not assert that. It is so to speak an empirical proposition hardened into a rule. It stipulates that the rule has been followed only when that is the result of the multiplication. It is thus withdrawn from being checked by experience, but now serves as a paradigm for judging experience.
(Bold type added.)
Commenting on this passage, Fogelin writes:
It is not altogether clear what Wittgenstein is saying here […] Here we can imagine someone saying, ‘Wittgenstein has things backward. It is the recognized necessity of 25 × 25 = 625 that leads us to adopt it as a rule, and not its adoption as a rule that makes it necessary’. Wittgenstein sees the force of this primitive complaint, and does not think that it can easily be swept aside.48
The complaint imagined by Fogelin captures the strong temptation to think of necessity in what I called intrinsic terms (as located in the proposition itself). Wittgenstein’s move here is exactly as Fogelin describes it (his initial hesitation notwithstanding): Namely, to advance the opposite thought, that it is extrinsic. Nevertheless, although he identifies the move correctly, I am afraid he misses the very reason why Wittgenstein makes it (as his hesitation, and the rest of the commentary, show): The rule’s special origin, a key-idea to which I now turn.
We are now closer to seeing why the account of normativity is ‘genealogical’—and also ‘naturalist’. We are thus in the position to address the second difficulty introduced above: Are the rules arbitrary, mere conventions, changeable at will? Is mathematical objectivity undermined?
It is the origin, or genealogy of an arithmetical proposition/rule that qualifies it to be called ‘necessary’—it has to be the result of hardening. Here, 25 × 25 = 625 originates in the one above, “(almost) everyone finds that 25 batches of 25 pebbles each is 625 pebbles”. Before I elaborate, let us note that, importantly, not all rules are like that. A comparison with Hacker’s rule of chess above will be edifying here. The rule “the king moves one square at a time” does not have this kind of origin—so, in this sense, it is completely arbitrary, a mere convention we have to accept if, and only if we want to play chess. This rule cannot be traced back to any regularity of the kind presented in the story above. In short, and crucially, this rule does not originate as a ‘peak’ result of an ‘experiment’, and thus it is not generated by hardening.49 To challenge, or reject it is not a serious, important matter: to do so is just to refuse to play chess. Challenging it may even be seen as constructive, and not deviant. One may argue that a version of chess in which the king is allowed to move, say, three squares at a time is more entertaining (and, after all, the rules of chess have changed throughout history.)
So, what is it for a proposition to be a proposition of mathematics? I have sketched here a two-stage genealogical account. What separates them is the ‘hardening’ moment. At the first stage, the following regularity holds as a matter of fact: an overwhelming majority of people does certain things in a certain way, i.e., uses the arrangement in Figure 7.1, and reports the same result, 625. In a nutshell, the first stage embodies the observation that ‘peaks appear’. But note that these are contingent facts: namely, that such convergence of technique and results exist and that they are pervasive (there is obviously nothing special about this multiplication).50 Wittgenstein’s philosophy of necessity does not attempt any ‘explanation’ of their occurrence.51 At this stage, there are no multiplication rules, no ‘mathematics’, only stable patterns of behavior: ‘(almost) everyone gets 625’. And, of course, “the arithmetical proposition does not assert that”— that, i.e., that ‘everyone gets 625’. This is so indeed; this is a report of what happens, an empirical proposition, not an arithmetical one.52
Thus, at the first stage we don’t have any arithmetic, only stable behavioral patterns (‘peaks’) which, importantly, include another social habit: communities tend to exclude the deviants (when the matters are perceived as serious, important). These kinds of stabilities encountered at this first, pre-hardening stage, may be, as we saw, extremely useful socially, and the community may be interested in re-enforcing them. Thus, we move on to a second stage, when explicit rules are introduced.
But we now know where these needed rules come from. Crucially, they are not mere inventions, figments of one’s (the Queen’s, or the community’s) imagination, i.e., arbitrary, random, whimsical. The temptation (among some commentators) to say that for Wittgenstein the mathematical propositions are established ‘by convention’ is understandable. Yet, when confronted with LFM, 107, there is little point in saying this:
Mathematical truth isn’t established by their all agreeing that it’s true—as if they were witnesses of it. Because they all agree in what they do, we lay it down as a rule, and put it in the archives. Not until we do that have we got to mathematics. One of the main reasons for adopting this as a standard, is that it’s the natural way to do it, the natural way to go—for all these people.
Neither the community, nor the Queen just decrees that the result is 625—this peak first appears in practice. So, we are dealing here with very special conventions: what is stipulated (perhaps never explicitly!) is that we change our attitude toward the empirical proposition ‘everyone gets 625’. This is not just any proposition, but an already maximally stable one (the result of applying an unanimously accepted technique).53 It is this proposition, which satisfies (i)-(ii) above, that is hardened into a norm, or rule: ‘Get 625 when multiply 25 and 25!’
Thus, the rules at the second stage are natural, being generated from (extremely stable) human habits, from the class of the accepted empirical propositions identified at the first stage. Since the propositions-regularities in this class have very large inertia, the rules they engender inherit this inertia. The objectivity of mathematics is thus preserved, since the rules, too, are stable, fixed; new rules cannot be introduced on a whim, and existing ones cannot be changed as one wishes (unless, presumably, one modifies their habitual basis, i.e., the whole form of life to begin with).54
Recall, moreover, that hardening is an extrinsic process; the result of the community’s change in attitude toward a set of empirical propositions— and not intrinsic, the result of recognizing something unassailable in the content of these propositions themselves.55 Thus, what happens is that certain empirical propositions (universally and peacefully accepted declaratives, descriptions of ‘experimental’ peaks) become infinitely ‘hard’ to overthrow, i.e., become ‘grammatical’56 (paradigms, objects of comparison, prescriptions, imperatives to be enforced—firmly, through Abrichtung). RFM VII-67 reads as follows:
We say: ‘If you really follow the rule in multiplying, you must all get the same result’ […] This is […] the expression of an attitude towards the technique of calculation, which comes out everywhere in our life. The emphasis of the must corresponds only to the inexorableness of this attitude both to the technique of calculating and to a host of related techniques.
Given their origin, the rules themselves are touched by contingency in two ways. Thus, one’s hopes for an absolutely secure account of necessity are illusory. First, the existence of the pre-hardening, convergence stage (the existence of peaks) is, as mentioned, a natural fact, which might not have obtained. Moreover, the hardening itself, i.e., the transition from the pre- to the post-hardening (convention-instituting) stage, might not have happened either: there might not have been any felt need to institute rules—although Wittgenstein once remarked (RFM I-74) that we have a “deep need” for conventions. Yet, the disappointment should not become despair, since contingency may not be such a major threat after all. We live in a world in which peaks do emerge, and the transition does happen: were this not the case—imagine there was no peak, but a whole range of results of 25 × 25 was reported (23, 19, 983, 77, etc.); or imagine that more than one peak appeared (e.g., 625 and 601)—we would not have the ‘mathematics’ as we know it today.
To make this thought more precise, let me make clear that the present account allows the possibility of some radically different, alien community or species whose experimental peaks, when faced with the rows of pebbles, are different from ours. They may converge and ‘peak’ on the answer 1250, say, because, due to some bizarre physical law, a pebble doubles itself instantly when one counts it. (There are similar stories in Wittgenstein’s writings. Recall footnote 47.) This could then be ‘hardened’ into the rule 25 × 25 = 1250. This alien community would likewise not be able to alter this rule on a whim or at will—to do so, they would have to fundamentally change their whole form of life. Just like ours, this rule would not be arbitrary, but the natural result of the empirical regularities holding in that region of the Universe. If one now asks what resources the present account has for saying that this sort of alien mathematical community would be objectively wrong, then the answer is simply—none. But, importantly, this should not worry us. Asking to establish this is to ask for too much; moreover, the problem we seem to face may not occur in the first place. For one thing, if the aliens are so different from ‘us’, in so many fundamental respects, even if we may wish to present some arguments to them to this effect, there would be no way to say whether they understand them. (By arguments, I mean more substantial reasons than to say ‘look, in the platonic heaven the result is 625, not 1250’.) For another, given the deep chasm between the two forms of life, there would presumably be no way to decide even more basic things, such as whether they actually understand ‘counting’, ‘equal’, ‘different’, ‘row’, etc., the same way we do. To push things even further, their form of life may not even contain the language game of asking for, and giving, reasons.
Back to our world, once hardening is completed we can begin to talk about necessity. These ‘hardened’ propositions constitute the framework within which we now perform legitimate inferences (like the ones Hacker mentioned above) between regular, empirical propositions: From “there are 12 groups of 12 soldiers each in the parade”, we are entitled to move to “there are 144 soldiers in the parade”. Crucially, these are moves (‘inferences’) which a very large majority of us makes anyway. What is left unhardened is (called) ‘possible’, and is decidable by ‘experiment’. Thus, we can talk about necessity only after the transition between the two poles Wittgenstein speaks in RFM VII-30 has been completed: “It might be said: experiment—calculation are poles between which human activities move”.
Returning to the fundamental problem posed at the very beginning, we now see that the equation 25 × 25 = 625 lacks a truth-value in a strict sense (is a rule). Yet it has one (is true), in a derivative sense. What is true is the statement M: “Someone follows the order multiply 25 and 25 when they get 625”. Hence, if a child gets some other result, say 601, we must say that they only thought they multiplied these numbers, or that they thought they followed the rule, when in fact they did not. (Or, more charitably, that they intended to follow the order to multiply, but failed— regardless of how ‘certain’ they have felt, or what neural mechanisms fired up in their brains.) Moreover, there is nothing wrong with saying that we have mathematical beliefs. To believe that 25 times 25 is 625 is just to believe proposition M above. And we don’t say that the multiplication order was properly followed as a result of some arbitrary decision, but on the ground of the natural, empirical regularity (i.e., because of the agreement in following the procedure in Figure 7.1, as opposed to some other procedure).
Finally, ‘must’ means something social too: that abrichtung-style measures are implemented to ensure that everyone follows the rule. They make us ‘blind’ to all the virtually infinite possibilities to deviate, one such possibility being exemplified by Figure 7.2. (Note also, in passing, that this is what ‘blind’ means in “I follow the rule blindly” in PI §219).57 For the few ones who are not capable to become ‘blind’ in this way, or refuse to, the consequences are serious, ranging from not graduating elementary school to being locked up in the psychiatric ward.