
GENERAL THEORY OF RELATIVITY
GENERAL THEORY OF RELATIVITY
GLOSSARY
absolute elsewhere Because light speed is an absolute speed limit, if one thing is to influence another, it must be possible for a beam of light to get from the first thing to the second in the available time. For example, imagine a bomb two light years from Earth that can be deactivated by laser. The bomb is set to detonate in one year’s time. This makes it impossible to deactivate the bomb before it explodes. In such a circumstance, the explosion is in the ‘absolute elsewhere’ of the Earth. The absolute elsewhere for an event is the region of spacetime that light cannot reach, so there can never be a causal connection between the initial event and that point in spacetime.
arrow of time Spacetime makes time another dimension alongside the three spatial dimensions. But time is different. It has a clear direction pointing from past to future, the arrow of time. Many physical processes are reversible – they would run in either time direction. But the second law of thermodynamics, which says that entropy in a closed system (the level of disorder in the system) stays the same or rises, provides a clear arrow of time that explains why, for instance, you can’t un-mix milk from a cup of tea.
black hole The result of an ageing star collapsing in on itself to the extent that nothing can resist the collapse and the entire star ends up as a dimensionless point. Black holes were one of the first predictions from Einstein’s general theory of relativity, and though they have never been directly observed, there is good indirect evidence for their existence. Get close enough to a black hole and spacetime warps so much that even light cannot get out.
calculus Independently developed by Newton and Leibniz, calculus is a mathematical tool for analyzing change in two broad forms: differential calculus covers rates of change and relative change, while integral calculus can be used to calculate sums across ranges of values and the area, volume, etc. of geometric shapes. Calculus is central to physics and widely applicable when numbers vary systematically.
differential equations Differential equations link something to its derivatives, which are the results of applying differential calculus. They typically express the rate at which something is changing, or how one variable quantity varies with respect to another.
general covariance Physicists prefer physical laws to be generally covariant – meaning that, for instance, however you move compared to the system being studied, the laws are unchanged. This is not the case for special relativity, as it only applies in ‘inertial frames of reference’ – when the system being observed is in steady motion compared with the observer. General relativity takes away the need for a particular reference frame, so has general convariance.
matrix A two-dimensional array of numbers or other mathematical items, which can be manipulated using special operations to undergo arithmetical procedures like addition and multiplication.
spacetime In special relativity, Einstein showed a relationship between space and time that made it impossible to regard each separately, making it useful to consider a four-dimensional entity comprising three dimensions of space and another of time. For general relativity, Einstein then had to consider that objects with mass warped spacetime, requiring an analysis of the curvature in spacetime.
tensor calculus Calculus was modified in the 19th century to handle changes in parts of a complex equation (partial differentiation) and changes in vector fields, where each point in a multidimensional space can have both a value and direction (vector calculus). Even this was not enough for general relativity, for which Einstein had to employ tensor calculus. Tensors are mathematical structures, usually in the form of a matrix, that show how different vectors and numbers relate to each other, and tensor calculus applies to a field in which each point in multidimensional space has an associated tensor.
BREAKING INERTIAL FRAMES
the 30-second theory
Special relativity can describe situations involving objects that are either stationary or moving in a straight line at a constant speed with respect to an observer. Both the observer and the object are said to occupy inertial frames of reference. However, it is more difficult to use special relativity to describe a situation where a force is acting on an object to change its speed or direction of travel, such as an object falling in a gravitational field. The object will now be in an accelerating frame of reference. In special relativity an accelerating frame of reference must be treated differently to an inertial frame of reference. The physical equations of special relativity do not take the same form in an accelerating frame as they do in an inertial frame. By rewriting the equations of special relativity using non-linear coordinate systems it is possible to deal with an accelerating frame of reference and, for example, describe the motion of particles moving in electric and magnetic fields. However, special relativity is founded on the principle that the speed of light is constant for all observers and this is not necessarily the case when the equations of special relativity are applied to accelerating frames of reference. General relativity was born out of Einstein’s attempts to apply the principles of special relativity to acceleration and gravity.
3-SECOND THRASH
Special relativity breaks down in situations involving accelerated motion and gravity.
3-MINUTE THOUGHT
What’s in a name? Special relativity was not called ‘special relativity’ at the time when Einstein published his initial paper, entitled ‘On the electrodynamics of moving bodies’. It was only later, as Einstein developed a generalized theory of relativity to deal with the limitations of his earlier work, that the name special relativity came into use. Why? Because, as Einstein wrote, ‘special relativity applies to the special case of the absence of a gravitational field’.
3-SECOND BIOGRAPHIES
GALILEO GALILEI
1564–1642
Italian astronomer who was the first to realize that the laws of the universe should be the same everywhere – that is, in all reference frames
ISAAC NEWTON
1643–1727
English physicist whose laws of motion established the idea of inertial frames of reference
30-SECOND TEXT
Leon Clifford
To a steadily moving observer, the laws of physics are unchanged, but when accelerating this no longer holds true.

THE HAPPIEST THOUGHT
the 30-second theory
Einstein’s starting point for general relativity was what he later called his ‘happiest thought’, which came to him while working at the Swiss patent office. Einstein realized that if someone falls, say, off a high building, his or her acceleration cancels out the pull of gravity. They are weightless. (Aircraft in free fall, so-called ‘vomit Comets’, simulate this to give experience of weightlessness.) The two things – acceleration and gravity – are indistinguishable in their effects: this is the ‘equivalence principle’. The implication was that if, for instance, you were in a spaceship with no windows and it was under constant acceleration, you would be pushed towards the rear of the craft, just as we are pushed into our seats when a plane accelerates down the runway. But if the same spaceship were sitting rear end down on a planet where the gravitational pull produced the same acceleration, you would also be pulled the same way towards the rear and would not be able to distinguish from your position in the spaceship whether you were accelerating or sitting still on the planet. This equivalence between acceleration and gravity would prove essential in understanding the way that gravity is produced as a warp in space and time.
3-SECOND THRASH
In his ‘happiest thought’ Einstein realized that acceleration and gravity are indistinguishable in their effects, which would lead on to his ideas on the nature of gravity itself.
3-MINUTE THOUGHT
Einstein said ‘I was sitting in a chair in the patent office at Bern when all of a sudden a thought occurred to me: “If a person falls freely he will not feel his own weight.” I was startled. The simple thought made a deep impression on me. It impelled me toward a theory of gravitation.’ This is why astronauts in orbit float around. They are in free fall towards Earth, but also move sideways, so miss the surface.
3-SECOND BIOGRAPHIES
GALILEO GALILEI
1564–1642
Italian natural philosopher who discovered the earlier equivalence principle that steady motion is indistinguishable from being motionless
ABRAHAM PAIS
1918–2000
Dutch-American physicist and Einstein biographer who shared Einstein’s ‘happiest thought’ remark, quoting an unpublished paper
30-SECOND TEXT
Brian Clegg
The observation that a person who is falling feels no gravity, seen in vomit Comets and space stations, inspired Einstein’s ‘happiest thought’.

HEAVY CLOCKS
the 30-second theory
Einstein predicted that gravity would slow down time. To see how this works we need to imagine two people – Alice and Ben – in a rocket that is accelerating, with Alice at the front and Ben at the back. Alice and Ben send light pulses between each other, and by measuring how much time elapses between each pulse they can each measure how quickly time is passing. Suppose that Alice sends two light pulses to Ben, and that she measures a time interval between sending the two pulses. Ben receives the two pulses at the back of the rocket; but because of the rocket’s acceleration the time interval between receiving the two pulses will be less than the time interval Alice measured between sending them. Suppose Alice measures her pulses to be 2 seconds apart, Ben may measure them to be only 1 second apart. Time will appear to pass more slowly for Ben than it does for Alice: his seconds last twice as long. From the principle of equivalence, what is true for an accelerating rocket is also true for a rocket stationary in a gravitational field. If the rocket were standing vertically on the surface of a planet, Ben’s clock would tick more slowly than Alice’s, because he is in a stronger gravitational field. So, gravity slows down time.
3-SECOND THRASH
Einstein’s principle of equivalence tells us that clocks will run slower in a stronger gravitational field; so clocks on Earth run slower than clocks orbiting on the International Space Station.
3-MINUTE THOUGHT
The Global Positioning System (GPS), which we use in our sat-navs, has to take account of the fact that a clock on Earth will run slower than a clock in a satellite because it is in a stronger gravitational field. If we did not correct for this effect, the system would give us incorrect positions and would be useless.
3-SECOND BIOGRAPHY
JOSEPH HAFELE
1933–2014
American physicist who, along with his colleague Richard Keating, in 1971 flew atomic clocks aboard commercial aeroplanes to test Einstein’s two predictions of time dilation in special relativity and time passing more quickly in a weaker gravitational field
30-SECOND TEXT
Rhodri Evans
In an accelerating ship, a clock slows down: the same effect should be (and is) observed with gravity.

A CURVE IN SPACE & TIME
the 30-second theory
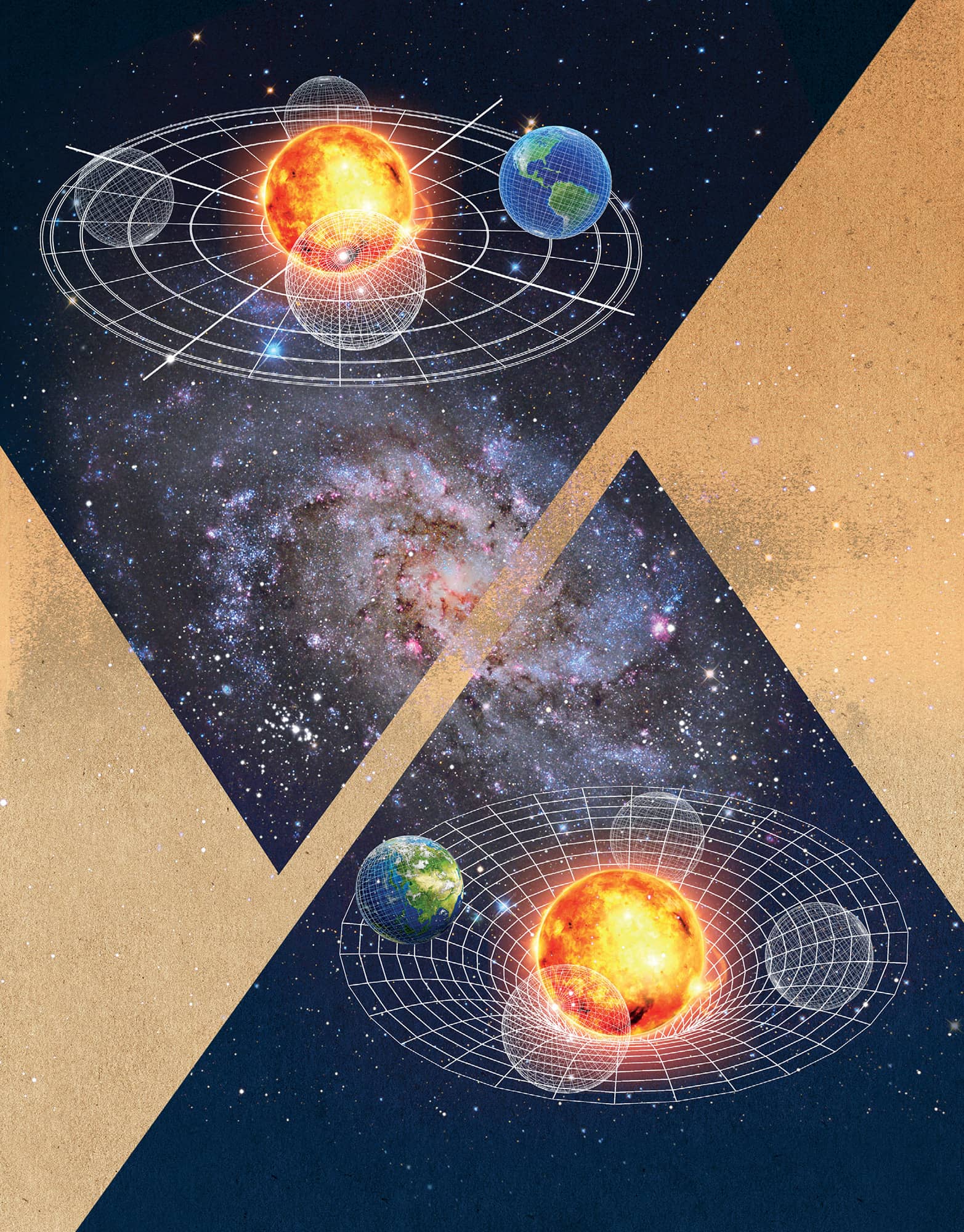
Special relativity has limitations when dealing with acceleration and gravity. However, Einstein believed that the laws of physics should apply in all circumstances including systems in accelerated motion and in the presence of gravitational fields. This means that equations and physical measurements such as time and distance, velocity and acceleration should be consistent – or, in mathematical language, covariant – between different frames of reference and in all systems of coordinates. The trouble was that the mathematics of special relativity run into problems with Euclidean geometry. The mathematical constant Pi, for example, would appear to change on an imaginary spinning disc due to the effects of special relativity shrinking the circumference of the disc. The solution to this problem, suggested by mathematician Marcel Grossman, was to adopt a non-Euclidean geometry to frame the principles of relativity. Euclidean geometry rests on the axiom that the shortest distance between two points is a straight line. In a non-Euclidean geometry, space becomes curved and the shortest distance between two points is a path across the curved surface – known as a geodesic. Einstein and Grossman’s key insight was to represent gravity as a curvature in non-Euclidean spacetime, i.e., they saw that gravity is curved spacetime.
3-SECOND THRASH
By thinking of gravity as a curvature in the fabric of spacetime, Einstein overcame the limitations imposed by special relativity.
3-MINUTE THOUGHT
Think of spacetime as two-dimensional, like one side of a piece of paper. The familiar three dimensions of space are squashed into one dimension running parallel with one side. Time runs at right angles. Objects with mass sit on the paper. This is the spacetime of special relativity. Replace the paper with a rubber sheet. Objects with mass sink into the sheet – deforming and curving it in a new dimension. This is the spacetime of general relativity.
3-SECOND BIOGRAPHIES
GEORG FRIEDRICH BERNHARD RIEMANN
1826–66
German mathematician who developed non-Euclidean geometry – essentially, the geometry of curved space
MARCEL GROSSMAN
1878–1936
Hungarian mathematician who introduced Einstein to the idea of non-Euclidean curved-space geometries
30-SECOND TEXT
Leon Clifford
The presence of a massive body like the Sun warps spacetime so that an object like the Earth moving in a straight line follows a curved path.
NEEDING A NEW MATHEMATICS
the 30-second theory
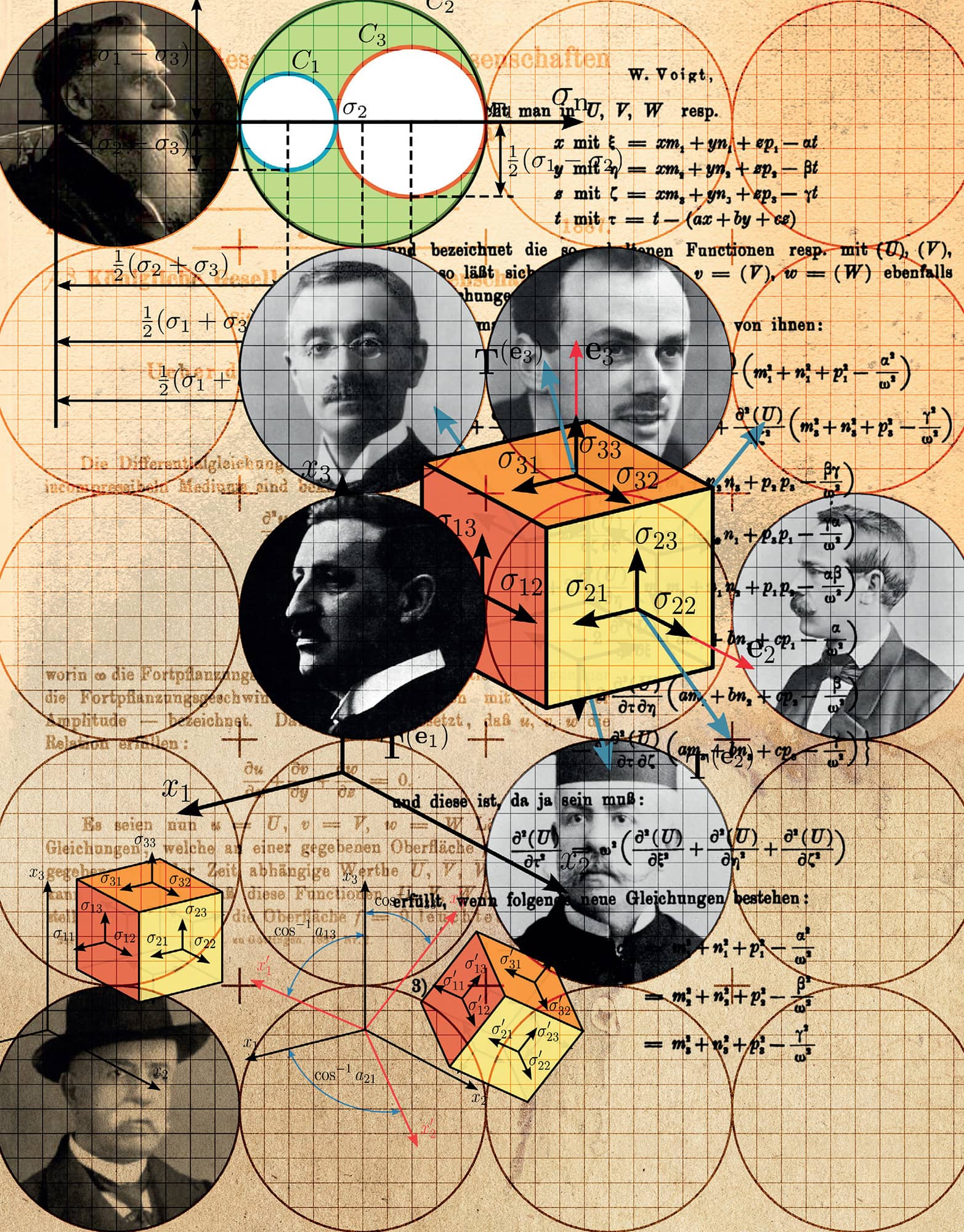
General relativity requires non-Euclidean geometry and a curved spacetime that cannot be described using conventional mathematics. This could have been a major stumbling block for the development of a generalized theory of relativity. Fortunately, in the course of the 19th century, mathematicians had been developing exactly the kind of mathematics that Einstein needed. General relativity requires the manipulation of complicated arrays of values similar to matrices that are called tensors. A matrix can describe a mathematical transformation mapping one state to another within a Euclidean space. Tensors can describe such transformations in non-Euclidean spaces with the greater flexibility needed to cope with the changing curvature of spacetime caused by gravitational fields. Tensors are complex mathematical objects that behave in peculiar ways. They need a bespoke notation to describe them and the theory of calculus must be extended to handle them. The properties of tensors, the notation needed to describe them and the rules of calculus to manipulate them had all been worked out by the time Einstein needed them. With Marcel Grossman’s help, Einstein mastered the techniques of tensor calculus sufficiently to express his theory as a set of elegant mathematical equations.
3-SECOND THRASH
General relativity was such a groundbreaking theory that Einstein needed a different kind of mathematics in order to describe the physics in the form of equations.
3-MINUTE THOUGHT
Physicists have a habit of finding uses for apparently obscure ideas in pure mathematics. James Clerk Maxwell’s equations pushed imaginary numbers into service. Einstein discovered a use for non-Euclidean geometries and tensor calculus. Paul Dirac applied a highly specialized form of algebra that has been built on by modern theorists. Some physicists believe there is a deep relationship between mathematics and reality. What new discoveries in physics may lurk in the existing theorems of pure mathematics?
3-SECOND BIOGRAPHIES
ELWIN BRUNO CHRISTOFFEL
1829–1900
German mathematician and physicist who created a notation for describing the tensors used in general relativity
WOLDEMAR VOIGT
1850–1919
German physicist and mathematician who applied the term tensor to the complicated matrix-like mathematical objects used in general relativity
30-SECOND TEXT
Leon Clifford
The equations of general relativity make use of the geometry of curved space and multidimensional objects called tensors.
THE HILBERT CHALLENGE
the 30-second theory
Einstein was not the only person working on the problem of general relativity; German mathematician David Hilbert was also on the case. Hilbert was fascinated by the mathematical challenge of expressing physical laws in an invariant form – so they remain the same in all circumstances. This means they work in all frames of reference and, unlike special relativity, do not depend on a particular system of coordinates; a property called general covariance. Hilbert pursued the mathematical challenge of seeking generally covariant equations for relativity. Where Einstein tackled this as a physics problem, Hilbert’s approach built up from mathematical axioms. Hilbert and Einstein knew each other, were in communication and were well aware of each other’s work. They completed their respective derivations of the field equations of general relativity virtually simultaneously. Hilbert submitted a draft of his paper in 1915 ahead of Einstein’s submission but Einstein’s paper appeared first. Einstein had done much of the groundwork on general relativity but Hilbert had been first to figure out how to solve the crucial final step. There remains uncertainty about how much the two men influenced each other and whether Hilbert’s work helped Einstein crack the problem. Hilbert never claimed credit for general relativity.
3-SECOND THRASH
German mathematician David Hilbert derived the field equations of general relativity at almost the same time as Einstein.
3-MINUTE THOUGHT
Physics was ripe for the emergence of relativity at the turn of the 20th century. Many mathematicians and physicists were working on the ideas that Einstein successfully fused. There is little doubt that, even without Einstein’s huge intellectual effort, someone would have discovered relativity. So,if history had taken a slightly different turn, we could now be talking about Hendrik Lorentz’s theory of special relativity and David Hilbert’s theory of general relativity.
3-SECOND BIOGRAPHIES
ERNST MACH
1838–1916
Austrian physicist whose view that the large-scale structure of the universe affects physical laws influenced Einstein’s approach
DAVID HILBERT
1862–1943
Outstanding German mathematician who helped structure the modern concepts of mathematics
30-SECOND TEXT
Leon Clifford
Einstein raced against the great German mathematician, David Hilbert, to be the first to complete the general theory of relativity.

THE EQUATIONS
the 30-second theory
In a series of four papers published in late 1915 Einstein outlined his theory of general relativity. These culminated with a short paper entitled Die Feldgleichungen der Gravitation (‘The Field Equations of Gravitation’), which contained a workable mathematical formulation of general relativity. The paper sets out a tensor relationship that yields a set of ten equations that Einstein describes as generally covariant; that is, they will hold true anywhere, in all frames of reference and in any coordinate system. Mathematically, the equations can be written as a set of non-linear partial differential equations. They describe the curvature of spacetime. The equations are consistent with the fundamental physical laws of the conservation of energy and the conservation of momentum. The fact that they are non-linear means they cannot always be solved; for example, in a situation where there is strong and changing curvature of spacetime – such as two black holes orbiting one another. The equations can be simplified where the gravitational field is very weak and space is not curved to be consistent with special relativity. The equations are equivalent to Newton’s laws when gravitational fields are weak and velocities are significantly less than the speed of light.
3-SECOND THRASH
Ten related equations form the core of general relativity, which describes how the gravitational field due to the presence of mass and energy curves spacetime.
3-MINUTE THOUGHT
Einstein showed that general relativity resolved an astronomical mystery that had been puzzling scientists. The orbit of the planet Mercury did not behave as it should according to Sir Isaac Newton’s laws. The point where Mercury’s orbit passes closest to the Sun, known as the perihelion, moves around the Sun – or precesses – fractionally faster than it should do according to Newton’s laws. Einstein’s equations precisely account for this mysterious variation in the precession of Mercury’s orbit.
3-SECOND BIOGRAPHIES
ISAAC NEWTON
1643–1727
English physicist and mathematician whose laws of motion described the orbits of the planets around the Sun
GEORGE FRANCIS ELLIS
1939–
South African physicist who discovered new solutions to the field equations with cosmological implications
30-SECOND TEXT
Leon Clifford
The first success of Einstein’s equations was in perfectly predicting the difference in the orbit of Mercury from Newton’s theory.

EDDINGTON’S EXPEDITION
the 30-second theory
According to Einstein’s theory of curved spacetime, the light from a distant star should be bent by a small but measurable amount as the light ray passes close to the Sun. Under certain assumptions Newton’s theory also predicts a deflection of light, but only by half the amount. Thus it ought to be possible to discriminate between the two theories by measuring the actual amount of bending, and in 1916 Einstein issued a challenge to astronomers to do just that. There was a catch, though: the necessary observations had to be made during a total solar eclipse, which is the only time stars are visible close to the Sun. The next suitable eclipse was due on 29 May 1919, when the path of totality stretched from South America to equatorial Africa. The British astronomer Arthur Eddington organized two expeditions, sending one team to Sobral in Brazil, while his own team headed for the island of Principe off the coast of Africa. Despite a series of frustrating problems – including cloudy skies at Principe and excessively hot weather at Sobral – the expeditions were ultimately successful, and Eddington was able to claim victory for Einstein.
3-SECOND THRASH
The first experimental confirmation of Einstein’s theory of general relativity came in 1919, when Eddington measured the deflection of starlight during a total solar eclipse.
3-MINUTE THOUGHT
Although it was widely accepted at the time that Eddington’s observations supported Einstein’s theory over Newton’s, they were actually far from being conclusive. All the photographs were degraded, and in most cases the margin of error encompassed both Einstein’s prediction and Newton’s. The average deflection came out very close to Einstein’s value, but only because Eddington discarded measurements that disagreed with his expectations. Later measurements confirmed Einstein’s theory with far greater certainty.
3-SECOND BIOGRAPHY
ARTHUR EDDINGTON
1882–1944
English astrophysicist, science popularizer and champion of general relativity
30-SECOND TEXT
Andrew May
Arthur Eddington used observation of the shift in position of stars seen near to the eclipsed Sun to test general relativity.
