FROM THE BIG BANG TO NOW
Let us use a timeline to summarize what we know as of this writing, about the history of our universe from the big bang to the present. By our universe I mean what we can see with our telescopes today and what we can infer from those observations about the past of that universe. We will consider the future in the following section and the possibility of other universes in the next chapter.
Since our current physics knowledge, based on accelerator experiments, “only” goes back to 10–12 second after the big bang, the best we can do is speculate about earlier times. However, our speculations need not be merely wild guesses if we base them on solid existing knowledge.
The reader should keep in mind that I do not claim to depict what actually exists in some ultimate, metaphysical reality. As I have emphasized, my philosophical position is that that we cannot access precise knowledge of that reality. All we can do is make observations, as quantitative as possible, and describe those with mathematical models. These are based on our own human notions, operationally defined, such as time, space, and temperature. The following is such a description, with temperatures given in eV. Keep in mind it is a simplified model and certainly not the final word.
10–43 second, 1028 eV. The Planck Time.
At the Planck time, our universe was an empty sphere of Planck dimensions, 10–35 meter. Any model seeking to describe an empty universe in terms of the human-contrived notions of space and time is maximally symmetric. It will automatically contain the implied conservation principles people call “laws” that I have termed “metalaws.” No lawgiver was involved, natural or supernatural. These models automatically obey the rules of quantum mechanics and relativity, which also follow from symmetries. This includes, in particular, the uncertainty principle and the Friedmann equations, which we use to model what happened next.
While empty of particles, quantum fluctuations in vacuum energy density or, if you prefer, the curvature of space-time, caused the sphere to expand and contract. At some unknown time, usually taken to be about 10–35 second, a positive fluctuation was large enough to trigger inflation. Friction in the expanding universe, predicted by the model, sufficiently slowed the return to equilibrium and our universe expanded exponentially by many orders of magnitude.
~10–34 second, 1024 eV. The End of Inflation (exact times unknown).
Like a damped harmonic oscillator, the same friction brought inflation to a halt and the almost-linear Hubble expansion took over. The first particles were generated from the energy lost. They were all massless.
Being maximally symmetric, the forces by which the particles interacted were unified as a single force. Maximal symmetry includes supersymmetry, with each particle and its spartner being identical with zero mass.
As the expanding universe cooled, a series of spontaneous (accidental, uncreated, undesigned) symmetry-breaking phase transitions took place that differentiated the types of particles and forces from one another. This was first step of the development of asymmetric structure in the universe. Particles were distinguished from antiparticles and given a slight preference. Or, more precisely, we label those given preference “particles” and the others “antiparticles.” Bosons were separated from fermions, as supersymmetry was broken. Leptons were differentiated from baryons (quarks). Gravity was distinguished from the forces described by a grand unified theory (GUT) model. The particles remained massless.
Then, with further cooling, the GUT symmetry was broken and the strong force was separated from the electroweak force. Gluons were separated from the other bosons. The universe was now composed of all the particles and antiparticles of the standard model, with the quarks and gluons forming a strongly interacting quark-gluon plasma while the other particles moved around freely, scattering off one another in what was a very dense medium.
Note: Most timelines you will see in earlier books and articles place symmetry-breaking phase transitions prior to inflation, since they assume the inflaton field was the GUT Higgs field (GUTH?). However, this need not have been the case and the GUT and phase transitions could have occurred after inflation when there were particles present to work with.
10–10 second, 100 GeV. Electroweak Symmetry Breaking.
At this point we can begin to make more definite statements because as a result of accelerator experiments we already understand the physics involved. About this time, the electroweak unity of the standard model was broken and the electromagnetic forces and weak nuclear forces separated, giving us the four distinct forces we experience today—gravity, electromagnetism, and the weak and strong forces. The Higgs boson appeared and gave mass to the weak bosons and leptons while the photon and gluon masses remained zero. The weak force thus became limited to a range of about 10–18 meter while the electromagnetic force remained infinite in range. Quarks also gained mass, however only partially by the Higgs mechanism and mainly from their strong interactions with gluons, which themselves remained massless and buried in the quark-gluon plasma.
The sparticles left behind by the breaking of supersymmetry gained large masses with the lightest neutral one possibly becoming the dark matter. But, as we have seen, this scenario has not yet produced the SUSY particles expected at the LHC, so this remains uncertain. Perhaps the sparticles are too high in mass to see at that collider. We will have to wait and see.
10–6 second, 1 GeV. Quark Confinement.
When the temperature of the universe dropped to about a billion electron volts, quarks and gluons fashioned nucleons and the many composite hadrons that were discovered in particle accelerators in the 1960s and 1970s. Most are very short-lived and so decayed away with only protons and neutrons and their antiparticles remaining, along with electrons, positrons, and photons. All were in quasi equilibrium.
About this same time, antinucleons and nucleons annihilated, leaving behind a residue of one in a billion protons and neutrons. Photons and leptons dominated.
1 second, 1 MeV. Synthesis of Light Nuclei.
Neutrinos decoupled, forming the cosmic neutrino background. Light nuclei began to form. Free neutrons were all absorbed into nuclei or decayed away into protons, antineutrinos, and electrons.
10 seconds, 100 keV. Positron Annihilation.
Positrons and electrons annihilated, leaving a residue of one in a billion electrons.
3 minutes, 25 keV. Radiation Dominance.
Nucleosynthesis terminated. The energy density of photons exceeded that of nuclei, giving radiation dominance. The universe was opaque since the photons were swimming around in a charged plasma of nuclei and electrons with which they interacted as they do in a dense fog.
60,000 years, 1 keV. Matter Dominance.
The density of nuclei exceeded the photon density, moving from radiation dominance to matter dominance. The universe remained opaque.
380,000 years, 700 eV. Photon Decoupling.
Atoms form (“recombination”), photons decouple, and the universe becomes transparent. The sky is bright orange and gets redder as the universe cools. Atomic matter starts to clump along with the dark matter.
5 million years, 0.01 eV. The Dark Age Begins.
The universe has cooled so that the background radiation is now well outside the visible spectrum and the sky becomes dark.
200 million years, 0.002 eV. Star Formation Begins.
First stars form and the Dark Age ends. The stars are much larger than the sun and contain no heavy elements, so they burn out fast and we have many supernovae that synthesize heavier elements. Supernova radiation reionizes space and makes it slightly foggy, but nowhere near as much as during the Dark Age. Light can still get through, though somewhat dimmed. Active galaxies such as quasars may also start forming, adding to the ionizing radiation.
~1 billion years, 0.001 eV. Galaxy formation.
Galaxies form. They have frequent collisions and contain supernovae that continue spreading heavy elements throughout space, which then become ingredients of the next generation of stars. These stars are less massive and more slowly burning, like the stars we have today. Active galaxy formation slows down.
~6 billion years, 4 × 10–4 eV. Cluster Formation.
Denser regions begin collapsing and forming the various structures of galaxy clusters and superclusters.
~7 billion years, 4 × 10–4 eV. Acceleration Begins.
Up until this time, the expansion of the universe was decelerating because of the dominance of matter and radiation, which have attractive gravity. However, their densities have been dropping while the dark-energy density has remained constant. That density now exceeds the other densities, and since it has negative pressure, the expansion of the universe slowly begins to accelerate.
~8 billion years, 3 × 10–4 eV. The Familiar Universe Appears.
First spiral galaxies are formed.
9.1 billion years, 3.2 × 10–4 eV. Solar System Forms.
Our sun and planets form.
13.8 billion years, 2.6 × 10–4 eV. Now.
THE FUTURE
While predicting the future is always a risky task, we can still ask what timeline into the future is implied by current knowledge.
5 billion years. Bye, Bye Earth.
Our sun uses its last hydrogen fuel and becomes a red giant, incinerating Earth. In the following billion years, the sun contracts to a white dwarf.
17 billion years. Merging.
Milky Way and Andromeda merge.
~40 billion years. Structure Formation Ends.
The exponential expansion caused by dark energy overcomes any remaining gravitational clumping and structure formation ceases.
~100 billion years. Bye, Bye, Other Galaxies.
Other galaxies have all moved behind the horizon of Milky Way/Andromeda, leaving it alone in the universe. Eventually everything is beyond the horizon of everything else.
~1 trillion years. Bye, Bye, Stars.
Remaining stars begin to die out, leaving behind black holes, neutron stars, brown dwarfs, and planets.
1033–1037 years. Bye, Bye, Matter.
Protons and other heavy particles decay, leaving behind a gas of photons, electrons, and neutrinos that continues to thin forever.
No Heat Death
In chapter 5, we learned that physicists in the nineteenth century developed the concept of “heat death” in which the universe ultimately must reach an equilibrium state of maximum entropy. However, they still labored under the assumption that the universe was a firmament in which bodies maintained a constant average separation from one another. In this situation, the entropy of the universe has a maximum, which we now know (they didn't) is equal to the entropy of a black hole of the same size.
But heat death never happens. Rather, the universe continues to expand indefinitely and evolves to a pure de Sitter space where each causal patch never reaches maximum entropy. Now, the possibility remains that the universe is ultimately closed, that is, the curvature parameter k = 1. Of course, inflation implies that the universe is still very flat, better than one part in 1060, but this can still happen with k = 1 where the universe has a tiny positive curvature.
Nevertheless, we can't look forward to a “big crunch” in which the expansion stops, the universe contracts back down to a Planck sphere, and everything begins all over again. This oscillatory universe was once very popular, but that was before the discovery of dark energy. As indicated above, about seven billion years ago (halfway back to the big bang) the energy densities of matter and radiation dropped below that of dark energy and the universe's expansion began to accelerate. If the source of dark energy is a cosmological constant, or something that looks very much like it, the energy density will remain constant while the radiation and matter die away and the universe continues to expand forever.
DID THE UNIVERSE HAVE A BEGINNING?
In 1970, Stephen Hawking and Roger Penrose showed that, within the framework of general relativity, our universe began as an infinitesimal point of infinite energy density in space called a singularity.1 Theologians, notably William Lane Craig, have lifted this out of context and used it to claim that space and time must have been created at that instant, thus showing that the universe had a beginning. They then proceed to argue, without theoretical proof or empirical evidence, that anything that begins has a cause and, in the case of the universe, in a huge leap of incongruity, that cause must be the personal God of Christianity.2
However, as Hawking pointed out in his 1988 bestseller A Brief History of Time, “There was in fact no singularity at the beginning of the universe…it can disappear [from the theory] once quantum effects are taken into account.”3 Penrose agreed. General relativity is not a quantum theory and so cannot be applied wherever quantum effects are important. And this, in particular, includes the beginning of the universe.
But Craig and his fellow faithful never give up. In a debate with philosopher Alex Rosenberg held at Purdue University on February 1, 2013, and in other debates and writings, Craig referred to a 2003 paper by Arvind Borde, Alan Guth, and Alexander Vilenkin (BGV), which Craig says proves that the universe had to have a beginning.4 It is to be noted that this paper is also based purely on general relativity and does not take quantum mechanics into account.
I have asked Vilenkin, whom I have known professionally for many years, “Does your theorem prove that the universe must have had a beginning?” He replied, “No. But it proves that the expansion of the universe must have had a beginning. You can evade the theorem by postulating that the universe was contracting prior to some time.”5 The same point has been made by Anthony Aguirre and Steven Gratton,6 and Sean Carroll and Jennifer Chen.7 Carroll skillfully engaged Craig in a debate on cosmology in New Orleans on February 21, 2014.8
In figure 15.1 we have a space-time diagram showing worldlines, which are paths in space-time, of particles emerging from the origin of an expanding universe.
BGV proved that all the worldlines had to originate from a point, which we can interpret as the beginning of our universe, that is, the one we live in. The other authors showed that the worldlines can be continued through the origin to the negative side of the time axis.
In short, our universe had a beginning, but it need not have been the beginning of everything.
Craig has come up with yet another argument for everything having a beginning and thus a creator. If it did not, Craig says, then it would have begun an infinite time ago, in which case we would never have reached the present.9
Recall that the same argument was made in the sixth century by John Philoponus and in the ninth century by Ya’qūb ibn Ishāq al-Kindī (see chapter 2). Theological arguments never die. Nor do they fade away.
In this regard, it is to be noted that most physicists and cosmologists (and even many mathematicians) use the term infinity when they really mean “endless” or “unbounded,” or often just “a very big number.” However, as brilliant work by mathematician Georg Cantor (1845–1919) showed, infinity is not a real number.10
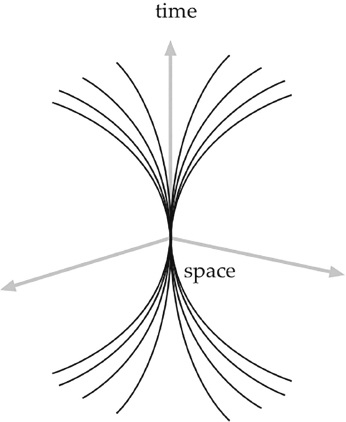
Figure 15.1. A space-time diagram showing worldlines of particles emerging from the origin of our universe. They can be extended in the negative time direction. Image by the author.
In 1925, the renowned mathematician David Hilbert (1862–1943) gave a talk in which he said, “The infinite is nowhere to be found in reality. It neither exists in nature nor provides a legitimate basis for rational thought.”11
I assume that by “reality” he was referring to the measurements of observed phenomena we make in the world with our scientific instruments. Also, I am not sure what Hilbert meant in saying that the concept of infinity provides no legitimate basis for rational thought. Certainly his and Cantor's work with infinity was eminently rational. And theoretical physicists are not being irrational when they use the well-defined mathematical concept of infinity in their theories, so long as they are careful not to apply it to any quantities measured by us experimental physicists or to give it ontological, that is, Platonic, meaning.
Craig quotes Hilbert to bolster his argument for a beginning to everything. If there is no actual infinity, then everything cannot be eternal, that is, could not have begun an infinite time ago. Otherwise it would have taken an infinite time to reach the present. However, as I pointed out in chapter 2 when discussing the same argument made by Philoponus, an eternal universe would not have had a beginning an infinite time ago. It had no beginning. Running a clock backward and counting the ticks: -1, -2, -3,…, will never get it to -∞. The time from now to any moment in the past is a finite number of ticks.
Another simple way to see why everything need not have had a beginning is based on the fact that there is no reason for it to have an end. Cosmology indicates that our current universe will simply go on expanding forever. Then, since time is perfectly symmetric (with the conventional direction of time just being a definition based on common experience), even if we can identify a point in the past as the beginning of the big bang, that need not have been the beginning of everything. If there is no end, there must be no beginning.
AT THE PLANCK TIME
Figure 15.1 shows worldlines passing through a point at the origin of a space-time diagram. However, it should be recalled that quantum mechanics requires that this be a finite region of space and time and not an infinitesimal point. If this region is as small as possible, it should be of Planck dimensions, that is, a four-dimensional sphere with a spatial radius on the order of the Planck length and temporal dimension on the order of the Planck time—small, but not an infinitesimal point. Because of the quantum uncertainty principle, such a sphere would have a mass equal to the Planck mass, 1028 eV, and thus meets the main criterion for a black hole described in chapter 6.
Since we cannot see inside a black hole, we have zero information about what went on before the Planck time. And so the earliest possible time that has any instrumental meaning in our universe is the Planck time, 10–43 second. Here we must again make a distinction between theory and observations. While we can always write equations assuming time is a continuous variable and having any possible value no matter how small, we cannot even in principle measure a shorter time interval than the Planck time. This is why I do not get excited when some theory based on continuous space-time has problems with infinities. They are not empirical physics problems; they are theoretical physicist problems. The theory is simply wrong and has to be corrected.
As mentioned, the maximum entropy of a spherical body is equal to the entropy of a black hole of the same radius. So, at the Planck time, the universe was in a state of complete disorder or maximum entropy. This implies that anything that may have gone on earlier has left no imprint on our universe—other than utter and complete randomness. So, even if there were a creation, divine or natural, our universe possesses no memory of it. Not only is the god of most religions ruled out, so is the Enlightenment deist creator god (see chapter 2). The only possible god is a “quantum deist” god who started up the universe by throwing dice and then teleporting itself away to another reality. But then, who needs such a god—one who has no effect on anything?
Now, you might inquire, if the universe began with maximum entropy, does that not violate the second law of thermodynamics, which says that the entropy has to be lower in the past than it is now? No, because the entropy was lower in the past. But then, you ask, how could it have been lower in the past if it was maximal in the past?
Easy. At the Planck time the entropy was maximal for a sphere of Planck dimensions. As the universe expanded in the big bang, so did its maximum allowable entropy. And so, since the Planck time there has been room for local structures to form as the loss of local entropy during the formation of structure is offset by an increase in entropy of the environment, namely, the rest of the universe.
QUANTUM GRAVITY
The common wisdom is that we will not be able to describe even theoretically what may have gone on prior to the Planck time until we develop a quantum theory of gravity that encompasses all the features of both quantum mechanics and general relativity. Currently, quantum field theory includes the special theory of relativity but not the general theory.
Early attempts at quantum gravity, by Richard Feynman and others in the 1950s, were patterned after highly successful quantum electrodynamics. In place of the spin-1 photon, which is the mediating boson of the electromagnetic force, a massless spin-2 graviton was introduced to carry the gravitational force between two masses.
But the mathematics simply did not work out, requiring other avenues of approach that have yet to succeed.12 Most depend heavily on supersymmetry and could come crashing down if SUSY fails to be verified at the Large Hadron Collider. This includes string theory, to which almost an entire generation of theoretical physicists has devoted their professional lives.
Gravity is clearly a different kind of force from the other two. You will often hear it said, even by prominent physicists, “Gravity is 1039 times weaker than the electric force.” But that number is just the ratio of strengths of the forces between a proton and an electron and is not true in all cases. If instead of these you have two particles with the same electric charges but masses equal to, say, the Planck mass (a more natural mass than that of a proton or an electron), gravity is 137 times stronger than the electric force! There simply is no way to define an absolute strength of gravity the way you can for the other forces.
However, I can suggest a simple explanation for why gravity on the scale of elementary particles is so weak compared to electromagnetism. Their masses are intrinsically low, starting out zero and gaining a small mass by the Higgs mechanism described in chapter 11. I will elaborate further in chapter 16.
It also should be remembered that in Einstein's general relativity, the phenomenon of gravitation results from the curvature of space and no explicit gravitational force enters the equations. In this model, Earth doesn't stay in orbit around the sun because the sun's force of gravity is pulling on it by exchanging gravitons or whatever; it follows the natural path it should follow in the absence of any forces—a geodesic through space-time, which happens to curve around the sun. More recently, it has been suggested that gravitation can be described as an “emergent” phenomenon that arises from the tendency of systems to move in the direction of higher entropy (see chapter 5 for a discussion of emergence).13
Even if we had a quantum theory of gravity, we would seem to have no way of checking its predictions in the Planck regime where it supposedly applies. Still, quantum gravity may possibly have measurable effects. For example, at distances near the Planck scale, space-time is expected to be “lumpy,” that is, instead of a smooth continuum we should see the famous “quantum foam” proposed by John Wheeler in 1955.14
Amazingly, it turns out that this foaminess is in principle detectable. Recall the discussion of gamma ray bursts in chapter 13. They take place in distant galaxies, billions of light years away and emit photons of high energies. They are believed to result from the collision of two neutron stars. These photons could interact with the quantum foam and be delayed in reaching Earth. This effect might be observed by measuring the arrival times of two or more photons that were emitted simultaneously from the same burst.
Using data on gamma-ray bursts taken by the Fermi Gamma-Ray Space Telescope, astronomer Robert Nemiroff and collaborators compared the arrival times of gamma-ray photons of different energies emanating from the same sources. In the case of gamma-ray burst GRB 090510 recorded in May 2009, which is seven billion light-years away, three photons were observed to arrive within a millisecond.15 The result places a limit on the size of the bubbles of space-time foam of 525 times less than the Planck length. Although the result needs to be independently confirmed, it would appear that space-time is smooth in the observable universe.
It might be asked how this result complies with the assertion I made in chapter 6 that no distance smaller than the Planck length can be measured. The answer has to do with the difference between theory and experiment, to which I have alluded time and again in this book. The actual measurement was not that of a distance shorter than the Planck length. However, that measurement was inserted into a theoretical model that then proceeded to predict a limit on bubble size much less than the Planck length. But that quantity itself exists only within the model and is not a direct observation.
THE BIVERSE
Let us now ask what might have existed on the negative side of our time axis, that is, prior to t = 0 in our past. Where did this primordial sphere of total chaos come from? While we possess no observable information on what may have gone on before the Planck time, we can continue to apply our best theoretical knowledge, that is, general relativity and quantum theory, which were both founded on empirical evidence from later times.
In a 2006 book and published article, I described a scenario that provides for a natural origin of our universe that follows from well-established physics and cosmology.16 It was based on a model proposed in 1982 by David Atkatz and Heinz Pagels.17 I worked out the scenario fully mathematically at a level accessible to undergraduate physics majors, relying heavily on a very nice tutorial published in 1994 by Atkatz, Quantum Cosmology for Pedestrians.18 Here I will just outline the procedure.
In 1982, Atkatz and Pagels had shown how our universe could appear as a quantum-tunneling event. This mechanism was also proposed by Vilenkin in 198219 and by James Hartle and Stephen Hawking in 1983.20
We start with the Friedmann equations for an empty, homogeneous, isotropic universe with positive curvature, that is, with curvature parameter k = +1. Although our universe is very close to being flat, this does not necessarily require that the global curvature parameter k = 0; it could have k = +1 or k = –1 and still be very, very flat after inflation. Atkatz and Pagels showed that tunneling only works for k = +1.
With this equation in hand, we then follow the usual rules by which one goes from a classical equation to a quantum mechanical one in which real numbers are replaced by mathematical operators.21 The result is surprisingly simple. You obtain the quantum mechanical time-independent Schrödinger equation for a nonrelativistic particle of mass equal to half the Planck mass and zero total energy, with a single dimension that is just the cosmological scale factor of the universe, which we can take to be the universe's radius. Note this is just a mathematical equivalence and does not imply that such a particle exists.
The derived equation is a simplified form of the Wheeler-DeWitt equation, whose solution is grandly referred to as “the wave function of the universe.”22 In the usual quantum mechanical way of interpreting wave functions, squaring the amplitude of the wave function of the universe gives the probability of finding a particular universe in an ensemble of many similar universes.
The resulting scenario is illustrated with the space-time diagram in figure 15.2. Time, t, is plotted vertically and two of the three dimensions of space, x and y, are shown in perspective. At a given time, the expanding spherical universe is projected as a circle perpendicular to the time axis. It emerges from a sphere of Planck dimension located at the origin, t = 0.
Also shown is a mirror universe on the opposite side the time axis from our universe. If we look at the Friedmann equations and other equations of cosmology, nothing prevents negative time. The mirror universe emerges from the same Planck sphere in the opposite time direction. Note this is consistent with the worldline picture shown in figure 15.1.
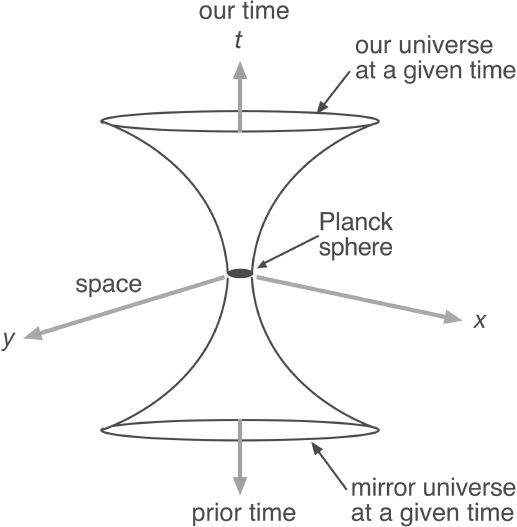
Figure 15.2. A space-time diagram showing our universe and a mirror universe emerging from the same Planck sphere in opposite time directions. One space dimension is suppressed, so the spherical universes and Planck sphere are projected as circles. Image by the author.
Now here is a widely unrecognized implication: While the mirror universe exists in our past, its arrow of time points opposite to ours. As we saw in chapter 5, no direction of time can be found in the equations of physics. The familiar, everyday arrow of time is a purely statistical definition in which forward time is the direction of most probable occurrences, as specified by the increasing entropy of our universe.23 And so, because the mirror universe is expanding in the direction opposite to ours, the direction of the arrow of time that would be defined by an observer in that universe points opposite to ours.
Since the Planck sphere contains no information or structure, it is functionally indistinguishable from “nothing” and we have two universes tunneling from nothing. I will call our universe and its mirror partner the biverse.
I should add that we do not expect the mirror universe to be identical to ours, since it is just as likely to be as dominated by randomness as ours is.
While I cannot prove this is how our universe came to be, no one has proved it did not. That is, we have a plausible scenario for the natural, uncreated origin of the universe based on established physics and cosmology. It is just one of several possible scenarios that have been proposed, but this strikes me as by far the simplest since it requires no new assumptions—just established physics and cosmology. If nothing else, the biverse serves to refute any claim that our universe could have originated only by means of a supernatural creation.
At this point, the reader might ask where the “established physics and cosmology” came from. Please be patient. We will shortly get to that.
THE MULTIVERSE
If two universes are possible, then why not three or four—or any number? Since the 1980s, scientists have recognized the possibility that our universe may be just one of an unlimited number of similar but not identical universes dubbed the multiverse. As we saw in chapter 12, soon after physicists came up with the idea of inflation it was realized that the bubble-formation process that produced our universe would likely generate many more.
From the beginning, theists objected vehemently to the whole notion of multiple universes. Virtually all world religions teach the divine creation of a single universe with a central place for humanity. The multiverse challenges that belief.
On July 7, 2005, Christoph Schönborn, Cardinal Archbishop of Vienna wrote in the New York Times, “The multiverse hypothesis in cosmology [was] invented to avoid the overwhelming evidence for purpose and design found in modern science.”24
Of course the Church also objected when Copernicus moved the Earth from the center of the solar system. And when Giordano Bruno said that ours was just one of many planets orbiting many suns, they burned him at the stake. Theists talk about humility, but they don't like it when science gives them a reason to be humble.
William Lane Craig has expressed similar sentiments as Schönborn. In the Purdue debate Craig said, “The proponents of chance have been forced to postulate the existence of a World Ensemble of other universes, preferably infinite in number and randomly ordered, so that life-permitting universes will appear by chance somewhere in the Ensemble.”25
These statements are not only wrong but are insults to serious scholars. The “World Ensemble” or multiverse was motivated by established science—with no thought whatsoever to theology. It is the conclusion of our best current models of cosmology based on the extremely precise observations of modern astronomy and our best knowledge of fundamental physics.
Besides, suffice it to say, none of the claimed scientific evidence for purpose and design in the universe can be honestly classified as overwhelming.
A common objection to the notion of multiple universes is that it introduces additional entities when only a single entity, a lone universe, is needed. In 1986 astronomer Edward Harrison wrote: “Take your choice: blind chance that requires multitudes of universes, or design that requires only one.”26
To help us decide on the best choice, we can apply the test of Ockham's razor, which favors the simplest hypothesis when there are several to choose from. At first glance, it might seem that a single universe is more parsimonious than multiple universes. However, Ockham's razor does not apply to the number of objects in a theory but rather to the number of hypotheses. The atomic theory of matter multiplied the number of objects physicists had to deal with by trillions of trillions. Yet it was simpler and more powerful than macroscopic thermodynamics, which preceded it and which can be completely derived from atomic theory. Similarly, since current science based on observations implies multiple universes, to postulate that only a single universe exists requires an additional hypothesis not required by the data. That is, the single universe hypothesis is the one that violates Ockham's razor.
In another objection, many nonbelieving scientists have joined theists in arguing that that the multiverse is “nonscientific” because we have no way of observing a universe outside our own. In fact, this is wrong. The multiverse is a legitimate scientific hypothesis since it seems to be an unavoidable consequence of eternal inflation, the current model of the early universe, to be discussed in the next section, which is based on our best observational data.
Our theories often contain unobservables such as quarks and black holes. And, as we will see shortly, empirical evidence for other universes is not beyond the realm of possibility. Any phenomenon forming part of a viable theory that is in principle detectable is a legitimate part of science.
The whole idea that many universes may exist is of such staggering consequence that it has been the subject of a huge literature in science, philosophy, and theology over the last thirty years or more.27 Rather than attempt to review all that has been written on the subject, I will restrict my discussion to the latest conclusions we can draw from the simplest assumptions.
ETERNAL INFLATION
In 1983, cosmologist Alexander Vilenkin, with some trepidation, suggested what is now called eternal inflation.28 According to eternal inflation, once expansion starts it never ends, with new universes being created all the time. In 1986, Andrei Linde elaborated the idea, showing how it was possible that the universe reproduces itself indefinitely and “may have no beginning or end.”29
Eternal inflation, as conceived by Vilenkin and Linde, results in the continual production of universes inside of other universes in a fractal-like structure.30 Basically, while a bubble universe is exponentially inflating to a much larger size, other bubbles can nucleate in an ever-growing empty de Sitter space surrounding the original bubble. This process continues eternally into the future.
In a model proposed by Anthony Aguirre of the Institute for Advanced Studies, Princeton, and Steven Gratton of Princeton University, the bubble nuclei are biverses similar to what I describe earlier, each with opposite arrows of time.31
What about the past? William Lane Craig has continued his effort to find evidence for a creation in cosmology by denying that time extends indefinitely into the past as well as to the future. Finally recognizing that our universe may not be all there is, he now says, “Even if our universe is just a tiny part of a so-called ‘multiverse’ composed of many universes, the BGV theorem requires that the multiverse itself must have an absolute beginning.”32
As we have seen, the BGV theorem requires only that inflation have a beginning. It says nothing about the beginnings of multiverses. Indeed, in the biverse scenario, two universes have the same beginning and expand in opposite directions of time. In the eternal inflation picture, new universes are continually formed in each of these expanding spaces with opposite arrows of time.
I suppose Craig can claim, without proof or reason, that the whole shebang, what we might call the Big Shebang, was created by God. But let us put off discussing the theological implications until the end of the book and first address some remaining scientific issues.
SOLVING THE ENTROPY PROBLEM
In From Eternity to Here, Sean Carroll addresses a common question: Why was the entropy of the universe so low to begin with? This is called the entropy problem. Well, my glib response is that it was low to begin with because that's how we define “begin”—when the entropy was minimum. However, the real question, as Carroll explains, is why did such a highly improbable state occur at any time at all? If the universe is the result of random processes, then it should have begun with much higher entropy. It was like tossing a billion dice and having them all come up boxcars.
Of course, if the multiverse is unlimited, then every possible combination will come up an unlimited number of times. But that's too easy an answer and about as uninformative as saying God did it.
After carefully considering all the options, Carroll shows that the eternal multiverse offers a plausible solution to the entropy problem. He asks the key question: What should the universe look like if it is perfectly natural? His answer: “A natural universe—one that didn't rely on finely-tuned low entropy boundary conditions at any point, past, present, or future—would basically look like empty space.”33
Now, as we have seen, empty space is described by the de Sitter solution of Friedmann's equations and can have a positive cosmological constant Λ, which is equivalent to a constant vacuum energy density and results in exponential inflation. The entropy can be shown to be inversely proportional to the magnitude of Λ. So when that is large, the entropy will be small.
In chapter 12, I described how, as suggested by Linde, quantum fluctuations in de Sitter space can drive the inflaton field up in potential energy, like a dad pushing his daughter on a swing. This is equivalent to generating a cosmological constant. In this case, we can envisage a region of space experiencing such a fluctuation and becoming an expanding bubble. Usually that bubble will shrink back to nothing.
Occasionally, however, the fluctuation will be occasionally very large. Then, because of friction, one bubble will remain at a higher energy long enough to expand by many orders of magnitude. It then can pinch off from the original background space to become a separate bubble universe.
Since the bubble universe has a large cosmological constant, it will have low entropy. This solves the entropy problem: In order for an inflating bubble universe to emerge from a quantum fluctuation, it must have low entropy. This does not violate the second law of thermodynamics because we can view the bubble universe plus background space as a single system in which the total entropy still increases, the background gaining the entropy lost (or more) when producing the bubble universe.
Note that all this can be done without any underlying dynamics, that is, by way of purely random, chaotic inflation. Since everything is symmetric, no specially created laws of physics are needed, just metalaws and bylaws. The models we use to describe what happens are logically required to contain, within their formulations, those principles that are required by symmetries—metalaws. This includes all the conservation laws, special and general relativity, and quantum mechanics. They follow from the symmetries of emptiness. Spontaneously broken symmetries then provide the bylaws needed for complexity to evolve.
DISCOVERING OTHER UNIVERSES
A common argument against other universes is that we have no way of ever observing them. However, perhaps we can. Early in our universe another universe may have been sufficiently close for its gravity to affect the isotropy of the CMB. Or, the bubbles may have collided, leaving a bruise on each. A detection of a large-scale anisotropy in the CMB could provide evidence for a universe outside our own. The Planck space telescope has confirmed several unexplained anomalies of this nature that were hinted at in earlier observations by WMAP.34
Since the observation of another universe beside our own would be the greatest scientific discovery in history, don't expect any cosmologists to make such a claim until they have ruled out every other possibility to the highest level of confidence and have seen the data independently verified several times. In the case of Planck, the investigating team has not deemed the evidence sufficiently significant to make any published claim.
Put simply, the single-universe hypothesis requires that the universe be spherically symmetric. Any significant deviation from this would prove there is something outside the universe. At some point, our theories may be able to make a prediction of the quantitative deviation from spherical symmetry expected in the multiverse model. And, at some point, the CMB data from future experiments may become sufficiently precise to test that prediction. This would make the multiverse hypothesis falsifiable. This prospect alone should be sufficient to permit the notion of multiple universes to remain a part of legitimate scientific discourse.
THE MANY WORLDS OF QUANTUM MECHANICS
Conventionally, the multiverse of modern cosmology has been considered as unconnected with the many-worlds interpretation (MWI) of quantum mechanics. Recently, however, some authors have suggested a connection. Let's see what that connection might be.
It is important for us to again make a clear distinction between the mathematical model that constitutes the quantum theory used in making calculations and the ontological interpretation of what that theory may be telling us about the real world. The first is physics. The second is metaphysics.
The quantum model, which was already largely in place by the 1930s, has been hugely successful in describing the behavior of matter at the extremes of small distances, low temperatures, and high densities. However, the radical manner by which quantum methodology deviates from that of classical physics, which still works very well in other domains, has created a never-ending debate over what it all means.
Unlike classical models such as Newtonian mechanics or Einsteinian relativity (considered “classical” in this context), the quantum model does not predict where a particle will be at some later time but rather only the probability that it will be found in a certain volume of space. The probability per unit volume is given by the square of the amplitude of a mathematical object called the wave function or, more generally, the state vector (see chapter 6).
In the 1920s, Niels Bohr and Werner Heisenberg formulated what is known as the Copenhagen interpretation of quantum mechanics. While undergoing countless changes and appearing in many different forms over the years, for a long time Copenhagen was the closest thing we had to a “conventional” philosophical view of the meaning of quantum mechanics. Because of its many variations, some of which have been given other identifications, I will not try to present a comprehensive review but instead will focus on a few basic ideas common to most interpretations that follow more or less along the same lines as Copenhagen.
The fundamental, underlying assumption of these interpretations is that individual physical events are not predetermined by the laws of physics, as in Newtonian mechanics, but occur spontaneously. However, the statistical behavior of ensembles of similar events is predetermined, and this is what the mathematical model provides.
For example, when an atom in an excited state drops down to a lower state and emits a photon, that specific event is not predetermined, which in practice means it is unpredictable. However, the intensity of that particular spectral line, which results from a large number of photons making the same transition, is precisely calculable.
Similarly, no existing theory can predict that a particular radioactive nucleus will decay at a certain moment, but the hypothesis that such decays are equally likely for all times in a given time interval leads to the exponential decay “law” that is obeyed to great precision. In fact, this result and that for atomic transitions provide strong empirical evidence that these processes are not predetermined. That is, it is not our ignorance that makes these events random. They really are random.
Crucial to our discussion of many worlds, Copenhagen treats measuring devices as classical systems and so the act of measurement constitutes a quantum-to-classical transition that is not described by the theory but is simply assumed to take place upon the act of measurement. Before the measurement of a particle's position is made, the wave function gives the probability for wherever the particle was known to be prior to that time. If nothing is known, the particle can be anywhere in the universe. After a measurement, the particle is known to be within the volume of the detector and so the wave function is said to instantaneously “collapse” to give the new probability. This is illustrated in Figure 15.3.
Einstein objected to the whole idea of instantaneous wave-function collapse, calling it a “spooky action at a distance.”35 The collapsing wave function must move faster than the speed of light, indeed, infinite speed.

Figure 15.3. An illustration of the collapse of the wave function of an electron. After passing through a large hole in a screen, the wave function is spread out in a region of space about as big as the hole. After being detected, it collapses to the size of the detector. Note that, in this example, the electron had an equal prior likelihood of being detected by either A or B. Image by the author.
Most common interpretations of quantum mechanics usually are said to be “deterministic” in the sense that the statistical probabilities, given by the wave function or state vector, are predetermined in the theory—analogous to the way that particle motion is predetermined in Newtonian mechanics. However, here it's the collective behavior of an ensemble of identical systems that is determined statistically, not the behavior of an individual system. I prefer to call quantum mechanics “indeterministic.”
Now let's look at some of the alternatives to this view. In the 1950s, David Bohm proposed an interpretation of quantum mechanics, based on a much earlier idea of Louis de Broglie, in which particle motion is predetermined. That motion is controlled by undetected subquantum forces, what de Broglie called “pilot waves.”36 While deterministic in principle, the model based on this interpretation does not predict individual particle motions. It still leads to the same statistical predictions as all other interpretations.37
In his 1957 doctoral dissertation at Princeton University, Hugh Everett III presented a brilliant new mathematical formulation of quantum mechanics. It removed the artificial separation between the quantum and classical realms that existed in the Copenhagen interpretation and it also did away with wave-function collapse.38 Both were significant improvements. Everett's formalism included the detector along with the object being observed in the full quantum system and incorporated all possible outcomes of an experiment.
While Everett's mathematics was impeccable, other authors attempted to give the model a philosophical interpretation in which each time a measurement is performed the universe (really) splits into two separate, disconnected universes.39 This became known as the many-worlds interpretation (MWI) of quantum mechanics.
Consider the situation shown in figure 15.3. The electron has a fifty-fifty chance of being detected at A or B. In the place of wave-function collapse, Everett's mathematical formalism has both possibilities take place. In the many-worlds ontological interpretation, the two events happen in separate universes or “worlds.” In one world, the electron hits A. In the other world, it hits B. If the probability of hitting A is 3/4 and that of hitting B is 1/4, then we have four worlds, three of which have A hit and one of which has B hit. In this way the statistical nature of quantum mechanics is explained.
Indeed, each time an observation is made, the universe is envisaged as splitting into multiple universes, one for each possible outcome of the experiment. However, once again we have an ontological prescription that predicts the same empirical, statistical results as all the other interpretations of quantum mechanics.
While still a graduate student at Princeton in the 1940s, Richard Feynman proposed a mathematical formulation of quantum mechanics called sum-over histories that was quite different from the models that had become standard at that time. (Isn't it wonderful the way physics graduate students are able to solve problems that their more-experienced and much-higher-paid mentors cannot?) In Feynman's model, a wave function or state vector does not even appear, although they can be derived from it.
In the traditional classical procedure, the path of a particle is calculated by applying some equation of motion or, equivalently, a principle such as least action. In Feynman's quantum picture, the particle is assumed to take all possible paths between a source and detector. The probability for a particular observation is then constructed from all the possible histories that could have led to the observation. Although very useful and popular among particle physicists, sum-over histories again led to the same empirical results.
Feynman never attempted to give an ontological interpretation of his model. In Timeless Reality I showed how this notion can be reified using time reversal.40 Although Everett does not reference Feynman, and their mathematics is different, Feynman's model was based on the same idea, namely, incorporate everything that can happen within the formulation. But please note: Just because we have empirically successful models in which everything happens that can happen, it does not follow that everything does happen in empirical reality.
Other interpretations of quantum mechanics exist, including variations on Feynman, that, like his, are not so extravagant as many worlds. The problem with the different interpretations is that some, notably many worlds, skirt very close to metaphysics. All are consistent with the data (those that are not are quickly discarded), but none makes a unique prediction. So we have no way of using the primary principle of scientific method—empirical testing—in order to judge one from the other. Since the calculational methodology we call quantum theory continues to agree with all observations, many of my fellow experimentalists are underwhelmed by the whole discussion and ask, “What else is new?”
Recent authors have seen a possible role for the many-worlds interpretation in cosmology. Recall my discussion of the biverse earlier in this chapter. I stated that what is called the wave function of the universe gives the probability of finding a universe with specific properties. Applying the many-worlds interpretation of quantum mechanics, the wave function of the universe represents an ensemble of parallel universes. Noted string theorist Leonard Susskind and others have suggested that this ensemble of universes can be associated with the many universes of the cosmological multiverse, which Susskind calls the megaverse.41
TIMELESS REALITY
One of the puzzles of the Wheeler-DeWitt equation, which gives the wave function of the universe, is its lack of a time variable. As described earlier in the section on the biverse, in its simplest form the Wheeler-DeWitt equation reduces mathematically to the quantum mechanical time-independent Schrödinger equation for a nonrelativistic particle of mass equal to half the Planck mass and zero total energy and with a single dimension that is just the cosmological scale factor. Solving it is analogous to perhaps the most familiar application of quantum mechanics, calculating the stationary states of the hydrogen atom from the Schrödinger equation. This gives the energy levels of the stable atom. Similarly, the wave function of the universe describes the stationary state of the universe, which has zero total energy.
So where, then, does time enter the picture? How can we have a model of the universe that does not contain that most basic of human conceptions—the passage of time?
In 1983, physicists Don Page and William Wootters wrote that a closed system such as the universe must be in a stationary state and so time is not a necessary element in the description of the world. They show that “the observed dynamical evolution of a system can be described entirely in terms of stationary observables.”42
In a second paper published in 1984, Wootters further explained:
Any statement we would ordinarily make regarding the time dependence of a system can without loss of observational content be cast in the form, “If the clock is found to be in the state…then the probability of finding the system in the state…is…”43
More recently a rash of papers have appeared from both physics and philosophical perspectives describing time as an “emergent phenomenon.”44 They are all highly technical, but I think I can present a simplified basic idea.
In my 2000 book Timeless Reality,45 I showed how the quantum state of a particle at a given place in space is actually a linear superposition of two states, each going in opposite directions in time. The result is that the state of the particle is “timeless.” A measurement of that particle breaks the coherence between the two states, and time “emerges,” although I did not use the term. Note that the process is time-symmetric so the biverse is a natural outcome.
Note also that, as I explained in chapter 6, space and time are closely related. In fact, both are operationally defined in terms of what is read on a clock. So, if time is emergent, so is space. Wootters recognized this and suggested that a workable quantum theory of gravity might be built on the idea of timelessness (and spacelessness).46 This possibility had been suggested even earlier by others, but the idea has not yet achieved fruition.
None of this should be given the mystical interpretation that the mind of a human observer creates time and space as some intrinsic reality. Time and space are names we give to quantities that are registered in the mechanism of a clock and have no meaning without a clock. No human or other sentient being need be involved in the actual measurement.
Once again we witness the futility of trying to attach metaphysical meaning to the quantities in physical models. If you can't read all these papers on emergent time (and emergent gravity), don't worry about it. This is just another example of model-builders seeking ways to logically build the operationally defined quantity we call time into the overarching model of a multiverse described by the timeless Wheeler-DeWitt equation.
ARE MANY WORLDS REAL? WHAT IS REAL?
Cosmologist Max Tegmark has made a strong claim that the many universes of both cosmology and quantum mechanics are part of an ultimate reality that is fundamentally mathematical. He has introduced what he calls the Mathematical Universe Hypothesis (MUH): “Our external physical reality is a mathematical structure.”47
Tegmark defines mathematical structure as “abstract entities with relations between them.” He argues that MUH provides for a description of external reality, a reality independent of us humans, involving “no baggage.” By that he means, “Our descriptions of entities in the external reality and relations between them [are] completely abstract, forgoing any words or other symbols used to denote them to be mere labels with no preconceived meanings whatsoever.”48
I find myself agreeing with Tegmark, with the exception of his calling this scheme “reality.” Based on my different experience as an observer and experimenter, I consider the equations of theorists such as Tegmark to be no more than beautiful and useful descriptions of what we record with our eyes and instruments, analogous to a photograph of the sunset taken from Waikiki Beach. For all its spectacular beauty, the photograph remains a crude human construction that, at best, only images reality. I believe Tegmark's picture is consistent with that interpretation—describing the objects of theoretical physics as mathematical relations between observations. He calls them “reality.” I call them “mathematical relations between observations.”
I am hardly alone in this opinion. In their bestselling book The Grand Design, published in 2010, Stephen Hawking and Leonard Mlodinow say, “There is no picture- or theory-independent concept of reality.” Instead, they endorse a philosophical doctrine they call model-dependent realism, which is “the idea that a physical theory or world-picture is a model (generally of a mathematical nature) and a set of rules that connect the elements of the model to observations.”49 They add, “It is pointless to ask whether a model is real, only whether it agrees with observations.”50
I am not sure how model-dependent realism differs from instrumentalism, which is the view I have promoted in this and previous books. In both cases we concern ourselves only with observations and, while we do not deny that they are the consequence of some ultimate reality, we do not insist that the models describing those observations correspond exactly to that reality.
William Lane Craig seems to think that model-dependent realism is equivalent to ontological pluralism, which is the notion that there are many different, independently valid realities.51 I can see how this ontological doctrine can result from taking our successful theories too seriously, as so many theoretical and experimental physicists do, and assuming they are the “true reality.” But this is not rational. First, theories are always being replaced by better ones. Second, often, as in the case of quantum mechanics, we have multiple interpretations. In both of these cases the different theories and interpretations are often logically inconsistent, although they all fit the same data. If one is real, they all are.
However, I don't think that's what Hawking and Mlodinow are saying at all, as the above quotation makes amply clear. Still, their choice of terms, calling their doctrine model-dependent realism, serves to confuse their position rather than clarify it.
Getting back to the connection between many worlds and the multiverse, at this stage in the game, until some empirical consequence can be extracted from this discussion, the significance of an association between the two becomes a matter of one's philosophical perspective. Are the many worlds in Everett's quantum mechanics “real”? Is the quantum wave function “real”? From my empiricist's perspective, I am not at all disturbed that a collapsing wave function is “spooky.” Casper the Ghost is spooky too, but no more and no less a human creation. All the worlds in Everett's model are likewise human creations—part of a model that successfully describes observations but provides no basis for assuming they all exist in whatever ultimate reality is out there. Similarly, Tegmark's mathematical reality and Hawking's and Mlodinow's model-dependent realism should not be mistaken for whatever ultimate reality may lie beyond our observational verification.
THE MORMON MULTIVERSE
We have seen that Christian theologians going back to Pope Pius XII in the 1950s and exemplified by William Lane Craig today have seized on the big bang as evidence for the creation of a finite universe a finite time ago in accord with the conventional Judeo-Christian-Islamic tradition. Even when the multiverse is brought into the picture, Craig argues that it had to be created ex nihilo as well. However, as we have seen in this chapter, the multiverse is now thought to be eternal.
No doubt the fine art of Christian apologetics will always find ways to reconcile Christian theology with whatever science comes up with, as they did with the teachings of Plato and Aristotle: Pick and choose what you like and ignore what you don't like. Other religions can do likewise.
However, I find it fascinating that Mormon scholars are saying that the multiverse is not only congenial to their particular cosmology but also inherent in it and helpful in resolving some of the difficulties that Mormon cosmology has with single-universe big-bang theory.52
According to Mormon scholar Hyrum Andrus,53 “Revelations to Smith concerning the nature and order of the cosmos indicate that there are innumerable worlds in space populated by organized beings in varying degrees of progressive attainment.”54
And, the universe is eternal. Andrus adds:
Joseph Smith had no part in the prevailing misconception of his day that matter was created out of nothing. Instead, he proclaimed that matter and intelligence are eternal and that God is in time and space as the great Organizer of self-existing matter and things. The Prophet instructed the brethren concerning the “Eternal Duration of Matter,” stating that “the elements are eternal,” that “earth, water, etc., had their existence in an elementary state from eternity,” and that “no part or particle of the great universe could become annihilated or destroyed.”
In any case, just as the cosmologies in the Torah, Old Testament, and Qur’an bear not the slightest resemblance to modern cosmology, neither does that found in the Mormon Book of Abraham, which like the Book of Mormon is regarded as a revelation from God to Joseph Smith. This describes a star or planet called Kolob at or near the throne of God that later Mormon scholars interpreted as the center of the universe.55 Needless to say, there is no empirical evidence for Kolob.
THE MULTIVERSE IN PHILOSOPHY AND LITERATURE
The idea of multiple universes and alternate worlds has been a favorite topic of speculative philosophy and science fiction for many years.56 In a Star Trek episode in the 1960s called “Mirror, Mirror,” Captain Kirk meets his other self. In Philip K. Dick's 1963 novel The Man in the High Castle, America is a Nazi puppet state.57 In Philip Roth's 2004 novel The Plot against America, Nazi sympathizer Charles Lindbergh defeats Franklin Roosevelt for president in 1940.58 Robert Cowley has edited a collection of essays by eminent historians titled What If? In which they envisage what history would have been like had certain major events turned out differently.59
As we saw in chapter 1, multiple worlds were included in the cosmology of the ancient atomists. In Academica, the Roman historian Livy (59 BCE–17 CE), who was not an atomist, wrote:
Would you believe that there exist innumerable worlds…and that just as we are at this moment close to Bauli and are looking towards Puteoli, so there are countless persons in exactly similar spots with our names, our honours, our achievements, our minds, our shapes, our ages, discussing the very same subject?60
In chapter 3, we saw that the great philosopher Leibniz recognized that many alternate worlds were possible. He justified the existence of a benevolent deity in the presence of all the evil and suffering in the world by proposing that this was still “the best of all possible worlds.” He pictured an infinite pyramid with a room for each possible world with ours, the one true world, at the apex.
In Candide, Voltaire ridiculed Leibniz's optimism through the adventures of his title character, who experiences many of the evils and sufferings of human life including the Lisbon earthquake and tsunami of 1755 that killed an estimated 40,000–50,000 people in Portugal, Spain, and Morocco. Leibniz is represented in the tale by Dr. Pangloss, “the greatest philosopher in the Roman Empire,” who continues to promote his optimistic view while suffering many misfortunes himself before being hanged by the Portuguese Inquisition to appease God.61
Finally, the “multiple worlds” mentioned in the previous sections and by the various historical figures described in the early chapters of this book should not be taken too literally as presaging the multiple universes of the scientific multiverse. These individuals were likely thinking in terms of other worlds like Earth, rather than other universes.
In the final chapter we will examine the theological implications of multiple planets and universes.
