Plate 1 Wheatstone’s drawings reproduced as red–green anaglyphs. (See pages 62–3.)

Plate 2 Julesz figure. Dot patterns, computer-generated with a small sideways shift of each dot in a central region of one picture, which in a stereoscope appears above the rest of the random pattern. This is a remarkably useful technique. For example, distortion illusion figures can be presented as dots shared between the eyes, so that neither eye alone can see the figure, though it is seen by the brain combining (fusing) the two sets of dots. If the distortion is still there, its origin must be ‘central’ in the brain, rather than in the retinas. (See page 64.) (From Julesz, Foundations of cyclopean perception (1971).)

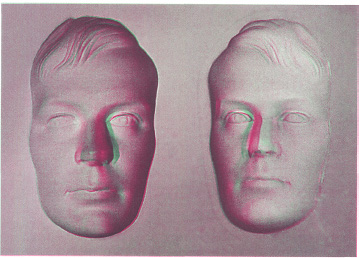
Plate 3 The hollow mask in stereo. The face on the left is normal—sticking out. The face on the right is actually hollow (cf. Figure 10.11). Does stereo information from the two eyes make the face on the right appear truly hollow? Here bottom-up stereo is pitted against top-down knowledge of faces. Which wins? The actually hollow face on the right appears convex like a normal face—in spite of the stereo information from your eyes. (It is better to investigate this with an actual hollow mask, viewed from different distances with both eyes.) (See pages 207–8, 210.)

Plate 4 For each eye this is a random dot pattern. When the patterns are combined by the brain, a figure appears—the Muller–Lyer ‘arrows’, or ‘corners’ are seen (see Figure 10.16) This appears distorted, as usual for the Muller–Lyer illusion. So the origin of the distortion must be in the brain (following fusion of the eyes’ images), not in the retinas. This makes a cognitive explanation probable. (See page 219.) (From Julesz, Foundations of cyclopean perception (1971).)

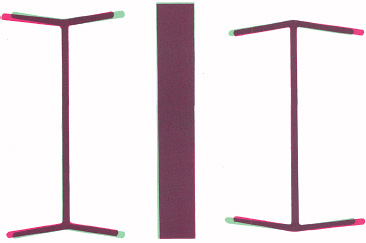
Plate 5 The 3-D appearance of the wire corners of the Muller–Lyer illusion. At the appropriate distance the distortion entirely disappears—when they are seen as corners rather than flat. This confirms that the usual distortion is due to inappropriate size-scaling, set by depth features on a flat surface. (See page 234.)

Plate 6 Poggendorff illusion in 3-D. When the oblique line is seen tilted in depth, the illusion disappears. It is now seen—as it is—as a straight line with no displacement. (See Fig. 10.13, page 213.)