
After Chapter 1.4, you will be able to:
Every change in velocity is motivated by a push or a pull—a force. In this section, we’ll examine how forces interact with one another, as well as how acceleration results from those forces.
Force (F) is a vector quantity that is experienced as pushing or pulling on objects. Forces
can exist between objects that are not even touching. While it is common for forces
to be exerted by one object pushing on another, there are even more instances in which
forces exist between objects nowhere near each other, such as gravity or electrostatic
forces between point charges. The SI unit for force is the newton (N), which is equivalent to one

When Newton observed apples falling out of trees, he was struck by the fact that they always fell perpendicularly to the ground, rather than sideways or even away from the ground. Furthermore, Newton began to wonder about the farthest reaches of gravity. If the apple feels this attractive pull toward the Earth, then what about the Moon? This force is what Newton would later term ‘universal gravitation’.
Acceleration due to gravity, g, decreases with height above the
Earth and increases the closer one gets to the Earth’s center of mass. Near the Earth’s
surface, use
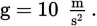
Gravity is an attractive force that is felt by all forms of matter. We usually think of gravity as acting on us to keep us from floating off of the Earth’s surface, or for holding the planets of our solar system in orbit. However, all objects exert gravitational forces on each other; there is a small (but measurable) force of gravity between you and this MCAT Physics and Math Review book, the chair you’re sitting on, and all the objects around you. Gravitational forces usually do not have much significance on a small scale because other forces tend to be much larger in magnitude. Only on the planetary level do gravitational forces really take on a significant value.
Newton’s third law states that the force of gravity on m1 from m2 is equal and opposite to the force of gravity on m2 from m1. This means that the force of gravity on you from the Earth is equal and opposite to the force of gravity from you on the Earth. Because the forces are equal but the masses are very different, the accelerations must also be very different, from F = ma (discussed later in this chapter). Because your mass is very small compared to the Earth, you experience a large acceleration from it. In contrast, because the Earth is massive, it experiences a tiny acceleration from the same magnitude of force.
The magnitude of the gravitational force between two objects is
where G is the universal gravitational constant
 m1 and m2 are the masses of the two objects, and r is the distance between their centers of mass. This equation is commonly tested in
the context of proportionalities. For instance, the magnitude of the gravitational
force is inversely related to the square of the distance (that is, if r is halved, then Fg will quadruple). The magnitude of the gravitational force is also directly related
to the masses of the objects (that is, if m1 is tripled, then Fg will triple).
m1 and m2 are the masses of the two objects, and r is the distance between their centers of mass. This equation is commonly tested in
the context of proportionalities. For instance, the magnitude of the gravitational
force is inversely related to the square of the distance (that is, if r is halved, then Fg will quadruple). The magnitude of the gravitational force is also directly related
to the masses of the objects (that is, if m1 is tripled, then Fg will triple).
Friction is a type of force that opposes the movement of objects. Unlike other kinds of forces, such as gravity or electromagnetic force, which can cause objects either to speed up or slow down, friction forces always oppose an object’s motion and cause it to slow down or become stationary. There are two types of friction: static and kinetic.
Static friction (fs) exists between a stationary object and the surface upon which it rests. The inequality that describes the magnitude of static friction is
where μs is the coefficient of static friction and N is the magnitude of the normal force. The coefficient of static friction is a unitless quantity that is dependent on the two materials in contact. The normal force is the component of the force between two objects in contact that is perpendicular to the plane of contact between the object and the surface upon which it rests.
Note the less-than-or-equal-to signs in the equation. These signify that there is a range of possible values for static friction. The minimum, of course, is zero. This would be the case if an object were resting on a surface with no applied forces. The maximum value of static friction can be calculated from the right side of the previous equation. One should not assume that objects that are stationary are experiencing a maximal static force of friction.
Consider trying to push a heavy piece of luggage. When a 25 N force is applied, the bag does not move. When a 50 N force is applied, the bag still does not move. When a 100 N force is applied, the bag slides a meter or so and slows to a rest. This setup implies that the maximal value of static friction is somewhere between 50 and 100 N; any applied force less than this threshold will not be sufficient to move the bag as there will be an equal but opposite force of static friction opposing the bag’s motion.
Contact points are the places where friction occurs between two rough surfaces sliding past each other. If the normal load—the force that squeezes the two together—rises, the total area of contact increases. That increase, more than the surface’s roughness, governs the degree of friction. This is illustrated in Figure 1.7 below.

Kinetic friction (fk) exists between a sliding object and the surface over which the object slides. Sometimes, students misidentify the presence of kinetic friction. A wheel, for example, that is rolling along a road does not experience kinetic friction because the tire is not actually sliding against the pavement. The tire maintains an instantaneous point of static contact with the road and, therefore, experiences static friction. Only when the tire begins to slide on, say, an icy patch will kinetic friction come into play. Any time two surfaces slide against each other, kinetic friction will be present and its magnitude can be measured according to this equation:
where μk is the coefficient of kinetic friction and N is the normal force. There are two important distinctions between this equation for kinetic friction and the previous equation for static friction. First, the kinetic friction equation has an equals sign. This means that kinetic friction will have a constant value for any given combination of a coefficient of kinetic friction and normal force. It does not matter how much surface area is in contact or even the velocity of the sliding object. Second, the two equations have a different coefficient of friction. The value of μs is always larger than the value of μk. Therefore, the maximum value for static friction will always be greater than the constant value for kinetic friction: objects will “stick” until they start moving, and then will slide more easily over one another.
The coefficient of static friction will always be larger than the coefficient of kinetic friction. It always requires more force to get an object to start sliding than it takes to keep an object sliding.
As previously mentioned in the discussion of static friction, pay close attention to the conditions set in an MCAT passage or question. Does it say that friction can be assumed to be negligible, or does it provide the coefficient of friction values, which will most likely need to be used in a calculation of friction? Friction will be incorporated into our examination of translational equilibrium later in this chapter.
Mass and weight are not the same. Mass (m) is a measure of a body’s inertia—the amount of matter in the object. Mass is a scalar quantity, and, as such, has magnitude only. The SI unit for mass is the kilogram, which is independent of gravity. One kilogram of material on Earth will have the same mass as one kilogram of material on the Moon. Weight (Fg), on the other hand, is a measure of gravitational force (usually that of the Earth) on an object’s mass. Because weight is a force, it is a vector quantity with units in newtons (N).
While mass and weight are not synonymous, they are related by the equation:
where Fg is the weight of the object, m is its mass, and g is acceleration due to gravity,
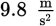 (usually rounded to
(usually rounded to
 ).
).
The weight of an object can be thought of as being applied at a single point in that object called the center of mass or gravity. The MCAT will not directly test your ability to determine center of mass; however, such a calculation may be an important step in a problem with the larger focus of Newtonian mechanics.
To illustrate this concept and calculation, consider a tennis racquet that has been thrown into the air. Each part of the racquet moves in its own pathway, so it’s not possible to represent the motion of the whole racquet as a single particle. However, one point within the racquet moves in a simple parabolic path, very similar to the flight of a ball. It is this point within the racquet that is known as the center of mass. This is clearly shown in Figure 1.8.

For a system in which particles are distributed in all three dimensions, the center of mass is defined by the three coordinates:
where m1, m2, and m3 are the three sample masses, and the x-, y- and z-values are coordinates. The center of gravity is related and corresponds to the single point at which one can conceptualize gravity acting on an object. Only for a homogeneous body (with symmetrical shape and uniform density) should one expect the center of gravity to be located at its geometric center. For example, we can approximate the center of gravity for a metal ball as the geometric center of the sphere. The same cannot be said, however, for a human body, television, or any asymmetrical, non-uniform object.
The center of mass of a uniform object is at the geometric center of the object.
Acceleration (a) is the rate of change of velocity that an object experiences as a result of some applied force. Acceleration, like velocity, is a vector quantity and is measured in SI units of meters per second squared. Acceleration in the direction opposite the initial velocity may be called deceleration. Average acceleration is defined as
where
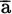 is the average acceleration, Δv is the change in velocity, and Δt is the change in time.
is the average acceleration, Δv is the change in velocity, and Δt is the change in time.
Instantaneous acceleration is defined as the average acceleration as Δt approaches zero.
On a graph of velocity vs. time, the tangent to the graph at any time t, which corresponds to the slope of the graph at that time, indicates the instantaneous acceleration. If the slope is positive, then the acceleration is positive and in the same direction as the velocity. If the slope is negative, then the acceleration is negative and in the opposite direction of the velocity (this is a deceleration).