In mathematics I can report no deficience, except it be that men do not sufficiently understand the excellent use of the Pure Mathematics. . . .
FRANCIS BACON
One can wisely doubt whether the study of mathematics is worth while and can find good authority to support him. As far back as about the year 400 A.D., St. Augustine, Bishop of Hippo in Africa and one of the great fathers of Christianity, had this to say:
The good Christian should beware of mathematicians and all those who make empty prophecies. The danger already exists that the mathematicians have made a covenant with the devil to darken the spirit and to confine man in the bonds of Hell.
Perhaps St. Augustine, with prophetic insight into the conflicts which were to arise later between the mathematically minded scientists of recent centuries and religious leaders, was seeking to discourage the further development of the subject. At any rate there is no question as to his attitude.
At about the same time that St. Augustine lived, the Roman jurists ruled, under the Code of Mathematicians and Evil-Doers, that “to learn the art of geometry and to take part in public exercises, an art as damnable as mathematics, are forbidden.”
Even the distinguished seventeenth-century contributor to mathematics, Blaise Pascal, decided after studying mankind that the pure sciences were not suited to it. In a letter to Fermat written on August 10, 1660, Pascal says: “To speak freely of mathematics, I find it the highest exercise of the spirit; but at the same time I know that it is so useless that I make little distinction between a man who is only a mathematician and a common artisan. Also, I call it the most beautiful profession in the world; but it is only a profession; and I have often said that it is good to make the attempt [to study mathematics], but not to use our forces: so that I would not take two steps for mathematics, and I am confident that you are strongly of my opinion.” Pascal’s famous injunction was, “Humble thyself, impotent reason.”
The philosopher Arthur Schopenhauer, who despised mathematics, said many nasty things about the subject, among others that the lowest activity of the spirit is arithmetic, as is shown by the fact that it can be performed by a machine. Many other great men, for example, the poet Johann Wolfgang Goethe and the historian Edward Gibbon, have felt likewise and have not hesitated to express themselves. And so the student who dislikes the subject can claim to be in good, if not living, company.
In view of the support he can muster from authorities, the student may well inquire why he is asked to learn mathematics. Is it because Plato, some 2300 years ago, advocated mathematics to train the mind for philosophy? Is it because the Church in medieval times taught mathematics as a preparation for theological reasoning? Or is it because the commercial, industrial, and scientific life of the Western world needs mathematics so much? Perhaps the subject got into the curriculum by mistake, and no one has taken the trouble to throw it out. Certainly the student is justified in asking his teacher the very question which Mephistopheles put to Faust:
Is it right, I ask, is it even prudence,
To bore thyself and bore the students?
Perhaps we should begin our answers to these questions by pointing out that the men we cited as disliking or disapproving of mathematics were really exceptional. In the great periods of culture which preceded the present one, almost all educated people valued mathematics. The Greeks, who created the modern concept of mathematics, spoke unequivocally for its importance. During the Middle Ages and in the Renaissance, mathematics was never challenged as one of the most important studies. The seventeenth century was aglow not only with mathematical activity but with popular interest in the subject. We have the instance of Samuel Pepys, so much attracted by the rapidly expanding influence of mathematics that at the age of thirty he could no longer tolerate his own ignorance and begged to learn the subject. He began, incidentally, with the multiplication table, which he subsequently taught to his wife. In 1681 Pepys was elected president of the Royal Society, a post later held by Isaac Newton.
In perusing eighteenth-century literature, one is struck by the fact that the journals which were on the level of our Harper’s and the Atlantic Monthly contained mathematical articles side by side with literary articles. The educated man and woman of the eighteenth century knew the mathematics of their day, felt obliged to be au courant with all important scientific developments, and read articles on them much as modern man reads articles on politics. These people were as much at home with Newton’s mathematics and physics as with Pope’s poetry.
The vastly increased importance of mathematics in our time makes it all the more imperative that the modern person know something of the nature and role of mathematics. It is true that the role of mathematics in our civilization is not always obvious, and the deeper and more complex modern applications are not readily comprehended even by specialists. But the essential nature and accomplishments of the subject can still be understood.
Perhaps we can see more easily why one should study mathematics if we take a moment to consider what mathematics is. Unfortunately the answer cannot be given in a single sentence or a single chapter. The subject has many facets or, some might say, is Hydra-headed. One can look at mathematics as a language, as a particular kind of logical structure, as a body of knowledge about number and space, as a series of methods for deriving conclusions, as the essence of our knowledge of the physical world, or merely as an amusing intellectual activity. Each of these features would in itself be difficult to describe accurately in a brief space.
Because it is impossible to give a concise and readily understandable definition of mathematics, some writers have suggested, rather evasively, that mathematics is what mathematicians do. But mathematicians are human beings, and most of the things they do are uninteresting and some, embarrassing to relate. The only merit in this proposed definition of mathematics is that it points up the fact that mathematics is a human creation.
A variation on the above definition which promises more help in understanding the nature, content, and values of mathematics, is that mathematics is what mathematics does. If we examine mathematics from the standpoint of what it is intended to and does accomplish, we shall undoubtedly gain a truer and clearer picture of the subject.
Mathematics is concerned primarily with what can be accomplished by reasoning. And here we face the first hurdle. Why should one reason? It is not a natural activity for the human animal. It is clear that one does not need reasoning to learn how to eat or to discover what foods maintain life. Man knew how to feed, clothe, and house himself millenniums before mathematics existed. Getting along with the opposite sex is an art rather than a science mastered by reasoning. One can engage in a multitude of occupations and even climb high in the business and industrial world without much use of reasoning and certainly without mathematics. One’s social position is hardly elevated by a display of his knowledge of trigonometry. In fact, civilizations in which reasoning and mathematics played no role have endured and even flourished. If one were willing to reason, he could readily supply evidence to prove that reasoning is a dispensable activity.
Those who are opposed to reasoning will readily point out other methods of obtaining knowledge. Most people are in fact convinced that their senses are really more than adequate. The very common assertion “seeing is believing” expresses the common reliance upon the senses. But everyone should recognize that the senses are limited and often fallible and, even where accurate, must be interpreted. Let us consider, as an example, the sense of sight. How big is the sun? Our eyes tell us that it is about as large as a rubber ball. This then is what we should believe. On the other hand, we do not see the air around us, nor for that matter can we feel, touch, smell, or taste it. Hence we should not believe in the existence of air.
To consider a somewhat more complicated situation, suppose a teacher should hold up a fountain pen and ask, What is it? A student coming from some primitive society might call it a shiny stick, and indeed this is what the eyes see. Those who call it a fountain pen are really calling upon education and experience stored in their minds. Likewise, when we look at a tall building from a distance, it is experience which tells us that the building is tall. Hence the old saying that “we are prone to see what lies behind our eyes, rather than what appears before them.”
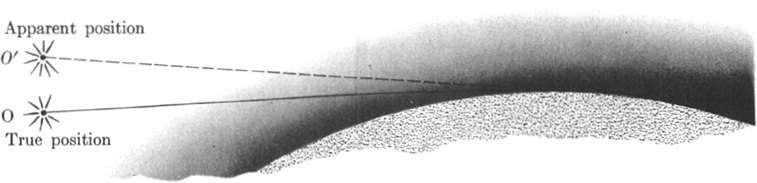
Every day we see the sun where it is not. For about five minutes before what we call sunset, the sun is actually below the geometrical horizon and should therefore be invisible. But the rays of light from the sun curve toward us as they travel in the earth’s atmosphere, and the observer at P (Fig. 1–1) not only “sees” the sun but thinks the light is coming from the direction O′P. Hence he believes the sun is in that direction.
Fig. 1–1.
Deviation of a ray by the earth's atmosphere.
The senses are obviously helpless in obtaining some kinds of knowledge, such as the distance to the sun, the size of the earth, the speed of a bullet (unless one wishes to feel its velocity), the temperature of the sun, the prediction of eclipses, and dozens of other facts.
If the senses are inadequate, what about experimentation or, in simple cases, measurement? One can and in fact does learn a great deal by such means. But suppose one wants to find a very simple quantity, the area of a rectangle. To obtain it by measurement, one could lay off unit squares to cover the area and then count the number of squares. It is at least a little simpler to measure the lengths of the sides and then use a formula obtained by reasoning, namely, that the area is the product of length and width. In the only slightly more complicated problem of determining how high a projectile will go, we should certainly not consider traveling with the projectile.
As to experimentation, let us consider a relatively simple problem of modern technology. One wishes to build a bridge across a river. How long and how thick should the many beams be? What shape should the bridge take? If it is to be supported by cables, how long and how thick should these be? Of course one could arbitrarily choose a number of lengths and thicknesses for the beams and cables and build the bridge. In this event, it would only be fair that the experimenter be the first to cross this bridge.
It may be clear from this brief discussion that the senses, measurement, and experimentation, to consider three alternative ways of acquiring knowledge, are by no means adequate in a variety of situations. Reasoning is essential. The lawyer, the doctor, the scientist, and the engineer employ reasoning daily to derive knowledge that would otherwise not be obtainable or perhaps obtainable only at great expense and effort. Mathematics more than any other human endeavor relies upon reasoning to produce knowledge.
One may be willing to accept the fact that mathematical reasoning is an effective procedure. But just what does mathematics seek to accomplish with its reasoning? The primary objective of all mathematical work is to help man study nature, and in this endeavor mathematics cooperates with science. It may seem, then, that mathematics is merely a useful tool and that the real pursuit is science. We shall not attempt at this stage to separate the roles of mathematics and science and to evaluate the relative merits of their contributions. We shall simply state that their methods are different and that mathematics is at least an equal partner with science.
We shall see later how observations of nature are framed in statements called axioms. Mathematics then discloses by reasoning secrets which nature may never have intended to reveal. The determination of the pattern of motion of celestial bodies, the discovery and control of radio waves, the understanding of molecular, atomic, and nuclear structures, and the creation of artificial satellites are a few basically mathematical achievements. Mathematical formulation of physical data and mathematical methods of deriving new conclusions are today the substratum in all investigations of nature.
The fact that mathematics is of central importance in the study of nature reveals almost immediately several values of this subject. The first is the practical value. The construction of bridges and skyscrapers, the harnessing of the power of water, coal, electricity, and the atom, the effective employment of light, sound, and radio in illumination, communication, navigation, and even entertainment, and the advantageous employment of chemical knowledge in the design of materials, in the production of useful forms of oil, and in medicine are but a few of the practical achievements already attained. And the future promises to dwarf the past.
However, material progress is not the most compelling reason for the study of nature, nor have practical results usually come about from investigations so directed. In fact, to overemphasize practical values is to lose sight of the greater significance of human thought. The deeper reason for the study of nature is to try to understand the ways of nature, that is, to satisfy sheer intellectual curiosity. Indeed, to ask disinterested questions about nature is one of the distinguishing marks of mankind. In all civilizations some people at least have tried to answer such questions as: How did the universe come about? How old is the universe and the earth in particular? How large are the sun and the earth? Is man an accident or part of a larger design? Will the solar system continue to function or will the earth some day fall into the sun? What is light? Of course, not all people are interested in such questions. Food, shelter, sex, and television are enough to keep many happy. But others, aware of the pervasive natural mysteries, are more strongly obsessed to resolve them than any business man is to acquire wealth and power.
Beyond improvement in the material life of man and beyond satisfaction of intellectual curiosity, the study of nature offers intangible values of another sort, especially the abolition of fear and terror and their replacement by a deep, quiet satisfaction in the ways of nature. To the uneducated and to those uninitiated in the world of science, many manifestations of nature have appeared to be agents of destruction sent by angry gods. Some of the beliefs in ancient and even medieval Europe may be of special interest in view of what happened later. The sun was the center of all life. As winter neared and the days became shorter, the people believed that a battle between the gods of light and darkness was taking place. Thus the god Wodan was supposed to be riding through heaven on a white horse followed by demons, all of whom sought every opportunity to harm people. When, however, the days began to lengthen and the sun began to show itself higher in the sky each day, the people believed that the gods of light had won. They ceased all work and celebrated this victory. Sacrifices were offered to the benign gods. Symbols of fertility such as fruit and nuts, whose growth is, of course, aided by the sun, were placed on the altars. To symbolize further the desire for light and the joy in light, a huge log was placed in the fire to burn for twelve days, and candles were lit to heighten the brightness.
The beliefs and superstitions which have been attached to events we take in stride are incredible to modern man. An eclipse of the sun, a threat to the continuance of the light and heat which causes crops to grow, meant that the heavenly body was being swallowed up by a dragon. Many Hindu people believe today that a demon residing in the sky attacks the sun once in a while and that this is what causes the eclipse. Of course, when prayers, sacrifices, and ceremonies were followed by the victory of the sun or moon, it was clear that these rituals were the effective agent and so had to be pursued on every such occasion. In addition, special magic potions drunk during eclipses insured health, happiness, and wisdom.
To primitive peoples of the past, thunder, lightning, and storms were punishments visited by the gods on people who had apparently sinned in some way. The stories in the Old Testament of the flood and of the destruction of Sodom and Gomorrah by fire and brimstone are examples of such acts of wrath by the God of the Hebrews. Hence there was continual concern and even dread about what the gods might have in mind for helpless humans. The only recourse was to propitiate the divine powers, so that they would bring good fortune instead of evil.
Fears, dread, and superstitions have been eliminated, at least in our Western civilization, by just those intellectually curious people who have studied nature’s mighty displays. Those “seemingly unprofitable amusements of speculative brains” have freed us from serfdom, given us undreamed of powers, and, in fact, have replaced negative doctrines by positive mathematical laws which reveal a remarkable order and uniformity in nature. Man has emerged as the proud possessor of knowledge which has enabled him to view nature calmly and objectively. An eclipse of the sun occurring on schedule is no longer an occasion for trembling but for quiet satisfaction that we know nature’s ways. We breathe freely, knowing that nature will not be willful or capricious.
Indeed, man has been remarkably successful in his study of nature. History is said to repeat itself, but, in general, the circumstances of the supposed repetition are not the same as those of the earlier occurrence. As a consequence, the history of man has not been too effective a guide for the future. Nature is kinder. When nature repeats herself, and she does so constantly, the repetitions are exact facsimiles of previous events, and therefore man can anticipate nature’s behavior and be prepared for what will take place. We have learned to recognize the patterns of nature and we can speak today of the uniformity of nature and delight in the regularity of her behavior.
The successes of mathematics in the study of inanimate nature have inspired in recent times the mathematical study of human nature. Mathematics has not only contributed to the very practical institutions such as banking, insurance, pension systems, and the like, but it has also supplied some substance, spirit, and methodology to the infant sciences of economics, politics, and sociology. Number, quantitative studies, and precise reasoning have replaced vague, subjective, and ineffectual speculations and have already given evidence of greater values to come.
As man turns to thoughts about himself and his fellow man, other questions occur to him which are as fundamental as any he can ask. Why is man born? What purposes does he serve or should he serve? What future awaits him? The knowledge acquired about our physical universe has profound implications for the origin and role of man. Moreover, as mathematics and science have amassed increasing knowledge and power, they have gradually encompassed the biological and psychological sciences, which in turn have shed further light on man’s physical and mental life. Thus it has come about that mathematics and science have profoundly affected philosophy and religion.
Perhaps the most profound questions in the realm of philosophy are, What is truth and how does man acquire it? Though we have no final answer to these questions, the contribution of mathematics toward this end is paramount. For two millenniums mathematics was the prime example of truths man had unearthed. Hence all investigations of the problem of acquiring truths necessarily reckoned with mathematics. Though some startling developments in the nineteenth century altered completely our understanding of the nature of mathematics, the effectiveness of the subject, especially in representing and analyzing natural phenomena, has still kept mathematics the focal point of all investigations into the nature of knowledge. Not the least significant aspect of this value of mathematics has been the insight it has given us into the ways and powers of the human mind. Mathematics is the supreme and most remarkable example of the mind’s power to cope with problems, and as such it is worthy of study.
Among the values which mathematics offers are its services to the arts. Most people are inclined to believe that the arts are independent of mathematics, but we shall see that mathematics has fashioned major styles of painting and architecture, and the service mathematics renders to music has not only enabled man to understand it, but has spread its enjoyment to all corners of our globe.
Practical, scientific, philosophical, and artistic problems have caused men to investigate mathematics. But there is one other motive which is as strong as any of these — the search for beauty. Mathematics is an art, and as such affords the pleasures which all the arts afford. This last statement may come as a shock to people who are used to the conventional concept of the true arts and mentally contrast these with mathematics to the detriment of the latter. But the average person has not thought through what the arts really are and what they offer. All that many people actually see in painting, for example, are familiar scenes and perhaps bright colors. These qualities, however, are not the ones which make painting an art. The real values must be learned, and a genuine appreciation of art calls for much study.
Nevertheless, we shall not insist on the aesthetic values of mathematics. It may be fairer to rest on the position that just as there are tone-deaf and color-blind people, so may there be some who temperamentally are intolerant of cold argumentation and the seemingly overfine distinctions of mathematics.
To many people, mathematics offers intellectual challenges, and it is well known that such challenges do engross humans. Games such as bridge, crossword puzzles, and magic squares are popular. Perhaps the best evidence is the attraction of puzzles such as the following: A wolf, a goat, and cabbage are to be transported across a river by a man in a boat which can hold only one of these in addition to the man. How can he take them across so that the wolf does not eat the goat or the goat the cabbage? Two husbands and two wives have to cross a river in a boat which can hold only two people. How can they cross so that no woman is in the company of a man unless her husband is also present? Such puzzles go back to Greek and Roman times. The mathematician Tartaglia, who lived in the sixteenth century, tells us that they were after-dinner amusements.
People do respond to intellectual challenges, and once one gets a slight start in mathematics, he encounters these in abundance. In view of the additional values to be derived from the subject, one would expect people to spend time on mathematical problems as opposed to the more superficial, and in some instances cheap, games which lack depth, beauty, and importance. The tantalizing and compelling pursuit of mathematical problems offers mental absorption, peace of mind amid endless challenges, repose in activity, battle without conflict, and the beauty which the ageless mountains present to senses tried by the kaleidoscopic rush of events. The appeal offered by the detachment and objectivity of mathematical reasoning is superbly described by Bertrand Russell.
Remote from human passions, remote even from the pitiful facts of nature, the generations have gradually created an ordered cosmos, where pure thought can dwell as in its natural home and where one, at least, of our nobler impulses can escape from the dreary exile of the actual world.
The creation and contemplation of mathematics offer such values.
Despite all these arguments for the study of mathematics, the reader may have justifiable doubts. The idea that thinking about numbers and figures leads to deep and powerful conclusions which influence almost all other branches of thought may seem incredible. The study of numbers and geometrical figures may not seem a sufficiently attractive and promising enterprise. Not even the founders of mathematics envisioned the potentialities of the subject.
So we start with some doubts about the worth of our enterprise. We could encourage the reader with the hackneyed maxim, nothing ventured, nothing gained. We could call to his attention the daily testimony to the power of mathematics offered by almost every newspaper and journal. But such appeals are hardly inspiring. Let us proceed on the very weak basis that perhaps those more experienced in what the world has to offer may also have the wisdom to recommend worth-while studies.
Hence, despite St. Augustine, the reader is invited to tempt hell and damnation by engaging in a study of the subject. Certainly he can be assured that the subject is within his grasp and that no special gifts or qualities of mind are needed to learn mathematics. It is even debatable whether the creation of mathematics requires special talents as does the creation of music or great paintings, but certainly the appreciation of what others have done does not demand a “mathematical mind” any more than the appreciation of art requires an “artistic mind.” Moreover, since we shall not draw upon any previously acquired knowledge, even this potential source of trouble will not arise.
Let us review our objectives. We should like to understand what mathematics is, how it functions, what it accomplishes for the world, and what it has to offer in itself. We hope to see that mathematics has content which serves the physical and social scientist, the philosopher, logician, and the artist; content which influences the doctrines of the statesman and the theologian; content which satisfies the curiosity of the man who surveys the heavens and the man who muses on the sweetness of musical sounds; and content which has undeniably, if sometimes imperceptibly, shaped the course of modern history. In brief, we shall try to see that mathematics is an integral part of the modern world, one of the strongest forces shaping its thoughts and actions, and a body of living though inseparably connected with, dependent upon, and in turn valuable to all other branches of our culture. Perhaps we shall also see how by suffusing and influencing all thought it has set the intellectual temper of our times.
1. A wolf, a goat, and a cabbage are to be rowed across a river in a boat holding only one of these three objects besides the oarsman. How should he carry them across so that the goat should not eat the cabbage or the wolf devour the goat?
2. Another hoary teaser is the following: A man goes to a tub of water with two jars, one holding 3 pt and the other 5 pt. How can he bring back exactly 4 pt?
3. Two husbands and two wives have to cross a river in a boat which can hold only two people. How can they cross so that no woman is in the company of a man unless her husband is also present?
RUSSELL, BERTRAND: “The Study of Mathematics,” an essay in the collection entitled Mysticism and Logic, Longmans, Green and Co., New York, 1925.
WHITEHEAD, ALFRED NORTH: “The Mathematical Curriculum,” an essay in the collection entitled The Aims of Education, The New American Library, New York, 1949.
WHITEHEAD, ALFRED NORTH: Science and the Modern World, Chaps. 2 and 3, Cambridge University Press, Cambridge, 1926.