“Invention is the most important product of man’s creative brain. The ultimate purpose is the complete mastery of mind over the material world, the harnessing of human nature to human needs.”
—Nikola Tesla
Long before the knowledge of electricity, many people were aware of the electric force. Descriptions of electricity were reported by Egyptians, Greeks, and Romans. Electricity would remain a mystery until 1600, when English scientist William Gilbert made a careful study of electricity and magnetism. Further work was continued by other notable scientists including Ben Franklin (his famous metal key and kite experiment in a storm). Real progress came in the 19th century, with regard to electricity and magnetism, from Hans Christian Orsted, Andre-Marie Ampere, Michael Faraday, and George Ohm. James Clerk Maxwell would definitively link them together into the concept electromagnetism.
The basic components of atoms are protons, neutrons, and electrons. Protons and neutrons form the nucleus (and are referred to collectively as nucleons), while the electrons keep their distance, swarming around the nucleus. Most of an atom consists of empty space. In fact, if a nucleus were the size of the period at the end of this sentence, then the electrons would be five meters away. So what holds such an apparently tenuous structure together? One of the most powerful forces in nature: the electromagnetic force. Protons and electrons have a quality called electric charge that gives them an attractive force. Electric charge comes in two varieties: positive and negative. A positive particle always attracts a negative particle, and particles of the same charge always repel each other. Protons are positively charged, and electrons are negatively charged.
Protons and electrons are intrinsically charged, but bulk matter is not. This is because the amount of charge on a proton exactly balances the charge on an electron, which is quite remarkable in light of the fact that protons and electrons are very different particles. Since most atoms contain an equal number of protons and electrons, their overall electric charge is 0, because the negative charges cancel out the positive charges. Therefore, in order for matter to be charged, an imbalance between the numbers of protons and electrons must exist. This can be accomplished by either the removal or addition of electrons (that is, by the ionization of some of the object’s atoms). If you remove electrons, then the object becomes positively charged, while if you add electrons, then it becomes negatively charged. Furthermore, charge is conserved. For example, if you rub a glass rod with a piece of silk, then the silk will acquire a negative charge and the glass will be left with an equal positive charge. Net charge cannot be created or destroyed. (Charge can be created or destroyed—it happens all the time—but net charge cannot.)
The magnitude of charge on an electron (and therefore on a proton) is denoted e. This stands for elementary charge because it’s the basic unit of electric charge. The charge of an ionized atom must be a whole number times e because charge can be added or subtracted only in lumps of size e. For this reason, we say that charge is quantized. To remind us of the quantized nature of electric charge, the charge of a particle (or object) is denoted by the letter q. In the SI system of units, charge is expressed in coulombs (abbreviated C). One coulomb is a tremendous amount of charge; the value of e is about 1.6 × 10−19 C.
Charge is quantized
q = n(±e)
where n = 0, 1, 2…
The electric force between two charged particles obeys a law that is very similar to that describing the gravitational force between two masses; they are both inverse-square laws. The electric force between two particles with charges of q1 and q2, separated by a distance r, is given by the equation
This is Coulomb’s Law. We interpret a negative FE as an attraction between the charges and a positive FE as a repulsion. The value of the proportionality constant, k, depends on the material between the charged particles. In empty space (vacuum)—or air, for all practical purposes—it is called Coulomb’s constant and has the approximate value k0 = 9 × 109 N · m2/C2. For reasons that will become clear later in this chapter, k0 is usually written in terms of a fundamental constant known as the permittivity of free space, denoted ε0, whose numerical value is approximately 8.85 × 10−12 C2/(N · m2). The equation that gives k0 in terms of ε0 is
Coulomb’s Law for the force between two point charges is then written as
Recall that the value of the universal gravitational constant, G, is 6.67 × 10−11 N · m2/kg2. The relative sizes of these fundamental constants show the relative strengths of the electric and gravitational forces. The value of k0 is 20 orders of magnitude larger than G.
Example 1 Consider two small spheres, one carrying a charge of + 1.5 nC and the other a charge of −2.0 nC, separated by a distance of 1.5 cm. Find the electric force between them. (“n” is the abbreviation for “nano,” which means 10−9.)
Solution. The electric force between the spheres is given by Coulomb’s Law:
The fact that FE is negative means that the force is one of attraction, which we naturally expect, since one charge is positive and the other is negative. The force between the spheres is along the line that joins the charges, as we’ve illustrated below. The two forces shown form an action/reaction pair.
Consider three point charges: q1, q2, and q3. The total electric force acting on, say, q2 is simply the sum of F1-on-2, the electric force on q2 due to q1, and F3-on-2, the electric force on q2 due to q3:
Fon 2 = F1-on-2 + F3-on-2
The fact that electric forces can be added in this way is known as superposition.
This is also true for more than two charges, for n charges:
Fon 2 = F1-on-2 + F3-on-2 + F4-on-2 +…+ Fn-on-2
Example 2 Consider four equal, positive point charges that are situated at the vertices of a square. Find the net electric force on a negative point charge placed at the square’s center.
Solution. Refer to the diagram below. The attractive forces due to the two charges on each diagonal cancel out: F1 + F3 = 0, and F2 + F4 = 0, because the distances between the negative charge and the positive charges are all the same and the positive charges are all equivalent. Therefore, by symmetry, the net force on the center charge is zero.
Example 3 If the two positive charges on the bottom side of the square in the previous example were removed, what would be the net electric force on the negative charge? Assume that each side of the square is 4.0 cm, each positive charge is 1.5 µC, and the negative charge is −6.2 nC. (μ is the symbol for “micro-,” which equals 10−6.)
Solution. If we break down F1 and F2 into horizontal and vertical components, then by symmetry the two horizontal components will cancel each other out, and the two vertical components will add
Since the diagram on the left shows the components of F1 and F2 making right triangles with legs each of length 2 cm, it must be that F1y = F1 sin 45° and F2y = F2 sin 45°. Also, the magnitude of F1 equals that of F2. So the net electric force on the negative charge is F1y + F2y = 2F sin 45°, where F is the strength of the force between the negative charge and each of the positive charges. If s is the length of each side of the square, then the distance r between each positive charge and the negative charge is r = 
and
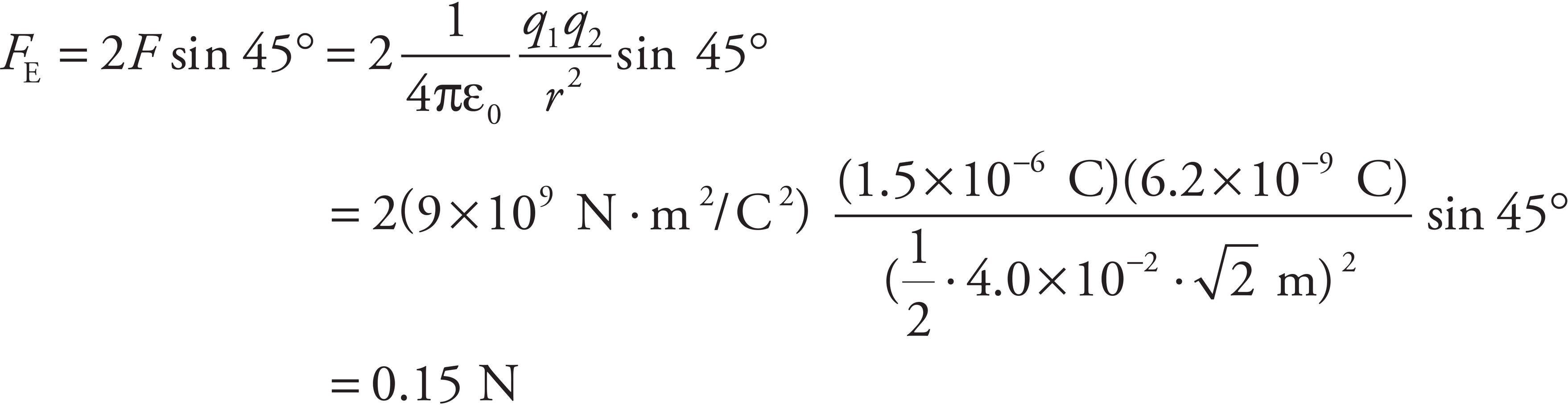
The direction of the net force is straight upward, toward the center of the line that joins the two positive charges.
Example 4 Two pith balls of mass m are each given a charge of +q. They are hung side-by-side from two threads each of length L, and move apart as a result of their electrical repulsion. Find the equilibrium separation distance x in terms of m, q, and L. (Use the fact that if θ is small, then tan θ ≈ sin θ.)
Solution. Three forces act on each ball: weight, tension, and electrical repulsion.
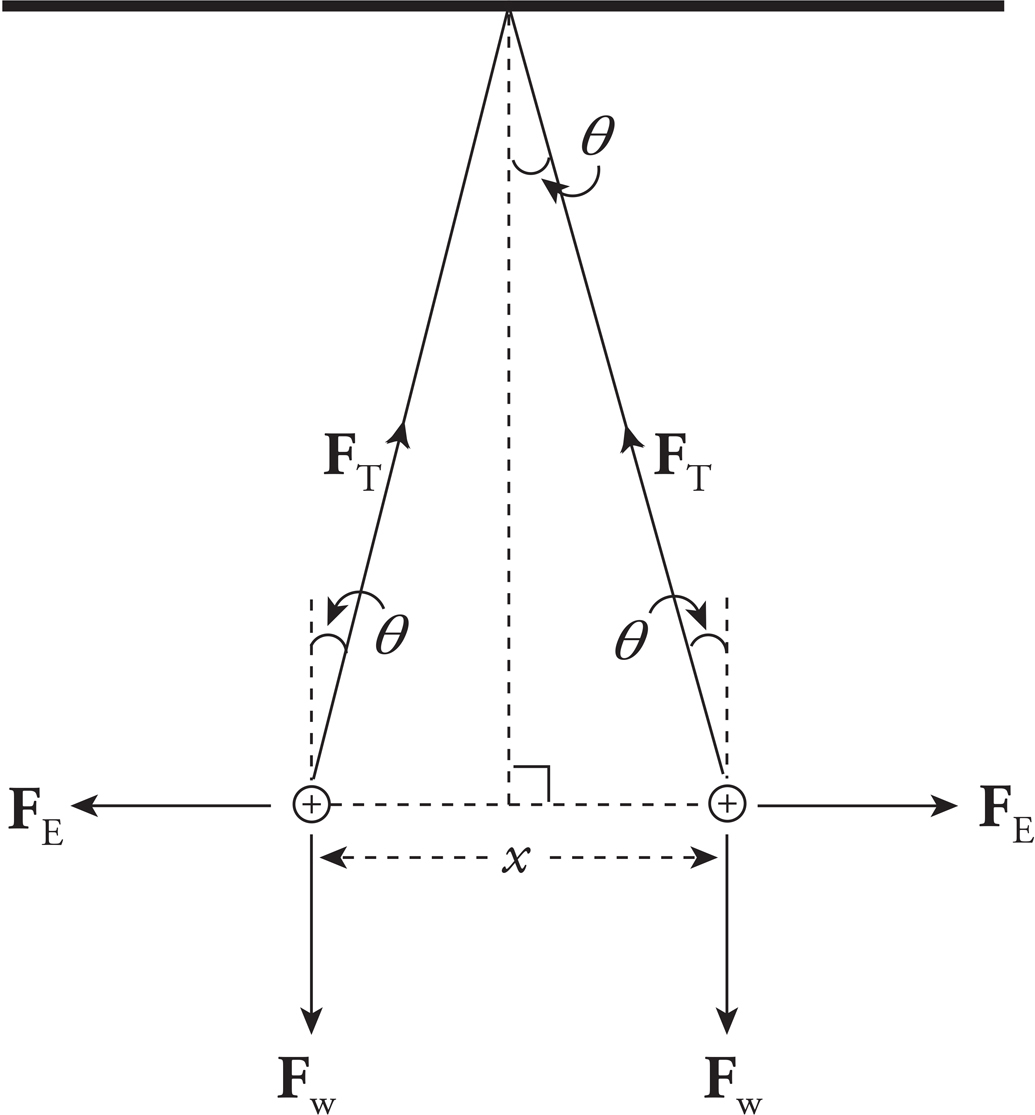
When the balls are in equilibrium, the net force each feels is zero. Therefore, the vertical component of FT must cancel out Fw and the horizontal component of FT must cancel out FE:
FT cos θ = Fw and FT sin θ = FE
Dividing the second equation by the first, we get tan θ = FE/Fw. Therefore,
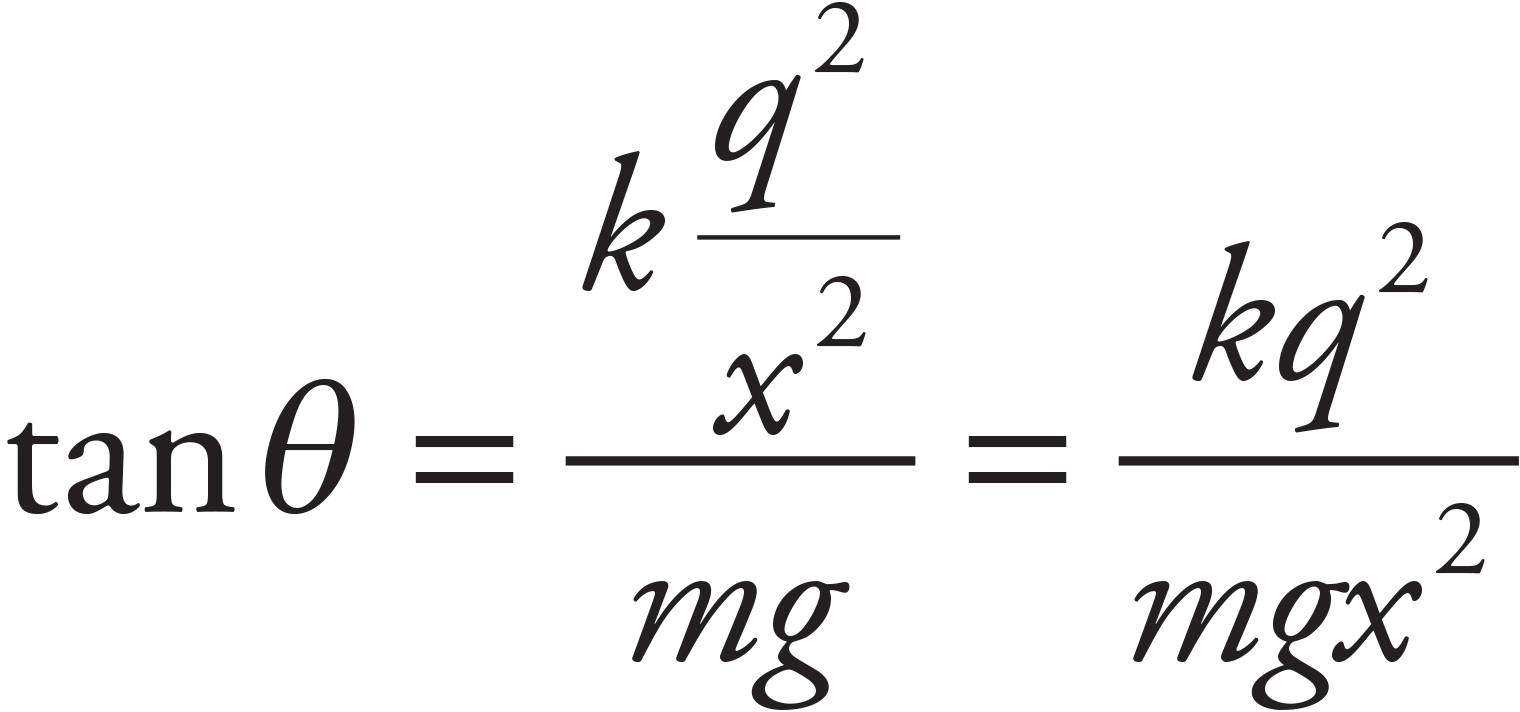
Now, to approximate: if θ is small, then tan θ ≈ sin θ and, from the diagram, sin θ = 
x/L. Therefore, the equation above becomes
The presence of a massive body such as the Earth causes objects to experience a gravitational force directed toward the Earth’s center. For objects located outside the Earth, this force varies inversely with the square of the distance and directly with the mass of the gravitational source. A vector diagram of the gravitational field surrounding the Earth looks like this:
We can think of the space surrounding the Earth as permeated by a gravitational field that’s created by the Earth. Any mass that’s placed in this field then experiences a gravitational force due to this field.
The same process is used to describe the electric force. Rather than having two charges reach out across empty space to each other to produce a force, we can instead interpret the interaction in the following way: the presence of a charge creates an electric field in the space that surrounds it. Another charge placed in the field created by the first will experience a force due to the field.
Consider a point charge Q in a fixed position and assume that it is positive. Now imagine moving a tiny positive test charge q around to various locations near Q. At each location, measure the force that the test charge experiences, and call it Fon q. Divide this force by the test charge q; the resulting vector is the electric field vector, E, at that location:
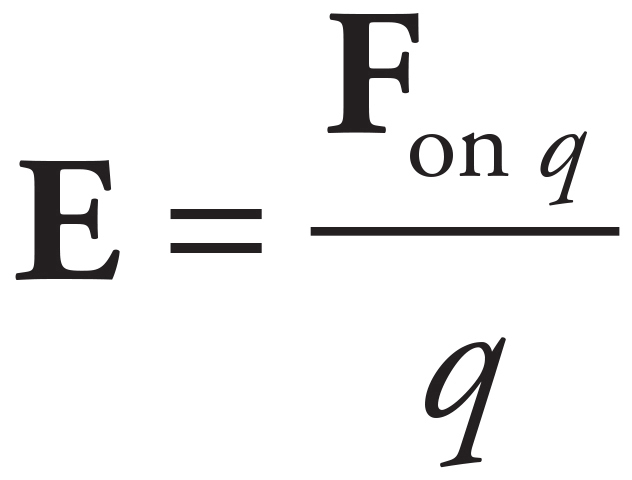
The reason for dividing by the test charge is simple. If we were to use a different test charge with, say, twice the charge of the first one, then each of the forces F we’d measure would be twice as much as before. But when we divided this new, stronger force by the new, greater test charge, the factors of 2 would cancel, leaving the same ratio as before. So this ratio tells us the intrinsic strength of the field due to the source charge, independent of whatever test charge we may use to measure it.
What would the electric field of a positive charge Q look like? Since the test charge used to measure the field is positive, every electric field vector would point radially away from the source charge. If the source charge is positive, the electric field vectors point away from it; if the source charge is negative, then the field vectors point toward it. And, since the force decreases as we get farther away from the charge (as 1/r2), so does the electric field. This is why the electric field vectors farther from the source charge are shorter than those that are closer.
Since the force on the test charge q has a strength of qQ/4πε0r2, when we divide this by q, we get the expression for the strength of the electric field created by a point-charge source of magnitude Q:
To make it easier to sketch an electric field, lines are drawn through the vectors such that the electric field vector is tangent to the line everywhere it’s drawn.
Now, your first thought might be that obliterating the individual field vectors deprives us of information, since the length of the field vectors told us how strong the field was. Well, although the individual field vectors are gone, the strength of the field can be figured out by looking at the density of the field lines. Where the field lines are denser, the field is stronger.
Electric field vectors can be added like any other vectors. If we had two source charges, their fields would overlap and effectively add; a third charge wandering by would feel the effect of the combined field. At each position in space, add the electric field vector due to one of the charges to the electric field vector due to the other charge: Etotal = E1 + E2. (This is another instance of superposition.) In the diagram below, E1 is the electric field vector at a particular location due to the charge +Q, and E2 is the electric field vector at that same location due to the other charge, −Q. Adding these vectors gives the overall field vector Etotal at that location.
If this is done at enough locations, the electric field lines can be sketched.
Note that, like electric field vectors, electric field lines always point away from positive source charges and toward negative ones. Two equal but opposite charges, like the ones shown in the diagram above, form a pair called an electric dipole.
If a positive charge +q were placed in the electric field above, it would experience a force that is in the same direction as the field line passing through +q’s location. After all, electric fields are sketched from the point of view of what a positive test charge would do. On the other hand, if a negative charge −q were placed in the electric field, it would experience a force that is in the direction opposite from the field line passing through −q’s location.
Finally, notice that electric field lines never cross.
The force generated on a charge that is in an electric field is given by the equation:
F = qE
Example 5 A charge q = +3.0 nC is placed at a location at which the electric field strength is 400 N/C. Find the force felt by the charge q.
Solution. From the definition of the electric field, we have the following equation:
Fon q = qE
Therefore, in this case, Fon q = qE = (3 × 10−9 C)(400 N/C) = 1.2 × 10−6 N.
Example 6 A dipole is formed by two point charges, each of magnitude 4.0 nC, separated by a distance of 6.0 cm. What is the strength of the electric field at the point midway between them?
Solution. Let the two source charges be denoted +Q and −Q. At Point P, the electric field vector due to +Q would point directly away from +Q, and the electric field vector due to −Q would point directly toward −Q. Therefore, these two vectors point in the same direction (from +Q to −Q), so their magnitudes would add.
Using the equation for the electric field strength due to a single point charge, we find that
Example 7 If a charge q = −5.0 pC were placed at the midway point described in the previous example, describe the force it would feel. (“p” is the abbreviation for “pico-,” which means 10−12.)
Solution. Since the field E at this location is known, the force felt by q is easy to calculate:
Fon q = qE = (−5.0 × 10−12 C)(8.0 × 104 N/C to the right) = 4.0 × 10−7 N to the left
Example 8 What can you say about the electric force that a charge would feel if it were placed at a location at which the electric field was zero?
Solution. Remember that Fon q = qE. So if E = 0, then Fon q = 0. (Zero field means zero force.)
An important subset of problems with electric fields deals with a uniform electric field. One method of creating this uniform field is to have two large conducting sheets, each storing charge, some distance apart. Near the edges of each sheet, the field may not be uniform, but near the middle, for all practical purposes the field is uniform. Having a uniform field means you can use kinematics equations and Newton’s laws just as if you had a uniform gravitational field (as in previous chapters).
Example 9 Positive charge is distributed uniformly over a large, horizontal plate, which then acts as the source of a vertical electric field. An object of mass 5 g is placed at a distance of 2 cm above the plate. If the strength of the electric field at this location is 106 N/C, how much charge would the object need to have in order for the electrical repulsion to balance the gravitational pull?
Solution. Clearly, since the plate is positively charged, the object would also have to carry a positive charge so that the electric force would be repulsive.
Let q be the charge on the object. Then, in order for FE to balance mg, we must have
Example 10 A proton, neutron, and electron are in a uniform electric field of 20 N/C that is caused by two large charged plates that are 30 cm apart. The particles are far enough apart that they don’t interact with each other. They are released from rest equidistant from each plate.
(a) What is the magnitude of the net force acting on each particle?
(b) What is the magnitude of the acceleration of each particle?
(c) How much work will be done on the particle when it collides with one of the charged plates?
(d) What is the speed of each particle when it strikes the plate?
(e) How long does it take to reach the plate?
Solution.
(a) Since E = 
, F = qE. Plugging in the values, we get
proton: F = (1.6 × 10−19 C)(20 N/C) = 3.2 × 10−18 N
electron: F = (1.6 × 10−19 C)(20 N/C) = 3.2 × 10−18 N
neutron: F = (0 C)(20 N/C) = 0 N
Note: Because the proton and electron have the same magnitude, they will experience the same force. If you’re asked for the direction, the proton travels in the same direction as the electric field and the electron travels in the opposite direction as the electric field. (This is as per the convention for electric fields.)
(b) Since F = ma, a = 
. Plugging in the values, we get
proton: a = 
electron: a = 
neutron: a = 
Notice that although the charges have the same magnitude of force, the electron experiences an acceleration almost 2,000 times greater due to its mass being almost 2,000 times smaller than the proton’s mass.
(c) Since W = Fd, we get W = qEd. Plugging in the values, we get
proton: W = (1.6 × 10−19 C)(20 N/C)(0.15 m) = 4.8 × 10−19 N
electron: W = (1.6 × 10−19 C)(20 N/C)(0.15 m) = 4.8 × 10−19 N
neutron: W = (0 C)(20 N/C)(0.15 m) = 0 N
(d) Recall one of the big five kinematics equations:
If the particles are midway between the 30 cm plates, they will travel 0.15 m.
Notice that, even though the force is the same and the same work is done on both charges, there is a significant difference in final velocities due to the large mass difference. An alternative solution to this would be using W = ΔKE → W =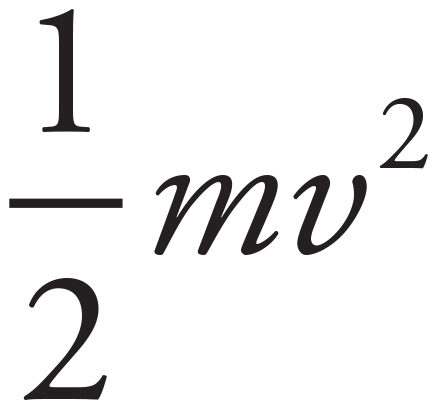 
. You would have obtained the same answers.
(e) Recall one of the big five kinematics equations:
Answers and explanations can be found in Chapter 13.
1. If the distance between two positive point charges is tripled, then the strength of the electrostatic repulsion between them will decrease by a factor of
(A) 3
(B) 6
(C) 8
(D) 9
2. Two 1 kg spheres each carry a charge of magnitude 1 C. How does FE, the strength of the electric force between the spheres, compare to FG, the strength of their gravitational attraction?
(A) FE < FG
(B) FE = FG
(C) FE > FG
(D) If the charges on the spheres are of the same sign, then FE > FG; but if the charges on the spheres are of the opposite sign, then FE < FG.
3. The figure below shows three point charges, all positive. If the net electric force on the center charge is zero, what is the value of y/x ?
(A) 
(B) 
(C) 
(D) 
4. The figure above shows two point charges, +Q and −Q. If the negative charge were absent, the electric field at Point P due to +Q would have strength E. With −Q in place, what is the strength of the total electric field at P, which lies at the midpoint of the line segment joining the charges?
(A) 0
(B) 
(C) E
(D) 2E
5. A sphere of charge +Q is fixed in position. A smaller sphere of charge +q is placed near the larger sphere and released from rest. The small sphere will move away from the large sphere with
(A) decreasing velocity and decreasing acceleration
(B) decreasing velocity and increasing acceleration
(C) increasing velocity and decreasing acceleration
(D) increasing velocity and increasing acceleration
6. An object of charge +q feels an electric force FE when placed at a particular location in an electric field, E. Therefore, if an object of charge −2q were placed at the same location where the first charge was, it would feel an electric force of
(A)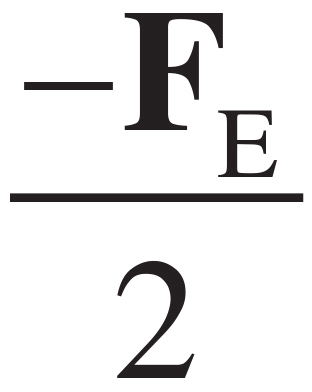 
(B) −2FE
(C) −2qFE
(D) 
7. A charge of −3Q is transferred to a solid metal sphere of radius r. Where will this excess charge reside?
(A) −Q at the center, and −2Q on the outer surface
(B) −3Q at the center
(C) −3Q on the outer surface
(D) −Q at the center, −Q in a ring of radius 
r, and −Q on the outer surface
8. How far apart are two charges (q1 = 8 × 10−6 C and q2 = 6 × 10−6 C) if the electric force exerted by the charges on each other has a magnitude of 2.7 × 10−2 N ?
(A) 1 m
(B) 2 m
(C) 3 m
(D) 4 m
9. Two charges (q1 and q2) are separated by a distance r. If the ratio of FG/FE is equal to 9.0 × 1043, what is the new ratio if the distance between the two charges is now 3r ?
(A) 1.0 × 1043
(B) 3.0 × 1043
(C) 9.0 × 1043
(D) 27.0 × 1043
1. In the figure shown, all four charges (+Q, +Q, −q, and −q) are situated at the corners of a square. The net electric force on each charge +Q is zero.
(a) Express the magnitude of q in terms of Q.
(b) Is the net electric force on each charge −q also equal to zero? Justify your answer.
(c) Determine the electric field at the center of the square.
2. Two charges, +Q and +2Q, are fixed in place along the y-axis of an x-y coordinate system as shown in the figure below. Charge 1 is at the point (0, a), and Charge 2 is at the point (0, −2a).
(a) Find the electric force (magnitude and direction) felt by Charge 1 due to Charge 2.
(b) Find the electric field (magnitude and direction) at the origin created by both Charges 1 and 2.
(c) Is there a point on the x-axis where the total electric field is zero? If so, where? If not, explain briefly.
(d) Is there a point on the y-axis where the total electric field is zero? If so, where? If not, explain briefly.
(e) If a small negative charge, −q, of mass m were placed at the origin, determine its initial acceleration (magnitude and direction).
3. Two point charges (q1 = +4 μC and q2 = +3 μC) are fixed in place according to the figure below.
(a) What are the magnitudes of the horizontal and vertical components of the electric field at the point (0, 4)?
(b) What are the magnitudes of the horizontal and vertical components of the electric force on a charge q3 = −2 μC placed at the point (0, 4)?
(c) In which quadrant would a positive charge q4 have to be placed in order for the electric field at point (0, 4) to be equal to 0?
Coulomb’s Law describes the force acting on two point charges and is given by
where 
is a constant equal to 9.0 × 109 N · m2/C2. You can use all the strategies you used in the Newton’s laws chapter to solve these types of problems.
The electric field is given by 
or 
.
Both the electric force and field are vector quantities and therefore all the rules for vector addition apply.