CHAPTER 9
6. Radians, Chords, Arcs, and Sectors
12. Imaginary and Complex Numbers
The SAT Math Test: Additional Topics
What other special topics are included on the SAT Math test?
About 10% (6 out of 58 points) of the SAT Math questions are “Additional Topics” questions. These include topics like
• analyzing triangles using the Pythagorean Theorem
• graphing circles and other figures in the xy-plane
• analyzing areas, circumferences, chords, and sectors of circles
• measuring angles and arcs in radians
• working with area and volume and their formulas
• using the theorems of congruence and similarity
• working with basic trigonometric relationships including cofunction identities
• calculating with imaginary and complex numbers
Why are these topics important?
These topics from geometry, trigonometry, and advanced analysis are crucial to work in engineering, physics, architecture, and even design. Although they are not essential to every college major, they do provide tools for understanding and analyzing advanced concepts across the curriculum.
Sound intimidating? It’s not.
Some of you have already spent some time in math class studying these topics. If not, the three skills described in these 12 lessons will give you the knowledge and practice you need to master them.
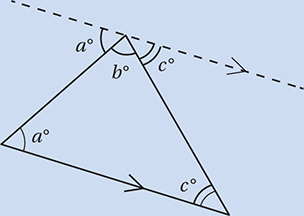
Lesson 1: Angles and Parallel Lines
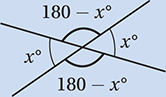
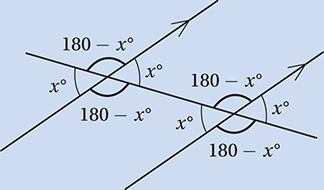
The Intersecting Lines Theorem
The Parallel Lines Theorem
The “ZCUF” Angles

In the figure above, ABCD is a parallelogram, and point B lies on  . If x = 40, what is the value of y ?
. If x = 40, what is the value of y ?
A) 40
B) 50
C) 60
D) 70
(Medium) Since ABCD is a parallelogram, we can use the Parallel Lines Theorem. Let’s mark the diagram with what we know. Note that the consecutive angles in the parallelogram (such as D and C, or D and A) are “C” or “U” angle pairs.
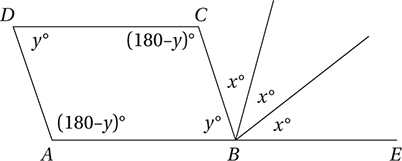
The Parallel Lines Theorem proves that in a parallelogram, consecutive angles are supplementary and opposite angles are congruent.
Since  is a straight (180°) angle:
is a straight (180°) angle:
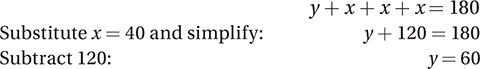
Therefore, the correct answer is C.
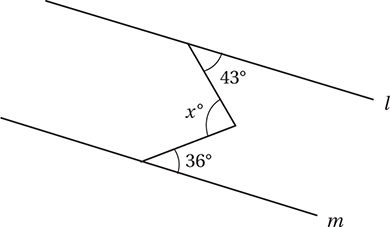
If lines l and m are parallel in the figure above, what is the value of x ?
A) 43
B) 79
C) 86
D) 101
(Hard) There are no obvious “ZCUF” angles, but we can fix that by drawing a line parallel to l and m through the vertex.
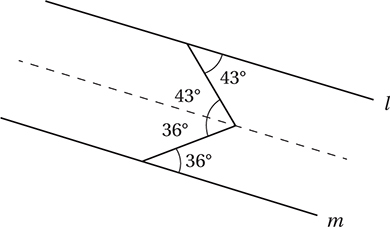
Now we have two Z-shaped pairs of angles, so the middle angle is the sum of two smaller angles of 36° and 43°, and therefore x = 36 + 43 = 79, and the correct answer is B.

Which of the following statements, if true, is sufficient to prove that line k is parallel to line l?
A) a = b
B) c = e
C) b = e
D) d = e
(Medium) The only “ZCUF” angle pair is choice B, since the angles measuring c° and e° form a Z shape. If they are congruent, then lines k and l must be parallel. Therefore, the correct answer is B.
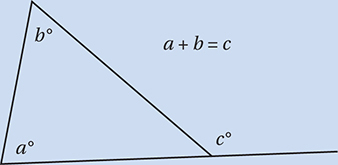
Lesson 2: Triangles
Angles in a Triangle Have a Sum of 180°
Side-Angle Theorem
Isosceles Triangle Theorem
Exterior Angle Theorem
The Triangle Inequality
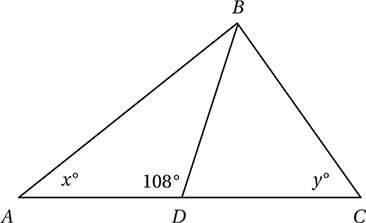
In the figure above, point D is on side AC of triangle ABC. If AD = DB = DC, what is the value of x + y ?
A) 72
B) 90
C) 96
D) 108
(Medium) Since angle ADB and angle BDC are supplementary and AD = DB = DC, we can use the Isosceles Triangle Theorem to mark up the diagram:

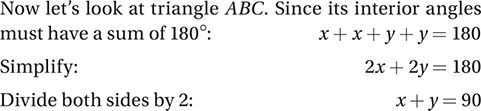
So the correct answer is B. Note that this fact is independent of the measures of the other two (108° and 72°) angles. As long as AD = DB = DC, this relationship will hold. We can see these angle relationships if we note that these three segments could all be radii of a circle centered at D.
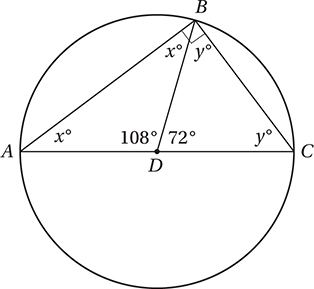
You might remember from geometry class that any inscribed angle (an angle inside a circle with a vertex on the circle) intercepts an arc on the circle that is twice its measure. Since angle ABC is an inscribed angle that intercepts a 180° arc, it must have a measure of 90° and therefore, x + y = 90.

The figure above shows three intersecting lines. What is the value of c in terms of a and b ?
A) 180 – a – b
B) 180 – a + b
C) 90 + b – a
D) a + b
(Easy) Notice that two of the angles are vertical to two interior angles of the triangle, and the other is an exterior angle.
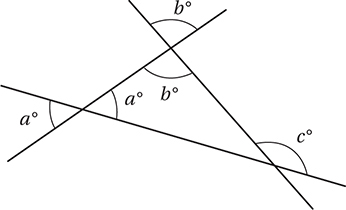
Since the c° angle is an exterior angle to the triangle, the Exterior Angle Theorem tells us that c = a + b, so the correct answer is D.
Alternatively, we could just choose reasonable values for a and b, like a = 50 and b = 90, and then analyze the diagram in terms of these values. This would imply that the interior angles of the triangle are 50°, 90°, and 40°, and c° would then be the measure of the supplement of 40°, which is 140°. If we then plug these values for a and b into all of the choices, the only one that yields 140 is D.
In the xy-plane, the distance from point A to point B is 24 centimeters and the distance from point A to point C is 18 centimeters. Which of the following could not be the distance from point B to point C, in centimeters?
A) 5
B) 6
C) 24
D) 42
(Medium) The Triangle Inequality says that the length of any side of a triangle must be between the sum and the difference of the other two side lengths. However, these three points might also be collinear, which would mean that one of the segment lengths is equal to either the sum or the difference of the other two. Therefore, the distance from B to C must be greater than or equal to 24 – 18 = 6 and less than or equal to 24 + 18 = 42. The only choice that is outside this range is choice A.
Lesson 3: Working in the xy-Plane
Working in the xy-Plane
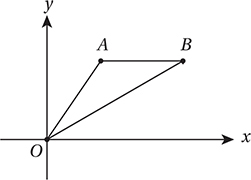
Note: Figure not drawn to scale.
In the xy-plane above, points A and B lie on the graph of the line y = 6.
If 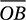 has a slope of
has a slope of  and AB = 5, what is the slope of
and AB = 5, what is the slope of 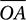 ?
?

(Medium-hard) First, let’s use one of the tips and drop two perpendicular segments from A and B to points C and D, respectively, on the x-axis.
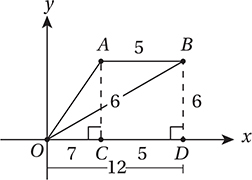
Since A and B lie on y = 6, they are both 6 units from the x-axis, and so AC = BD = 6. Since the slope of OB is 1/2, BD/OD = 1/2, and therefore OD = 12. Since AB = 5, CD = 5 also, and therefore OC = 12 – 5 = 7. (Don’t worry that  looks shorter than
looks shorter than  in the diagram. Remember, the figure is not drawn to scale!) This gives us everything we need to find the slope of
in the diagram. Remember, the figure is not drawn to scale!) This gives us everything we need to find the slope of  which connects (0, 0) to (7, 6). By the slope formula from Chapter 6, Lesson 5:
which connects (0, 0) to (7, 6). By the slope formula from Chapter 6, Lesson 5:

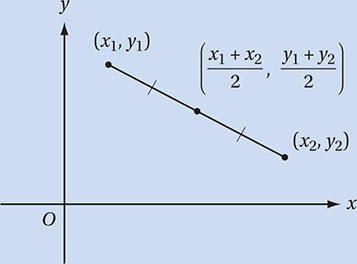
In the figure above, point M (not shown) is the midpoint of 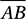 and point N (not shown) is the midpoint of
and point N (not shown) is the midpoint of 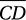 . What is the slope of
. What is the slope of 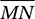 ?
?

(Medium) To find the midpoint of a segment, we just take the average of the endpoints. Point M, the mid-point of 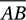 , has coordinates
, has coordinates 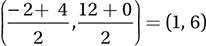 , and point N, the midpoint of
, and point N, the midpoint of 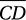 , has coordinates
, has coordinates 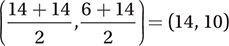 .
.
Therefore, the slope of 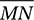 is
is 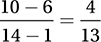 or 0.307 or 0.308.
or 0.307 or 0.308.
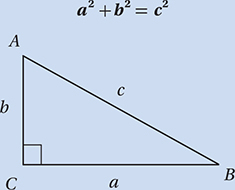
Lesson 4: The Pythagorean Theorem
The Pythagorean Theorem
Special Right Triangles
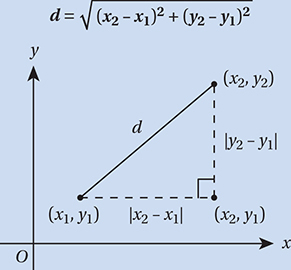
The Distance Formula
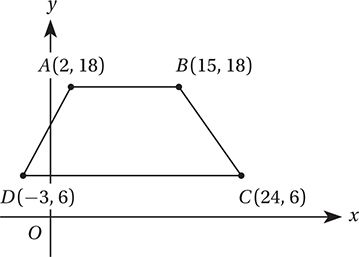
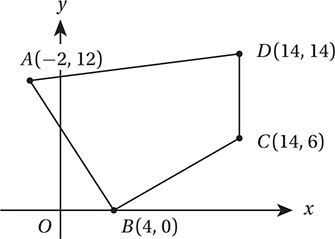
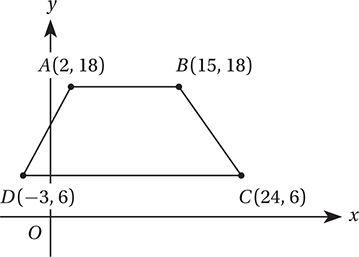
What is the perimeter of quadrilateral ABCD in the figure above?

(Medium) The perimeter of a figure is the distance around its edges. We can find AB and DC because the length of a horizontal segment is just the absolute difference of the x-coordinates of its endpoints. Therefore, AB = 15 – 2 = 13 and DC = 24 – (–3) = 27. To find AD and BC, we can drop two vertical lines from points A and B to the bottom edge. This shows that AD and BC are hypotenuses of two right triangles:
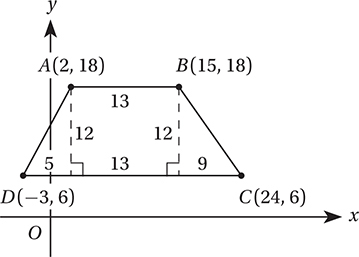
So, we can find AD and BC with the Pythagorean Theorem.

Note that triangle on the left is a 5-12-13 right triangle, and the triangle on the right is a 3-4-5 right triangle.

So, the perimeter of ABCD is 13 + 15 + 27 + 13 = 68.
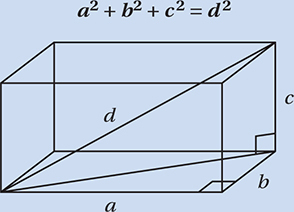
The 3-D Distance Formula
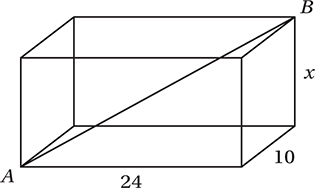
The figure above shows a rectangular box with a length of 24, a width of 10, and a height of x. If 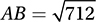 , what is the volume of the box, in cubic units?
, what is the volume of the box, in cubic units?

(Hard) The most straightforward way to approach the problem is to use a form of the 3-D distance formula to find x, and then use the volume formula for a box.
But rather than just applying a formula blindly, let’s walk through the reasoning.
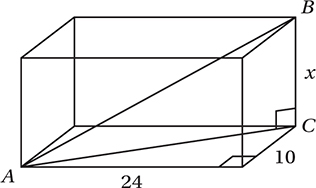
Let’s draw 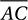 connecting the opposite vertices of the base. This is the hypotenuse of a right triangle with legs of 10 and 24. If you’re on the ball, you’ll note that these fit the pattern of a 5-12-13 triangle with all the sides doubled, and so AC = 26. If not, no worries: just use the Pythagorean Theorem:
connecting the opposite vertices of the base. This is the hypotenuse of a right triangle with legs of 10 and 24. If you’re on the ball, you’ll note that these fit the pattern of a 5-12-13 triangle with all the sides doubled, and so AC = 26. If not, no worries: just use the Pythagorean Theorem:
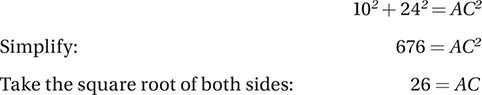
Now we turn our attention to triangle ABC, which is also a right triangle. Since we know the length of one leg and the hypotenuse, we can use the Pythagorean Theorem again:

Therefore, the volume of the box is (24)(10)(6) = 1440.
Exercise Set 1: Geometry (No Calculator)

In the figure above, MNOP is a square and Q is the midpoint of 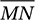 . If
. If 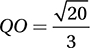 , what is the area of square MNOP ?
, what is the area of square MNOP ?



Lines l and m are parallel in the figure above. What is the value of x ?
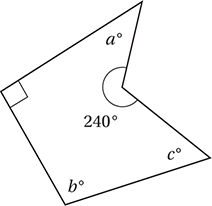

In the figure above, what is the value of a + b + c ?
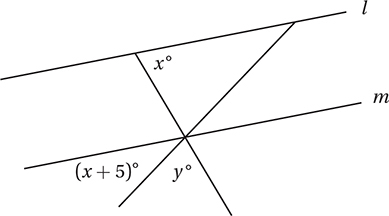
Lines l and m are parallel in the figure above. Which of the following expresses the value of y in terms of x ?
A) 95 − 2x
B) 165 − 2x
C) 175 − 2x
D) 185 − 2x
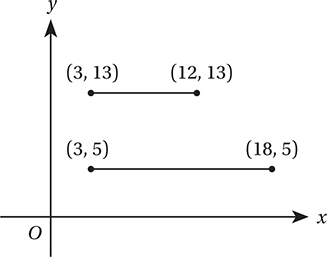
In the figure above, what is the distance between the midpoints (not shown) of the two line segments?
A) 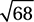
B) 
C) 
D) 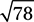
What is the perimeter of an equilateral triangle inscribed in a circle with circumference 24π ?
A) 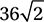
B) 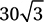
C) 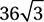
D) 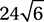
Exercise Set 1: Geometry (Calculator)
Questions 7–9 are based on the figure below.
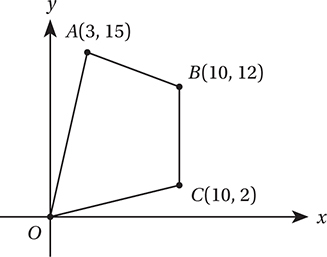
Note: Figure not drawn to scale.
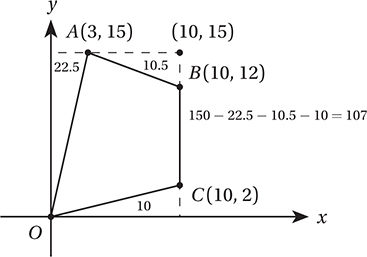
In the figure above, what is the perimeter of quadrilateral ABCO, to the nearest integer?

In the figure above, what is the area, in square units, of ABCO ?

In the figure above, point K (not shown) is the mid-
point of 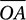 , and point M (not shown) is the midpoint of
, and point M (not shown) is the midpoint of 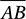 . What is the slope of
. What is the slope of 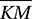 ?
?

In the xy-plane, point H has coordinates (2, 1) and point J has coordinates (11, 13). If 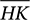 is parallel to the x-axis and
is parallel to the x-axis and  is parallel to the y-axis, what is the perimeter of triangle HJK ?
is parallel to the y-axis, what is the perimeter of triangle HJK ?

Note: Figure not drawn to scale.
In the figure above, what is the value of x ?
A) 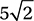
B) 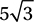
C) 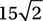
D) 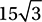

Questions 12–15 are based on the information below.
In the xy-plane, ABCD is a square. Point A has coordinates (–1, 2) and point B has coordinates (3, 5).
Which of the following could be the coordinates of C ?
A) (0, 9)
B) (6, 0)
C) (2, –2)
D) (–4, 6)
What is the area of square ABCD ?
A) 25
B) 28
C) 30
D) 32
What is the slope of 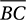 ?
?
A) 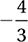
B) 
C) 
D) 
What is the distance between C and the midpoint of 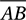 ?
?
A) 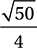
B) 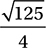
C) 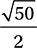
D) 

EXERCISE SET 1: GEOMETRY ANSWER KEY
No Calculator
1. 16/9 or 1.77 or 1.78 If we define x as the length of 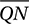 , then the length of one side of the square is 2x, and so the area of square MNOP is (2x)(2x) = 4x2. To find this value, we can apply the Pythagorean Theorem to right triangle QNO:
, then the length of one side of the square is 2x, and so the area of square MNOP is (2x)(2x) = 4x2. To find this value, we can apply the Pythagorean Theorem to right triangle QNO:

2. 133 The key is to notice simple relationships between angles until we get around to x.
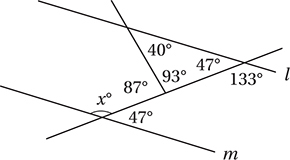
3. 210 Draw three lines as shown:

Since the polygon divides into 3 triangles, the sum of its internal angles is (3)(180°) = 540°. Therefore a + b + c + 240 + 90 = 540, and so a + b + c = 210.
4. C Using the Crossed Lines Theorem and the Parallel Lines Theorem, we can mark up the diagram like this:
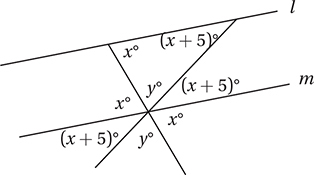
This shows that x + y + x + 5 = 180, and so y = 175 – 2x.
5. B The midpoint of the top segment is 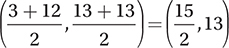 , and the midpoint of the bottom segment is
, and the midpoint of the bottom segment is 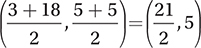 , therefore, the distance between them is
, therefore, the distance between them is 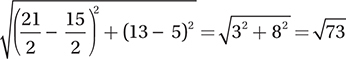 .
.
6. C To solve this problem we must draw a diagram and find the relationship between the radius of the circle and the sides of the triangle. By the Isosceles Triangle Theorem, if all three sides of a triangle are congruent, then all three angles must be congruent. Since these angles also must have a sum of 180°, they must each be 60°. If we draw the bisectors of each of these angles, we divide the triangle into six smaller triangles. These smaller triangles are congruent 30°-60°-90° triangles, as shown here:
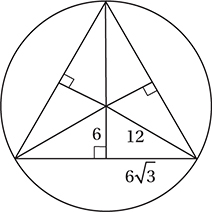
Since the circumference of the circle (2πr) is 24π, its radius is 12. Since each of the hypotenuses of our right triangles is also a radius of the circle, we can find all of the sides of these triangles using the 30°-60°-90° relationships. Each side of the equilateral triangle is therefore 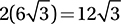 , and its perimeter is therefore
, and its perimeter is therefore  .
.
Calculator
7. 43 Using the distance formula, we can calculate the lengths of each segment. 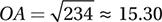
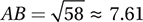 BC = 10, and
BC = 10, and 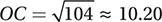 . Therefore, the perimeter is approximately 15.30 + 7.61 + 10 + 10.20 = 43.11, which rounds to 43.
. Therefore, the perimeter is approximately 15.30 + 7.61 + 10 + 10.20 = 43.11, which rounds to 43.
8. 107 Since we do not have a formula that directly calculates the area of such an odd-shaped quadrilateral, we must analyze its area in terms of simpler shapes. The simplest way to do this is by drawing a box around it. This turns the area of interest into a rectangle minus three right triangles, all of which have areas that can be easily calculated.
9. 6/5 or 1.2 The midpoint of 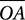 is (1.5, 7.5) and the midpoint of
is (1.5, 7.5) and the midpoint of 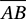 is (6.5, 13.5); therefore, the slope of the segment between them is 6/5.
is (6.5, 13.5); therefore, the slope of the segment between them is 6/5.
10. 36 If point K is on the same horizontal line as (2, 1), it must have a y-coordinate of 1, and if it is on the same vertical line as (11, 13), it must have an x-coordinate of 11. Therefore, K is the point (11, 1), and so HK = 9, JK = 12, and 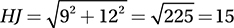 . Notice that it is a 3-4-5 triangle!
. Notice that it is a 3-4-5 triangle!
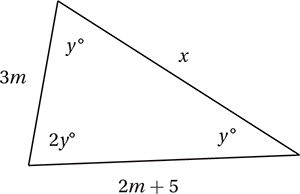
11. C Since the sum of the interior angles of any triangle is 180°, y + y + 2y = 4y = 180, and therefore y = 45. Therefore, this is a 45°-45°-90° right triangle. Since two angles are equal, the two opposite sides must also be equal, so 3m = 2m + 5 and so m = 5 and the two legs each have measure 15. Using the Pythagorean Theorem or the 45°-45°-90° shortcut, we can see that  .
.
12. A The key to questions 12 through 15 is a good diagram in the xy-plane that represents the given information:
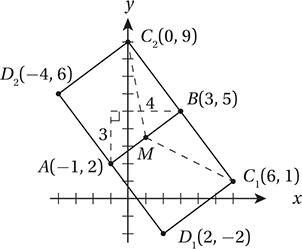
If ABCD is a square, then the points A, B, C, and D must appear in that order around the square. Notice that to get from point A to point B, we must move 4 units to the right and 3 units up. This means that, in order to get to point C along a perpendicular of the same length, we must go either 3 units right and 4 units down, or 3 units left and 4 units up. This puts us either at (6, 1) or (0, 9).
13. A The diagram shows that AB is the length of the hypotenuse of a right triangle with legs 3 and 4. You should recognize this as the special 3-4-5 right triangle. If AB = 5, then the area of the square is 52 = 25.
14. A Notice that the slope of 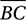 is the same regardless of which option we choose for C. In either case, the slope formula tells us that the slope is –4/3.
is the same regardless of which option we choose for C. In either case, the slope formula tells us that the slope is –4/3.
15. D The midpoint of 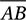 (point M above) is (1, 3.5). We can use the distance formula to find the distance between this point and either of the possible locations of C. (Notice that the distance is the same either way.) Alternately, we might notice that MC is the hypotenuse of a right triangle with legs 5 and 2.5. Either way, we get a value of
(point M above) is (1, 3.5). We can use the distance formula to find the distance between this point and either of the possible locations of C. (Notice that the distance is the same either way.) Alternately, we might notice that MC is the hypotenuse of a right triangle with legs 5 and 2.5. Either way, we get a value of 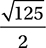 .
.
Lesson 5: Circles
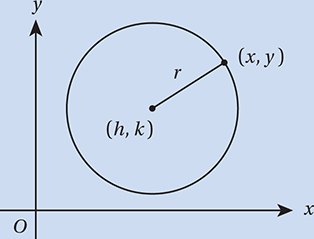
Equations of Circles
Which of the following equations represents a circle in the xy-plane that passes through the point (1, 5) and has a center of (3, 2)?
A) (x – 3)2 + (y – 2)2 = 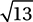
B) (x – 3)2 + (y – 2)2 = 13
C) (x – 1)2 + (y – 5)2 = 13
D) (x – 3)2 + (y – 2)2 = 25
(Easy) Since our circle has a center at (3, 2), its equation must have the form (x – 3)2 + (y – 2)2 = r2, which eliminates choice C. Now we can just plug in the point (1, 5) and solve:

Therefore, the equation is (x – 3)2 + (y – 2)2 = 13, and the correct answer is B.
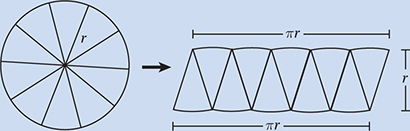
Circumference and Area of Circles
What is the area, in square centimeters, of a circle with a circumference of 16π centimeters?
A) 8π
B) 16π
C) 32π
D) 64π
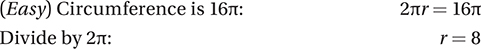
Therefore, the area is πr2 = π(8)2 = 64π, so the correct answer is D.
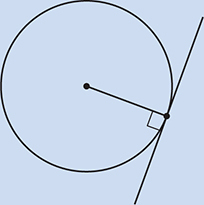
Tangents to Circles
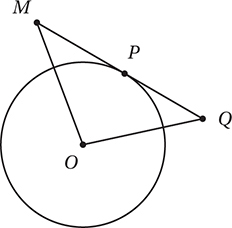
In the figure above, 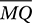 is tangent to the circle at point P, MO =
is tangent to the circle at point P, MO =  , and OQ =
, and OQ = 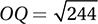 . If the circle has an area of 100π, what is the area of triangle MOQ?
. If the circle has an area of 100π, what is the area of triangle MOQ?

(Hard) Draw radius 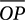 . Since this is a radius to the point of tangency, it must be perpendicular to
. Since this is a radius to the point of tangency, it must be perpendicular to 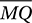 . Let’s also indicate the given measures.
. Let’s also indicate the given measures.

Notice that  is the height of triangle MOQ if
is the height of triangle MOQ if  is taken as its base. To find MQ, we can use the Pythagorean Theorem to find MP and PQ and just add them together.
is taken as its base. To find MQ, we can use the Pythagorean Theorem to find MP and PQ and just add them together.
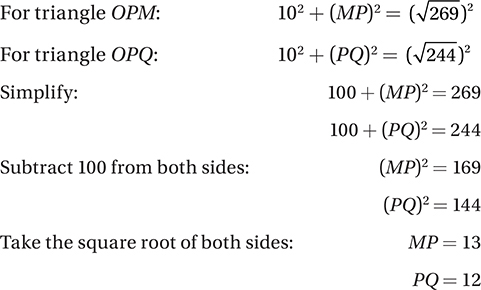
So MQ = MP + PQ = 13 + 12 = 25, and the area of triangle 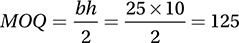 .
.
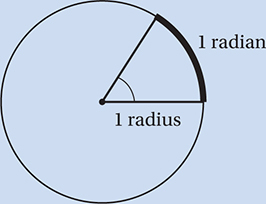
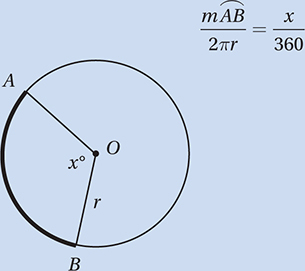
Lesson 6: Radians, Chords, Arcs, and Sectors
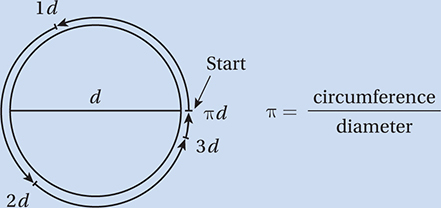
Radians
What is the degree measure of an angle that measures 4.5 radians?
A) 4.5π°
B) 
C) 
D) 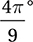
(Medium) Here, we just need to convert radians to degrees by using the conversion factor: 4.5 radians × 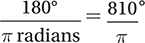 , so the correct answer is C.
, so the correct answer is C.
Chords
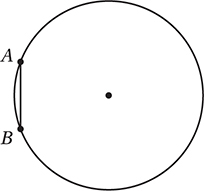
The circle above has an area of 100π square centimeters. If AB = 8, how far is 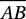 from the center of the circle?
from the center of the circle?
A) 6
B) 8
C) 
D) 
(Medium) First, let’s draw three extra line segments:
Since the area = πr2 = 100π, r = 10. The perpendicular from the center to the chord is the distance from the center to the chord. This segment also bisects the chord, dividing it into two equal segments of 4 centimeters each. This allows us to use the Pythagorean

Take the square root of both sides:

Therefore, the correct answer is D.
Arcs
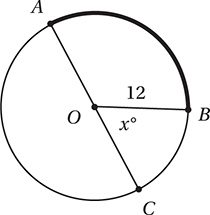
Note: Figure not drawn to scale.
In the figure above, AC is a diameter of the circle with center O, OB = 12, and the length of arc AB is 7π. What is the value of x ?
A) 60
B) 72
C) 75
D) 78
(Medium) Since the circle has a radius of 12, its circumference is 2π(12) = 24π, and so arc AC = 12π. If arc AB = 7π, then the length of arc BC is 12π – 7π = 5π. Since the central angle of x° is the same fraction of 360° as its arc BC is to the entire circumference,

Therefore, the correct answer is C.
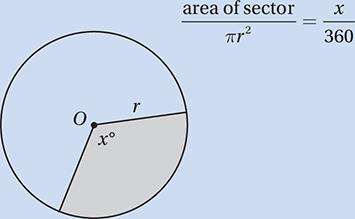
Sectors
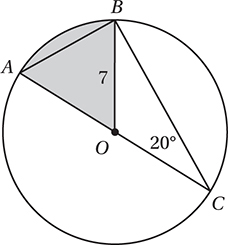
Note: Figure not drawn to scale.
In the figure above, AC is a diameter of the circle with center O and OB = 7. If the measure of ACB is 20°, what is the area of the shaded sector?
A) 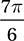
B) 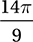
C) 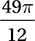
D) 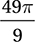
(Medium-hard) Since 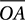 ,
,  , and
, and  are all radii, triangles AOB and BOC are isosceles. Therefore, we can find all the angles with the Isosceles Triangle Theorem and the Angle Sum Theorem:
are all radii, triangles AOB and BOC are isosceles. Therefore, we can find all the angles with the Isosceles Triangle Theorem and the Angle Sum Theorem:
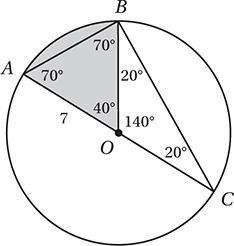
Since the central angle of the sector is 40°, the area of the sector is 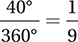 the area of the circle. Since the area of the circle is π(7)2 = 49π, the area of the sector is
the area of the circle. Since the area of the circle is π(7)2 = 49π, the area of the sector is 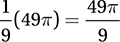 square units. Therefore, the correct answer is D.
square units. Therefore, the correct answer is D.
Lesson 7: Areas and Volumes
Reference Information
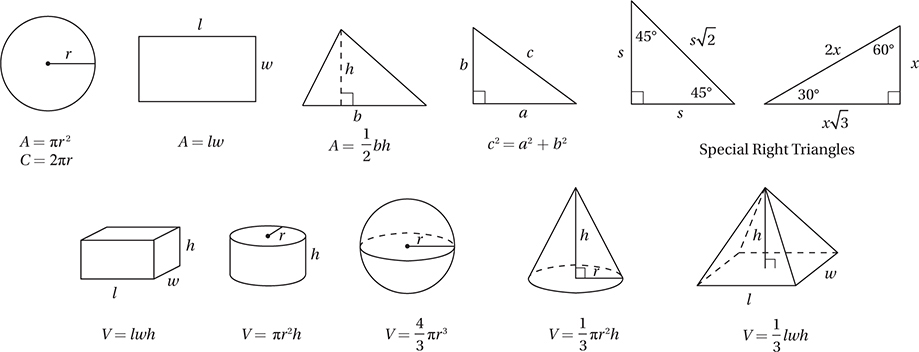
The number of degrees of arc in a circle is 360.
The number of radians of arc in a circle is 2π.
The sum of the measures in degrees of the angles of a triangle is 180.
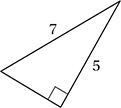
What is the area, in square units, of the right triangle above?
A) 
B) 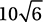
C) 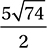
D) 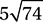
(Medium) By the Pythagorean Theorem, the missing side of the triangle is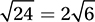 , so the area of the triangle is
, so the area of the triangle is 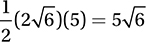 , and the correct answer is A.
, and the correct answer is A.

The figure above shows a wooden cylindrical tube with a length of 10 centimeters and a diameter of 4 centimeters. It has a cylindrical hole with a diameter of 2 centimeters that extends 40% of the length of the tube. If the density of the wood is 4.2 grams per cubic centimeter, what is the mass of this tube, to the nearest gram?
A) 151 grams
B) 343 grams
C) 468 grams
D) 475 grams
(Medium) The large cylinder has a radius of 2 and a length of 10, so its volume is π(2)2(10) = 40π. The cylindrical hole has a radius of 1 and a length of (.040)(10) = 4, so the volume of the hole is π(1)2(4) = 4π. Therefore, the total volume of the closed tube is 40π – 4π = 36π ≈ 113.1. Since the mass is equal to the volume times the density, its mass is (113.1)(4.2) = 475.02 grams, so the correct answer is D.
The Strange Area Rule
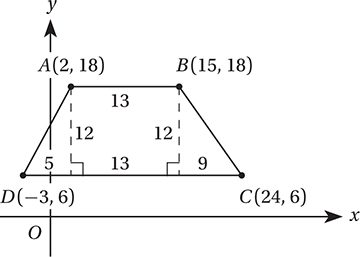
What is the area, in square units, of quadrilateral ABCD above?

(Medium) The Reference Information on the SAT doesn’t give us any formula for calculating the area of a trapezoid (and don’t even worry if you forgot the technical name for the shape), but that’s okay. All we need to know is that we can look at this area in two different ways: as a rectangle plus two right triangles:
or as a bigger rectangle minus two right triangles:
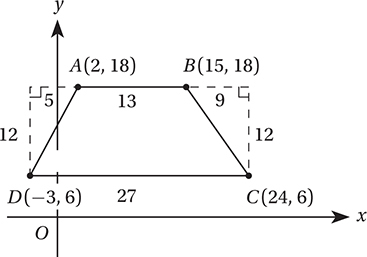
We should get the same result with either method. With the first method, the area of the trapezoid is the area of the rectangle plus the areas of two right triangles. This gives us a total area of (12)(13) + (1/2)(5)(12) + (1/2)(9)(12) = 156 + 30 + 54 = 240. With the second method, the area of the trapezoid is the area of the large rectangle minus the areas of the two right triangles. This gives us a total area of (12)(27) – (1/2)(5)(12) – (1/2)(9)(12) = 324 – 30 – 54 = 240.
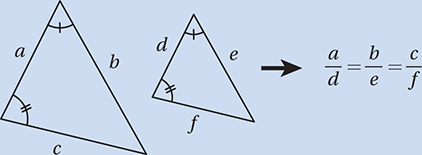
Lesson 8: Similarity
Similarity
The AA (Angle-Angle) Theorem
Perimeters, Areas, and Volumes of Similar Figures
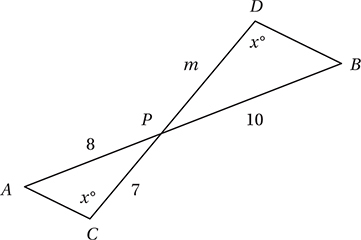
In the figure above, 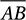 and
and  are line segments that intersect at point P. What is the value of m ?
are line segments that intersect at point P. What is the value of m ?

(Medium) Since the vertical angles ∠APC and ∠BPD are congruent, the two triangles are similar by the AA Theorem, so the corresponding sides are proportional:

In the figure above, line ℓ is parallel to line m and segment BF is perpendicular to line m. What is the length of segment AG ?

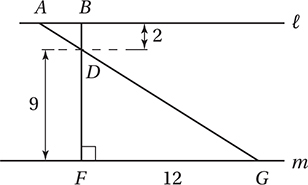
(Medium-hard) To find AG, we can find AD and DG and add them together. To find DG, we can use the Pythagorean Theorem or just note that triangle DFG is in the 3-4-5 triangle family, and so DG = 15. The two triangles are similar by the AA Theorem, because vertical angles are congruent and angles ABD and DFG are both right angles. Since BD and DF are corresponding sides, the ratio of sides is  and therefore
and therefore 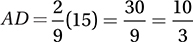 . Adding DG and AD gives
. Adding DG and AD gives 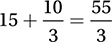 or 18.3.
or 18.3.

The figure above shows a fluted glass filled with water to a height of 8 centimeters. The interior of the glass is a right cone. If the water in the glass has a volume of 64 milliliters, how many more milliliters of water must be added so that the water reaches a height of 12 centimeters? (1 milliliter = 1 cubic centimeter)

(Hard) Many students will make this problem harder by using the cone volume formula (V =  πr2h). This is messy and unnecessary. Instead, note that the original volume of water and the final volume of water are similar cones. Since the heights of these cones are in a ratio of 12:8, or 3:2, each length in the larger cone is 1.5 times the corresponding length in the smaller cone. By the “ratio of volumes” theorem on the previous page, this means that the volume of the larger cone is (1.5)3 = 3.375 times the volume of the smaller one. Therefore, the final volume is 3.375(64) = 216 milliliters, which means we must add 216 – 64 = 152 more milliliters of water.
πr2h). This is messy and unnecessary. Instead, note that the original volume of water and the final volume of water are similar cones. Since the heights of these cones are in a ratio of 12:8, or 3:2, each length in the larger cone is 1.5 times the corresponding length in the smaller cone. By the “ratio of volumes” theorem on the previous page, this means that the volume of the larger cone is (1.5)3 = 3.375 times the volume of the smaller one. Therefore, the final volume is 3.375(64) = 216 milliliters, which means we must add 216 – 64 = 152 more milliliters of water.

The figure above shows a scale drawing of a rectangular garden surrounded by a walkway that is 6 feet wide. If the ratio of a to b is 5:8, and the ratio of c to d is 3:4, what is the area of the garden (not including the walkway), in square feet?

(Hard) Since the ratio of the width of the garden to its length is 5 to 8, let’s call the width of the garden 5x and the length of the garden 8x, where all lengths are in feet. Now, since the walkway is 6 feet wide all around, c = 5x + 6 + 6 = 5x + 12 feet and d = 8x + 6 + 6 = 8x + 12 feet. Since we are given the ratio of these two lengths, we can set up an equation and solve.

Since the length and width of the garden are 5x and 8x, the dimensions are 5(3) = 15 feet and 8(3) = 24 feet, and so the area of the garden is (15)(24) = 360 square feet.
Exercise Set 2: Geometry (No Calculator)
A cereal company sells oatmeal in two sizes of cylindrical containers. The radius of the larger container is twice that of the smaller, and the height of the larger container is 50% greater than the smaller. If the smaller container holds 10 ounces of oatmeal, how many ounces can the larger container hold?

Note: Figure not drawn to scale.
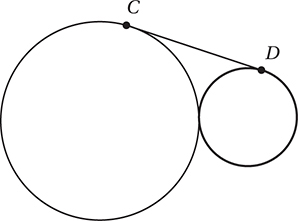
In the figure above,  is tangent to both circles, which are tangent to each other. If the smaller circle has a circumference of 4π and the larger circle has a circumference of 16π, what is the length of
is tangent to both circles, which are tangent to each other. If the smaller circle has a circumference of 4π and the larger circle has a circumference of 16π, what is the length of  ?
?

What is the area, in square inches, of a circle with diameter 6π2 inches?
A) 9π4
B) 9π5
C) 36π4
D) 36π5
What is the length of the longest line segment that connects two vertices of a rectangular box that is 6 units wide, 4 units long, and 2 units tall?
A) 
B) 
C) 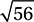
D) 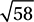
Which of the following equations represents a circle in the xy-plane that intersects the x-axis at (3, 0) and (9, 0) ?
A) (x – 6)2 + (y – 4)2 = 25
B) (x – 3)2 + (y – 9)2 = 25
C) (x – 6)2 + (y – 4)2 = 36
D) (x – 3)2 + (y – 9)2 = 36
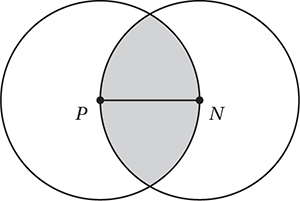
In the figure above, P and N are the centers of the circles and PN = 6. What is the area of the shaded region?
A) 
B) 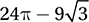
B) 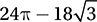
B) 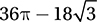

The diagram above shows a hexagon with all sides congruent and all angles congruent. What is the value of k ?
A) 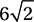
B) 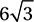
C) 
D) 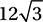
Exercise Set 2: Geometry (Calculator)

What is the area, in square units, of the quadrilateral above?

What is the degree measure, to the nearest whole degree, of an angle that measures 5.6 radians?

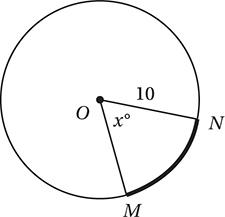
In the figure above, arc 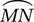 has a length of 11.5. To the nearest integer, what is the value of x ?
has a length of 11.5. To the nearest integer, what is the value of x ?

The Great Pyramid in Giza, Egypt, has a height of 140 meters and a volume of 2.6 million cubic meters. If a scale model of the Great Pyramid is to be built that is 2 meters high, what will be the volume, in cubic meters, of this model?

Which of the following equations defines a circle that is tangent to the y-axis?
A) (x – 2)2 + (y + 3)2 = 2
B) (x – 2)2 + (y + 3)2 = 3
C) (x – 2)2 + (y + 3)2 = 4
D) (x – 2)2 + (y + 3)2 = 9

Questions 13 and 14 refer to the diagram below.

The figure above shows two rectangles that share a common vertex, and 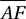 is a line segment that passes through C.
is a line segment that passes through C.
What is the ratio of the area of rectangle ABCD to the area of rectangle AEFG ?
A) 3:5
B) 9:25
C) 5:8
D) 25:64
If CD = 9, what is the perimeter of rectangle AEFG ?
A) 67.2
B) 72.6
C) 76.2
D) 78.6

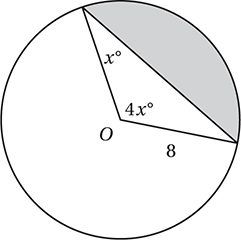
Point O is the center of the circle above. What is the area of the shaded region?
A) 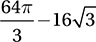
B) 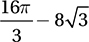
C) 
D) 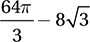
EXERCISE SET 2: GEOMETRY ANSWER KEY
No Calculator
1. 60 If the smaller cylinder has a radius of r and a height of h, its volume is πr2h. The larger cylinder therefore must have a radius of 2r and a height of 1.5h, and a volume of π(2r)2(1.5h) = 6πr2h. Since this is 6 times the volume of the smaller cylinder, it must hold 10 × 6 = 60 ounces of oatmeal.
2. 8 First, let’s draw the radii to the points of tangency, the segment joining the centers, and the segment from the center of the smaller circle that is perpendicular to the radius of the larger circle. Since the tangent segment is perpendicular to the radii, these segments form a rectangle and a right triangle.
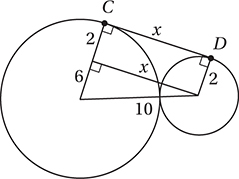
Since the circumference of the smaller circle is 4π, its radius is 2, and since the circumference of the larger circle is 16π, its radius is 8. The hypotenuse of the right triangle is the sum of the two radii: 2 + 8 = 10. One of the legs of the right triangle is the difference of the two radii: 8 – 2 = 6.
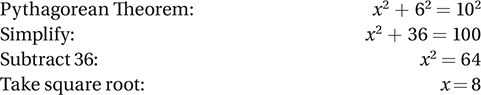
3. B 
4. C From the 3-D Distance Formula back in Lesson 4, the length of the diagonal is 
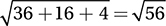 .
.
5. A All of the equations are clearly equations of circles, so our only task is to verify that one of these equations is satisfied by both point (3, 0) and point (9, 0). Simply by plugging these coordinates into the equations, we can verify that only the equation in (A) is true for both points: (3 – 6)2 + (0 – 4)2 = 25 and (9 – 6)2 + (0 – 4)2 = 25.
6. C In this problem, we have to take advantage of the Strange Area Rule from Lesson 7. First we should draw the segments from P and N to the points of intersection. Since each of these segments is a radius, they have equal measure (6), and form two equilateral 60°-60°-60° triangles.
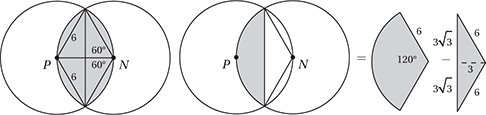
The shaded region is composed of two circle “segments,” each of which is a sector minus a triangle, as shown in the figure above. The sector, since it has a 120° central angle, has an area 1/3 of the whole circle, or (1/3) (π(6)2) = 12π and the triangle has area 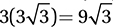 . Therefore, the shaded region has an area of
. Therefore, the shaded region has an area of 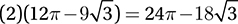 .
.
7. B Each side of the hexagon has length 9 – 3 = 6. Each interior angle of a regular hexagon has measure (6 – 2)(180°)/6 = 120°, so the segments shown form two 30°-60°-90° triangles with lengths shown below.
Therefore, 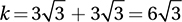 .
.
Calculator
8. 142 First, let’s draw a rectangle around the figure as shown.

This shows that the area we want is the area of the rectangle minus the areas of the three triangles: (16)(14) – (1/2)(2)(16) – (1/2)(12)(6) – (1/2)(10)(6) = 224 – 16 – 36 – 30 = 142.
9. 321 To convert any angle from radians to degrees, we just multiply by the conversion factor (180°)/(π radians). 56 × 180°/π = 320.86 ≈ 321°.
10. 66 In a circle with radius 10, and arc of length 11.5 has a radian measure of 11.5/10 = 1.15 radians. In degrees, this equals 1.15 × 180°/π = 65.89° ≈ 66°.
11. 7.58 If two similar solids have sides in ratio of a:b, then their volumes are in a ratio of a3:b3. The ratio of the heights is 140:2 = 70:1, so the ratio of volumes is 703:13 = 343,000:1. This means that the volume of the model is 2,600,000 ÷ 343,000 ≈ 7.58 cubic meters.
12. C As a quick sketch will verify, in order for a circle to be tangent to the y-axis, its radius must equal the absolute value of the x-coordinate of its center. Since the center of each square is (2, –3), the radius must be 2. The only circle with a radius of 2 is C.
13. D By the AA Theorem, triangle ACD is similar to triangle AFG, and so rectangle ABCD is similar to rectangle AEFG. The ratio of the corresponding sides is equal to the ratio of their diagonals, which is 15:24 = 5:8. Therefore, the ratio of their areas is 52:82 = 25:64.
14. A If CD = 9, we can find AD by the Pythagorean Theorem. (AD)2 + (CD)2 = (AC)2

15. A The two radii and the chord form an isosceles triangle.x + x + 4x = 180

Therefore, the diagram should look like this:
As we saw in question 6, this portion of the circle is called a “segment,” and we find its area by taking the area of the sector minus the area of the triangle. The sector has area (120/360)(π82) = 64π/3, and the triangle has area 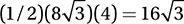 , so the segment has an area of
, so the segment has an area of 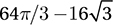 .
.
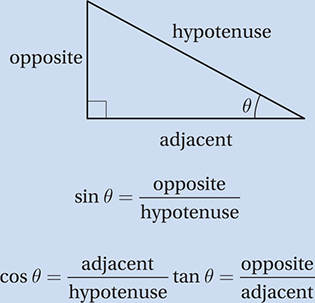
Lesson 9: Basic Trigonometry
The Trigonometric Functions
The Unit Circle
Which of the following is equivalent to  (No calculator)
(No calculator)
A) 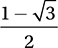
B) 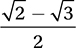
C) 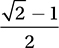
D) 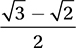
(Medium-hard) You may find it useful to convert the angles to degree measures using the conversion factor, 180°/π, from Lesson 6. This gives us π/4 radians = 45° and π/6 radians = 30°. Note that these are angles in the special right triangles from the Reference Information at the beginning of the test.
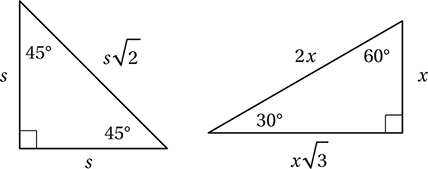
Using the definitions for sine and cosine, these triangles shows us that 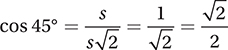 and
and 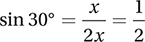 . Therefore,
. Therefore,  =
= 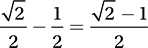 ; so the correct answer is C.
; so the correct answer is C.
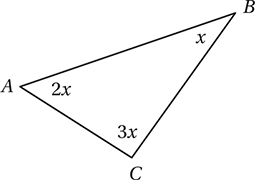
Note: Figure not drawn to scale.
In the triangle above, x represents the measure, in radians, of the smallest angle. What is the tangent of 2x ?
A) 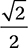
B) 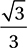
C) 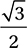
D) 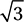
(Medium-hard) Although the question tells us that x represents a radian measure, the important information is that the measures of the three angles are in a ratio of 1:2:3, which is true regardless of what angle units we use. For convenience, let’s consider the measures in degrees. Since the angle measures in a triangle must have a sum of 180° (or π if you prefer to work in radians), x + 2x + 3x = 180, so 6x = 180 and x = 30. Therefore, the three angles are 30°, 60°, and 90°, and so this is one of our special right triangles from the Reference Information:
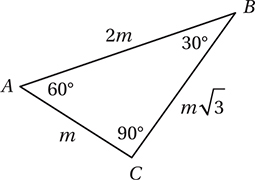
From the definition of the tangent function (TOA), 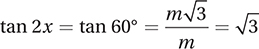 , and so the correct answer is D.
, and so the correct answer is D.
Lesson 10: The Pythagorean Identity
The Pythagorean Identity
If 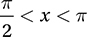 and sin x = 0.8, what is the value of cos x ?
and sin x = 0.8, what is the value of cos x ?
A) –0.80
B) –0.60
C) –0.25
D) 0.60

Since x is an angle in the second quadrant, the cosine is negative, so the correct answer is B.

If b is an angle measure that satisfies the equation above, what is the value of (sin b - cos b)2?
A) 
B) 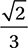
C) 
D) 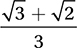
(Hard) Let’s simplify by applying the Pythagorean Identity.

Now we need to find the value of sin b cos b, which we can find with the given equation.

Substitute sin b cos b =  into original expression:
into original expression:
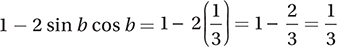
So the correct answer is A.
Lesson 11: The Cofunction Identities
Trigonometry of Complementary Angles
If sin x = a and x + y = 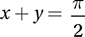 , what is the value of sin y ?
, what is the value of sin y ?
A) a
B) 1 – a2
C) 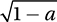
D) 
(Medium-hard)

Complementary angle theorem:
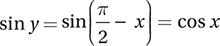
Pythagorean Identity:

Therefore, the correct answer is D.
If sin y = 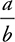 and 0 < y <
and 0 < y <  , which of the following is equal to sin
, which of the following is equal to sin 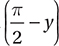 ?
?
A) 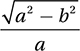
B) 
C) 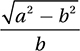
D) 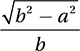
(Hard) Since y is the measure of an acute angle, it can be the interior angle of a right triangle. Since its sine is a/b, we can say that the opposite side has measure a and the hypotenuse has measure b.
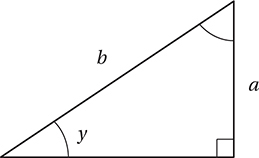
Now use the Pythagorean Theorem to find the other leg:

Also, we know that the other acute angle has a measure of 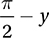 , so let’s complete the picture:
, so let’s complete the picture:
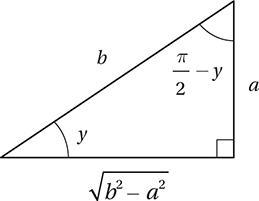
By the definition of sine: 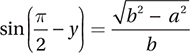
So the correct answer is D.
Exercise Set 3: Trigonometry (No Calculator)
What is the greatest possible value of f if 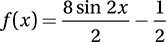 ?
?

If 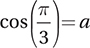 , what is the value of
, what is the value of  ?
?

If (sin x – cos x)2 = 0.83, what is the value of (sin x + cos x)2 ?

Which of the following is equivalent to 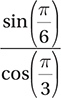 ?
?
A) 
B) 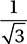
C) 
D) 1
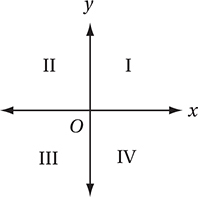
If θ is an angle in standard position on the xy-plane above, and if sin θ < 0 and sin θ cos θ < 0, then θ must be in which quadrant?
A) I
B) II
C) III
D) IV
If 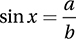 and
and  , which of the following expressions is equal to
, which of the following expressions is equal to 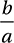 ?
?
A) 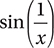
B) 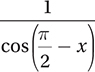
C) 1 – sin2 x
D) 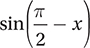
If sin b = a, which of the following could be the value of cos (b + π) ?
A) 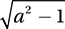
B) a2 – 1
C) 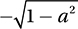
D) 1 – a2
If 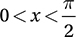 and
and 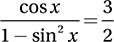 , what is the value of cos x ?
, what is the value of cos x ?
A) 
B) 
C) 
D) 
EXERCISE SET 3: TRIGONOMETRY ANSWER KEY
No Calculator
1. 7/2 or 3.5 The discussion in Lesson 9 about the definition of the sine function and the unit circle made it clear that the value of the sine function ranges from –1 to 1. Therefore, the maximum value of 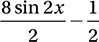 is
is 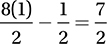 or 3.5.
or 3.5.
2. 1/36 or .027 or .028 A radian measure of π/3 is equivalent to 60°. If you haven’t memorized the fact that cos(60°) = ½, you can derive it from the Reference Information at the beginning of every SAT Math section, which includes the 30°-60°-90° special right triangle. Since 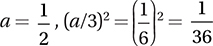 .
.
3. 1.17 
4. D 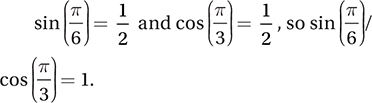 .
.
5. D If sin θ < 0, then θ must be either in quadrant III or in quadrant IV. (Remember that sine corresponds to the y-coordinates on the unit circle, so it is negative in those quadrants where the y-coordinates are negative.) If sin θ cos θ < 0, then cos θ must be positive (because a negative times a positive is a negative). Since cos θ is only positive in quadrants I and IV (because cosine corresponds to the x-coordinates on the unit circle), θ must be in quadrant IV.
6. B First, notice that a/b and b/a are reciprocals. Next, we can use the identity in Lesson 10 that 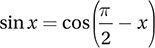 to see that choice (B) is just the reciprocal of sin x. Alternately, we can just choose a value of x, like x = 1, and evaluate sin 1 = 0.841. The correct answer is the expression that gives a value equal to the reciprocal of 0.841, which is 1/0.841 = 1.19. Plugging in x = 1 gives (A) 0.841, (B) 1.19, (C) 0.292, (D) 0.540.
to see that choice (B) is just the reciprocal of sin x. Alternately, we can just choose a value of x, like x = 1, and evaluate sin 1 = 0.841. The correct answer is the expression that gives a value equal to the reciprocal of 0.841, which is 1/0.841 = 1.19. Plugging in x = 1 gives (A) 0.841, (B) 1.19, (C) 0.292, (D) 0.540.
7. C Recall from the Pythagorean Identity that 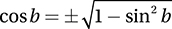 . Substituting sin b = a gives
. Substituting sin b = a gives 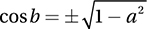 . The angle b + π is the reflection of angle b through the origin, so cos(b + π) is the opposite of cos b, which means that
. The angle b + π is the reflection of angle b through the origin, so cos(b + π) is the opposite of cos b, which means that 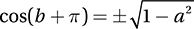 .
.
8. D Recall from the Pythagorean Identity that cos2 x = 1 – sin2 x.

Lesson 12: Imaginary and Complex Numbers
Imaginary Numbers
Powers of i
If i2 = 1, what is the value of 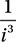 ? (No calculator)
? (No calculator)
A) i
B) –i
C) 1
D) –1
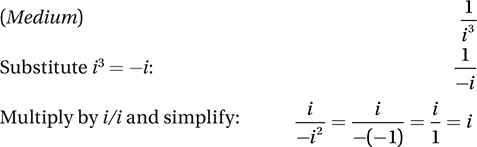
Therefore, the correct answer is A.
If i2 = –1, what is the value of i34 ? (No calculator)
A) i
B) –i
C) 1
D) –1
(Medium-hard) Since there is a remainder of 2 when 34 is divided by 4, i34 = i2 = –1; so the correct answer is D.
Complex Numbers
Complex Conjugates
Adding and Multiplying Complex Numbers
If i2 = 1, what is the value of (3 + i)(3 – i)?
A) 9 − i
B) 9 + i
C) 8
D) 10
(Easy) This is the product of complex conjugates. Recall from Chapter 6, Lesson 3, that the product of conjugates is a difference of squares. Just FOIL and simplify.
(3 + i)(3 – i) = 9 – 3i + 3i – i2 = 9 –(–1) = 10
Therefore, the correct answer is D.
If i2 = 1, and 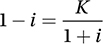 , what is the value of K2?
, what is the value of K2?
A) 2i
B) 4i
C) 4 + i
D) 4

Therefore, the correct answer is D.
Which of the following is NOT equal to i6 − i2?
A) i5 − i
B) i4
C) 2i3 + 2i
D) 1 + i6
(Medium) Our handy “powers of i” calculator from the previous page tells us that i6 − i2 = −1−(−1) = 0.
Now let’s look at the choices:
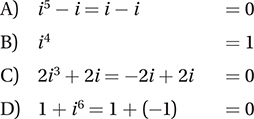
Therefore, the correct answer is B.
Dividing Complex Numbers
If i2 = 1 and 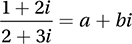 , what is the value of a + b ?
, what is the value of a + b ?

(Medium-hard) Start by multiplying top and bottom by the conjugate of the denominator:

FOIL and simplify:
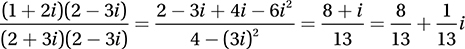
Therefore, 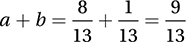 or 0.692.
or 0.692.
Absolute Values of Complex Numbers
Which of the following complex numbers has the greatest absolute value?
A) –1 – 7i
B) 2 – 6i
C) –3 + 5i
D) 4 + 4i
(Easy) Let’s calculate the absolute values with the formula.
A) 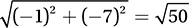
B) 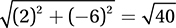
C) 
D) 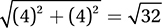
Therefore, the correct answer is A.
Using the Calculator for Complex Arithmetic
Exercise Set 4: Complex Numbers (No Calculator)
If a + bi = (1 + 2i)(3 – 4i), where a and b are constants and 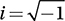 , what is the value of a + b ?
, what is the value of a + b ?

If 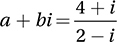 , where a and b are constants and
, where a and b are constants and  , what is the value of a ?
, what is the value of a ?

For what value of b does (b + i)2 = 80 + 18i ?

The solutions of the equation x2 – 2x + 15 = 0 are 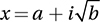 and
and 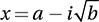 , where a and b are positive numbers. What is the value of a + b ?
, where a and b are positive numbers. What is the value of a + b ?

Given that 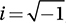 , which of the following is equal to
, which of the following is equal to 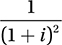 ?
?
A) 
B) 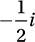
C) 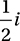
D) 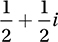
Which of the following expressions is equal to (2 + 2i)2 ?
A) 0
B) 4i
C) 8i
D) 4 – 4i
If B(3 + i) = 3 – i, what is the value of B ?
A) 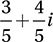
B) 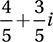
C) 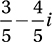
D) 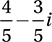
x2 + kx = –6
If one of the solutions to the equation above is 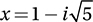 , what is the value of k ?
, what is the value of k ?
A) –4
B) –2
C) 2
D) 4
If im = –i, which of the following CANNOT be the value of m ?
A) 15
B) 18
C) 19
D) 27
EXERCISE SET 4: COMPLEX NUMBERS ANSWER KEY
No Calculator
1. 13 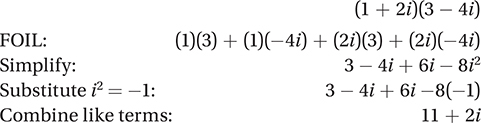
2. 7/5 or 1.4 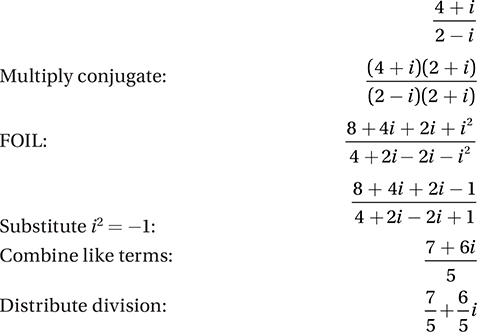
3. 9 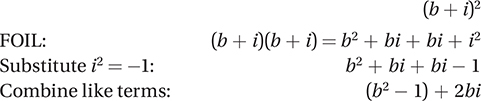
Since this must equal 80 + 18i, we can find b by solving either b2 – 1 = 80 or 2b = 18. The solution to both equations is b = 9.
4. 15 The equation we are given is a quadratic equation in which a = 1, b = –2, and c = 15. Therefore, we can use the quadratic formula:

5. B 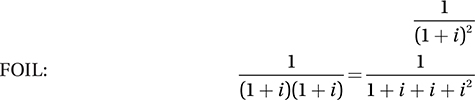
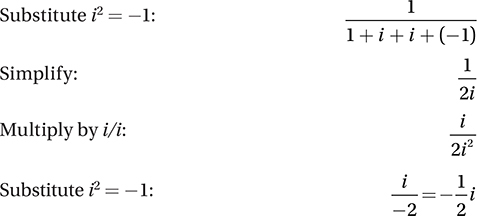
6. C 
7. D 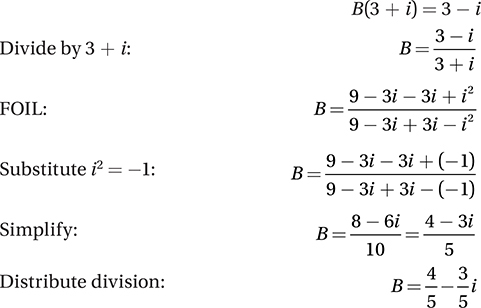
8. B 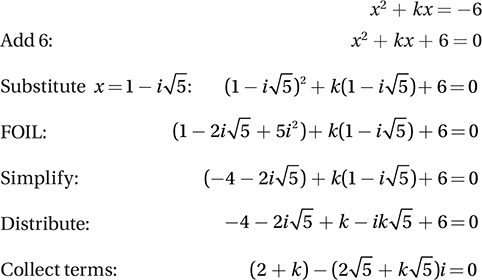
Therefore, both 2 + k = 0 and 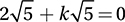 . Solving either equation gives k = –2.
. Solving either equation gives k = –2.
9. B As we discussed in Lesson 10, the powers of i are “cyclical,” and im = –i if and only if m is 3 more than a multiple of 4. The only number among the choices that is not 3 more than a multiple of 4 is B, 18.






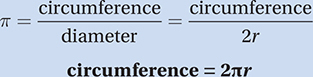
 as a conversion factor to convert a degree measure to radians, and
as a conversion factor to convert a degree measure to radians, and  as a conversion factor to convert a radian measure to degrees.
as a conversion factor to convert a radian measure to degrees.

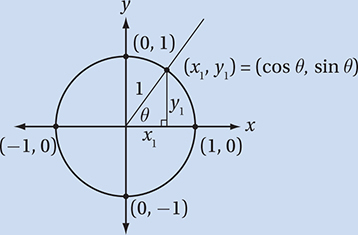
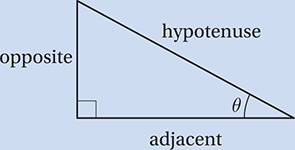


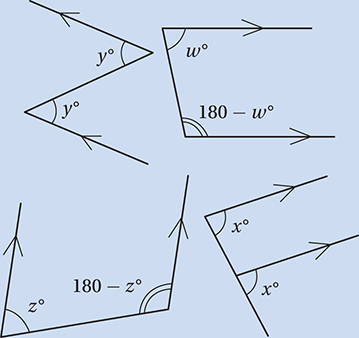
 radians). So, if one of the angles has a radian measure of x, the other has a measure of
radians). So, if one of the angles has a radian measure of x, the other has a measure of 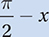 , as in the diagram above.
, as in the diagram above.
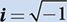

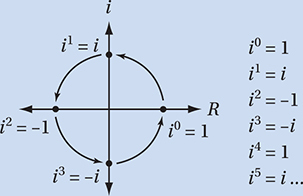
 . Every complex number a + bi corresponds to the point (a, b) in the complex plane.
. Every complex number a + bi corresponds to the point (a, b) in the complex plane.
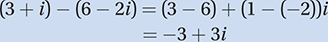
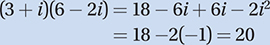

 .
.