
Those who fall in love with practice without science are like pilots who board a ship without rudder or compass.1
—Leonardo da Vinci

 hroughout history certain numbers and ratios in nature have been incorporated by artists into their creations. These numbers are picked up sometimes consciously, but usually unwittingly as subliminal messages from nature. The numbers of the Fibonacci series (1, 1, 2, 3, 5, 8 …) are often the same ones significant in genetics and in phyllotaxis (pertaining to arrangements of the veins on leaves, and the leaves and branches on plants). The ubiquitous ratio issuing from the series ϕ = 1. 618 034 … is approximated in altogether prosaic items—three-by-five index cards, playing cards, postcards, and credit cards—and also the monumental: the architecture of Bramante and Le Corbusier, the music of Mozart and Bartók, the proportions of the paintings of Velázquez and Leonardo da Vinci. To reiterate, these numbers and ratios seem to be picked up subconsciously and incorporated into works of art inadvertently, as a product of the artist’s aesthetic intuition. However, in other instances, as in the case of Leonardo, they are employed after experimentation and applied with full premeditation.
hroughout history certain numbers and ratios in nature have been incorporated by artists into their creations. These numbers are picked up sometimes consciously, but usually unwittingly as subliminal messages from nature. The numbers of the Fibonacci series (1, 1, 2, 3, 5, 8 …) are often the same ones significant in genetics and in phyllotaxis (pertaining to arrangements of the veins on leaves, and the leaves and branches on plants). The ubiquitous ratio issuing from the series ϕ = 1. 618 034 … is approximated in altogether prosaic items—three-by-five index cards, playing cards, postcards, and credit cards—and also the monumental: the architecture of Bramante and Le Corbusier, the music of Mozart and Bartók, the proportions of the paintings of Velázquez and Leonardo da Vinci. To reiterate, these numbers and ratios seem to be picked up subconsciously and incorporated into works of art inadvertently, as a product of the artist’s aesthetic intuition. However, in other instances, as in the case of Leonardo, they are employed after experimentation and applied with full premeditation.
Among all of the artistic creations of the distant past two monumental edifices stand out for their use of “nature’s numbers,” associated with “dynamic symmetry,” or the divine proportion. These are the Great Pyramid and the Parthenon, creations of a pair of great civilizations separated by two millennia.
I was five years old the first time I saw the pyramids in the valley of Giza, just outside Cairo. It was January 1946, not quite five months after the end of the war in the Pacific. My father, a young major in the Turkish army, had just been assigned as the assistant military attaché from Ankara to London. Before heading off to London, my mother, father, and I sailed from Istanbul to Alexandria, where we boarded a train bound for Cairo. In the Egyptian capital we were to make a stopover of a few days, then fly on to London, but the conclusion of the war created a logistic nightmare in Cairo, which became a bottleneck with tens of thousands of British soldiers waiting there for their trip home. What was to have been a few-day’s stopover for us stretched out to two months. There was no room on flights bound for London and the only ships making the transit to England were troop carriers.
My first impression of the pyramids was of their immensity, rising endlessly into the sky. I remember well riding a camel around the base of the Great Pyramid, where a street photographer shot our picture (Plate 3, upper right), and watching a fellah with great admiration scramble up to the top in exchange for the baksheesh that my father gave him. But the source of my abiding interest in Egyptology came about much later from discussions with Kurt Mendelssohn, a professor of physics at Oxford.
Mendelssohn was a specialist in cryogenics and medical physics, and by avocation, an Egyptologist.2 A physics education has been described as a problem-solving degree. One learns to formulate questions, cull the best approaches in order to resolve the questions, and invoke the indispensable tool of mathematics. Although Mendelssohn was not a trained archaeologist, he was applying his considerable problem-solving skills to address issues in Egyptology, and this sort of cross-semination often bears original fruit. I found his views eminently compelling. Having revisited the pyramids and made some simple calculations for myself, I am convinced more than ever of their validity—notwithstanding the fact that they may be at variance with some of the standard explanations of Egyptologists, and most certainly with anyone who attributes divine or mystical properties to the shape.
A pseudoscientific theory that can be quickly eliminated is the claim that ancient Egyptian mariners sailed across the Atlantic and taught the Mesoamericans how to build pyramids. The first pyramids appearing in Central America were in Teotihuacán, near Mexico City, and date from the first century B.C. Several centuries later the Maya built pyramids in a number of city-states, including Tikal, Uxmal, Palenque, and Kabah. Several centuries later still the Toltecs built their pyramid, known as the Castillo, in Chichén Itzá. Finally, in the fourteenth and fifteenth centuries the Aztecs built a number of pyramids in their capital, Tenochtitlan, on the site of modern-day Mexico City. Pyramid building in Central America spanned fifteen hundred years. None of the structures even slightly resemble the Egyptian pyramids, and most importantly, the Egyptians had long been out of the pyramid-building business by the time it was taken up in the New World.
“Pyramid power”—attributing special properties to the pyramid shape for preserving food, retarding aging, and maintaining the sharp edge on razor blades—has gained legitimacy as an element of popular culture. No scientific evidence exists to confirm such mysterious properties, and no physical principle can possibly confer any validity to such claims. Pyramid power belongs in the same family of pseudosciences as astrology, numerology, and palm reading, and no self-respecting scientist would accept its claims. Nonetheless, a number of remarkable mathematical relations exist in the Egyptian pyramids that can be supported by science.
“The pyramids of Egypt are immensely large, immensely ancient, and, by general consensus, immensely useless,” so Mendelssohn liked to say.3 They were built at the dawn of civilization, beginning about 4,700 years ago. So old are they that among Egyptians there is a common saying, “Man fears time, and time fears the pyramids.” In all, there exist approximately fifty pyramids spanning a millennium, and most of them are quite unremarkable. The first seven, however, built in an astonishingly brief time of a little over a century are monumental. But they outnumber the pharaohs of the Third and Fourth Dynasties who reigned during that time, rendering unlikely the generally accepted view that the exclusive purpose of a pyramid was to serve as a burial tomb or mausoleum for a pharaoh.
For a century and a half after Egyptology was conceived in the late eighteenth century, the theories regarding the building of pyramids were based largely on the writings of the Greek historian Herodotus, who had visited in the fifth century B.C. and interviewed local inhabitants. These theories had posited that the pyramids were built by slave labor; that 100,000 slaves worked simultaneously on the Great Pyramid of Khufu (or Greek, Cheops); that a giant ramp had been built in order to elevate the stones to the upper levels of the structure. When he wrote his History the pyramids were nearly as distant in time from Herodotus as Herodotus is from us. It is not difficult to see where he went wrong.
The maximum surface area of a pyramid would have been achieved just as the pyramid reached its completion. That area divided into a population of 100,000 would place approximately thirty workers in each five square feet of space along with the two-ton cubic stone that they were dragging up with them. (If only one-third of this number actually worked on the pyramid itself, that would put ten workers—plus the rock—on each five square feet of the surface.) Highly improbable! It is also highly improbable that a colossal mud-brick ramp was built in order to raise the stones. As the pyramid rose in place, the slope of the ramp would have to have been changed continuously. There would have been frequent occurrences of workers falling off the edges of the ramp. Indeed the edges themselves would have caved in frequently from the traffic, and the volume of the ramp would have to have been greater than the pyramid itself. This is simply not a cost-effective technique.
An alternative theory that makes more sense is to have a set of four mud-brick ramps, coiled around the pyramid, and rising as the pyramid itself takes shape within the square-cornered helical structure. Each ramp would start from one corner of the base, and together with the other three, spiral upward in the same direction. Three of the ramps could have been used for the workers to haul up the stones and the fourth for the workers to descend with their equipment. After the completion of the pyramid the coiling ramps would have been removed, leaving a polished limestone surface with no trace of the scaffolding. That this may have been the best method certainly does not guarantee that it was the one employed. The builders, although lacking modern technological devices, were no less clever than modern engineers and architects.
Finally, it is also unlikely that the pyramids were built with slave labor. It may have required as many as 20,000 soldiers to keep 100,000 slaves in line. And who was to feed this mass of people? Fortunately, a number of clues exist to resolve the critical issues of who built the pyramids and why, and the lesser question of the number of workers required. Until the emergence of the dynastic system around 3000 B.C., a jumble of tribes occupied Upper and Lower Egypt, making invasions and threats of invasion common. The trend toward unification began with the dynastic system, but was not fully achieved until around 2650 B.C. With the reign of Djoser (2668–2649 B.C.), the second pharaoh of the Third Dynasty.
When kings of the First and Second Dynasties died, they were buried in tombs located below mastabas, one-story slab-like buildings. These monoliths, characteristically rising with a slope of 3:1 (3 parts rise to 1 part run), were not especially difficult for grave robbers to penetrate. Then suddenly with the reign of Djoser the unification of Upper and Lower Egypt was complete. A powerful army was formed; the sun god Ra became overwhelmingly prominent, and pyramids became the preferred burial tombs for pharaohs. What better way to worship the sun god than by building a man-made mountain in the form of a sunburst effect—a pyramid. The farmers, working seasonally (according to the rise and fall of the flooding Nile), might have been encouraged to come to work on the pyramid project as a way of buying indulgence for the afterlife, the next life being far more important than this ephemeral one. Thus, rather than a reviled project of slave labor, a pyramid would have been a public works project, a labor of devotion. This could all be a stretch, but the improbable ideas of the 100,000 laborers, 20,000 guards, and the need to feed them all, makes this stretch a little less unappealing. In the last decade of the twentieth century, the eminent Egyptologist Zahi Hawass reported the discovery of workers’ houses near the base of the pyramids, replete with accoutrements of ordinary subjects, pointing to the validity of Mendelssohn’s hypothesis of free men being employed on the project. Hawass estimates that 20,000 workers were employed on the Great Pyramid at any given time, and that they worked for about two months before returning to their villages, when another group of 20,000 took their place.
Can anyone really be identified as the first scientist, or deserve the accolade “architect of civilization?” In fact it is not difficult to make a case for a legendary character by the name of Imhotep being accorded both of these astounding mantles. Djoser’s step pyramid in Saqqara was the first ever built, and the architect of the project was known to have been Djoser’s chief vizier, Imhotep, a medical sage, astronomer, mathematician, and architect. Imhotep came to be revered as a god of healing and was in time identified by the Greeks with their own Asklepios. Isaac Asimov in his biographies of scientists singled out Imhotep as the very first, and added: “there was not to be another for over two thousand years.”4 Moreover, if the hypothesis is valid that the building of the first pyramid served as the catalyst for the unification of Egypt, and if the project was masterminded as a public works project by one man—Imhotep—then he is indeed deserving of those honorifics.
The step pyramid, rising to a height of two hundred feet—comparable to a twenty-story building—is not a true pyramid, rather it resembles a set of six mastabas, gradually decreasing in footprint and height, stacked one on top of the other. In its internal structure, however, there is most likely a tower rising with a slope of 3:1, buttressed by the series of mastaba-like steps, each rising with the same slope. Without dismantling the pyramid it would be impossible to confirm this, but a clue is offered by the second pyramid, built in Maydum, thirty-five miles south of Saqqara, and otherwise known as the “collapsed pyramid” (Plate 3, upper left). This failed structure had initially been designed as a step pyramid modeled after Djoser’s. The lowest two courses are still present, and these reveal a finished surface, suggesting that it was not until the pyramid’s completion as a step pyramid that the builders returned to modify it, indeed, to convert it into a true pyramid. The rubble of the collapsed structure presents a silhouette very much resembling a colossal chocolate kiss. The desired angle of slope of the “true pyramid” was 52°, a point that will gain significance later on. A number of factors conspired to bring about the rockslide of the collapsed pyramid: the angle at which the additional blocks were stacked; the polished surfaces of the finished steps failing to give these blocks sufficient adhesion; the relatively small size of the blocks, and the fact that the blocks were not adequately squared or precisely fitted.5 For a pyramid the size of the collapsed pyramid, composed of limestone, the pressure on stones at the base would be of the order of 50 kg/cm2 (710 lbs./sq. in.) if the stones were precisely squared. And the pressure at the base would be around 1,000 kg/cm2 (over 14,000 lbs./sq. in.) on the protruding edges if the stones were not precisely squared. Whereas limestone can withstand 50 kg/cm2 pressure, it would crumble when subjected to 1,000 kg/cm2. Candy makers have long known that a cone-shaped chocolate candy, when placed on a baking sheet and heated, assumes the shape of a chocolate kiss as a result of plastic flow. And it is the classic effect of plastic flow that the collapsed pyramid displays. Moreover, the collapse would have occurred so quickly that the workers on its surface would not have had time to escape to safety. Mendelssohn suggests that a systematic excavation of the rubble surrounding the exposed tower, the core, may well uncover a large number of buried skeletons still preserved by the dry climate.
At Dashur, not far away, the building of a third pyramid had commenced even before the completion of the pyramid at Maydum. The initial plan evidently was to make it a true pyramid with slopes of 52°. Then mysteriously midway up the sides the slope was changed to 43.5°. The prevailing theory in Egyptology is that the king for whom the pyramid had been designated passed away prematurely, and the builders decided to truncate the building to save time. But a calculation reveals that the time saved in the process—based on the stone saved—is merely 10 percent. More likely, as Mendelssohn suggests, it was the sudden catastrophic collapse of the pyramid in Maydum that triggered the builders’ desperate modification of their own pyramid. Because of its double-angle the edifice is known as the bent pyramid. As it is, the pyramid is well over 300 feet high, or higher than a thirty-story building. If the lower surfaces, rising at 52°, were to be extrapolated, however, they would meet at a point well above 400 feet, and put this pyramid into the same class of colossal pyramids as the Khafre (or the Greek, Chephren) and the Great Pyramid of Khufu in the valley of Giza.
The fourth pyramid, built also in Dashur, was again a true pyramid and had the field-tested, safe angle of 43.5° for its surfaces. This is the red pyramid, the name derived from the reddish stone used as facing on its surfaces, and is associated with the pharaoh Snefru, father of Khufu, and grandfather of Khafre. This pyramid has a height of over 300 feet, comparable to that of the bent pyramid.
Then in the valley of Giza the fifth and sixth pyramids—Khufu and Khafre—were built, climbing majestically at 52° to elevations comparable to fifty-story buildings. And finally, a seventh pyramid, Menkaure, a relative runt at twenty-five stories tall, was built in the same valley. In 1995 members of the David H. Koch Pyramids Radiocarbon Project undertook a definitive program to date the pyramids. They collected tiny bits of organic material embedded in the gypsum used for mortar in the pyramids and tested them for their carbon-14 (C14) activity rates. (The half-life of C14 is 5,715 years, which means that in 5,715 years only half of the original activity will remain in the sample.) The building of the entire complex at Giza was determined to have lasted eighty-five years, from 2589 to 2504 B.C.6 This marks the highest development of Egyptian pyramid building.
A dramatic decline followed this flurry of activity. And although pyramids continued to be built for another 2,500 years, they were all vastly overshadowed by the first seven, especially numbers five and six. Indeed, by the end of the Fourth Dynasty the Egyptians were essentially out of the pyramid building business.
By the mid-twentieth century aerial photography offered archaeologists a bird’s-eye view of the topography of the land and the opportunity to visualize the pattern at the site of human settlement—in the same sense that a person examining an oriental carpet from a normal standing view has a considerable advantage in appreciating the pattern of the carpet than someone examining one tiny portion of it. A little later aerial infrared photography provided additional capabilities. Materials possessing different heat capacities cool off at different rates. Since rocks cool off faster (and emit infrared radiation with greater intensity) than the surrounding earth, infrared photographs can reveal buried foundation walls even where the overlying ground is perfectly smooth. A substantial step beyond aerial photography is offered by remote sensing—satellites that provide much larger areas to view and much wider ranges of the electromagnetic spectrum. Exploration of natural resources and investigation of ecological concerns have been the greatest benefactors of this technology. Along with successes in establishing the geomorphology of various sites, hitherto unsuspected ruins have been discovered. Lying deep below shifting sand dunes in Saudi Arabia the ruins of the fabled lands of frankincense and myrrh were discovered by NASA in the early 1990s.
A conjecture was presented earlier in this chapter that it might have been the sunburst effect that inspired the shape of the pyramid—a man-made mountain for the pharaoh to ascend to the sun god. In a recent paper geologist Farouk El-Baz, a specialist in remote sensing technology, offered an alternative conjecture, but also one inspired by nature.7 Remote sensing technology had revealed below the obscuring layers of sand in the eastern Sahara the existence of dried riverbeds, meandering in wide swaths. This was offered by El-Baz as evidence of vastly different climatic conditions in the distant past, and archaeological excavations of the area have turned up sites of human settlement. Catastrophic drought in lands west of the Nile took place around 5,000 years ago and forced the migration eastward of the nomadic inhabitants. According to El Baz’s theory it is the cultural melding of desert people and the pioneer farmers of the Nile valley that ultimately catalyzed the unification of Upper and Lower Egypt. The memory the nomads brought with them of the eastern Sahara may have served six or eight generations later as the inspiration for pyramids, and even the Sphinx. Natural formations created by the action of water and wind, the latter in a well-defined north-to-south direction, may have created the models that served the generation of pyramid builders.
Earlier we saw that King Djoser’s step pyramid comprised six mastabas stacked on top of one another, each rising at a 3: 1 slope (or an angle of 72°). That slope appeared again in the core of the collapsed pyramid, and it was speculated that most likely a tower of the same slope was embedded in the structure of the step pyramid. Extrapolating the sides of the tower creates the “golden triangle”—the isosceles triangle with the angles 36°—72°—72° (Plate 3, upper left). Had Imhotep, the mastermind behind the step pyramid, recognized the golden triangle? Most likely not. He simply knew from the design of mastabas that a slope of “three parts rise to one part run” was a safe angle, and a healthy design to incorporate into his design.
The second pyramid to be built—the collapsed pyramid—was initially completed as a step pyramid with 72° slopes, but then a drastic modification was applied in an attempt to convert it by way of a casing into a true pyramid. The angle chosen for this casing was 52°. Though the pyramid collapsed due to fatal design faults discussed earlier, that 52° angle has a special significance. If a circle were to be laid out using a prescribed radius the circumference would of course be 2π times the radius. If that circle is formed into a square so that the circumference of the original circle and the perimeter of the square are exactly the same, the sides of a pyramid constructed on that base, with a height equal to the radius of the original circle, would have a slope of 52°. The simplicity of that scheme may have been the inspiration in the choice of the angle, or it may have had some magical connotations. We do not know.
In the bent pyramid the lower portion of the pyramid was built at 52°, but midway up, the angle was changed to 43.5°. Following Mendelssohn’s conjecture, the builders in desperation changed the angle to stave off the collapse of the structure, having just witnessed the collapse of pyramid two. The ratio of the perimeter-to-height of a 43.5° pyramid happens to be 3π, lightening the load and again suggesting a preoccupation with π. The fourth pyramid in the series, the red pyramid, was also built at the safe angle of 43.5°. The bent and red pyramids have survived the better part of 5,000 years.
In the case of the Khufu the parameters are well known: 230 meters (or 500 cubits) on each of four sides, and a height of 146.4 meters originally (which has since been reduced to 137 meters by erosion or clandestine quarry activity). The ratio of the full perimeter 4 × 230 meters = 920 meters divided by the 146.4-meter height is 2π. The stones or blocks used are much larger than those used in the earlier pyramids (of the order of 1 meter on an edge) and very precisely shaped. In building the pyramid a circle with a circumference of 4 × 230 meters (or 920 meters) was laid out using rope. The circle was then physically “squared” by teams of laborers tugging at diametrically opposite points on the rope, achieving the required right angles at the corners by making sure that the diagonals were precisely the same length.
For the original measurements, the ratio of one edge of the base to altitude is 1.57, fairly close to the golden ratio of 1.62, and if one were to inscribe the Khufu in a golden rectangle, the tip of the pyramid would stick out only slightly. More importantly, focusing on just the triangular shape of one face, the ratio of the altitude of a face to one-half the length of the base is exactly 1.62. But what is far more intriguing is a computation involving the areas of the facades and the base, first recognized by the mathematician and astronomer Johannes Kepler (1571–1630). The base has an area Ψ = 52,900 m2, the four sides have a combined area ∆ = 85,647 m2. These values can be related as follows:
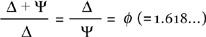
This is a restatement of the law of divine proportion.
The unexplained question here is one of a “chicken and egg.” What came first for the ancient Egyptian architects who designed the pyramids at Giza? Were they attempting to build imposing structures whose surface areas satisfied the relationship described above? Did they wish even before the first stone was laid in place for the final product to relate in some way to the divine proportion? Did they know that for a pyramid shape to reveal the law of divine proportion its edges must rise at an angle of 52° (Plate 3, center left)? In other words, were they fully aware of the implications of their design? Did they know what they had accomplished in this regard? The answer to all these questions is, “probably not.” Most likely the builders simply wanted to have the height of their pyramid defined by the radius of a circle the circumference of which equaled the perimeter of the pyramid. It was fortuitous that such a pyramid would also exhibit the properties of divine proportion. Whether these precise symmetries are intentional or accidental, we are justified in describing the Khufu and Khafre as golden pyramids.
The Greek army in 479 B.C. defeated the Persians at Plataea, ushering in a period of peace and prosperity, the Golden Age of Greece. The period saw an unprecedented explosion in artistic creativity that included the development of the Athenian Acropolis in honor of the city’s namesake and patron goddess Athena. In 447 B.C. work on the temples astride that massive stone outcropping commenced. Fifteen years later in 432 B.C. the Parthenon was completed, although work on the other buildings continued for another thirty-five years. By any measure the Parthenon is the most sublime of all extrovert buildings ever built. Although the Greeks could make arches by fitting together wedge-shaped stones, they had not extended the idea into spanning large spaces by making domes of such stones. That would await the Romans with their Pantheon in the first century A.D. Hence the interior of the Parthenon, busy with columns to support wooden beams and a heavy roof, was anything but commodious, anything but introverted.
Collaborating on the design of the Parthenon were Athens’s premier sculptor-architect Phidias and the architects Callicrates and Ictinus. The columns—fluted shafts topped with capitals of the Doric order—supported a pediment with Phidias’s sculpture, carved as no stone had ever been carved before. The friezes consist of ninety-two metopes in low relief alternating with vertically fluted triglyphs. The sculpture in the pediment, depicting the Olympians after their victory over their dreaded enemies, the Titans, is meant to be emblematic of the victory of civilization over barbarism. The east and west facades of the Parthenon both form golden rectangles, or exhibit length-to-width ratios of ϕ. As for the assignment of the symbol ϕ to the golden ratio, this is an entirely modern development. It was early in twentieth century that American mathematician Mark Barr first denoted the golden ratio by Phidias’s monogram.
The architects introduced several imaginative measures to eliminate unfavorable optical illusions. For example, a perfectly straight horizontal line would normally appear to sag in the middle, because it would naturally be sighted against the horizon, which itself has a convex curvature; columns which are cylindrical would appear to be concave midway up. In order to counter these effects, the Parthenon was built on a convex base of a 5.7-kilometer (3.5-mile) radius of curvature. Columns rising perpendicular to a convex curvature, however, would diverge slightly at their tops, an effect that would be barely visible, but a source of subtle discomfort. In order to avoid a splayed appearance the columns were aimed or sighted to converge at a common point approximately 2.4 kilometers (1.5 miles) in the sky. The midsections of the columns incorporated a slight bulge, entasis, negating the optical illusion in the other direction. How they arrived at these happy numbers is a mystery, but the scheme clearly works! Finally, they used fluted columns, grooved vertically, as opposed to plain cylindrical columns, which would have appeared lumbering and heavy.
It is the confluence of all of these elements—the artificial constructs to correct for detracting optical illusions, unerring proportions, the majestic perch atop the Acropolis, and Phidias’s immortal sculpture—all working in concert to render the edifice the epitome of classical Greek architecture. As for Phidias’s carvings that once adorned the pediment and were painted in vivid colors, only a scant few remain on site. Virtually the entire original assembly was carted off to the British Museum by Lord Elgin early in the nineteenth century. The pediment in its present state offers little clue to the original beauty of the edifice. A scale model of the original carvings, however, is on display in the small museum atop the Acropolis, offering a glimpse into the Parthenon’s original magnificence (Plate 4, bottom).
On a personal note, I have been to the Parthenon many times. On one occasion I was there with a friend, an academic dean.8 At the end of the day, when we were just about to leave the site he bent over, pretending to tie his shoe laces, and picked up a loose stone as a souvenir. Clearly nervous, he confided to me his utter terror of being discovered in stealing a piece of the hallowed edifice, “Who knows what part of the building it came from?” he mused in a whisper. But just then a large truck appeared and dumped tons of stones for tourists visiting on the next day.
Although only a single column remains of the Artemisium (temple of Artemis, or Diana for the Romans) in Ephesus, it is believed that some of the same proportions with which its builders imbued the Parthenon were also featured in the Artemisium. This Artemisium was considerably larger than the Parthenon in all of its dimensions and ranked as one of the Seven Wonders of the Ancient World, a distinction not bestowed on the Parthenon. In a painful tragedy of history a madman burned down the Artemisium in the same year that Alexander the Great was born in Macedonia. The reason the arsonist, Herostratos, gave for his deed: he wanted his name to be remembered in perpetuity. In the meandering path that he took with his armies through Asia Minor, Alexander stopped over the site and founded a new Ephesus a few kilometers away. It is the ruins of that magnificent city founded by Alexander that tourists see on their visits to Ephesus now.
That nature inspires the designs of artists and architects is inarguable. Columns and capitals are another case in point. Technically a column consists of the vertical shaft, the stem topped by a block, the capital. The Egyptians usually employed cylindrical columns with capitals modeled after flowers and pods of lotus and papyrus plants, displayed nowhere with more drama than in Luxor and Karnak. In Knossos on the island of Crete the columns are evocative of the trunks of trees, inverted so that the upper parts of the column display a widened, flange-like appearance. The capitals of the Parthenon’s columns were in the Doric order, consisting of plain slabs, but two other types of capitals employed by the Greeks—the Ionic and the Corinthian—most certainly reflected patterns in nature. Capitals in the Corinthian order, the most decorative of the lot, were embellished with acanthus leaves, and became favored by the Romans. The swirling shape of the chambered nautilus, incorporating the logarithmic spiral into the capital design, inspired the Ionic order.
The artists of classical antiquity incorporated the divine proportion into a variety of objects, ranging from vases to eating utensils, from paintings to statuary. To the sculptors of classical Greece and Rome the divine proportion was recognized as ideal for the human anatomy: the length of the fingers to the hand, the hand to the forearm, the forearm to the full arm, etc. Among these proportions there is the ratio of one’s height to the height of one’s navel. In the statue of Venus de Milo (second century B.C.) the ratio of the height to the height-of-navel is close to ϕ. This can be effectively dramatized by inscribing the subject in a golden rectangle and constructing a square in the lower portion of the rectangle. The upper edge of the square will then be seen to pass very close to the navel. The interested reader could make similar observations in numerous other classical masterworks, including Aphrodite, Eros, and Pan (c. 100 B.C.), Hermes and the Infant Dionysus (c. 340 B.C.), and the father with his two sons in the Laocoön (first century A.D.). In that last work it is necessary to “straighten” the three figures into upright positions digitally, or employ the low-tech method of measuring the subjects in the statue directly with a pliable tailor’s tape measure. Locked in mortal combat with a number of serpents coiled around them, all three figures are slightly hunched over in anguish and stress, their navels approximately 0.618 of their heights.
I recently put the hypothesis of ϕ being the ratio of one’s height to the height of one’s navel to the test with a group of twenty-one university students, ten male, eleven female. The point of the exercise ostensibly was to demonstrate the statistical analysis of data, by computing averages, uncertainties, and standard deviations. In reality such a small group cannot yield meaningful statistics. Nonetheless, it was a blind test, and for the record, the results for the average and standard deviation measurements were 1.618 ± 0.04.
Any discussion of the ancient Greeks in the context of the divine proportion would be incomplete if it did not include mention of Yale University art historian Jay Hambidge. Professor Hambidge started in the 1920s to publish an exhaustive series of analyses of the Hellenistic vase. He found many of the vases he examined to be conducive to analyses in terms of the golden ratio. It was his work, rather than that of anyone else in modern times, that rekindled interest in dynamic symmetry and divine proportion in general.9
A figure in two dimensions has two types of symmetry. It has line symmetry if a line can be drawn through it so that each point on one side of the line has a matching point on the opposite side at the same perpendicular distance from the line. An equilateral triangle possesses three-fold line symmetry; a square, four-fold line symmetry; a regular polygon of n sides, n-fold line symmetry. Finally, a circle has infinite-fold line symmetry.
In discussing point symmetries in this short technical interlude, we begin by introducing a convenient unit for measuring angles: the radian, where π-radians corresponds to 180°. A figure has point symmetry if it can be rotated about a point so that it replicates its original shape (but specifically excluded is the trivial case of rotation by 2π radians (360°), thus a full turn. An equilateral triangle can be rotated about a point at its center by 2π/3 radians (120°) and by 4π/3 radians (240°) in fulfilling the condition above. A square can be rotated through a point at its center by multiples of 90° (2π/4, 4π/4 and 6π/4 radians) in order to replicate the original picture. A regular polygon of n sides should possess (n–1)-fold point symmetry, with rotations by 2π/n, 4π/n, 6π/n, … 2(n–1) π/n radians, all recreating the original shape.
The expression mathematical mosaics refers to configurations of regular polygons which completely cover a surface so that an equal number of polygons of each kind are arranged around a regular array of points called lattice points, defining the vertices of the polygons. In practical terms, if a surface is to be covered by the same kind of regular polygon, the possibilities turn out to be limited: equilateral triangles, squares, and hexagons are the only figures that can form a homogeneous pattern of tiling. If the idea is extended to three dimensions, optimization—in this instance, minimization of material for construction of walls with an attendant maximization of the internal volume—is achieved by hexagonal tiling. Meanwhile the construction also produces unusual structural strength against compression. Such are the results of applying calculus to a geometric and engineering problem. But long before mathematicians recognized hexagons as viable shapes for flat space tiling, nature had made the discovery. Bees were constructing their honeycombs in this pattern, presumably having made the calculation intuitively!
Meanwhile, pentagons, requiring rotations by 2π/5 radians to replicate themselves, cannot tile a flat surface by themselves. The resulting pattern displays rhombus-shaped gaps. Seven-sided regular polygons, heptagons, requiring rotations by 27π/7 radians, would not be able to form a flat mosaic pattern, since adding new heptagons would result in overlapping rhombus-shaped areas. Similarly it would be impossible to tile with octagons (eight-sided regular polygons) or with decagons (ten-sided regular polygons).
Combinations of regular polygons or an unlimited number of irregular polygons can all be used for tiling, for example, parallelograms, isosceles triangles, and so on. A common pattern of tiling is seen in the combination of octagons and squares in a periodic array.
In the late 1970s an intriguing mathematical tiling pattern emerged from the drafting board of the Oxford mathematical physicist Roger Penrose. In his pattern two types of rhombuses—“skinny rhombuses” with interior angles 36° and 144° and “fat rhombuses” with the angles 72° and 108°—are assembled in the aperiodic pattern that is the heart of Penrose tiling. The skinny rhombus, bisected, would yield a pair of isosceles triangles with angles 72°—36°—72°, the golden triangle. Thus it should be no surprise that on an infinite plane the ratio of the number of fat rhombuses to the number of skinny rhombuses is the golden ratio ϕ (= 1.618 034 …), the irrational number generated from the Fibonacci series.
Beyond the regular polygon used in mathematical tiling are a virtually unlimited number of abstract shapes, all understood in terms of symmetry operations allowed on a flat surface. The Moors in Northern Africa and Spain, the Selçuk and Ottoman Turks in the Middle East, and the Persians raised two-dimensional abstract design and calligraphy to a level of extraordinary sophistication and beauty. The Moors adorned their special buildings with patterns revealing tacit understanding of space symmetry concepts, epitomized in the tiles of the Alhambra Palace in Granada and the Great Mosque in Córdoba.
During the Islamic Mughal rule in north central India, Shah Jah ā n created an architectural masterpiece for the ages in the Taj Mahal, a mausoleum for his favorite wife, Mumt ā z Mahal. Constructed wholly of white marble, the seventeenth-century edifice raises calligraphy and mathematical mosaics to a peerless art form. Typically seen among the carved marble window traceries are aperiodic patterns of six-pointed stars, regular hexagons, and stylized tulips in two different sizes.
The twentieth-century Dutch crystallographer Maurits Cornelis Escher (1898–1972), intrigued by graphics techniques, employed symmetry operations in generating inexhaustible patterns of realistic (and sometimes mythical) figures in his graphic artwork. Some of his graphics consisted of flattened images, others incorporated perspective—stairs and lattice structures, knots, and Möbius strips. Escher often challenged the viewer with the liberties he took with one-point, two-point, three-point, and even four-point perspective, for example, ascending staircases that transform mysteriously into descending staircases. Unencumbered by religious interdicts such as the ones imposed on Islamic artists (forbidding the depiction of humans and animals), Escher utilized these figures with abandon in his symmetric musings. Dark horsemen facing the right are interposed between light horsemen facing the left. The symmetry operations that leave the pattern invariant are simple translation in vertical and horizontal directions plus reflection. In the reflection process the shading of the horsemen is also interchanged.
Earlier we saw that the different types of regular polygons that could create a homogeneous mosaic were only three in number. However, irregular mosaics, consisting of different regular polygons, are unlimited in number. Escher played off the same symmetry operations that characterize mathematical mosaics and created enticing, albeit simple, graphics. In light of his early training as a crystallographer, it is understandable that his art would often incorporate scientific themes. Later, in Chapter 7, we will take a closer look at one of Escher’s creations. More immediately, however, we shall examine three-dimensional bodies—regular and semiregular polyhedra—and their underlying symmetries.
The regular polyhedron is defined as a three-dimensional solid comprising regular polygons for its surfaces—and with all its surfaces, edges and vertices identical. The regular polyhedra are the four-sided tetrahedron, the six-sided cube, the eight-sided octahedron, the twelve-sided dodecahedron, and the twenty-sided icosahedron (Figure 5.1). There are only five types, a happenstance that Lewis Carroll described as “provokingly few in number.” Although all five types had been identified by Pythagoras two hundred years before Plato was born, they are nonetheless collectively known as platonic solids, named in honor of Plato by the geometer Euclid.
For the ancient Greeks all material in nature was composed of only four elements—earth, fire, air, and water—taken in different admixtures. Atoms of fire had tetragonal shape; atoms of earth, cubic; atoms of air, octahedral; and those of water, icosahedral. The number of regular polyhedra, however, clearly outnumbered by one the number of recognized elements. To the Pythagoreans, the fifth polyhedron had monumental significance. An omerta, or a code of silence, was imposed regarding the dodecahedron, divulging its secret meaning—the shape of the universe—to outsiders could earn a traitor the death penalty! The Pythagorean Academy was located in Crotone, on the sole of the Italian boot. Founded by Pythagoras about the sixth century B.C., the fields of academic interest were mathematics, natural philosophy, and music. Mysticism and numerology, however, seem to have characterized the underlying philosophy of the Pythagoreans, a cult in the modern sense.

Figure 5.1. Regular and semi-regular polyhedra and the golden pyramid: (A) tetrahedron, four sides; (B) cube, six sides; (C) octahedron, eight sides; (D) dodecahedron, twelve sides; (E) icosahedron, twenty sides. (F) Fifteen golden rectangles span the interior of the icosahedron (only three of which are seen here); (G) the truncated dodecahedron; (H) the truncated icosahedron, or an icosahedron with its vertices clipped; (I) the geodesate, or a tessellated dodecahedron, closely resembles Buckminster Fuller’s geodesic dome; (J) the stellated dodecahedron; (K) the stellated icosahedron; (L) the golden pyramid
Intuitively it is quite easy to see how there can be only five platonic solids. The plane figure with least number of sides is the triangle. The smallest number of triangles around a point in three dimensions is three. Also, four and five triangles form polyhedra, but six tile the plane. Thus only three, four, and five can form polyhedra. With squares, three around a point form a cube, but four tile the plane. With pentagons, again only three is possible, with four going beyond the plane. Accordingly, three, four, and five triangles; three squares; and three pentagons are all that fit around a single point in three-dimensional space.
In the early seventeenth century Johannes Kepler, German-born mathematician and assistant to the Danish astronomer Tycho Brahe, succeeded in formulating the three laws of planetary motion. Before actually getting his hands on Tycho’s observational data and undertaking the massive computational effort which ultimately lead to these laws, however, he ruminated on the peculiar pattern of spacing between the planets. Among a number of mathematical schemes that he considered was the notion of using the five regular polyhedra as the spacers, and the task as he saw it was to identify the order in which they were to be employed (Figure 5.2).
An intriguing encounter with the dodecahedron takes place in Salvador Dali’s Sacrament of the Last Supper. In the painting in the National Gallery of Art in Washington, the Twelve Apostles, heads bowed, are seen flanking the figure of Christ. In the background is the setting sun, and on the tabletop, the shadows cast by the apostles, the piece of bread, and the glass of wine. The figure of Christ, somewhere between transparent and translucent, casts no shadow. At the top of the scene of Dali’s Last Supper are the sheltering and protective arms of God, face unseen. But just below God’s arms, and framing the scene, is the unmistakable shape of a dodecahedron. The painting possesses almost perfect bilateral symmetry, one side mirroring the other. The positions of Christ’s arms are not symmetrical, nor is the configuration of the islands in the background (a view thought to be from Dali’s house in Catalonia). The painting reflects some of the artist’s experiences from the late 1930s and 1940s. Dali, like many other artists and intellectuals of the time, including Picasso, sided strongly against the fascists in the Spanish Civil War. During this time he was profoundly influenced by a meeting with the elderly Sigmund Freud. After a period of agnosticism he returned to Christianity and abandoned the themes of anarchy in his art. Finally he found inspiration in examining anew Renaissance art of the early sixteenth century.

Figure 5.2. Johannes Kepler’s geometric construction employing the five regular polyhedra as “spacers” between the orbits of the known planets (Courtesy History of Science Collections, University of Oklahoma, Norman)
Dali’s Last Supper exudes a dreamlike quality (a clear influence of Freudian psychology) and a classical perspective (the influence of the Italian Renaissance and, especially, Leonardo da Vinci’s Last Supper). Dali, a superb technician with a brush, created this surrealistic work in 1955. It is pleasantly puzzling. In both works Jesus Christ is backlit—in Leonardo’s mural, by a window immediately behind him, and in Dali’s painting, by the setting sun. Of course, Dali’s Last Supper does not begin to approach the psychological drama and power captured in Leonardo’s Last Supper (which we will look at in more detail in Chapter 9). Regarding the dodecahedron incorporated in the composition, Dali explained: “I wanted to materialize the maximum of luminous and Pythagorean instantaneousness, based on the celestial Communion of the number twelve: twelve hours of the day—twelve months of the year—the twelve pentagons of the dodecahedron—twelve signs of the zodiac around the sun—the twelve Apostles around Christ.”
The ratio of the painting’s length to width is 1.603, close to the golden ratio ϕ =1.618. Whether this choice of proportion was a conscious attempt to employ that classical ratio, a product of his intuitive artistic sensibilities, or a simple coincidence, he did not explain. But it would not be wild speculation to presume that Dali—a numerologist with a deep mystical bent—would have been euphoric had he known that he had unwittingly incorporated in his painting the Pythagorean Academy’s closely guarded secret—the dodecahedron—emblematic of the universe.10 It is unlikely that he knew about the significance, for he would have mentioned it in his explanation of the painting.
By mixing a variety of regular polygons, while adhering to the requirement that at all vertices the arrangement of polygons is the same, one obtains the solid figures called semiregular polyhedra. For example, the icosahedron with twenty equilateral triangles, in having its vertices cropped, becomes a truncated icosahedron, a figure characterized by two hexagons and one pentagon at each vertex. The modern soccer ball is a truncated icosahedron, usually with the hexagonal leather patches dyed white and the pentagonal patches, black.
Another semiregular shape, the cuboctahedron, has fourteen faces—eight equilateral triangles and six squares—each vertex surrounded by the sequence of a triangle, a square, a second triangle, and a second square (classified by the scheme 3-4-3-4). Here a physical significance is to be found: the arrangement offers the model for close-packing of identical spheres in space, which is of considerable interest in crystallography. In the following section the classification of crystal structure will be presented within a more general topic about patterns in nature.
A subtle connection exists between the icosahedron and the golden rectangle. Close examination of this polyhedron, supporting twenty triangular sides and thirty edges, reveals the existence of golden rectangles spanning opposite pairs of edges. Each pair of diametrically opposite edges forms two shorter sides of a golden rectangle, and since there exist thirty edges on an icosahedron, there must exist a total of fifteen embedded golden rectangles in all. The relationship between numbers of vertices, faces, and edges for any convex polyhedron—regular or irregular—was given by the great Swiss mathematician Leonard Euler (1707–1783): the number of vertices plus the number of faces equals the number of edges plus 2.
There also exist classes of solid objects that are neither regular nor semiregular, but are familiar to most individuals, for example, pyramids (triangular sides with a variety of polygonal bases), prisms (cylindrical shapes with a variety of polygonal cross-sections), the frustum (a cone with its pinnacle truncated parallel to its base. The tetrahedron encountered earlier is a pyramid with a triangular base. Certainly the most familiar of pyramids is the square-based pyramid employed by the ancient Egyptians and discussed at length earlier in this chapter. As the number of sides of the base increases, the resulting figures become the pentagonal pyramid, hexagonal pyramid, and so on—indeed, in the limit when the number of sides reaches infinity, the pyramid transforms to the familiar cone. In this context one special square-based pyramid discussed earlier was the pyramid whose base perimeter exactly equals 2π times the height of the pyramid itself. The condition, of course, created the pyramid rising at 52° and incorporating the divine proportion. (Recall this relationship was displayed in the Khufu and the Khafre pyramids.)
Most individuals can readily visualize the simpler regular polyhedra, such as the cube and the tetrahedron, and even the octahedron. The more complicated of the regular polyhedra (the dodecahedron and the icosahedron) and semiregular polyhedra (the truncated dodecahedron and the truncated icosahedron) require considerably more cogitation. Here mentally rotating the body around various axes may be required in order to examine the underlying symmetry. But at the beginning of the twenty-first century, it is more convenient to write equations and computer code and have a high-speed computer draw the polyhedra. In the stellated dodecahedron there exist pentagonal pyramids protruding from each of the twelve surfaces, and in the the stellated icosahedron, triangular pyramids or tetrahedra protrude from each of the twenty triangular faces. Finally, in the dodecahedral geodesate, the surfaces of a dodecahedron are tessellated onto the surface of a circumscribed sphere. This is the geodesic sphere of Buckminster Fuller (1885–1983), patented in 1954. The best-known dome built in this style is the sixty-five meter (200 foot) high dome at the United States Pavilion in Expo ’67 in Montreal. An inner layer of hexagonal elements are connected to triangular elements on the outside, and those, in turn, are overlaid by a transparent plastic skin.
Dispersed among Leonardo da Vinci’s manuscripts, along with drawings, notes, doodles, and computations, are also a variety of polyhedral creations, products of what Leonardo called his “geometric recreation.” With possibilities for endless variation, these regular and semiregular polyhedra seem to have been a source of fascination for him. Leonardo was born at approximately the same time as Johannes Gutenberg’s publication of the first book in movable type in Europe—the Bible—and participated in the publication of only one book, De divina proportione (Venice, 1509). De divina proportione was the product of collaboration between Leonardo, the Franciscan priest and mathematician Fra Luca Pacioli, and the artist Piero della Francesca, although inexplicably it is Pacioli who appears as the sole author. In addition to explanatory text, the book offers sixty illustrations—including a variety of polyhedra and the design of the letters for a new font (Vitruvian letters). There is also one drawing that examines the proportions of the human face seen in profile. An equilateral triangle has been constructed with a vertex located at the base of the skull. The original drawings for the book are housed in the Biblioteca Ambrosiana in Milan (Figure 5.3).
The “official” author of De divina proportione, Pacioli, was a talented mathematician who is held in reverence in the field of accounting as the patriarch of the double-entry bookkeeping system. He had introduced the system in a treatise on mathematics in 1494, giving credit to “Leonardo of Pisa” (Fibonacci), who had introduced it three hundred years earlier in his Summa. Contemporary Islamic scholars, however, point out that the double-entry system had been known to medieval Islamic mathematicians. Although no documents have survived, this claim may be quite correct. It is certainly reminiscent of the applied mathematics and science known to have come down from medieval Islamic scholars.

Figure 5.3. A sampling of Leonardo’s illustrations from De divina proportione (Courtesy of the library of the National Gallery of Art)
There is in the foregoing a wider connection in mathematics, aesthetics, and science that draws the two Leonardos—da Vinci and Fibonacci—under the same intellectual umbrella. Ultimately, however, there is also the powerful image of intellectual tributaries rising much earlier: in ancient Egypt, India, Babylon, and classical Greece, but its full confluence not occurring until much later. Along with some remarkable discoveries there are also some fundamental mistakes, especially in natural philosophy, that had been perpetuated. Full reckoning was to come after the medieval times of the Islamic scholars, and even after the Renaissance. In the High Renaissance Leonardo da Vinci would first begin to challenge Aristotelian, Ptolemaic, and Galenic errors in natural philosophy and the entrenched misapprehensions of the Church (a topic of Chapter 10). In the sixteenth century the works of Copernicus and Vesalius would signal a turning of the tide in the prevailing intellectual order, the high tide appearing early in the seventeenth century with Galileo finding himself locked in struggle with the Church. Although Galileo would lose his battle, the process, moved to the new venue of northern Europe, would establish its own inexorable course and make unprecedented progress.