in which even the nuclear force,
supposedly the strongest of all forces,
cannot resist the crush of gravity
Zwicky
In the 1930s and 1940s, many of Fritz Zwicky’s colleagues regarded him as an irritating buffoon. Future generations of astronomers would look back on him as a creative genius.
“By the time I knew Fritz in 1933, he was thoroughly convinced that he had the inside track to ultimate knowledge, and that everyone else was wrong,” says William Fowler, then a student at Caltech (the California Institute of Technology) where Zwicky taught and did research. Jesse Greenstein, a Caltech colleague of Zwicky’s from the late 1940s onward, recalls Zwicky as “a self-proclaimed genius.¼ There’s no doubt that he had a mind which was quite extraordinary. But he was also, although he didn’t admit it, untutored and not self-controlled. ¼ He taught a course in physics for which admission was at his pleasure. If he thought that a person was sufficiently devoted to his ideas, that person could be admitted. ¼ He was very much alone [among the Caltech physics faculty, and was] not popular with the establishment. . . . His publications often included violent attacks on other people.”
Zwicky—a stocky, cocky man, always ready for a fight—did not hesitate to proclaim his inside track to ultimate knowledge, or to tout the revelations it brought. In lecture after lecture during the 1930s, and article after published article, he trumpeted the concept of a neutron star—a concept that he, Zwicky, had invented to explain the origins of the most energetic phenomena seen by astronomers: supernovae, and cosmic rays. He even went on the air in a nationally broadcast radio show to popularize his neutron stars. But under close scrutiny, his articles and lectures were unconvincing. They contained little substantiation for his ideas.
It was rumored that Robert Millikan (the man who had built Caltech into a powerhouse among science institutions), when asked in the midst of all this hoopla why he kept Zwicky at Caltech, replied that it just might turn out that some of Zwicky’s far-out ideas were right. Millikan, unlike some others in the science establishment, must have seen hints of Zwicky’s intuitive genius—a genius that became widely recognized only thirty-five years later, when observational astronomers discovered real neutron stars in the sky and verified some of Zwicky’s extravagant claims about them.
Fritz Zwicky among a gathering of scientists at Caltech in 1931. Also in the photograph are Richard Tolman (who will be an important figure later in this chapter), Robert Millikan, and Albert Einstein. [Courtesy of the Archives, California Institute of Technology.]
Among Zwicky’s claims, the most relevant to this book is the role of neutron stars as stellar corpses. As we shall see, a normal star that is too massive to die a white-dwarf death may die a neutron-star death instead. If all massive stars were to die that way, then the Universe would be saved from the most outrageous of hypothesized stellar corpses: black holes. With light stars becoming white dwarfs when they die, and heavy stars becoming neutron stars, there would be no way for nature to make a black hole. Einstein and Eddington, and most physicists and astronomers of their era, would heave a sigh of relief.
Zwicky had been lured to Caltech in 1925 by Millikan. Millikan expected him to do theoretical research on the quantum mechanical structures of atoms and crystals, but more and more during the late 1920s and early 1930s, Zwicky was drawn to astrophysics. It was hard not to be entranced by the astronomical Universe when one worked in Pasadena, the home not only of Caltech but also of the Mount Wilson Observatory, which had the world’s largest telescope, a reflector 2.5 meters (100 inches) in diameter.
In 1931 Zwicky latched on to Walter Baade, a new arrival at Mount Wilson from Hamburg and Göttingen and a superb observational astronomer. Baade and Zwicky shared a common cultural background: Baade was German, Zwicky was Swiss, and both spoke German as their native language. They also shared respect for each other’s brilliance. But there the commonality ended. Baade’s temperament was different from Zwicky’s. He was reserved, proud, hard to get to know, universally well informed—and tolerant of his colleagues’ peculiarities. Zwicky would test Baade’s tolerance during the coming years until finally, during World War II, he and Zwicky would split violently. “Zwicky called Baade a Nazi, which he wasn’t, and Baade said he was afraid that Zwicky would kill him. They became a dangerous pair to put in the same room,” recalls Jesse Greenstein.
During 1932 and 1933, Baade and Zwicky were often seen in Pasadena, animatedly conversing in German about stars called “novae,” which suddenly flare up and shine 10,000 times more brightly than before; and then, after about a month, slowly dim down to normalcy. Baade, with his encyclopedic knowledge of astronomy, was aware of tentative evidence that, in addition to these “ordinary” novae, there might also be unusual, rare, superluminous novae. Astronomers at first had not suspected that those novae were superluminous, since they appeared through telescopes to be roughly the same brightness as ordinary novae. However, they resided in peculiar nebulas (shining “clouds”); and in the 1920s, observations at Mount Wilson and elsewhere began to convince astronomers that those nebulas were not simply clouds of gas in our own Milky Way galaxy, as had been thought, but rather were galaxies in their own right-giant assemblages of nearly 1012 (a trillion) stars, far outside our own galaxy. The rare novae seen in these galaxies, being so much farther away than our own galaxy’s ordinary novae, would have to be intrinsically far more luminous than ordinary novae in order to have a similar brightness as seen from Earth.
Baade collected from the published literature all the observational data he could find about each of the six superluminous novae that astronomers had seen since the turn of the century. These data he combined with all the observational information he could get about the distances to the galaxies in which they lay, and from this combination he computed how much light the superluminous novae put out. His conclusion was startling: During flare-up these superluminous novae were typically 108 (100 million) times more luminous than our Sun! (Today we know, thanks largely to work in 1952 by Baade himself, that the distances were underestimated in the 1930s by nearly a factor of 10, and that correspondingly1 the superluminous novae are nearly 1010—10 billion—times more luminous than our Sun.)
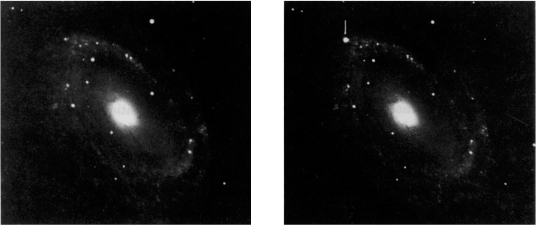
The galaxy NGC 4725 in the constellation Coma Berenices. .Left: As photographed on 10 May 1940, before a supernova outburst. Right: On 2 January 1941 during the supernova outburst. The white line points to the supernova, in the outer reaches of the galaxy. This galaxy is now known to be 30 million light-years from Earth and to contain 3 × 1011 (a third of a trillion) stars. [Courtesy California Institute of Technology.]
Zwicky, a lover of extremes, was fascinated by these superluminous novae. He and Baade discussed them endlessly and coined for them the name supernovae. Each supernova, they presumed (correctly), was produced by the explosion of a normal star. And the explosion was so hot, they suspected (this time incorrectly), that it radiated far more energy as ultraviolet light and X-rays than as ordinary light. Since the ultraviolet light and X-rays could not penetrate the Earth’s atmosphere, it was impossible to measure just how much energy they contained. However, one could try to estimate their energy from the spectrum of the observed light and the laws of physics that govern the hot gas in the exploding supernova.
By combining Baade’s knowledge of the observations and of ordinary novae with Zwicky’s understanding of theoretical physics, Baade and Zwicky concluded (incorrectly) that the ultraviolet radiation and X-rays from a supernova must carry at least 10,000 and perhaps 10 million times more energy even than the visible light. Zwicky, with his love of extremes, quickly assumed that the larger factor, 10 million, was correct and quoted it with enthusiasm.
This (incorrect) factor of 10 million meant that during the several days that the supernova was at its brightest, it put out an enormous amount of energy: roughly a hundred times more energy than our Sun will radiate in heat and light during its entire 10-billion-year lifetime. This is about as much energy as one would obtain if one could convert a tenth of the mass of our Sun into pure, luminous energy!
(Thanks to decades of subsequent observational studies of supernovae—many of them by Zwicky himself—we now know that the Baade-Zwicky estimate of a supernova’s energy was not far off the mark. However, we also know that their calculation of this energy was badly flawed: Almost all the outpouring energy is carried by particles called neutrinos and not by X-ray and ultraviolet radiations as they thought. Baade and Zwicky got the right answer purely by luck.)
What could be the origin of this enormous supernova energy? To explain it, Zwicky invented the neutron star.
Zwicky was interested in all branches of physics and astronomy, and he fancied himself a philosopher. He tried to link together all phenomena he encountered in what he later called a “morphological fashion.” In 1932, the most popular of all topics in physics or astronomy was nuclear physics, the study of the nuclei of atoms. From there, Zwicky extracted the key ingredient for his neutron-star idea: the concept of a neutron.
Since the neutron will be so important in this chapter and the next, I shall digress briefly from Zwicky and his neutron stars to describe the discovery of the neutron and the relationship of neutrons to the structure of atoms.
After formulating the “new” laws of quantum mechanics in 1926 (Chapter 4), physicists spent the next five years using those quantum mechanical laws to explore the realm of the small. They unraveled the mysteries of atoms (Box 5.1) and of materials such as molecules, metals, crystals, and white-dwarf matter, which are made from atoms. Then, in 1931, physicists turned their attention inward to the cores of atoms and the atomic nuclei that reside there.
The nature of the atomic nucleus was a great mystery. Most physicists thought it was made from a handful of electrons and twice as many protons, bound together in some as yet ill-understood way. However, Ernest Rutherford in Cambridge, England, had a different hypothesis: protons and neutrons. Now, protons were already known to exist. They had been studied in physics experiments for decades, and were known to be about 2000 times heavier than electrons and to have positive electric charges. Neutrons were unknown. Rutherford had to postulate the neutron’s existence in order to get the laws of quantum mechanics to explain the nucleus successfully. A successful explanation required three things: (1) Each neutron must have about the same mass as a proton but have no electric charge, (2) each nucleus must contain about the same number of neutrons as protons, and (3) all the neutrons and protons must be held together tightly in their tiny nucleus by a new type of force, neither electrical nor gravitational-a force called, naturally, the nuclear force. (It is now also called the strong force.) The neutrons and protons would protest their confinement in the nucleus by claustrophobic, erratic, high-speed motions; these motions would produce degeneracy pressure; and that pressure would counterbalance the nuclear force, holding the nucleus steady at its size of about 10−13 centimeter.
The Internal Structures of Atoms
An atom consists of a cloud of electrons surrounding a central, massive nucleus. The electron cloud is roughly 10−8 centimeter in size (about a millionth the diameter of a human hair), and the nucleus at its core is 100,000 times smaller, roughly 10−13 centimeter; see the diagram below. If the electron cloud were enlarged to the size of the Earth, then the nucleus would become the size of a football field. Despite its tiny size, the nucleus is several thousand times heavier than the tenuous electron cloud.
The negatively charged electrons are held in their cloud by the electrical pull of the positively charged nucleus, but they do not fall into the nucleus for the same reason as a white-dwarf star does not implode: A quantum mechanical law called the Pauli exclusion principle forbids more than two electrons to occupy the same region of space at the same time (two can do so if they have opposite “spins,” a subtlety ignored in Chapter 4). The cloud’s electrons therefore get paired together in cells called “orbitals.” Each pair of electrons, in protest against being confined to its small cell, undergoes erratic, high-speed “claustrophobic” motions, like those of electrons in a white-dwarf star (Chapter 4). These motions give rise to “electron degeneracy pressure,” which counteracts the electrical pull of the nucleus. Thus, one can think of the atom as a tiny white-dwarf star, with an electric force rather than a gravitational force pulling the electrons inward, and with electron degeneracy pressure pushing them outward.
The right-hand diagram below sketches the structure of the atomic nucleus, as discussed in the text; it is a tiny cluster of protons and neutrons, held together by the nuclear force.
In 1931 and early 1932, experimental physicists competed vigorously with each other to test this description of the nucleus. The method was to try to knock some of Rutherford’s postulated neutrons out of atomic nuclei by bombarding the nuclei with high-energy radiation. The competition was won in February 1932 by a member of Rutherford’s own experimental team, James Chadwick. Chadwick’s bombardment succeeded, neutrons emerged in profusion, and they had just the properties that Rutherford had postulated. The discovery was announced with fanfare by newspapers around the world, and naturally it caught Zwicky’s attention.
The neutron arrived on the scene in the same year as Baade and Zwicky were struggling to understand supernovae. This neutron was just what they needed, it seemed to Zwicky. Perhaps, he reasoned, the core of a normal star, with densities of, say, 100 grams per cubic centimeter, could be made to implode until it reached a density like that of an atomic nucleus, 1014 (100 trillion) grams per cubic centimeter; and perhaps the matter in that shrunken stellar core would then transform itself into a “gas” of neutrons—a “neutron star” Zwicky called it. If so, Zwicky computed (correctly in this case), the shrunken core’s intense gravity would bind it together so tightly that not only would its circumference have been reduced, but so would its mass. The stellar core’s mass would now be 10 percent lower than before the implosion. Where would that 10 percent of the core’s mass have gone? Into explosive energy, Zwicky reasoned (correctly again; see Figure 5.1 and Box 5.2).
If, as Zwicky believed (correctly), the mass of the shrunken stellar core is about the same as the mass of the Sun, then the 10 percent of it that is converted to explosive energy, when the core becomes a neutron star, would produce 1046 joules, which is close to the energy that Zwicky thought was needed to power a supernova. The explosive energy might heat the outer layers of the star to an enormous temperature and blast them off into interstellar space (Figure 5.1), and as the star exploded, its high temperature might make it shine brightly in just the manner of the supernovae that he and Baade had identified.
Zwicky did not know what might initiate the implosion of the star’s core and convert it into a neutron star, nor did he know how the core might behave as it imploded, and therefore he could not estimate how long the implosion would take (is it a slow contraction or a high-speed implosion?). (When the full details were ultimately worked out in the 1960s and later, the core turned out to implode violently; its own intense gravity drives it to implode from about the size of the Earth to 100 kilometers circumference in less than 10 seconds.) Zwicky also did not understand in detail how the energy from the core’s shrinkage might create a supernova explosion, or why the debris of the explosion would shine very brightly for a few days and remain quite bright for a few months, rather than a few seconds or hours or years. However, he knew-or he thought he knew-that the energy released by forming a neutron star was the right amount, and that was enough for him.
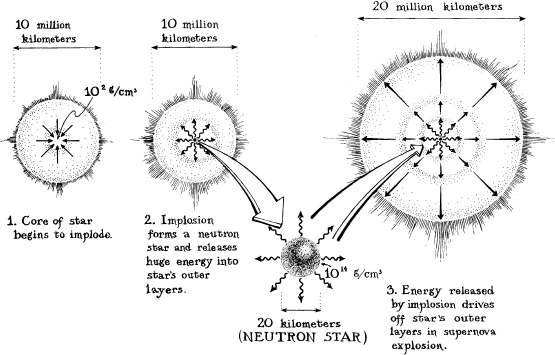
5.1 Fritz Zwicky’s hypothesis for triggering supernova explosions: The supernova’s explosive energy comes from the implosion of a star’s normal-density core to form a neutron star.
Box 5.2
The Equivalence of Mass and Energy
Mass, according to Einstein’s special relativity laws, is just a very compact form of energy. It is possible, though how is a nontrivial issue, to convert any mass, including that of a person, into explosive energy. The amount of energy that comes from such conversion is enormous. It is given by Einstein’s famous formula E =Mc2, where E is the explosive energy, M is the mass that gets converted to energy, and c =2.99792 × 108 meters per second is the speed of light. From the 75-kilogram mass of a typical person this formula predicts an explosive energy of 7 × 1018 joules, which is thirty times larger than the energy of the most powerful hydrogen bomb that has ever been exploded.
The conversion of mass into heat or into the kinetic energy of an explosion underlies Zwicky’s explanation for supernovae (Figure 5.1), the nuclear burning that keeps the Sun hot (later in this chapter), and nuclear explosions (next chapter).
Zwicky was not content with just explaining supernovae; he wanted to explain everything in the Universe. Among all the unexplained things, the one getting the most attention at Caltech in 1932–1933 was cosmic rays—high-speed particles that bombard the Earth from space. Caltech’s Robert Millikan was the world leader in the study of cosmic rays and had given them their name, and Caltech’s Carl Anderson had discovered that some of the cosmic-ray particles were made of antimatter.2 Zwicky, with his love of extremes, managed to convince himself (correctly it turns out) that most of the cosmic rays were coming from outside our solar system, and (incorrectly) that most were from outside our Milky Way galaxy—indeed, from the most distant reaches of the Universe—and he then convinced himself (roughly correctly) that the total energy carried by all the Universe’s cosmic rays was about the same as the total energy released by supernovae throughout the Universe. The conclusion was obvious to Zwicky (and perhaps correct3): Cosmic rays are made in supernova explosions.
It was late 1933 by the time Zwicky had convinced himself of these connections between supernovae, neutrons, and cosmic rays. Since Baade’s encyclopedic knowledge of observational astronomy had been a crucial foundation for these connections, and since many of Zwicky’s calculations and much of his reasoning had been carried out in verbal give-and-take with Baade, Zwicky and Baade agreed to present their work together at a meeting of the American Physical Society at Stanford University, an easy day’s drive up the coast from Pasadena. The abstract of their talk, published in the 15 January 1934 issue of the Physical Review, is shown in Figure 5.2. It is one of the most prescient documents in the history of physics and astronomy.
It asserts unequivocally the existence of supernovae as a distinct class of astronomical objects—although adequate data to prove firmly that they are different from ordinary novae would be produced by Baade and Zwicky only four years later, in 1938. It introduces for the first time the name “supernovae” for these objects. It estimates, correctly, the total energy released in a supernova. It suggests that cosmic rays are produced by supernovae—a hypothesis still thought plausible in 1993, but not firmly established (see Footnote 3). It invents the concept of a star made out of neutrons—a concept that would not become widely accepted as theoretically viable until 1939 and would not be verified observationally until 1968. It coins the name neutron star for this concept. And it suggests “with all reserve” (a phrase presumably inserted by the cautious Baade) that supernovae are produced by the transformation of ordinary stars into neutron stars—a suggestion that would be shown theoretically viable only in the early 1960s and would be confirmed by observation only in the late 1960s with the discovery of pulsars (spinning, magnetized neutron stars) inside the exploding gas of ancient supernovae.
5.2 Abstract of the talk on supernovae, neutron stars, and cosmic rays given by Walter Baade and Fritz Zwicky at Stanford University in December 1933.
Astronomers in the 1930s responded enthusiastically to the Baade—Zwicky concept of a supernova, but treated Zwicky’s neutron-star and cosmic-ray ideas with some disdain. “Too speculative” was the general consensus. “Based on unreliable calculations,” one might add, quite correctly. Nothing in Zwicky’s writings or talks provided more than meager hints of substantiation for the ideas. In fact, it is clear to me from a detailed study of Zwicky’s writings of the era that he did not understand the laws of physics well enough to be able to substantiate his ideas. I shall return to this later in the chapter.
Some concepts in science are so obvious in retrospect that it is amazing nobody noticed them sooner. Such was the case with the connection between neutron stars and black holes. Zwicky could have begun to make that connection in 1933, but he didn’t; it would get made in a tentative way six years later and definitively a quarter century later. The tortured route that finally rubbed physicists’ noses in the connection will occupy much of the rest of this chapter.
To appreciate the story of how physicists came to recognize the neutron-star/black-hole connection, it will help to know something about the connection in advance. Thus, the following digression:
What are the fates of stars when they die? Chapter 4 revealed a partial answer, an answer embodied in the right-hand portion of Figure 5.3 (which is the same as Figure 4.4). That answer depends on whether the star is less massive or more massive than 1.4 Suns (Chandrasekhar’s limiting mass).
If the star is less massive than the Chandrasekhar limit, for example if the star is the Sun itself, then at the end of its life it follows the path labeled “death of Sun” in Figure 5.3. As it radiates light into space, it gradually cools, losing its thermal (heat-induced) pressure. With its pressure reduced, it no longer can withstand the inward pull of its own gravity; its gravity forces it to shrink. As it shrinks, it moves leftward in Figure 5.3 toward smaller circumferences, while staying always at the same height in the figure because its mass is unchanging. (Notice that the figure plots mass up and circumference to the right.) And as it shrinks, the star squeezes the electrons in its interior into smaller and smaller cells, until finally the electrons protest with such strong degeneracy pressure that the star can shrink no more. The degeneracy pressure counteracts the inward pull of the star’s gravity, forcing the star to settle down into a white-dwarf grave on the boundary curve (white-dwarf curve) between the white region of Figure 5.3 and the shaded region. If the star were to shrink even more (that is, move leftward from the white-dwarf curve into the shaded region), its electron degeneracy pressure would grow stronger and make the star expand back to the white-dwarf curve. If the star were to expand into the white region, its electron degeneracy pressure would weaken, permitting gravity to shrink it back to the white-dwarf curve. Thus, the star has no choice but to remain forever on the white-dwarf curve, where gravity and pressure balance perfectly, gradually cooling and turning into a black dwarf—a cold, dark, solid body about the size of the Earth but with the mass of the Sun.
If the star is more massive than Chandrasekhar’s 1.4-solar-mass limit, for example if it is the star Sirius, then at the end of its life it will follow the path labeled “death of Sirius.” As it emits radiation and cools and shrinks, moving leftward on this path to a smaller and smaller circumference, its electrons get squeezed into smaller and smaller cells; they protest with a rising degeneracy pressure, but they protest in vain. Because of its large mass, the star’s gravity is strong enough to squelch all electron protest. The electrons can never produce enough degeneracy pressure to counterbalance the star’s gravity4; the star must, in Arthur Eddington’s words, “go on radiating and radiating and contracting and contracting, until, I suppose, it gets down to a few kilometers radius, when gravity becomes strong enough to hold in the radiation, and the star can at last find peace.”
Or that would be its fate, if not for neutron stars. If Zwicky was right that neutron stars can exist, then they must be rather analogous to white-dwarf stars, but with their internal pressure produced by neutrons instead of electrons. This means that there must be a neutron-star curve in Figure 5.3, analogous to the white-dwarf curve, but at circumferences (marked on the horizontal axis) of roughly a hundred kilometers, instead of tens of thousands of kilometers. On this neutron-star curve neutron pressure would balance gravity perfectly, so neutron stars could reside there forever.
Suppose that the neutron-star curve extends upward in Figure 5.3 to large masses; that is, suppose it has the shape labeled B in the figure. Then Sirius, when it dies, cannot create a black hole. Rather, Sirius will shrink until it hits the neutron-star curve, and then it can shrink no more. If it tries to shrink farther (that is, move to the left of the neutron-star curve into the shaded region), the neutrons inside it will protest against being squeezed; they will produce a large pressure (partly due to degeneracy, that is, “claustrophobia,” and partly due to the nuclear force); and the pressure will be large enough to overwhelm gravity and drive the star back outward. If the star tries to reexpand into the white region, the neutrons’ pressure will decline enough for gravity to take over and squeeze it back inward. Thus, Sirius will have no choice but to settle down onto the neutron-star curve and remain there forever, gradually cooling and becoming a solid, cold, black neutron star.
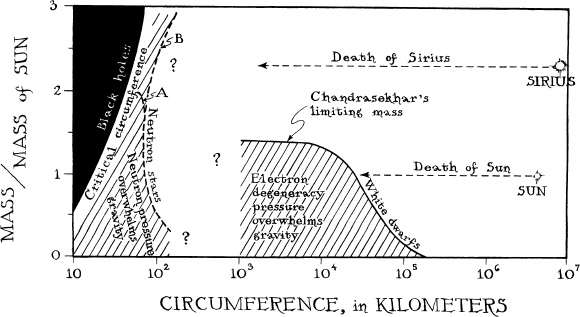
5.3 The ultimate fate of a star more massive than the Chandrasekhar limit of 1.4 Suns depends on how massive neutron stars can be. If they can be arbitrarily massive (curve B), then a star such as Sirius, when it dies, can only implode to form a neutron star; it cannot form a black hole. If there is an upper mass limit for neutron stars (as on curve A), then a massive dying star can become neither a white dwarf nor a neutron star; and unless there is some other graveyard available, it will die a black-hole death.
Suppose, instead, that the neutron-star curve does not extend upward in Figure 5.3 to large masses, but bends over in the manner of the hypothetical curve marked A. This will mean that there is a maximum mass that any neutron star can have, analogous to the Chandrasekhar limit of 1.4 Suns for white dwarfs. As for white dwarfs, so also for neutron stars, the existence of a maximum mass would herald a momentous fact: In a star more massive than the maximum, gravity will completely overwhelm the neutron pressure. Therefore, when so massive a star dies, it must either eject enough mass to bring it below the maximum, or else it will shrink inexorably, under gravity’s pull, right past the neutron-star curve, and then—if there are no other possible stellar graveyards, nothing but white dwarfs, neutron stars, and black holes-it will continue shrinking until it forms a black hole.
Thus, the central question, the question that holds the key to the ultimate fate of massive stars, is this: How massive can a neutron star be? If it can be very massive, more massive than any normal star, then black holes can never form in the real Universe. If there is a maximum possible mass for neutron stars, and that maximum is not too large, then black holes will form—unless there is yet another stellar graveyard, unsuspected in the 1930s.
This line of reasoning is so obvious in retrospect that it seems amazing that Zwicky did not pursue it, Chandrasekhar did not pursue it, Eddington did not pursue it. Had Zwicky tried to pursue it, however, he would not have got far; he understood too little nuclear physics and too little relativity to be able to discover whether the laws of physics place a mass limit on neutron stars or not. At Caltech there were, however, two others who did understand the physics well enough to deduce neutron-star masses: Richard Chace Tolman, a chemist turned physicist who had written a classic textbook called Relativity, Thermodynamics, and Cosmology; and J. Robert Oppenheimer, who would later lead the American effort to develop the atomic bomb.
Tolman and Oppenheimer, however, paid no attention at all to Zwicky’s neutron stars. They paid no attention, that is, until 1938, when the idea of a neutron star was published (under the slightly different name of neutron core) by somebody else, somebody whom, unlike Zwicky, they respected: Lev Davidovich Landau, in Moscow.
Landau
Landau’s publication on neutron cores was actually a cry for help: Stalin’s purges were in full swing in the U.S.S.R., and Landau was in danger. Landau hoped that by making a big splash in the newspapers with his neutron-core idea, he might protect himself from arrest and death. But of this, Tolman and Oppenheimer knew nothing.
Landau was in danger because of his past contacts with Western scientists:
Soon after the Russian revolution, science had been targeted for special attention by the new Communist leadership. Lenin himself had pushed a resolution through the Eighth Congress of the Bolshevik party in 1919 exempting scientists from requirements for ideological purity: “The Problem of industrial and economic development demands the immediate and widespread use of experts in science and technology whom we have inherited from capitalism, despite the fact that they inevitably are contaminated with bourgeois ideas and customs.” Of special concern to the leaders of Soviet science was the sorry state of Soviet theoretical physics, so, with the blessing of the Communist party and the government, the most brilliant and promising young theorists in the U.S.S.R. were brought to Leningrad (Saint Petersburg) for a few years of graduate study, and then, after completing the equivalent of a Ph.D., were sent to Western Europe for one or two years of postdoctoral study.
Why postdoctoral study? Because by the 1920s physics had become so complex that Ph.D.-level training was not sufficient for its mastery. To promote additional training worldwide, a system of postdoctoral fellowships had been set up, funded largely by the Rockefeller Foundation (profits from capitalists’ oil ventures). Anyone, even ardent Russian Marxists, could compete for these fellowships. The winners were called “postdoctoral fellows” or simply “postdocs.”
Why Western Europe for postdoctoral study? Because in the 1920s Western Europe was the mecca of theoretical physics; it was the home of almost every outstanding theoretical physicist in the world. Soviet leaders, in their desperation to transfuse theoretical physics from Western Europe to the U.S.S.R., had no choice but to send their young theorists there for training, despite the dangers of ideological contamination.
Of all the young Soviet theorists who traveled the route to Leningrad, then to Western Europe, and then back to the U.S.S.R., Lev Davidovich Landau would have by far the greatest influence on physics. Born in 1908 into a well-to-do Jewish family (his father was a petroleum engineer in Baku on the Caspian Sea), he entered Leningrad University at age sixteen and finished his undergraduate studies by age nineteen. After just two years of graduate study at the Leningrad Physicotechnical Institute, he completed the equivalent of a Ph.D. and went off to Western Europe, where he spent eighteen months of 1929–30 making the rounds of the great theoretical physics centers in Switzerland, Germany, Denmark, England, Belgium, and Holland.
Left: Lev Landau, as a student in Leningrad in the mid-1920s. Right: Landau, with fellow physics students George Gamow and Yevgenia Kanegiesser, horsing around in the midst of their studies in Leningrad, ca. 1927. In reality, Landau never played any musical instrument. [Left: courtesy AlP Emilio Segre Visual Archives, Margarethe Bohr Collection; right: courtesy Library of Congress.]
A fellow postdoctoral student in Zurich, German-born Rudolph Peierls, later wrote, “I vividly remember the great impression Landau made on all of us when he appeared in Wolfgang Pauli’s department in Zurich in 1929.¼ It did not take long to discover the depth of his understanding of modern physics, and his skill in solving basic problems. He rarely read a paper on theoretical physics in detail, but looked at it long enough to see whether the problem was interesting, and if so, what was the author’s approach. He then set to work to do the calculation himself, and if the answer agreed with the author’s he approved of the paper.” Peierls and Landau became the best of friends.
Tall, skinny, intensely critical of others as well as himself, Landau despaired that he had been born a few years too late. The golden age of physics, he thought, had been 1925–27 when de Broglie, Schrödinger, Heisenberg, Bohr, and others were creating the new quantum mechanics; if born earlier, he, Landau, could have been a participant. “All the nice girls have been snapped up and married, and all the nice problems have been solved. I don’t really like any of those that are left,” he said in a moment of despair in Berlin in 1929. But, in fact, explorations of the consequences of the laws of quantum mechanics and relativity were only beginning, and those consequences would hold wonderful surprises: the structure of the atomic nucleus, nuclear energy, black holes and their evaporation, superfluidity, superconductivity, transistors, lasers, and magnetic resonance imaging, to name only a few. And Landau, despite his pessimism, would become a central figure in the quest to discover these consequences.
Upon his return to Leningrad in 1931, Landau, who was an ardent Marxist and patriot, resolved to focus his career on transfusing modern theoretical physics into the Soviet Union. He succeeded enormously, as we shall see in later chapters.
Soon after Landau’s return, Stalin’s iron curtain descended, making further travel to the West almost impossible. As George Gamow, a Leningrad classmate of Landau’s, later recalled: “Russian science now had become one of the weapons for fighting the capitalistic world. Just as Hitler was dividing science and the arts into Jewish and Aryan camps, Stalin created the notion of capitalistic and proletarian science. It [was becoming] ¼ a crime for Russian scientists to ‘fraternize’ with scientists of the capitalistic countries.”
The political climate went from bad to horrid. In 1936 Stalin, having already killed 6 or 7 million peasants and kulaks (landowners) in his forced collectivization of agriculture, embarked on a several-year-long purge of the country’s political and intellectual leadership, a purge now called the Great Terror. The purge included execution of almost all members of Lenin’s original Politburo, and execution or forced disappearance, never to be seen again, of the top commanders of the Soviet army, fifty out of seventy-one members of the Central Committee of the Communist party, most of the ambassadors to foreign countries, and the prime ministers and chief officials of the non-Russian Soviet Republics. At lower levels roughly 7 million people were arrested and imprisoned and 2.5 million died—half of them intellectuals, including a large number of scientists and some entire research teams. Soviet biology, genetics, and agricultural sciences were destroyed.
In late 1937 Landau, by now a leader of theoretical physics research in Moscow, felt the heat of the purge nearing himself. In panic he searched for protection. One possible protection might be the focus of public attention on him as an eminent scientist, so he searched among his scientific ideas for one that might make a big splash in West and East alike. His choice was an idea that he had been mulling over since the early 1930s: the idea that “normal” stars like the Sun might possess neutron stars at their centers—neutron cores Landau called them.
The reasoning behind Landau’s idea was this: The Sun and other normal stars support themselves against the crush of their own gravity by means of thermal (heat-induced) pressure. As the Sun radiates heat and light into space, it must cool, contract, and die in about 30 million years’ time—unless it has some way to replenish the heat that it loses. Since there was compelling geological evidence, in the 1920s and 1930s, that the Earth had been kept at roughly constant temperature for 1 billion years or longer, the Sun must be replenishing its heat somehow. Arthur Eddington and others had suggested (correctly) in the 1920s that the new heat might come from nuclear reactions, in which one kind of atomic nucleus is transmuted into another—what is now called nuclear burning or nuclear fusion; see Box 5.3. However, the details of this nuclear burning had not been worked out sufficiently, by 1937, for physicists to know whether it could do the job. Landau’s neutron core provided an attractive alternative.
Just as Zwicky could imagine powering a supernova by the energy released when a normal star implodes to form a neutron star, so Landau could imagine powering the Sun and other normal stars by the energy released when its atoms, one by one, get captured onto a neutron core (Figure 5.4).
5.4 Lev Landau’s speculation as to the origin of the energy that keeps a normal star hot.
Nuclear Burning (Fusion) Contrasted with I Ordinary Burning
Ordinary burning is a chemical reaction. In chemical reactions, atoms get combined into molecules, where they share their electron clouds with each other; the electron clouds hold the molecules together. Nuclear burning is a nuclear reaction. In nuclear burning, atomic nuclei get fused together (nuclear fusion) to form more massive atomic nuclei; the nuclear force holds the more massive nuclei together.
The following diagram shows an example of ordinary burning: the burning of hydrogen to produce water (an explosively powerful form of burning that is used to power some rockets that lift payloads into space). Two hydrogen atoms combine with an oxygen atom to form a water molecule. In the water molecule, the hydrogen and oxygen atoms share their electron clouds with each other, but do not share their atomic nuclei.
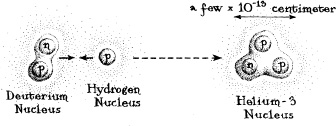
The following diagram shows an example of nuclear burning: the fusion of a deuterium (“heavy hydrogen”) nucleus and an ordinary hydrogen nucleus to form a helium-3 nucleus. This is one of the fusion reactions that is now known to power the Sun and other stars, and that powers hydrogen bombs (Chapter 6). The deuterium nucleus contains one neutron and one proton, bound together by the nuclear force; the hydrogen nucleus consists of a single proton; the helium-3 nucleus created by the fusion contains one neutron and two protons.
Capturing an atom onto a neutron core was much like dropping a rock onto a cement slab from a great height: Gravity pulls the rock down, accelerating it to high speed, and when it hits the slab, its huge kinetic energy (energy of motion) can shatter it into a thousand pieces. Similarly, gravity above a neutron core should accelerate infalling atoms to very high speeds, Landau reasoned. When such an atom plummets into the core, its shattering stop converts its huge kinetic energy (an amount equivalent to 10 percent of its mass) into heat. In this scenario, the ultimate source of the Sun’s heat is the intense gravity of its neutron core; and, as for Zwicky’s supernovae, the core’s gravity is 10 percent efficient at converting the mass of infalling atoms into heat.
The burning of nuclear fuel (Box 5.3), in contrast to capturing atoms onto a neutron core (Figure 5.4), can convert only a few tenths of 1 percent of the fuel’s mass into heat. In other words, Eddington’s heat source (nuclear energy) was roughly 30 times less powerful than Landau’s heat source (gravitational energy).5
Landau had actually developed a more primitive version of his neutron- core idea in 1931. However, the neutron had not yet been discovered then and atomic nuclei had been an enigma, so the capture of atoms onto the core in his 1931 model had released energy by a totally speculative process, one based on an (incorrect) suspicion that the laws of quantum mechanics might fail in atomic nuclei. Now that the neutron had been known for five years and the properties of atomic nuclei were beginning to be understood, Landau could make his idea much more precise and convincing. By presenting it to the world with a big splash of publicity, he might deflect the heat of Stalin’s purge.
In late 1937, Landau wrote a manuscript describing his neutron-core idea; to make sure it got maximum public attention, he took a series of unusual steps: He submitted it for publication, in Russian, to Doklady Akademii Nauk (Reports of the Academy of Sciences of the U.S.S.R, published in Moscow), and in parallel he mailed an English version to the same famous Western physicist as Chandrasekhar had appealed to, when Eddington attacked him (Chapter 4), Niels Bohr in Copenhagen. (Bohr, as an honorary member of the Academy of Sciences of the D.S.S.R., was more or less acceptable to Soviet authorities even during the Great Terror.) With his manuscript, Landau sent Bohr the following letter:
5 November 1937, Moscow
Dear Mr. Bohr!
I enclose an article about stellar energy, which I have written. If it makes physical sense to you, I ask that you submit it to Nature. If it is not too much trouble for you, I would be very glad to learn your opinion of this work.
With deepest thanks.
Yours, L. Landau
(Nature is a British scientific magazine that publishes, quickly, announcements of discoveries in all fields of science and that has one of the highest worldwide circulations among serious scientific journals.)
Landau had friends in high places-high enough to arrange that, as soon as word was received back that Bohr had approved his article and had submitted it to Nature, a telegram would be sent to Bohr by the editorial staff of Izvestia. (Izvestia was one of the two most influential newspapers in the U.S.S.R., a newspaper run by and in behalf of the Soviet government.) The telegram went out on 16 November 1937 saying:
Inform us, please, of your opinion of the work of Professor Landau. Telegraph to us, please, your brief conclusion.
Editorial Staff, Izvestia
Bohr, evidently a bit puzzled and worried by the request, replied from Copenhagen that same day:
The new idea of Professor Landau about neutron cores of massive stars is of the highest level of excellence and promise. I will be happy to send a short evaluation of it and of various other researches by Landau. Inform me please, more exactly, for what purpose my opinion is needed.
Bohr
The Izvestia staff responded that they wanted to publish Bohr’s evaluation in their newspaper. They did just that on 23 November, in an article that described Landau’s idea and praised it highly:
This work of Professor Landau’s has aroused great interest among Soviet physicists, and his bold idea gives new life to one of the most important processes in astrophysics. There is every reason to think that Landau’s new hypothesis will turn out to be correct and will lead to solutions to a whole series of unsolved problems in astrophysics.... Niels Bohr has given an extremely complimentary evaluation of the work of this Soviet scientist [Landau], saying that “The new idea of L. Landau is excellent and very promising.”
This campaign was not enough to save Landau. Early in the morning of 28 April 1938, the knock came on the door of his apartment, and he was taken away in an official black limousine as his wife-to-be Cora watched in shock from the apartment door. The fate that had befallen so many others was now also Landau’s.
The limousine took Landau to one of Moscow’s most notorious political prisons, the Butyrskaya. There he was told that his activities as a German spy had been discovered, and he was to pay the price for them. That the charges were ludicrous (Landau, a Jew and an ardent Marxist spying for Nazi Germany?) was irrelevant. The charges almost always were ludicrous. In Stalin’s Russia one rarely knew the real reason one had been imprisoned-though in Landau’s case, there are indications in recently revealed KGB files: In conversations with colleagues, he had criticized the Communist party and the Soviet government for their manner of organizing scientific research, and for the massive arrests of 1936–37 that ushered in the Great Terror. Such criticism was regarded as an “anti-Soviet activity” and could easily land one in prison.
Landau was lucky. His imprisonment lasted but one year, and he survived it—just barely. He was released in April 1939 after Pyotr Kapitsa, the most famous Soviet experimental physicist of the 1930s, appealed directly to Molotov and Stalin to let him go on grounds that Landau and only Landau, of all Soviet theoretical physicists, had the ability to solve the mystery of how superfluidity comes about.6 (Superfluidity had been discovered in Kapitsa’s laboratory, and independently by J. F. Allen and A. D. Misener in Cambridge, England, and if it could be explained by a Soviet scientist, this would demonstrate doubly to the world the power of Soviet science.)
Landau emerged from prison emaciated and extremely ill. In due course, he recovered physically and mentally, solved the mystery of superfluidity using the laws of quantum mechanics, and received the Nobel Prize for his solution. But his spirit was broken. Never again could he withstand even mild psychological pressure from the political authorities.
Oppenheimer
In California, Robert Oppenheimer was in the habit of reading with care every scientific article published by Landau. Thus, Landau’s article on neutron cores in the 19 February 1938 issue of Nature caught his immediate attention. Coming from Fritz Zwicky, the idea of a neutron star as the energizer for supernovae was—in Oppenheimer’s view—a far-out, flaky speculation. Coming from Lev Landau, a neutron core as the energizer for a normal star was worthy of serious thought. Might the Sun actually possess such a core? Oppenheimer vowed to find out.
Oppenheimer’s style of research was completely different from any encountered thus far in this book. Whereas Baade and Zwicky worked together as co-equal colleagues whose talents and knowledge complemented each other, and Chandrasekhar and Einstein each worked very much alone, Oppenheimer worked enthusiastically amidst a large entourage of students. Whereas Einstein had suffered when required to teach, Oppenheimer thrived on teaching.
Like Landau, Oppenheimer had gone to the mecca of theoretical physics, Western Europe, to get educated; and like Landau, Oppenheimer, upon returning home, had launched a transfusion of theoretical physics from Europe to his native land.
By the time of his return to America, Oppenheimer had acquired so tremendous a reputation that he received offers of faculty jobs from ten American universities including Harvard and Caltech, and from two in Europe. Among the offers was one from the University of California at Berkeley, which had no theoretical physics at all. “I visited Berkeley,” Oppenheimer recalled later, “and I thought I’d like to go there because it was a desert.” At Berkeley he could create something entirely his own. However, fearing the consequences of intellectual isolation, Oppenheimer accepted both the Berkeley offer and the Caltech offer. He would spend the autumn and winter in Berkeley, and the spring at Caltech. “1 kept the connection with Caltech.¼ it was a place where 1 would be checked if 1 got too far off base and where 1 would learn of things that might not be adequately reflected in the published literature.”
At first Oppenheimer, as a teacher, was too fast, too impatient, too overbearing with his students. He didn’t realize how little they knew; he couldn’t bring himself down to their level. His first lecture at Caltech in the spring of 1930 was a tour de force—powerful, elegant, insightful. When the lecture was over and the room had emptied, Richard Tolman, the chemist-turned-physicist who by now was a close friend, remained behind to bring him down to earth: “Well, Robert,” he said; “that was beautiful but 1 didn’t understand a damned word.”
However, Oppenheimer learned quickly. Within a year, graduate students and postdocs began flocking to Berkeley from all over America to learn physics from him, and within several years he had made Berkeley a more attractive place even than Europe for American theoretical physics postdocs.
One of Oppenheimer’s postdocs, Robert Serber, later described what it was like to work with him: “Oppie (as he was known to his Berkeley students) was quick, impatient, and had a sharp tongue, and in the earliest days of his teaching he was reputed to have terrorized the students. But after five years of experience he had mellowed (if his earlier students were to be believed). His course [on quantum mechanics] was an inspirational as well as an educational achievement. He transmitted to his students a feeling of the beauty of the logical structure of physics and an excitement about the development of physics. Almost everyone listened to the course more than once, and Oppie occasionally had difficulty in dissuading students from coming a third or fourth time.¼
“Oppie’s way of working with his research students was also original. His group consisted of 8 or 10 graduate students and about a half dozen postdoctoral fellows. He met the group once a day in his office. A little before the appointed time, the members straggled in and disposed themselves on the tables and about the walls. Oppie came in and discussed with one after another the status of the student’s research problem while the others listened and offered comments. All were exposed to a broad range of topics. Oppenheimer was interested in everything; one subject after another was introduced and coexisted with all the others. In an afternoon they might discuss electrodynamics, cosmic rays, astrophysics and nuclear physics.”
Each spring Oppenheimer piled books and papers into his convertible and several students into the rumble seat, and drove down to Pasadena. “We thought nothing of giving up our houses or apartments in Berkeley,” said Serber, “confident that we could find a garden cottage in Pasadena for twenty-five dollars a month.”
For each problem that interested him, Oppenheimer would select a student or postdoc to work out the details. For Landau’s problem, the question of whether a neutron core could keep the Sun hot, he selected Serber.
Robert Serber (left) and Robert Oppenheimer (right) discussing physics, ca. 1942. [Courtesy D.S. Information Agency.]
Oppenheimer and Serber quickly realized that, if the Sun has a neutron core at its center, and if the core’s mass is a large fraction of the Sun’s mass, then the core’s intense gravity will hold the Sun’s outer layers in a tight grip, making the Sun’s circumference far smaller than it actually is. Thus, Landau’s neutron-core idea could work only if neutron cores can be far less massive than the Sun.
“How small can the mass of a neutron core be?” Oppenheimer and Serber were thus driven to ask themselves. “What is the minimum possible mass for a neutron core?” Notice that this is the opposite question to the one that is crucial for the existence of black holes; to learn whether black holes can form, one needs to know the maximum possible mass for a neutron star (Figure 5.3 above). Oppenheimer as yet had no inkling of the importance of the maximum-mass question, but he now knew that the minimum neutron-core mass was central to Landau’s idea.
In his article Landau, also aware of the importance of the minimum neutron-core mass, had used the laws of physics to estimate it. With care Oppenheimer and Serber scrutinized Landau’s estimate. Yes, they found, Landau had properly taken account of the attractive forces of gravity inside and near the core. And yes, he had properly taken account of the degeneracy pressure of the core’s neutrons (the pressure produced by the neutrons’ claustrophobic motions when they get squeezed into tiny cells). But no, he had not taken proper account of the nuclear force that neutrons exert on each other. That force was not yet fully understood. However, enough was understood for Oppenheimer and Serber to conclude that probably, not absolutely definitely, but probably, no neutron core can ever be lighter than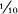 
of a solar mass. If nature ever succeeded in creating a neutron core lighter than this, its gravity would be too weak to hold it together; its pressure would make it explode.
At first sight this did not rule out the Sun’s possessing a neutron core; after all, a 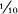-solar-mass core, which was allowed by Oppenheimer and Serber’s estimates, might be small enough to hide inside the Sun without affecting its surface properties very much (without affecting the things we see). But further calculations, balancing the pull of the core’s gravity against the pressure of surrounding gas, showed that the core’s effects could not be hidden: Around the core there would be a shell of white-dwarf-type matter weighing nearly a full solar mass, and with only a tiny amount of normal gas outside that shell, the Sun could not look at all like we see it. Thus, the Sun could not possess a neutron core, and the energy to keep the Sun hot must come from somewhere else.
Where else? At the same time as Oppenheimer and Serber in Berkeley were doing these calculations, Hans Bethe at Cornell University in Ithaca, New York, and Charles Critchfield at George Washington University in Washington, D.C., were using the newly developed laws of nuclear physics to demonstrate in detail that nuclear burning (the fusion of atomic nuclei; Box 5.3) can keep the Sun and other stars hot. Eddington had been right and Landau had been wrong—at least for the Sun and most other stars. (As of the early 1990s, it appears that a few giant stars might, in fact, use Landau’s mechanism.)
Oppenheimer and Serber had no idea that Landau’s paper was a desperate attempt to avoid prison and possible death, so on 1 September 1938, as Landau languished in Butyrskaya Prison, they submitted their critique of him to the Physical Review. Since Landau was a great enough physicist to take the heat, they said quite frankly: “An estimate of Landau . . . led to the value 0.001 solar masses for the limiting [minimum] mass [of a neutron core]. This figure appears to be wrong. . . . [Nuclear forces] of the often assumed spin exchange type preclude the existence of a [neutron] core for stars with masses comparable to that of the Sun.”
Landau’s neutron cores and Zwicky’s neutron stars are really the same thing. A neutron core is nothing but a neutron star that happens, somehow, to find itself inside a normal star. To Oppenheimer this must have been clear, and now that he had begun to think about neutron stars, he was drawn inexorably to the issue that Zwicky should have tackled but could not: What, precisely, is the fate of massive stars when they exhaust the nuclear fuel that, according to Bethe and Critchfield, keeps them hot? Which corpses will they create: white dwarfs? neutron stars? black holes? others?
Chandrasekhar’s calculations had shown unequivocally that stars less massive than 1.4 Suns must become white dwarfs. Zwicky was speculating loudly that at least some stars more massive than 1.4 Suns will implode to form neutron stars, and in the process generate supernovae. Might Zwicky be right? And will all massive stars die this way, thus saving the Universe from black holes?
One of Oppenheimer’s great strengths as a theorist was an unerring ability to look at a complicated problem and strip away the complications until he found the central issue that controlled it. Several years later, this talent would contribute to Oppenheimer’s brilliance as the leader of the American atomic bomb project. Now, in his struggle to understand stellar death, it told him to ignore all the complications that Zwicky was trumpeting about—the details of the stellar implosion, the transformation of normal matter into neutron matter, the release of enormous energy and its possible powering of supernovae and cosmic rays. All this was irrelevant to the issue of the star’s final fate. The only relevant thing was the maximum mass that a neutron star can have. If neutron stars can be arbitrarily massive (curve B in Figure 5.3 above), then black holes can never form. If there is a maximum possible neutron-star mass (curve A in Figure 5.3), then a star heavier than that maximum, when it dies, might form a black hole.
Having posed this maximum-mass question with stark clarity, Oppenheimer went about solving it, methodically and unequivocally—and, as was his standard practice, in collaboration with a student, in this case a young man named George Volkoff. The tale of Oppenheimer and Volkoff’s quest to learn the masses of neutron stars, and the central contributions of Oppenheimer’s Caltech friend Richard Tolman, is told in Box 5.4. It is a tale that illustrates Oppenheimer’s mode of research and several of the strategies by which physicists operate, when they understand clearly some of the laws that govern the phenomenon they are studying, but not all: In this case Oppenheimer understood the laws of quantum mechanics and general relativity, but neither he nor anyone else understood the nuclear force very well.
Despite their poor knowledge of the nuclear force, Oppenheimer and Volkoff were able to show unequivocally (Box 5.4) that there is a maximum mass for neutron stars, and it lies between about half a solar mass and several solar masses.
In the 1990s, after fifty years of additional study, we know that Oppenheimer and Volkoff were correct; neutron stars do, indeed, have a maximum allowed mass, and it is now known to lie between 1.5 and 3 solar masses, roughly the same ballpark as their estimate. Moreover, since 1967 hundreds of neutron stars have been observed by astronomers, and the masses of several have been measured with high accuracy. The measured masses are all close to 1.4 Suns; why, we do not know.
The Tale of Oppenheimer, Volkoff, and Tolman: AQuest for Neutron-Star Masses
When embarking on a complicated analysis, it is helpful to get one’s bearings by beginning with a rough, “order-of-magnitude” calculation, a calculation accurate only to within a factor of, say, 10. In keeping with this rule of thumb, Oppenheimer began his assault on the issue of whether neutron stars can have a maximum mass by a crude calculation, just a few pages long. The result was intriguing: He found a maximum mass of 6 Suns for any neutron star. If a detailed calculation gave the same result, then Oppenheimer could conclude that black holes might form when stars heavier than 6 Suns die.
A “detailed calculation” meant selecting a mass for a hypothetical neutron star, then asking whether, for that mass, neutron pressure inside the star can balance gravity. If the balance can be achieved, then neutron stars can have that mass. It would be necessary to choose one mass after another, and for each ask about the balance between pressure and gravity. This enterprise is harder than it might sound, because pressure and gravity must balance each other everywhere inside the star. However, it was an enterprise that had been pursued once before, by Chandrasekhar, in his analysis of white dwarfs (the analysis performed using Arthur Eddington’s Braunschweiger calculator, with Eddington looking over Chandrasekhar’s shoulder; Chapter 4).
Oppenheimer could pattern his neutron-star calculations after Chandrasekhar’s white-dwarf calculations, but only after making two crucial changes: First, in a white dwarf the pressure is produced by electrons, and in a neutron star by neutrons, so the equation of state (the relation between pressure and density) will be different. Second, in a white dwarf, gravity is weak enough that it can be described equally well by Newton’s laws or by Einstein’s general relativity; the two descriptions will give almost precisely the same predictions, so Chandrasekhar chose the simpler description, Newton’s. By contrast, in a neutron star, with its much smaller circumference, gravity is so strong that using Newton’s laws might cause serious errors, so Oppenheimer would have to describe gravity by Einstein’s general relativistic laws.* Aside from these two changes—a new equation of state (neutron pressure instead of electron) and a new description of gravity (Einstein’s instead of Newton’s)—Oppenheimer’s calculation would be the same as Chandrasekhar’s.
Having gotten this far, Oppenheimer was ready to turn the details of the calculation over to a student. He chose George Volkoff, a young man from Vancouver, who had emigrated from Russia in 1924.
Oppenheimer explained the problem to Volkoff and told him that the mathematical description of gravity that he would need was in a textbook that Richard Tolman had written, Relativity, Thermodynamics, and Cosmology. The equation of state for the neutron pressure, however, was a more difficult issue, since the pressure would be influenced by the nuclear force (with which neutrons push and pull on each other). Although the nuclear force was becoming well understood at the densities inside atomic nuclei, it was very poorly understood at the ten times higher higher densities that neutrons would face deep inside a massive neutron star. Physicists did not even know whether the nuclear force was attractive at these densities or repulsive (whether neutrons pulled on each other or pushed), and thus there was no way to know whether the nuclear force reduced the neutrons’ pressure or increased it. But Oppenheimer had a strategy to deal with these unknowns.
Pretend, at first, that the nuclear force doesn’t exist, Oppenheimer suggested to Volkoff. Then all the pressure will be of a sort that is well understood; it will be neutron degeneracy pressure (pressure produced by the neutrons’ “claustrophobic” motions). Balance this neutron degeneracy pressure against gravity, and from the balance, calculate the structures and masses that neutron stars would have in a universe without any nuclear force. Then, afterward, try to estimate how the stars’ structures and masses will change if, in our real Universe, the nuclear force behaves in this, that, or some other way.
With such well-posed instructions it was hard to miss. It took only a few days for Volkoff, guided by daily discussions with Oppenheimer and by Tolman’s book, to derive the general relativistic description of gravity inside a neutron star. And it took only a few days for him to translate the well-known equation of state for degenerate electron pressure into one for degenerate neutron pressure. By balancing the pressure against the gravity, Volkoff obtained a complicated differential equation whose solution would tell him the star’s internal structure. Then he was stymied. Try as he might, Volkoff could not solve his differential equation to get a formula for the star’s structure; so, like Chandrasekhar with white dwarfs, he was forced to solve his equation numerically. Just as Chandrasekhar had spent many days in 1934 punching the buttons of Eddington’s Braunschweiger calculator to compute the analogous white-dwarf structure, so Volkoff labored through much of November and December 1938, punching the buttons of a Marchant calculator.
While Volkoff punched buttons in Berkeley, Richard Tolman in Pasadena was taking a different tack: He strongly preferred to express the stellar structure in terms of formulas instead of just numbers off a calculator. A single formula can embody all the information contained in many many tables of numbers. If he could get the right formula, it would contain simultaneously the structures of stars of 1 solar mass, 2 solar masses, 5 solar masses—any mass at all. But even with his brilliant mathematical skills, Tolman was unable to solve Volkoff’s equation in terms of formulas.
“On the other hand,” Tolman presumably argued to himself, “we know that the correct equation of state is not really the one Volkoff is using. Volkoff has ignored the nuclear force; and since we don’t know the details of that force at high densities, we don’t know the correct equation of state. So let me ask a different question from Volkoff. Let me ask how the masses of neutron stars depend on the equation of state. Let me pretend that the equation of state is very ‘stiff,’ that is, that it gives exceptionally high pressures, and let me ask what the neutron-star masses would be in that case. And then let me pretend the equation of state is very ‘soft,’ that is, that it gives exceptionally low pressures, and ask what then would be the neutron-star masses. In each case, I will adjust the hypothetical equation of state into a form for which I can solve Volkoff’s differential equation in formulas. Though the equation of state I use will almost certainly not be the right one, my calculation will still give me a general idea of what the neutron-star masses might be if nature happens to choose a stiff equation of state, and what they might be if nature chooses a soft one.”
On 19 October, Tolman sent a long letter to Oppenheimer describing some of the stellar-structure formulas and neutron-star masses he had derived for several hypothetical equations of state. A week or so later, Oppenheimer drove down to Pasadena to spend a few days talking with Tolman about the project. On 9 November, Tolman wrote Oppenheimer another long letter, with more formulas. In the meantime, Volkoff was punching away on his Marchant buttons. In early December, Volkoff finished. He had numerical models for neutron stars with masses 0.3, 0.6, and 0.7 solar mass; and he had found that, if there were no nuclear force in our Universe, then neutron stars would always be less massive than 0.7 solar mass.
What a surprise! Oppenheimer’s crude estimate, before Volkoff started computing, had been a maximum mass of 6 solar masses. To protect massive stars against forming black holes, the careful calculation would have had to push that maximum mass up to a hundred Suns or more. Instead, it pushed the mass down—way down, to 0.7 solar mass.
Tolman came up to Berkeley to learn more of the details. Fifty years later Volkoff recalled the scene with pleasure: “I remember being greatly overawed by having to explain to Oppenheimer and Tolman what I had done. We were sitting out on the lawn of the old faculty club at Berkeley. Amidst the nice green grass and tall trees, here were these two venerated gentlemen and here was I, a graduate student just completing my Ph.D., explaining my calculations.”
Now that they knew the masses of neutron stars in an idealized universe with no nuclear force, Oppenheimer and Volkoff were ready to estimate the influence of the nuclear force. Here the formulas that Tolman had worked out so carefully for various hypothetical equations of state were helpful. From Tolman’s formulas one could see roughly how the star’s structure would change if the nuclear force was repulsive and thereby made the equation of state more “stiff” than the one Volkoff had used, and the change if it was attractive and thereby made the equation of state more “soft.” Within the range of believable nuclear forces, those changes were not great. There must still be a maximum mass for neutron stars, Tolman, Oppenheimer, and Volkoff concluded, and it must lie somewhere between about a half solar mass and several solar masses.
*See the discussion in the last section of Chapter 1 (“The Nature of Physical Law”) of the relationship between different descriptions of the laws of physics and their domains of validity.
Oppenheimer and Volkoffs conclusion cannot have been pleasing to people like Eddington and Einstein, who found black holes anathema. If Chandrasekhar was to be believed (as, in 1938, most astronomers were coming to understand he should), and if Oppenheimer and Volkoff were to be believed (and it was hard to refute them), then neither the white-dwarf graveyard nor the neutron-star graveyard could inter massive stars. Was there any conceivable way at all, then, for massive stars to avoid a black-hole death? Yes; two ways.
First, all massive stars might eject so much matter as they age (for example, by blowing strong winds off their surfaces or by nuclear explosions) that they reduce themselves below 1.4 solar masses and enter the white-dwarf graveyard, or (if one believed Zwicky’s mechanism for supernovae, which few people did) they might eject so much matter in supernova explosions that they reduce themselves below about 1 solar mass during the explosion and wind up in the neutron-star graveyard. Most astronomers, through the 1940s and 1950s, and into the early 1960s–if they thought at all about the issue–espoused this view.
Second, besides the white-dwarf, neutron-star, and black-hole graveyards, there might be a fourth graveyard for massive stars, a graveyard unknown in the 1930s. For example, one could imagine a graveyard in Figure 5.3 at circumferences intermediate between neutron stars and white dwarfs—a few hundred or a thousand kilometers. The shrinkage of a massive star might be halted in such a graveyard before the star ever gets small enough to form either a neutron star or a black hole.
If World War II and then the cold war had not intervened, Oppenheimer and his students, or others, would likely have explored such a possibility in the 1940s and would have showed firmly that there is no such fourth graveyard.
However, World War II did intervene, and it absorbed the energies of almost all the world’s theoretical physicists; then after the war, crash programs to develop hydrogen bombs delayed further the return of physicists to normalcy (see the next chapter).
Finally, in the mid-1950s, two physicists emerged from their respective hydrogen bomb efforts and took up where Oppenheimer and his students had left off. They were John Archibald Wheeler at Princeton University in the United States and Yakov Borisovich Zel’dovich at the Institute of Applied Mathematics in Moscow—two superb physicists, who will be major figures in the rest of this book.
Wheeler
In March 1956, Wheeler devoted several days to studying the articles by Chandrasekhar, Landau, and Oppenheimer and Volkoff. Here, he recognized, was a mystery worth probing. Could it really be true that stars more massive than about 1.4 Suns have no choice, when they die, but to form black holes? “Of all the implications of general relativity for the structure and evolution of the Universe, this question of the fate of great masses of matter is one of the most challenging,” Wheeler wrote soon thereafter; and he set out to complete the exploration of stellar graveyards that Chandrasekhar and Oppenheimer and Volkoff had begun.
To make his task very precise, Wheeler formulated a careful characterization of the kind of matter from which cold, dead stars should be made: He called it matter at the endpoint of thermonuclear evolution, since the word thermonuclear had become popular for the fusion reactions that power nuclear burning in stars and also power the hydrogen bomb. Such matter would be absolutely cold, and it would have burned its nuclear fuel completely; there would be no way, by any kind of nuclear reaction, to extract any more energy from the matter’s nuclei. For this reason, the nickname cold, dead matter will be used in this book instead of “matter at the endpoint of thermonuclear evolution.”
Wheeler set himself the goal to understand all objects that can be made from cold, dead matter. These would include small objects like balls of iron, heavier objects such as cold, dead planets made of iron, and still heavier objects: white dwarfs, neutron stars, and whatever other kinds of cold, dead objects the laws of physics allow. Wheeler wanted a comprehensive catalog of cold, dead things.
John Archibald Wheeler, ca. 1954. [Photo by Blackstone-Shelburne, New York City; courtesy J. A. Wheeler.]
Wheeler worked in much the same mode as had Oppenheimer, with an entourage of students and postdocs. From among them he selected B. Kent Harrison, a serious-minded Mormon from Utah, to work out the details of the equation of state for cold, dead matter. This equation of state would describe the details of how the pressure of such matter rises as one gradually compresses it to higher and higher density—or, equivalently, how its resistance to compression changes as its density Increases.
Wheeler was superbly prepared to give Harrison guidance in computing the equation of state for cold, dead matter, since he was among the world’s greatest experts on the laws of physics that govern the structure of matter: the laws of quantum mechanics and nuclear physics. During the preceding twenty years, he had developed powerful mathematical models to describe how atomic nuclei behave; with Niels Bohr he had developed the laws of nuclear fission (the splitting apart of heavy atomic nuclei such as uranium and plutonium, the principle underlying the atomic bomb); and he had been the leader of a team that designed the American hydrogen bomb (Chapter 6). Drawing on this expertise, Wheeler guided Harrison through the intricacies of the analysis.
The result of their analysis, the equation of state for cold, dead matter, is depicted and discussed in Box 5.5. At the densities of white dwarfs, it was the same equation of state as Chandrasekhar had used in his white-dwarf studies (Chapter 4); at neutron-star densities, it was the same as Oppenheimer and Volkoff had used (Box 5.4); at densities below white dwarfs and between white dwarfs and neutron stars, it was completely new.
With this equation of state for cold, dead matter in hand, John Wheeler asked Masami Wakano, a postdoc from Japan, to do with it what Volkoff had done for neutron stars and Chandrasekhar for white dwarfs: Combine the equation of state with the general relativistic equation describing the balance of gravity and pressure inside a star, and from that combination deduce a differential equation describing the star’s structure; then solve the differential equation numerically. The numerical calculations would produce the details of the internal structures of all cold, dead stars and, most important, the stars’ masses.
The calculations for the structure of a single star (the distribution of density, pressure, and gravity inside the star) had required Chandrasekhar and Volkoff many days of effort as they punched the buttons of their calculators in Cambridge and Berkeley in the 1930s. Wakano in Princeton in the 1950s, by contrast, had at his disposal one of the world’s first digital computers, the MANIAC—a room full of vacuum tubes and wires that had been constructed at the Princeton Institute for Advanced Study for use in the design of the hydrogen bomb. With the MANIAC, Wakano could crunch out the structure of each star in less than an hour.
Box 5.5
The Harrison–Wheeler Equation of State for Cold, Dead Matter
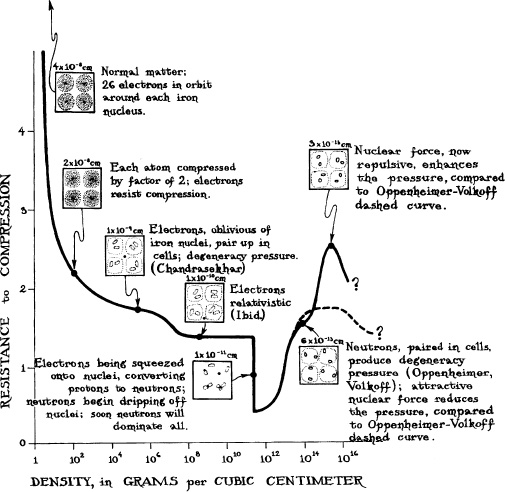
The drawing above depicts the Harrison–Wheeler equation of state. Plotted horizontally is the matter’s density. Plotted vertically is its resistance to compression (or adiabatic index, as physicists like to call it)—the percentage increase in pressure that accompanies a 1 percent increase in density. The boxes attached to the curve show what is happening to the matter, microscopically, as it is compressed from low densities to high. The size of each box, in centimeters, is written along the box’s top.
At normal densities (left edge of the figure), cold, dead matter is composed of iron. If the matter’s atomic nuclei were heavier than iron, energy could be released by splitting them apart to make iron (nuclear fission, as in an atomic bomb). If its nuclei were lighter than iron, energy could be released by joining them together to make iron (nuclear fusion, as in a hydrogen bomb). Once in the form of iron, the matter can release no more nuclear energy by any means whatsoever. The nuclear force holds neutrons and protons together more tightly when they form iron nuclei than when they form any other kind of atomic nucleus.
As the iron is squeezed from its normal density of 7.6 grams per cubic centimeter up toward 100, then 1000 grams per cubic centimeter, the iron resists by the same means as a rock resists compression: The electrons of each atom protest with “claustrophobic” (degeneracy-like) motions against being squeezed by the electrons of adjacent atoms. The resistance at first is huge not because the repulsive forces are especially strong, but rather because the starting pressure, at low density, is very low. (Recall that the resistance is the percentage increase in pressure that accompanies a 1 percent increase in density. When the pressure is low, a strong increase in pressure represents a huge percentage increase and thus a huge “resistance.” Later, at higher densities where the pressure has grown large, a strong pressure increase represents a much more modest percentage increase and thus a more modest resistance.)
At first, as the cold matter is compressed, the electrons congregate tightly around their iron nuclei, forming electron clouds made of electron orbitals. (There are actually two electrons, not one, in each orbital—a subtlety overlooked in Chapter 4 but discussed briefly in Box 5.1.) As the compression proceeds, each orbital and its two electrons are gradually confined into a smaller and smaller cell of space; the claustrophobic electrons protest this confinement by becoming more wave-like and developing higher-speed, erratic, claustrophobic motions (“degeneracy motions”; see Chapter 4). When the density has reached 105 (100,000) grams per cubic centimeter, the electrons’ degeneracy motions and the degeneracy pressure they produce have become so large that they completely overwhelm the electric forces with which the nuclei pull on the electrons. The electrons no longer congregate around the iron nuclei; they completely ignore the nuclei. The cold, dead matter, which began as a lump of iron, has now become the kind of stuff of which white dwarfs are made, and the equation of state has become the one that Chandrasekhar, Anderson, and Stoner computed in the early 1930s (Figure 4.3): a resistance of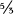 
, and then a smooth switch to 
at a density of about 107 grams per cubic centimeter when the erratic speeds of the electrons near the speed of light.
The transition from white-dwarf matter to neutron-star matter begins at a density of 4 × 1011 grams per cubic centimeter, according to the Harrison–Wheeler calculations. The calculations show several phases to the transition: In the first phase, the electrons begin to be squeezed into the atomic nuclei, and the nuclei’s protons swallow them to form neutrons. The matter, having thereby lost some of its pressure-sustaining electrons, suddenly becomes much less resistant to compression; this causes the sharp cliff in the equation of state (see diagram above). As this first phase proceeds and the resistance plunges, the atomic nuclei become more and more bloated with neutrons, thereby triggering the second phase: Neutrons begin to drip out of (get squeezed out of) the nuclei and into the space between them, alongside the few remaining electrons. These dripped-out neutrons, like the electrons, protest the continuing squeeze with a degeneracy pressure of their own. This neutron degeneracy pressure terminates the over-the-cliff plunge in the equation of state; the resistance to compression recovers and starts rising. In the third phase, at densities between about 1012 and 4 × 1012 grams per cubic centimeter, each neutron-bloated nucleus completely disintegrates, that is, breaks up into individual neutrons, forming the neutron gas studied by Oppenheimer and Volkoff, plus a tiny smattering of electrons and protons. From there on upward in density, the equation of state takes on the Oppenheimer–Volkoff neutron-star form (dashed curve in the diagram when nuclear forces are ignored; solid curve using the best 1990s understanding of the influence of nuclear forces).
The results of Wakano’s calculations are shown in Figure 5.5. This figure is the firm and final catalog of cold, dead objects; it answers all the questions we raised, early in this chapter, in our discussion of Figure 5.3.
In Figure 5.5, the circumference of a star is plotted rightward and its mass upward. Any star with circumference and mass in the white region of the figure has a stronger internal gravity than its pressure, so its gravity makes the star shrink leftward in the diagram. Any star in the shaded region has a stronger pressure than gravity, so its pressure makes the star expand rightward in the diagram. Only along the boundary of white and shaded do gravity and pressure balance each other perfectly; thus, the boundary curve is the curve of cold, dead stars that are in pressure/gravity equilibrium.
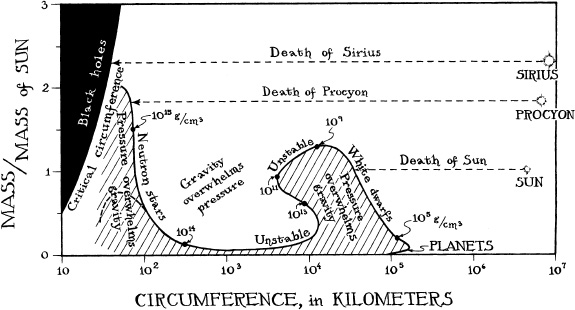
5.5 The circumferences (plotted horizontally), masses (plotted vertically), and central densities (labeled on curve) for cold, dead stars, as computed by Masami Wakano under the direction of John Wheeler, using the equation of state of Box 5.5. At central densities above those of an atomic nucleus (above 2 x 1014 grams per cubic centimeter), the solid curve is a modern, 1990s, one that takes proper account of the nuclear force, and the dashed curve is that of Oppenheimer and Volkoff without nuclear forces.
As one moves along this equilibrium curve, one is tracing out dead “stars” of higher and higher densities. At the lowest densities (along the bottom edge of the figure and largely hidden from view), these “stars” are not stars at all; rather, they are cold planets made of iron. (When Jupiter ultimately exhausts its internal supply of radioactive heat and cools off, although it is made mostly of hydrogen rather than iron, it will nevertheless lie near the rightmost point on the equilibrium curve.) At higher densities than the planets are Chandrasekhar’s white dwarfs.
When one reaches the topmost point on the white-dwarf part of the curve (the white dwarf with Chandrasekhar’s maximum mass of 1.4 Suns7 ) and then moves on to still higher densities, one meets cold, dead stars that cannot exist in nature because they are unstable against implosion or explosion (Box 5.6). As one moves from white-dwarf densities toward neutron-star densities, the masses of these unstable equilibrium stars decrease until they reach a minimum of about 0.1 solar mass at a circumference of 1000 kilometers and a central density of 3 × 1013 grams per cubic centimeter. This is the first of the neutron stars; it is the “neutron core” that Oppenheimer and Serber studied and showed cannot possibly be as light as the 0.001 solar mass that Landau wanted for a core inside the Sun.
Unstable Inhabitants of the Gap between White Dwarfs and Neutron Stars
Along the equilibrium curve in Figure 5.5, all the stars between the white dwarfs and the neutron stars are unstable. An example is the star with central density 1013 grams per cubic centimeter, whose mass and circumference are those of the point in Figure 5.5 marked 1013. At the 1013 point this star is in equilibrium; its gravity and pressure balance each other perfectly. However, the star is as unstable as a pencil standing on its tip.
If some tiny random force (for example, the fall of interstellar gas onto the star) squeezes the star ever so slightly, that is, reduces its circumference so it moves leftward a bit in Figure 5.5 into the white region, then the star’s gravity will begin to overwhelm its pressure and will pull the star into an implosion; as the star implodes, it will move strongly leftward through Figure 5.5 until it crosses the neutron-star curve into the shaded region; there its neutron pressure will skyrocket, halt the implosion, and push the star’s surface back outward until the star settles down into a neutron-star grave, on the neutron-star curve.
By contrast, if, when the star is at the 1013 point, instead of being squeezed inward by a tiny random force, its surface gets pushed outward a bit (for example, by a random increase in the erratic motions of some of its neutrons), then it will enter the shaded region where pressure overwhelms gravity; the star’s pressure will then make its surface explode on outward across the white-dwarf curve and into the white region of the figure; and there its gravity will take over and pull it back inward to the white-dwarf curve and a white-dwarf grave.
This instability (squeeze the 1013 star a tiny bit and it will implode to become a neutron star; expand it a tiny bit and it will explode to become a white dwarf) means that no real star can ever live for long at the 1013 point—or at any other point along the portion of the equilibrium curve marked “unstable.”
Moving on along the equilibrium curve, we meet the entire family of neutron stars, with masses ranging from 0.1 to about 2 Suns. The maximum neutron-star mass of about 2 Suns is somewhat uncertain even in the 1990s because the behavior of the nuclear force at very high densities is still not well understood. The maximum could be as low as 1.5 Suns but not much lower, or as high as 3 Suns but not much higher.
At the (approximately) 2-solar-mass peak of the equilibrium curve, the neutron stars end. As one moves further along the curve to still higher densities, the equilibrium stars become unstable in the same manner as those between white dwarfs. and neutron stars (Box 5.6). Because of this instability, these “stars,” like those between white dwarfs and neutron stars, cannot exist in nature. Were they to form, they would immediately implode to become black holes or explode to become neutron stars.
Figure 5.5 is absolutely firm and unequivocal: There is no third family of stable, massive, cold, dead objects between the white dwarfs and the neutron stars. Therefore, when stars such as Sirius, which are more massive than about 2 Suns, exhaust their nuclear fuel, either they must eject all of their excess mass or they will implode inward past white-dwarf densities, past neutron-star densities, and into the critical circumference—where today, in the 1990s, we are completely certain they must form black holes. Implosion is compulsory. For stars of sufficiently large mass, neither the degeneracy pressure of electrons nor the nuclear force between neutrons can stop the implosion. Gravity overwhelms even the nuclear force.
There remains, however, a way out, a way to save all stars, even the most massive, from the black-hole fate: Perhaps all massive stars eject enough mass late in their lives (in winds or explosions), or during their deaths, to bring them below about 2 Suns so they can end up in the neutron-star or white-dwarf graveyard. During the 1940s, 1950s, and early 1960s, astronomers tended to espouse this view, when they thought at all about the issue of the final fates of stars. (By and large, however, they didn’t think about the issue. There were no observational data pushing them to think about it; and the observational data that they were gathering on other kinds of objects—normal stars, nebulas, galaxies—were so rich, challenging, and rewarding as to absorb the astronomers’ full attention.)
Today, in the 1990s, we know that heavy stars do eject enormous amounts of mass as they age and die; they eject so much, in fact, that most stars born with masses as large as 8 Suns lose enough to wind up in the white-dwarf graveyard, and most born between about 8 and 20 Suns lose enough to wind up in the neutron-star graveyard. Thus, nature seems almost to protect herself against black holes. But not quite: The preponderance of the observational data suggest (but do not yet firmly prove) that most stars born heavier than about 20 Suns remain so heavy when they die that their pressure provides no protection against gravity. When they exhaust their nuclear fuel and begin to cool, gravity overwhelms their pressure and they implode to form black holes. We shall meet some of the observational data suggesting this in Chapter 8.
There is much to be learned about the nature of science and scientists from the neutron-star and neutron-core studies of the 1930s.
The objects that Oppenheimer and Volkoff studied were Zwicky’s neutron stars and not Landau’s neutron cores, since they had no surrounding envelope of stellar matter. Nevertheless, Oppenheimer had so little respect for Zwicky that he declined to use Zwicky’s name for them, and insisted on using Landau’s instead. Thus, his article with Volkoff describing their results, which was published in the 15 February 1939 issue of the Physical Review, carries the title “On Massive Neutron Cores.” And to make sure that nobody would mistake the origin of his ideas about these stars, Oppenheimer sprinkled the article with references to Landau. Not once did he cite Zwicky’s plethora of prior neutron-star publications.
Zwicky, for his part, watched with growing consternation in 1938 as Tolman, Oppenheimer, and Volkoff pursued their studies of neutronstar structure. How could they do this? he fumed. Neutron stars were his babies, not theirs; they had no business working on neutron star—sand, besides, although Tolman would talk to him occasionally, Oppenheimer was not consulting him at all!
In the plethora of papers that Zwicky had written about neutron stars, however, there was only talk and speculation, no real details. He had been so busy getting under way a major (and highly successful) observational search for supernovae and giving lectures and writing papers about the idea of a neutron star and its role in supernovae that he had never gotten around to trying to fill in the details. But now his competitive spirit demanded action. Early in 1938 he did his best to develop a detailed mathematical theory of neutron stars and tie it to his supernova observations. His best effort was published in the 15 April 1939 issue of the Physical Review under the title “On the Theory and Observation of Highly Collapsed Stars.” His paper is two and a half times longer than that of Oppenheimer and Volkoff; it contains not a single reference to the two-months-earlier Oppenheimer–Volkoff article, though it does refer to a subsidiary and minor article by Volkoff alone; and it contains nothing memorable. Indeed, much of it is simply wrong. By contrast, the Oppenheimer–Volkoff paper is a tour de force, elegant, rich in insights, correct in all details.
Despite this, Zwicky is venerated today, more than half a century later, for inventing the concept of a neutron star, for recognizing, correctly, that neutron stars are created in supernova explosions and energize them, for proving observationally, with Baade, that supernovae are indeed a unique class of astronomical objects, for initiating and carrying through a definitive, decades-long observational study of supernovae—and for a variety of other insights unrelated to neutron stars or supernovae.
How is it that a man with so meager an understanding of the laws of physics could have been so prescient? My own opinion is that he embodied a remarkable combination of character traits: enough understanding of theoretical physics to get things right qualitatively, if not quantitatively; so intense a curiosity as to keep up with everything happening in all of physics and astronomy; an ability to discern, intuitively, in a way that few others could, connections between disparate phenomena; and, of not least importance, such great faith in his own inside track to truth that he had no fear whatsoever of making a fool of himself by his speculations. He knew he was right (though he often was not), and no mountain of evidence could convince him to the contrary.
Landau, like Zwicky, had great self-confidence and little fear of appearing a fool. For example, he did not hesitate to publish his 1931 idea that stars are energized by superdense stellar cores in which the laws of quantum mechanics fail. In mastery of theoretical physics, Landau totally outclassed Zwicky; he was among the top ten theorists of the twentieth century. Yet his speculations were wrong and Zwicky’s were right. The Sun is not energized by neutron cores; supernovae are energized by neutron stars. Was Landau, by contrast with Zwicky, simply unlucky? Perhaps partly. But there is another factor: Zwicky was immersed in the atmosphere of Mount Wilson, then the world’s greatest center for astronomical observations. And he collaborated with one of the world’s greatest observational astronomers, Walter Baade, who was a master of the observational data. And at Caltech he could and ‘did talk almost daily with the world’s greatest cosmic-ray observers. By contrast, Landau had almost no direct contact with observational astronomy, and his articles show it. Without such contact, he could not develop an acute sense for what things are like out there, far beyond the Earth. Landau’s greatest triumph was his masterful use of the laws of quantum mechanics to explain the phenomenon of superfluidity, and in this research, he interacted extensively with the experimenter, Pyotr Kapitsa, who was probing superfluidity’s details.
For Einstein, by contrast with Zwicky and Landau, close contact between observation and theory was of little importance; he discovered his general relativistic laws of gravity with almost no observational input. But that was a rare exception. A rich interplay between observation and theory is essential to progress in most branches of physics and astronomy.
And what of Oppenheimer, a man whose mastery of physics was comparable to Landau’s? His article, with Volkoff, on the structure of neutron stars is one of the great astrophysics articles of all time. But, as great and beautiful as it is, it “merely” filled in the details of the neutron-star concept. The concept was, indeed, Zwicky’s baby—as were supernovae and the powering of supernovae by the implosion of a stellar core to form a neutron star. Why was Oppenheimer, with so much going for him, far less innovative than Zwicky? Primarily, I think, because he declined—perhaps even feared—to speculate. Isidore I. Rabi, a close friend and admirer of Oppenheimer, has described this in a much deeper way:
“[I]t seems to me that in some respects Oppenheimer was overeducated in those fields which lie outside the scientific tradition, such as his interest in religion, in the Hindu religion in particular, which resulted in a feeling for the mystery of the Universe that surrounded him almost like a fog. He saw physics clearly, looking toward what had already been done, but at the border he tended to feel that there was much more of the mysterious and novel ‘than there actually was. He was insufficiently confident of the power of the intellectual tools he already possessed and did not drive his thought to the very end because he felt instinctively that new ideas and new methods were necessary to go further than he and his students had already gone.”
1. The amount of light received at Earth is inversely proportional to the square of the distance to the supernova, so a factor 10 error in distance meant a factor 100 error in Baade’s estimate of the total light output.
2. Antimatter gets its name from the fact that when a particle of matter meets a particle of antimatter, they annihilate each other.
3. It turns out that cosmic rays are made in many different ways. It is not yet known which way produces the most cosmic rays, but a strong possibility is the acceleration of particles to high speeds by shock waves in gas-cloud remnants of supernova explosions, long after the explosions are finished. If this is the case, then in an indirect sense Zwicky was correct.
4. The reason was explained in Box 4.2.
5. This may seem surprising to people who think of the nuclear force as far more powerful than the gravitational force. The nuclear force is, indeed, far more powerful when one has only a few atoms or atomic nuclei at one’s disposal. However, when one has several solar masses’ worth of atoms (1057 atoms) or more, then the gravitational force of all the atoms put together can become overwhelmingly more powerful than their nuclear force. This simple fact in the end guarantees, as we shall see later in this chapter, that when a massive star dies its huge gravity will overwhelm the repulsion of its atomic nuclei and will crunch them to form a black hole.
6. Superfluidity is a complete absence of viscosity (internal friction) that occurs in some fluids when they are cooled to a few degrees above absolute zero temperature—that is, cooled to about minus 270 degrees Celsius.
7. Actually, the maximum white-dwarf mass in Figure 5.5 (Wakano’s calculation) is 1.2 Suns, which is slightly less than the 1.4 Suns that Chandrasekhar calculated. The difference is due to a different chemical composition: Wakano’s stars were made of “cold, dead matter” (mostly iron), which has 46 percent as many electrons as nucleons (neutrons and protons). Chandrasekhar’s stars were made of elements such as helium, carbon, nitrogen, and oxygen, which have 50 percent as many electrons as nucleons. In fact, most white dwarfs in our Universe are more nearly like Chandrasekhar’s than like Wakano’s. That is why, in this book, I consistently quote Chandrasekhar’s value for the maximum mass: 1.4 Suns.