. . . from motion’s simple laws
Could trace the secret hand of Providence
Wide-working through this universal frame.
JAMES THOMSON in his
Memorial Poem to Newton
While Galileo was fashioning the new science of motion, Johannes Kepler was making dramatic contributions to one of the most far-reaching developments in the history of Western civilization. This development was begun by Nicolaus Copernicus and its essence was a radically new mathematical theory of planetary motions.
Up to the sixteenth century the only sound and useful astronomical theory was the geocentric system of Hipparchus and Ptolemy which we examined in Chapter 8. This was the theory accepted by professional astronomers and applied to calendar reckoning and navigation. It was, however, a rather sophisticated creation in that its strength lay entirely in the mathematical effectiveness of the scheme. The deferents and epicycles had no physical significance in themselves nor did the theory give any physical or intuitive reasons that the planets should move on epicycles attached to deferents.
The author of the next great celestial drama was Nicolaus Copernicus, who lived about 1400 years after Ptolemy. Copernicus was born in Poland in 1473 and, after studying mathematics and science at the University of Cracow, decided to go to Italy, the center of the revived Greek learning. At the University of Bologna, which he entered in 1497, he studied astronomy. Then for ten years he studied medicine and law and secured a doctor’s degree in both fields. He also became learned in Greek and mathematics. In 1500 Copernicus was appointed a canon of the Cathedral of Frauenberg in East Prussia, but he did not assume his duties until 1512 when he had finished his studies in Italy. The job, which entailed mainly the management of estates owned by the Cathedral, left Copernicus with plenty of time to make astronomical observations and to think about the relevant theory. After years of reflection and observation Copernicus finally evolved a new theory of planetary motions which he incorporated in a classic work, On the Revolutions of the Heavenly Spheres. This appeared in 1543, the year in which Copernicus died.
As we have already noted, when Copernicus began to think about astronomy, the Ptolemaic theory was the only sound and effective system in existence. This theory had become somewhat more complicated during the intervening centuries in that more epicycles had been added to those introduced by Ptolemy, to make the theory fit the increased amount of observational data gathered largely by the Arabs. In Copernicus’ time the theory required a total of 77 circles to describe the motion of the sun, moon, and the five planets known then.
Copernicus had studied the Greek works and had become convinced that the universe was mathematically and harmoniously designed. Harmony demanded a more pleasing theory than the complicated extensions of Ptolemaic theory. Copernicus read that some Greek authors, notably Aristarchus, had suggested the possibility that the sun might be stationary and that the earth revolved about the sun and rotated on its axis at the same time. He decided to explore this possibility. He was in a sense overimpressed with Greek thought, for he, too, believed that the motions of heavenly bodies must be circular or, at worst, a combination of circular motions since circular motion was natural motion. Moreover, he also accepted the belief that each planet must move at a constant speed on its epicycle, and that the center of each epicycle must move at a constant speed on the circle which carried it. Such principles were axiomatic for him. Copernicus even adds an argument which shows the somewhat mystic character of sixteenth-century thinking. He says that a variable speed could be caused only by a variable power; but God, the cause of all motions, is constant.
The upshot of such reasoning was that Copernicus used the scheme of deferent and epicycles to describe the motions of the heavenly bodies, with, however, the all important difference that the sun was at the center of each deferent, while the earth itself became a planet moving about the sun and rotating on its axis. Nevertheless, he achieved considerable simplification. He was able to reduce the total number of circles, deferents and epicycles, to 34 instead of the 77 required under the geocentric view.
However, the remarkable simplification was achieved by Johannes Kepler, one of the most intriguing figures in the history of science. In a life beset by many personal misfortunes and hardships occasioned by social and political events, Kepler had the good fortune to become in 1600 an assistant to the famous astronomer Tycho Brahe. Brahe was then engaged in making extensive new observations, the first such major undertaking since Greek times. These observations, together with others which Kepler made himself, were invaluable to him in his later work. When Brahe died in 1601 Kepler succeeded him as Imperial Mathematician to the Emperor Rudolph II of Austria, King of Bohemia.
Kepler’s scientific reasoning is fascinating. Like Copernicus, he was a mystic and, like Copernicus, he believed that the world was designed by God in accordance with some simple and beautiful mathematical plan. This belief dominated all his thinking. But Kepler also had qualities which we now associate with scientists. He could be coldly rational. His fertile imagination triggered the conception of new theoretical systems. But he knew that theories must fit observations and, in his later years, saw even more clearly that empirical data may indeed suggest the fundamental principles of science. Copernicus, too, wanted his theory to fit observational data; yet he held to the heliocentric view, although the differences between theoretical predictions and astronomical data were greater than might be accounted for by experimental errors alone. Kepler, on the other hand, sacrificed his most beloved theories when he saw that they did not fit observational data, and it was precisely this incredible persistence in refusing to tolerate discrepancies which any other scientist of his day would have disregarded that led him to espouse radical ideas. He also had the humility, patience, and energy to perform extraordinary labor which mark great men.
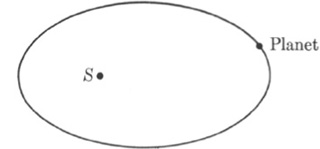
In his book On the Motion of the Planet Mars, published in 1609, Kepler announced the first two of his three famous laws of planetary motion. The first of these is especially remarkable, for Kepler broke with the tradition held for 2000 years that circles or spheres must be used to describe heavenly motions. Instead of resorting to deferent and several epicycles, which both Ptolemy and Copernicus had used to describe the motion of any one planet, Kepler found that a single ellipse would do. His first law states that each planet moves on an ellipse and that the sun is at one (common) focus of each of these elliptical paths (Fig. 15–1). The other focus of each ellipse is merely a mathematical point at which nothing physical exists.
Kepler’s first law utilizes a geometrical figure which had been introduced and studied by the Greeks of the classical period. Had the ellipse and its properties not yet been known, and had Kepler been faced with the double problem of abstracting the proper path from a multitude of data and conceiving the ellipse, he might possibly have ended up in an impasse. By working out the properties of this curve Euclid, Apollonius, and Archimedes determined the course of our civilization just as decisively as if they had stood at Kepler’s side.
Fig. 15–1.
Each planet moves in an ellipse about the sun.
Fig. 15–2.
Kepler’s law of equal areas.
Kepler’s first law is of immense value in comprehending readily the paths of the planets. But astronomy must go much further if it is to be interesting in itself and useful. It must tell us how to predict the positions of the planets. If one finds by observation that a planet is at a particular position, P say in Fig. 15–2, he might like to know when it might be at some other position, a solstice or an equinox, for example. What is needed is the velocity with which the planets move along their respective paths.
Here, too, Kepler made a radical step. Copernicus, as we noted earlier, and the Greeks had always used constant velocities. A planet moved along its epicycle so as to cover equal arcs in equal times, and the center of each epicycle also moved at a constant velocity on another epicycle or on a deferent. But Kepler’s observations told him that a planet moving on its ellipse does not move at a constant speed. Kepler searched hard and long for the correct law of velocities and found it. What he discovered was that if a planet moves from P to Q (Fig. 15–2) in, say one month, then it will also move from P′ to Q′ in one month, provided that the area PSQ equals the area P′SQ′. Since P is nearer the sun than P′ is, the arc PQ must be larger than the arc P′Q′ if the areas PSQ and P′SQ′ are equal. Hence the planets do not move at a constant velocity. In fact, they move faster when closer to the sun.
Kepler was overjoyed to discover this second law. Although it is not so simple to apply as a law of constant velocity, it nonetheless confirmed his fundamental belief that God had used mathematical principles to design the universe. God had chosen to be just a little more subtle, but a mathematical law clearly determined how fast the planets moved.
Another major problem remained open. What law described the distances of the planets from the sun? The problem was now complicated by the fact that a planet’s distance from the sun was not constant but varied from a least to a greatest value (see Fig. 15–2). Hence Kepler searched for a new principle which would take this fact into account. Now he believed that nature was not only mathematically but harmoniously designed and he took this word “harmony” very literally. Thus he believed that there was a music of the spheres which produced a harmonious tonal effect, not one given off in actual sounds but discernible by some translation of the facts about planetary motions into musical notes. He followed this lead and after an amazing combination of mathematical and musical arguments, arrived at the law that if T is the period of revolution of any planet and D is its mean distance from the sun, then
T2 = kD3,
where k is a constant which is the same for all the planets. This statement is Kepler’s third law of planetary motion and the one which he triumphantly announced in his book The Harmony of the World (1619).
The work of Copernicus and Kepler is by far the most dramatic, startling, and influential development in the formation of modern culture. The first surprising feature, and one which in itself makes their work astounding, is the sharp break from existing thought. Copernicus and Kepler were educated in a milieu which accepted the geocentric theory of Ptolemy as almost unquestionable truth. Moreover, both were scientifically cautious. Nor did either really have at his disposal any unusual observations which conflicted sharply with Ptolemy’s theory. Copernicus, as a matter of fact, was not a great observer and did not seem to mind leaving his work somewhat at odds with observations. Kepler did have access to more numerous and more reliable data and showed greater tenacity in making the theory fit the data, but there was nothing in these observations which suggested that an entirely new theory must be introduced.
While casting aside the weight of centuries, Copernicus and Kepler could give only token rebuttals to the numerous scientific arguments against a moving earth which Ptolemy had advanced against Aristarchus. How could the heavy earth be put into motion? That the other planets were in motion even according to Ptolemaic theory was explained by the doctrine that these were made of special light matter and therefore easily moved. About the best answer Copernicus could give was that it was natural for a sphere to move. Another scientific argument against the earth’s rotation maintained that rotation would cause objects to fly off into space just as an object on a rotating platform will fly off. Copernicus had no answer to this argument. To the further objection that a rotating earth should itself fly apart, Copernicus replied weakly that since the earth’s motion was natural, it could not destroy the body. Then he countered by asking why the sky did not fall apart under the very rapid daily motion which the geocentric theory called for. Yet another objection declared that if the earth rotated from west to east, an object thrown up into the air should fall back to the west of its original position because the earth moved on while the object was in the air. If, moreover, the earth revolved about the sun, then, since the velocity of an object is proportional to its weight, or so at least Greek and Renaissance physics maintained, lighter objects on the earth should be left behind. Even the air should be left behind. To the last argument Copernicus replied that air is earthy and so moves in sympathy with the earth.
These scientific objections to a moving earth were weighty ones and could not be dismissed as the stubborness of doubters who refused to see the truth. The substance of the matter is that a rotating and revolving earth did not fit in with the physical theory of motion due to Aristotle and common in Copernicus’ and Kepler’s time.
Another class of scientific arguments against a heliocentric theory came from astronomy proper. The most serious one stemmed from the fact that the heliocentric theory regarded the stars as fixed. In six months the earth changes its position in space by 186 million miles. Hence if one notes the direction of a particular star at one time and again six months later, he should observe a difference in direction. But this difference was not observed in Copernicus’ and Kepler’s time. Copernicus answered that the stars are so far away that the difference in direction was too small to be observed. However, his explanation did not satisfy the critics, who countered that if the stars were that distant, then they should not be clearly observable. In this instance, Copernicus’ answer was correct. The change in direction over a six-month period for the nearest star is an angle of 0.76″, and this was first detected by the mathematician Bessel in 1838 who, of course, by that time had a good telescope at his disposal.
A further, powerful argument against a moving earth contended that we do not feel any motion despite the fact that the earth is presumably moving around the sun at 18 miles per second and that a person on the equator is rotating at the rate of about 0.3 miles per second. Our senses, on the contrary, tell us that the sun is moving in the sky. Of course, there are counterarguments which would mean more to us today because we have the experience of traveling at high speeds. A person traveling in an airplane at 400 miles per hour does not feel the motion. But to the people of Copernicus’ time the argument that we do not feel ourselves moving at the very high speeds called for by the new astronomy was convincing.
In view of the numerous and sound arguments against the heliocentric theory and the challenge it posed to the prevailing religious thinking of the times, what made Copernicus and Kepler take up this long-discarded thought and pursue it so courageously? For what most other men would call a mess of pottage, they broke with established physics, philosophy, religion, and common sense.
Both were convinced that the universe is mathematically designed and hence that a true pattern of the motions was inherent. Moreover, this design was instituted by God, and God would surely have used a simple and harmonious pattern. But Ptolemaic theory had become so encumbered in the sixteenth century that it was no longer simple or beautiful. Hence Copernicus and Kepler believed, when each found a more harmonious and simpler theory, that their work was indeed a description of the divine order of things.
There are many passages in Copernicus’ On the Revolutions of the Heavenly Spheres and in Kepler’s writings which bear unmistakable testimony to this motivation as the central force in the search for a new theory and to their conviction that they had found the right one when it proved to be simpler. Copernicus says of his theory:
We find therefore, under this orderly arrangement, a wonderful symmetry in the universe, and a definite relation of harmony in the motion and magnitude of the orbs, of a kind that it is not possible to obtain in any other way.
Kepler remarks of his later work wherein he had already introduced the elliptical theory of motion, “I have attested it as true in my deepest soul and I contemplate its beauty with incredible and ravishing delight.” The work of Copernicus and Kepler is the work of men searching the universe for the harmony which their religious convictions assured them must exist and which must be describable mathematically and simply because God had so designed the universe. What distinguishes their religious convictions from those of their contemporaries is that they did not tie themselves to literal interpretations of the Holy Writings. They searched for the word of God in the heavens.
The core of the argument which Copernicus and Kepler presented for the heliocentric theory was its mathematical simplicity. Their philosophical and religious convictions assured them that the world is mathematically and simply designed; accordingly the fact that a heliocentric view was mathematically simpler than the geocentric one determined their position. The mathematical simplicity of the new view was, in fact, the sole argument they could advance. Only persons possessed of the unshakable conviction that mathematics is the essence of the design of the universe and that the omnipotent mathematician would necessarily prefer simplicity would dare to advance such a radical theory and would have had the courage to defend it against the opposition it was sure to and did encounter in those times.
The new theory appealed to astronomers, geographers, and navigators because it simplified their theoretical and arithmetical work. Hence many of these men adopted the new view just as a mathematical convenience, even though they were not convinced of its truth. While this feature of the new theory carried little weight with Copernicus and Kepler, it nonetheless had the effect of making more and more people think in terms of a heliocentric view, and, since one tends to accept as true what is familiar, there is no doubt that this practical aspect did, in the long run, help to gain adherents for the theory.
Support for the new theory came from an unexpected development. Early in the seventeenth century the telescope was invented, and Galileo, upon hearing of this invention, built one himself. He then proceeded to make observations of the heavens which startled his age. He detected four moons of Jupiter (we now can observe twelve), and this discovery showed that a moving planet can have satellites. Hence it was likely that the earth, too, could be in motion and yet have a satellite, our moon. Galileo saw irregular surfaces and mountains on the moon, spots on the sun, and a bulge around the equator of Saturn (which we now call the rings of Saturn). Here was further evidence that the planets were like the earth and certainly not perfect bodies composed of some special ethereal substance, as Greek and medieval thinkers had believed. The Milky Way, which had hitherto appeared to be just a broad band of light, could be seen with the telescope to be composed of thousands of separate stars, each of which gave off light. Thus, there were other suns and presumably other planetary systems suspended in the heavens. Moreover, the heavens clearly contained more than seven moving bodies, a number which had been accepted as sacrosanct. Copernicus had predicted that if human sight could be enhanced, then man would be able to observe phases of Venus and Mercury, that is, to observe that more or less of each planet’s hemisphere facing the earth is lit up by the sun, just as the naked eye can discern the phases of the moon. Galileo did discover the phases of Venus.
All of Galileo’s observations were made with a telescope of such limited power that, as has been said, it is remarkable he could find Jupiter, let alone the moons of Jupiter. Many of his discoveries were in direct support of a heliocentric theory; others served primarily to challenge current beliefs and to at least prepare some minds for a more objective examination of the new theory. Galileo, himself, though he lectured on Ptolemaic theory until 1605, had been converted to Copernicanism by a work of Kepler. In 1611 he openly declared for Copernicanism. His own observations convinced him that the Copernican system was correct, and in the classic Dialogue on the Great World Systems he defended it strongly. By the middle of the seventeenth century the scientific world was willing to proceed on a heliocentric basis.
One word of caution regarding the work of Copernicus and Kepler: these men believed that the heliocentric theory was true for the reasons already cited. This is not the view we hold today. If the criterion is truth, then heliocentric theory is not to be preferred to Ptolemaic theory. Scientific theories, we now believe, are the work of man. The mind supplies the patterns which organize observations. We may indeed prefer the heliocentric theory because it is simpler and agrees better with observations, but we do not regard it as the last word. Another theory, which still may not be the truth, may be conceived and produce even better results. As a matter of fact, one was—the theory of relativity. We shall not anticipate too much. The evolution of the concept of truth as it applies to mathematics and mathematical theories of science will be a continuing concern.
1. What is a geocentric astronomical system? a heliocentric astronomical system?
2. Did Copernicus break completely with Greek astronomy?
3. Is the sun at the center of the Keplerian system?
4. What innovations did Kepler introduce into the Copernican system?
5. To reconstruct Kepler’s improvement on Copernicus, suppose that a planet P moves once around its epicycle while the center of the epicycle moves once completely around the sun. What path does the planet seem to follow in relation to the sun? Would it be simpler to accept this single path as opposed to the combination of epicycle and deferent?
6. What scientific objections were there to an earth in motion?
7. Why did Copernicus and Kepler advocate the new heliocentric theory?
8. Why do you accept the heliocentric theory?
9. State Kepler’s first law of planetary motion.
10. State Kepler’s second law of planetary motion.
11. If we take the earth’s average distance from the sun, 93,000,000 mi, as the unit of distance and the earth’s period of revolution, 1 year, as the unit of time, then Kepler’s third law says that T2 = D3. If the average distance of Neptune from the sun is 2,797,000,000 mi, how long does it take the planet to complete one revolution around the sun?
In view of the fact that Galileo had discovered the laws which underlie terrestrial motions and Kepler had discovered the basic laws of planetary motion one would expect that the scientists of the seventeenth century would have regarded the theory of motion to be complete. But to scientists who seek the ultimate design of our universe, the two accomplishments we have just described immediately suggested more profound problems. A comparison of these two classes of laws, namely Galileo’s for terrestrial motions and Kepler’s for heavenly motions, revealed several basic differences. In the first place, Galileo had started with clear physical principles, such as the first law of motion and the constant downward acceleration of objects moving near the surface of the earth, and had deduced the formulas which describe straight-line and curvilinear motions. Kepler’s three laws, though they fitted observations within the limits of observational errors, did not rest on physical principles. They were merely accurate mathematical descriptions of collections of data. Moreover, the three laws were logically independent of one another. Secondly, for terrestrial motions, the parabola was found to be the basic path of curvilinear motion, whereas for planetary motion, the ellipse was the basic path.
This comparison raised several questions. Could one establish any logical relationship among the Keplerian laws or were they really independent? What physical principles determined planetary motions? The mathematical laws, accurate and succinct as they were, presented after all only a rather bleak account, without giving any insight into, or rationale for, the motions. And why should parabolic paths prevail on earth and elliptical paths in the heavens?
The overriding question, however, which bothered the leading scientists of the latter half of the seventeenth century was: Could one establish a connection between the laws of terrestrial motion and the laws of planetary motion? Perhaps the very same physical principles which Galileo had used to deduce the paths of objects moving near the earth could lead to the laws describing the motion of the planets. In this event, the two classes of laws would be united; the Keplerian laws would be related to each other by being deduced from a common basis; and the physical reasons for planetary motion would be revealed.
The thought that all the phenomena of motion should follow from one set of physical principles might seem grandiose and inordinate to reasonable people, but it occurred very naturally to the religious mathematicians of the seventeenth century. God had designed the universe, and it was to be expected that all phenomena of nature would follow one master plan. One mind designing a universe would almost surely have employed one set of basic principles to govern as many related phenomena as possible. Since the scientists of the seventeenth century were engaged in the quest for God’s design of nature, it seemed very reasonable to them that they should seek the unity underlying the diverse earthly and heavenly motions. As phrased by Newton, this goal was
to derive two or three general principles of motion from phenomena, and afterwards to tell us how the properties and actions of all corporeal things follow from these manifest principles. . .
A less cogent but to mathematicians nonetheless significant indication of the existence of some unity was furnished by the fact that parabola and ellipse were both conic sections. The common mathematical origin of these curves warranted some belief that parabolic and elliptical motions were but special cases of some fundamental principle of motion.
In the seventeenth century, there were other less weighty but perhaps more pressing reasons to pursue the study of motion beyond the stage reached by Galileo and Kepler. Another open question was how to relate heavenly and earthly motions in a more limited but practical connection. This was the problem described earlier of determining the longitude of a ship at sea. Although navigators had used the stars, sun, and moon to determine the locations of their ships, the positions of these celestial bodies at various times of the year had yet to be related more precisely to the longitudes of points on the earth. In the seventeenth century it seemed that the moon would be most suitable for the determination of longitude because its closeness permitted accurate observation of its position from points on the earth. Hence, more precise information about the motion of the moon around the earth was needed. This became a major scientific problem of the age.
Any great advance in mathematics and science is almost always the work of many men contributed bit by bit over hundreds of years. Then one man smart enough to distinguish the worthy ideas of his predecessors from the welter of suggestions and results and imaginative and audacious enough to fit the significant ideas into a master plan makes the culminating and definitive step. In the problem of unifying all the phenomena of motion, the decisive step was made by Isaac Newton.
He was born in 1642, premature and weak. His mother was already widowed and so preoccupied with running the family farm that she could pay no attention to the boy. The elementary education Newton received in local schools of a small English town could hardly have given him much of a start, and in his youth Newton showed no promise. His family sent him to Cambridge University, where he entered Trinity College in 1661. Here, at last, Newton got the opportunity to study the works of Copernicus, Kepler, and Galileo, and here he had at least one good teacher, the distinguished mathematician Isaac Barrow. His university work was not outstanding and he had, in fact, such difficulties with geometry that he almost changed his course of study from science to law. However, Barrow did recognize that Newton had ability.
Newton finished his undergraduate work; at that point an outbreak of the plague in the area around London led to the closing of the university. He, therefore, spent the years 1665 and 1666 in the quiet of the family home at Woolsthorpe. During this period Newton initiated his great work in mechanics, mathematics, and optics. He realized that the law of gravitation, which we shall examine shortly, was the key to an embracing science of mechanics; he obtained a general method for treating the problems of the calculus (see Chapters 16 and 17); and through experiments he made the epochal discovery that white light such as sunlight is really composed of all colors from violet to red. “All this,” Newton said later in life, “was in the two plague years of 1665 and 1666, for in those days I was in the prime of my age for invention, and minded mathematics and philosophy [science] more than at any other time since.”
Newton returned to Cambridge in 1667 and was elected a Fellow of Trinity College. In 1669, Isaac Barrow resigned his professorship of mathematics to devote himself to theology, and Newton was appointed in Barrow’s place. Newton apparently was not a successful teacher, for few students attended his lectures; nor did anyone comment on the originality of the material he presented.
In 1684 his friend Edmond Halley, the astronomer of Halley’s comet fame, urged him to publish his work on gravitation and even assisted him editorially and financially. Thus in 1687 the classic of science, the Mathematical Principles of Natural Philosophy, often briefly referred to as the Principia or the Principles, appeared. This book received much acclaim and, aside from three Latin editions, appeared in many languages. One popularization was entitled New-tonianism for Ladies. The Principia is written in the deductive manner of Euclid; that is, it contains definitions, axioms, and hundreds of theorems and corollaries. Its conciseness makes it difficult reading. To excuse this aspect, Newton told a friend that he had made the Principia difficult on purpose “to avoid being baited by little smatterers in mathematics.” He thereby hoped to avoid the criticisms heaped on his earlier papers on light.
After about thirty years of creative activity which included some work in chemistry, Newton became depressed and suffered a nervous breakdown. He left Cambridge University to become Warden of the British Mint in 1696 and thereafter confined his scientific activities to the investigation of an occasional problem. He did, however, devote himself to theological studies, which he regarded as more fundamental than science and mathematics because the latter disciplines concerned only the physical world. In fact, had Newton been born two hundred years earlier he would almost surely have become a theologian. An example of his theological writing is The Chronology of the Ancient Kings Amended, in which he sought to determine the dates of Biblical events by utilizing astronomical facts mentioned in connection with these events.
During his last years and posthumously he was honored in many ways. He was President of the Royal Society of London from 1703 to his death; he was knighted in 1705; and he was buried in Westminster Abbey.
In his philosophy and method of science, Newton followed Galileo. He, too, believed that the universe was mathematically designed by God and that mathematics and science should strive to uncover that glorious design. Like Galileo, he was convinced that fundamental physical principles should be quantitative statements about the real qualities of the world, space, time, mass, weight, and force. From these principles and with the axioms and theorems of mathematics, it should be possible to deduce the laws of nature. Newton expressed this philosophy in the preface to his Principles:
. . . for the whole burden of philosophy [science] seems to consist in this—from the phenomena of motion to investigate the forces of nature, and from these forces to demonstrate the other phenomena. . .
By investigating the forces of nature, he meant to arrive at the basic laws governing the operation of these forces and to deduce the consequences.
The first problem, then, in executing such a program is to discover the fundamental principles. Like Galileo, Newton insisted on obtaining these by direct study of the physical world rather than by searching one’s mind for hypotheses that seemed to be reasonable or by accepting Biblical passages.
Here, too, Newton is explicit. In another of his famous books, Opticks, first published in 1704, he says:
Thus analysis consists in making observations and experiments and in drawing general conclusions by induction, and admitting of no objections against the conclusions, but such as are taken from experiments or other certain truths.
What Newton sought to emphasize and what required emphasis in his time is that generalizations must be based on some experimental or observational grounds, and that no hypothesis can be tolerated which is contrary to a single bit of physical evidence. Further, deductions made from the basic principles must also be in accord with physical evidence, for only by continued agreement between deductively established conclusions and experimental tests can one acquire confidence that the original generalizations are correct.
With such principles of scientific method clearly in mind, Newton turned to the problem of finding the physical principles which would lead to a unifying theory of earthly and celestial motions. He was, of course, familiar with the principles unearthed by Galileo. But these were presumably not enough. It was clear from the first law of motion that the planets must be acted on by a force which pulls them toward the sun, for if no force were acting, each planet would move in a straight line. The idea of a force which constantly pulls each planet toward the sun had occurred to many men, Kepler, the famous experimental physicist, Robert Hooke, the physicist and renowned architect, Christopher Wren, Halley and others, even before Newton set to work. It had also been conjectured that this force exerted on a distant planet must be weaker than that exerted on a nearer one and, in fact, that this force must decrease as the square of the distance between sun and planet increased. But, prior to Newton’s work, none of these thoughts about a gravitational force advanced beyond speculation.
Newton adopted these ideas. However, in his attempt to tie in the action of the gravitational force with motions on the earth, a line of thinking occurred to him which was highly imaginative and certainly original in his time but which is now an almost daily experience. He considered the problem of what happens when a projectile is shot out horizontally from the top of a mountain. As Newton knew and as we know from our study of Galileo’s work, the projectile follows a parabolic path to earth (see Chapter 14, Fig. 14–3). If the horizontal speed of the projectile is increased, then the path is wider but remains parabolic. However, Galileo had assumed that the earth was flat and that the projectiles were given moderate initial horizontal speeds such as a cannon might impart to shells. Newton then asked himself what would happen if the sphericity of the earth were taken into account, and if the horizontal speeds of the projectiles were gradually increased. If the sphericity of the earth is taken into account, then projectiles with small horizontal speeds will follow the paths PA and PB of Fig. 15–3. As the speed is increased somewhat, the projectile might take a path such as PC. Suppose now that the speed is increased still more. Would the projectile fall off into space? Not necessarily. As the projectile travels into space, it is pulled toward the earth. But the pull of a spherical earth is directed toward the center, and hence the projectile, subjected to this continuous pull toward the center, need not fall off into space. It might, in fact, continue to circle the earth indefinitely if the earth pulled it in just enough so that it would not wander out into space and yet not fall to earth.
And so Newton concluded in his Principles:
And after the same manner that a projectile, by the force of gravity, may be made to revolve in an orbit, and go round the whole earth, the moon also, either by the force of gravity, if it is endowed with gravity, or by any other force, that impels it toward the earth, may be continually drawn aside towards the earth, out of the rectilinear [straight-line] way which by its innate force [inertia] it would pursue; and would be made to revolve in the orbit which it now describes; nor could the moon without some such force be retained in its orbit. If this force were too small, it would not sufficiently turn the moon out of a rectilinear course; if it were too great, it would turn it too much, and draw the moon from its orbit toward the earth. It is necessary that the force be of a just quantity, and it belongs to the mathematicians to find the force that may serve exactly to retain a body in a given orbit with a given velocity;. . .
Fig. 15–3.
Projectiles shot out horizontally from the top of a mountain with increasing horizontal velocities.
This argument which showed how the motion of the moon around the earth could be related to motions occurring on earth was immediately extended to the motions of the planets about the sun. The planets, set into motion some-how, are attracted by the sun and are presumably pulled in just enough to keep them from flying off into space or from crashing into the sun.
Thus Newton had some reason to suppose that the same force which pulled projectiles to earth caused the moon to revolve around the earth and the planets to revolve around the sun. He had now to determine precisely how strong the force of gravitation is, that is, how it depends upon the bodies involved and upon the distances between the bodies.
Before we can understand Newton’s law of gravitation we must distinguish two properties of matter, mass and weight. Newton’s first law of motion says that if no force is applied, bodies continue at the speed they already have. Stated otherwise, the law says that bodies have inertia; they persist in the motion they already have unless compelled to do otherwise by the application of force. This inertia or resistance of matter to change in speed is called inertial mass or just mass.
Do all objects have the same mass? Not at all. Since mass exhibits itself in an object’s resistance to change in speed, we can appeal to experience to see that different objects may possess different masses. Suppose, for example, that a small and a large ball of lead are at rest on the ground and one wishes to start them moving. Experience tells us that we must exert more force to get the larger ball rolling than to get the smaller one rolling with the same speed. Since more force is required in the first case, the larger ball must possess more mass. Or we can imagine the force that might be required to stop these balls if they were rolling toward us at the same speed. Again, more force would be required to stop the larger one. Thus the masses of objects are not the same.
We shall not present physical methods of measuring mass. It suffices to know that by adopting a unit of mass, just as we adopt a unit of length, we can compare all other masses with this unit and so determine exactly how much mass there is in any individual piece of matter. Mass is measured in pounds or grams, the pound being approximately 454 grams.
Bodies falling to earth possess acceleration. Hence some force must be acting to produce this change in speed. The force, as Galileo and others realized, is the pull of the earth or the force of gravity. We feel this pull when we hold an object in our hands. This particular force applied to an object is called the weight of the object. Hence weight and mass are by no means the same. Mass is inertia or resistance to change in speed, and weight is a force exerted by the earth.
However, there is a remarkable, experimentally determined relationship between the mass and weight of an object, namely, near the surface of the earth the weight is always 32 times the mass; in symbols:
The quantity 32 is precisely the acceleration which all bodies falling to earth possess. Thus equation (1) says that the force, w, which the earth exerts on a mass, m, is the acceleration with which it causes the mass to fall times the mass. When the mass m is measured in pounds, the weight w is measured in poundals. Thus a mass of one pound has a weight of 32 poundals. For convenience, 32 poundals are called one pound of weight. Of course, a pound of mass and a pound of weight are not the same physical quantities; and it is confusing at times to use the same unit for both mass and weight. Yet, as we shall see in a moment, this confusion is not too serious. (If the mass is measured in grams, the quantity which replaces 32 is 980, and the units are centimeters per sec2. Then instead of (1) we have
w = 980m,
and the weight w is measured in dynes.)
Because weight and mass are so intimately related, we do not trouble in ordinary life to distinguish between the two properties. Large masses have large weights, and so, even in those instances where we are actually concerned with the mass of an object, we often tend to think of weight. For example, if one were to try to start an automobile rolling by pushing it, he would have to exert considerable force. The average person relates this fact to the great weight of the automobile. However, weight plays no role here because the force of gravity acts downward and has no effect on motion along the ground. The forceful push is required because the mass resists change in speed. Hence, it is the mass of the automobile rather than the weight which calls for the exertion of great force.
1. Why do people usually fail to distinguish between mass and weight?
2. Let us assume that the relationship between weight and mass on the moon has the same mathematical form as on the earth; that is, the weight is a constant times the mass. The acceleration which the moon gives to all bodies falling toward its surface is 5.3 ft/sec2. If a man weighs 160 lb on the earth, what will he weigh on the moon?
3. If the acceleration which the sun imparts to bodies falling toward its surface is 27 times that imparted by the earth, what would a man whose weight on the earth is 160 lb weigh on the sun?
Newton adopted the conjecture already made by his contemporaries, namely, that the force of attraction, F, between any two bodies of masses m and M, respectively, separated by a distance r is given by the formula
In this formula, G is a constant; i.e., it is the same number, no matter what m, M, and r may be. The numerical value of this constant, which we shall determine later, depends upon the units used for mass, force, and distance.
From the mathematical standpoint, formula (2) represents a new type of functional relationship. The quantity F is the dependent variable which depends upon three independent variables, m, M, and r, the quantity G being a constant. If we give values to m, M, and r, then the value of F is determined. Such a function is, of course, more complicated than, say the formula d = 96t – 16t2, which contains just one independent variable, t, and one dependent variable, d. When one is working in a situation in which m and M are fixed and only r can vary, then F is a function of just one independent variable. For example, if G were 1, m were 2, and M were 3, then the relationship between F and r would be F = 6/r2. This formula expresses the dependence of the gravitational force between two fixed masses on the distance between them.
Newton had yet to show that formula (2) was the correct quantitative expression for this force. To apply formula (2) and to work with forces in general, Newton adopted a second quantitative physical principle which proved to be just as important as his law of gravitation. As we noted in the preceding section, near the surface of the earth the force of gravity of the earth gives to objects an acceleration of 32 ft/sec2, and the force is 32 times the mass of the object. Newton generalized this relationship and affirmed that whenever any force acts on an object it gives that object an acceleration. Moreover, the relationship of force, mass of the object, and acceleration imparted to the object, is
In this formula F is the amount of force applied to the object of mass m, and a is the amount of acceleration imparted to the object. In the special case where F is the weight w, the value of a is 32 ft/sec2. Formula (3) is known as Newton’s second law of motion. It applies to any force, whether or not it be the force of gravity. As in the case of formula (1), if m is measured in pounds and a in feet and seconds, then F is measured in poundals. Thus a force of 32 poundals gives a mass of 1 pound an acceleration of 32 ft/sec2. The unit, pound, is also used for forces with the understanding that one pound of force equals 32 poundals.
Let us see how Newton tested his law of gravitation. We shall write (2) in the slightly different form
If we compare (3) and (4), we observe that the quantity GM/r2 in (4) plays the role of a in (3); that is, the law of gravitation can be viewed as stating that the gravitational force F gives a mass m the acceleration GM/r2. In symbols,
Now let M be the mass of the earth and let m be the mass of a small body near the surface of the earth. Then there is the question of what r in (5) represents. It is supposed to represent the distance between the two masses. Shall we take it then to be the distance from the mass m to the surface of the earth or to some point in the interior? If the two masses were separated by millions of miles, as are the earth and sun, one might idealize each mass and regard it as concentrated at one point because the size of each mass is small compared with the distance between them. But for objects near the surface of the earth, the value of r depends heavily upon what point in or on the surface of the earth is chosen as the position of the earth. Newton conjectured (and later proved) that for purposes of gravitational attraction the mass of the earth could be regarded as though it were concentrated at the earth’s center. Hence, with respect to the earth’s gravitational acceleration acting on a mass m near the surface of the earth, r in (5) can be taken to be 4000 miles or 21,120,000 feet. This value of r is essentially the same for all objects near the surface of the earth. Moreover, the mass M of the earth is constant, and so is G. Hence, for all objects near the surface of the earth, the entire right side of (5) is constant. Consequently, the acceleration which gravity imparts to all objects near the surface of the earth is constant. This is precisely what Galileo had found and, in fact, he had determined that the constant is 32 ft/sec2. Thus Newton’s law of gravitation met its first test, for it yielded as a special case a well established fact.
1. Suppose that the gravitational force varies with the distance between two definite masses according to the formula F = 6/r2. Show graphically how F varies with r.
2. Knowing that the acceleration of objects near the surface of the earth is 32 ft/sec2, use formula (5) to calculate the acceleration which the earth exerts on objects 1000 mi above the surface of the earth.
3. Suppose that an object falls to earth from a point 1000 mi above the surface. May we use the formula d = 16t2 to compute the time it takes to fall this distance?
4. What is the mass of an object which weighs 150 lb? (One pound of weight is 32 poundals.)
5. How much force is required to give an automobile weighing 3000 lb an acceleration of 12 ft/sec2?
With some support for the law of gravitation we can now, following Newton’s example, adopt it as an axiom of physics and see what conclusions we may draw from this axiom and the other axioms of physics and mathematics. The law itself states that the force of gravitation F between any two masses m and M is given by the formula
where r is the distance between the masses. Formula (6) leads immediately to a better understanding of the relationship between weight and mass and to an extension of the concept of weight. Let M be the mass of the earth and let m be the mass of some other object. Since F is the force with which the earth attracts this object, we can regard F as the weight of the object, for this attractive force is what we have meant by weight. However, we now see that the force or weight depends upon the distance r between the two masses. Hence, the weight of an object is not really a fixed number but varies with the distance of the object from the earth or, more precisely, from the center of the earth (see Section 15–8). If an object of mass m is at the surface of the earth, its weight is given by
but the same object taken 1000 miles above the surface of the earth will have the weight:
The value F2 is considerably less than F1 because the denominator in the second expression is much larger. We see, then, that the farther an object of mass m is from the surface of the earth, the less is its weight. On the other hand, the mass of the object, that is its resistance to change in speed, is the same at all locations. Thus we can see more clearly that the weight and mass of an object are quite different properties.
The concept of weight can, and in the present scientific era must, be extended still further. So far we have considered the weight of an object to be the force with which the earth attracts the object. But now let us imagine that the object were taken to the moon and, for simplicity, let us suppose that no matter other than the moon and the object exist in space. May we speak of the weight of the object on the moon? The law of gravitation applies to moon and object, and so the moon will attract the object. This attractive force will be the weight of the object on the moon. To calculate this weight, we have but to let M in (6) be the mass of the moon, m, the mass of the object, and r, the radius of the moon. We know that the radius of the moon is 1080 miles or 1080 · 5280 feet. The mass of the moon can be determined by methods similar to those used later (Section 15–10) to compute the masses of the earth and sun. The result of the calculation, which we may accept for present purposes, is that the weight of an object on the moon is ![]() its weight on earth.
its weight on earth.
We can extend the notion of weight still further. Suppose that an object is in space somewhere between earth and moon. According to the law of gravitation, the earth attracts the object, and so does the moon. Since these attractions oppose each other, we may regard the weight of the object as the net attraction. If we now think of the object as moving from the earth to the moon, then the attractive force of the earth decreases while that of the moon increases. At the outset, the earth’s force is stronger, but at some point in the path to the moon the two forces will be equal and oppositely directed so that the net weight of the object will be zero. This point is located at a distance of about 24,000 miles from the moon along the line from the earth to the moon. All of the above considerations about weight are now no longer purely academic flights of fancy but are important factors in the process of determining the paths of rockets which are sent out to strike the moon.
1. Suppose that a person weighs 150 lb at the surface of the earth where, of course, his distance from the center of the earth is 4000 mi. What would this person weigh at a point 4000 mi above the surface of the earth?
2. How does the law of gravitation enable us to further differentiate between the mass and weight of an object?
3. Suppose that of two objects on the earth, one has twice the mass of the other. Show that the force with which the earth attracts the first one is twice the force with which the earth attracts the second.
4. What would a man whose present weight is 150 lb weigh if the earth’s mass were one-tenth of what it is?
5. Suppose that the earth’s mass were twice as large as it is. What change would there be in the acceleration of falling bodies? Would a body which is dropped from a height of 1000 ft reach the ground sooner than it now does?
6. It is stated in the text that all bodies near the surface of the earth fall with the same acceleration. Suppose that an object is several thousand miles from the surface of the earth. How would the acceleration of its fall to the earth compare with the acceleration of a body near the surface?
7. Suppose that the mass of the moon were the same as the mass of the earth. The radius of the moon is about ![]() the radius of the earth. What would a man who weighs 150 lb on the earth weigh on the moon?
the radius of the earth. What would a man who weighs 150 lb on the earth weigh on the moon?
8. The earth’s attractive force acts quite differently on objects in the interior of the earth than on objects outside the earth. In the former case the force is given by the formula F = GmMr/R3, where m is the mass of the object, M the mass of the earth, R the radius of the earth, and r the distance of the object from the center of the earth. Compare the variation of this attractive force as r varies, with the force given by formula (6).
9. Suppose that the law of gravitation were F = GmM/r instead of formula (6). Compare the variation of weight with distance from the center of the earth according to this formula with the variation of weight according to (6).
10. Consider all objects at a distance of 5000 mi from the center of the earth. Is the ratio of weight to mass the same for all these objects?
The essence of the scientific method created by Galileo and Newton is to establish basic quantitative physical principles and to apply mathematical reasoning to these principles. The law of gravitation and the first and second laws of motion are such physical principles. We shall see now that Newton was able to make some remarkable deductions from these principles.
The law of gravitation contains the constant G. Many calculations based on the law of gravitation require that one know G. In principle, this quantity is easily measured. One has but to take two known masses, place them a measured distance apart, and measure the force with which the two masses attract each other. Then, since
we see that every quantity in (9) is given except G, so that we have a simple algebraic equation for G. The actual experiments which have been made to measure G are a little more complicated because the force F is small for ordinary masses. However, the experiments have been performed, and the value of G turns out to be 1.07/109. The notation 109 is scientific shorthand for the product in which 10 occurs as a factor 9 times, i.e., one billion. This value of G presupposes that masses are measured in pounds, distances in feet, and forces in poundals (practical English system). (In the centimer-gram-sec-ond (cgs) system of units, G is 6.67/108.)
With the value of G known, it is a simple matter to calculate the mass of the earth. We may recall that formula (5), which is an immediate consequence of the law of gravitation and the second law of motion, states that the acceleration which the earth imparts to any other mass is
where r is the distance between the two masses. We have also learned that when r is 4000 miles or 21,120,000 feet, then a = 32. Let us substitute these values and the value of G in (10). Then
and we have obtained a simple equation for the unknown M. To shorten the somewhat complicated arithmetic, let us approximate and write 21,120,000 as 21,000,000 or as 21 · 106. Then by a theorem on exponents (see Section 5–3)
(21 · 106)2 = (21)2 · (106)2 = 441 · 1012.
Substituting the value just obtained in (11) yields
The factors 109 and 1012 can be combined, for the first factor means 10 · 10 · 10 · · · , wherein 10 occurs 9 times, and the second factor means that 10 occurs 12 times. Then in the product of these two factors 10 occurs 21 times. Hence
Multiplying both sides of this equation by 441 · 1021 and dividing both sides by 1.07, we obtain
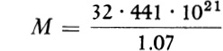
or
Since there are 2000 pounds in one ton, we may divide the right side by 2000 and write
Hence some simple algebra applied to formula (10) was all that was needed to calculate the mass of the earth. Let us note clearly that this quantity is not the weight of the earth. Technically the earth has no weight since weight is, by définition, the force which the earth exerts on other masses. However, a mass of 6.5· 1021 tons would weigh the same amount of tons, and so one can get some idea of the earth’s mass.
From the knowledge just obtained we can deduce some information about the interior of the earth. The earth is approximately spherical in shape, and since the volume, V, of a sphere is 477r3/3, where r is the radius, we can compute the volume of the earth. Thus
![]()
We shall approximate 2112 by 21 · 102 and use the value of 3.14 for π. Then
![]()
Since ![]() is about 39,000, we have
is about 39,000, we have
V = 39,000 · 106 · 1012 = 39 · 1021 = 3.9 · 1022 cubic feet.
We next divide the mass of the earth, in pounds, by the volume to find the mass per cubic foot. Thus
The mass per cubic foot of water is 62.5 pounds. We see then that the mass per cubic foot of earth is about 5.5 times the mass per cubic foot of water. This figure of 5.5, incidentally, is the density of the earth.
Examination of the earth’s surface shows that it consists mostly of water and sand. Since the quantity of rock visible on the surface does not account for the ratio 5.5, the conclusion follows that the interior of the earth must contain heavy minerals.
Only a little more work is required to compute the mass of the sun. We shall again begin with the law of gravitation and the second law of motion. The two masses involved now are the mass of the sun, S, and the mass of the earth, E. Then the law of gravitation states that the force with which the sun attracts the earth is
where r is the distance from the earth to the sun. According to Newton’s second law of motion, the force which the sun exerts on the earth gives the earth an acceleration a such that
Since the forces in (17) and (18) are the same, we may equate the right sides. Then
We may next divide both sides of (19) by £ and obtain
In this last equation we know G and r. If we knew a, the acceleration of the earth, we could calculate S. Let us see what we can do about calculating a.
The acceleration which the sun imparts to the earth causes the earth to depart from a straight-line path, which it might otherwise pursue, and “fall” toward the sun just enough to keep it on its elliptical path. (The acceleration which the earth imparts to the moon has the same effect on the lunar orbit.)
Fig. 15–4.
The sun’s pull on the earth causes the earth to “fall” the distance QR in t seconds.
We shall suppose, for the sake of simplicity, that the path of the earth is circular. Let us imagine that the earth is at the point P (Fig. 15–4) in its path around the sun. If there were no gravitational force, the earth would shoot straight out along the tangent at P into space in accordance with the first law of motion. Let us suppose that in time t, the earth would have reached the point Q. The distance traveled would be the velocity of the earth in its path around the sun, v say, multiplied by t. Hence PQ = vt. However, during that time t, the sun pulls the earth in a distance QR or d. Since SPQ is a right triangle,
(r + d)2 = r2 + (vt)2.
Squaring r + d and substituting the result, we obtain
r2 + 2dr + d2 = r2 + v2t2.
We subtract r2 from both sides of this equation and find that
2dr + d2 = v2t2.
Applying the distributive axiom on the left side permits us to write
Now d is the distance that the earth falls in time t. Let us suppose that it falls with constant acceleration. (We shall soon let t become very small so that the acceleration can well be taken as constant.) If a body falls a distance d with constant acceleration a, then we know from our work in Chapter 13 (Section 13–5, Exercise 14) that
![]()
or
Let us substitute this value of 2d in (21). Then
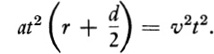
We now divide both sides of this equation by t2 and obtain
Thus far t was arbitrarily chosen, and d was the distance the earth fell toward the sun in time t. Our result so far, then, is valid for any value of t. If we now let t become smaller and smaller, d will also decrease. When £ = 0, it follows from (22) that d = 0. In this case, (23) becomes
ar = v2
or
This result states that the acceleration which the sun imparts to the earth at each point P of the earth’s path is the square of the earth’s velocity divided by the distance of the earth from the sun. This acceleration is called centripetal (i.e., center-seeking) acceleration, because it causes the earth to move toward the center of its path.
We now have the quantity a which we needed in (20). Substitution of (24) in (20) yields

We may multiply both sides by r and divide both sides by G to obtain
Every term on the right side of this equation is known. The distance r is 93,000,000 miles or 4.9 · 1011 feet. The velocity, vy of the earth is the circumference of the earth’s path divided by the number of seconds in one year:
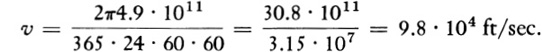
Hence
In Section 15–10 we learned that G = 1.07/109. Thus, using these values of r, v2, and G in (25), we have
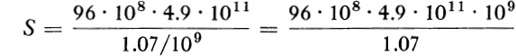
or
Hence the mass of the sun is 4.40 · 1030 pounds. Since- the earth’s mass was previously found to be 1.31 - 1025 pounds, we see that the mass of the sun is 3.36 · 105 or 336,000 times the mass of the earth.
We can determine the mass per cubic foot of the sun in the same manner as we calculated the mass per cubic foot of the earth. The mass of the sun is now known, and the radius, computed in Chapter 7, is 432,000 miles, or 2.28 · 109 feet. We shall not reproduce the calculations, but state the result: the mass per cubic foot proves to be 90 pounds. Since a cubic foot of water has a mass of 62.5 pounds, we see that the mass per cubic foot of the sun is about ![]() that of water; that is, the density of the sun is about
that of water; that is, the density of the sun is about ![]() .
.
The examples given in this section further illustrate how mathematical reasoning can be applied to physical laws (in our case, to the second law of motion and the law of gravitation) in order to deduce fundamental knowledge about the universe. We did, of course, also use some experimentally obtained facts such as the value of G and the acceleration of bodies near the earth’s surface. However, mathematics has been the main tool, and it obtains for us such remarkable information as the mass of the earth and the mass of the sun.
1. Suppose an object moves in a circle at a constant speed. Is the motion subject to an acceleration?
2. Does the formula for the acceleration of the earth given in (24) depend upon the law of gravitation?
3. If you whirl an object on a string of radius 5 ft, at the rate of 50 ft/sec, what is the centripetal acceleration acting on the object? What force exerts this centripetal acceleration?
4. Use formula (24) with the understanding that v is the velocity of the moon and r is the distance of the moon from the earth, to calculate the acceleration of the moon. (The period of the moon’s path around the earth is ![]() days, and the distance of the moon from the earth is 240,000 mi.)
days, and the distance of the moon from the earth is 240,000 mi.)
5. Using the figures in the text for the mass and radius of the sun, calculate the ratio of the mass to the volume of the sun.
We have repeatedly used the quantity 32 ft/sec2 as the acceleration which the earth gives to objects near its surface. This figure is perfectly satisfactory for most purposes, but it is not strictly accurate even for motions near the earth’s surface. Actually, the acceleration of falling bodies decreases from 32.257 ft/sec2 at either pole to 32.089 ft/sec2 at the equator.* The discovery of this decrease was at first not surprising to the seventeenth-century scientists. Newton had already proved that the earth is not strictly spherical, but has the shape of a somewhat flattened sphere (Fig. 15–5); that is, for example, the lengths O A, OB, OC, and OD are not equal, but are successively larger. Since the general formula for the acceleration due to gravity [see (5)] is GM/r2, where G and M are fixed and r is the distance from the center of the earth, this acceleration is less at C, say, than at B because r is larger at C than at B. Hence we should expect the acceleration due to gravity to decrease as the location varies from A to D. Now the values of G and M were known. Moreover, Newton and Huygens had computed lengths such as O A, OB, and so forth, and therefore were able to determine what the acceleration should be at points such as A, B, C, and D. These calculations based on the expression GM/r2, call for only a small percentage of the decrease actually measured. Thus precise measurement revealed a discrepancy between the acceleration predicted by the law of gravitation and the actual acceleration of falling bodies. This discrepancy required explanation.
Fig. 15–5.
The spheroidal shape of the earth.
The problem was solved by Huygens. Objects on the surface of the earth would fly off into space if the earth did not pull them toward the center, just as an object whirled at the end of a string would fly off into space if the hand at the center did not exert an inward pull. Thus the earth’s gravitational force has two effects. Even if the earth did not rotate, it would pull all objects toward the center, simply because the earth’s mass attracts the object. But since the earth does rotate, it must also exert an inward pull so that objects do not fly off into space but remain on or near the surface of the earth. This latter effect is a centripetal force. In a sense, the two effects of the earth’s gravitational force, that is, the force which causes objects to fall to the surface, or weight, and the centripetal force, are of the same nature. The centripetal force also pulls objects toward the earth’s center, but pulls them in just enough to keep them on a circular course. The weight, on the other hand, pulls objects toward the earth from the circular course to which they are kept by the centripetal force.
Let us express quantitatively what we have just described. By Newton’s second law, the centripetal force must produce an acceleration (centripetal acceleration) on the object. Now formula (24) gives the centripetal acceleration which the sun exerts on the earth. However, this formula is really quite general, that is, if we replace sun and earth in the argument which led to (24) by the earth and an object on or near the earth’s surface, then the argument still holds, provided that v is the velocity of the object and r is its distance from the center of the circle in which the object rotates. Newton’s second law then tells us that the centripetal force must be the mass of the object times the centripetal acceleration, that is mv2/r.
The force with which the earth pulls an object straight down, i.e., the weight, equals the mass of the object times the acceleration of its fall. It is this acceleration which we measure when we observe the fall of objects and which varies from pole to equator. We shall now denote it by g. Then the weight is mg.
According to Huygens the gravitational force which the earth exerts on objects supplies both the centripetal force and the weight. The centripetal force must be directed toward the center of the circle of latitude on which the object rotates. However, the weight is directed toward the center of the earth. Hence we cannot write a simple formula which expresses precisely how the earth’s gravitational force is apportioned to provide the centripetal force and the weight at any latitude. However at the extreme cases of latitude, that is, at the equator, and at the poles, the apportionment is simple. At the equator the centripetal force must be directed toward the center of the earth. If we denote the radius of the earth by R, then at the equator
At the North Pole, for example, an object does not travel in a circle as the earth rotates, and so no centripetal force is required to keep it rotating with the earth. Hence at that location,
Clearly g is larger in (29) than in (28).
What can we say about the apportionment at intermediate latitudes? Formula (28) is no longer correct because the circle on which an object rotates does not have radius R, but has a smaller radius. Also the velocity, v, of the object depends on its latitude. Moreover, as we have already noted, the direction of the centripetal force required to keep the object rotating with the earth must be directed toward the center of the latitude circle. The effect of all these factors is to decrease the centripetal force required to keep the object rotating with the earth as the latitude increases, and this force is zero at the poles. Hence more and more of the gravitational force is applied to the weight of the object, the quantity mg, and since m is constant, g increases from the equator to the poles. Almost the full increase in g is due to the rotation of the earth, the balance being due to the shape of the earth. We can turn our argument around. We observe that g increases from the equator to the poles. This increase can be explained by assuming that the earth rotates. Hence we have reason to believe that the earth rotates.
The numerical value of g, that is, the acceleration of falling bodies, has, of course, been of importance for centuries. But it has additional importance today. Let us consider a satellite which circles the earth once every hour. The circular paths of satellites do have the center of the earth as their center. Although the satellite moves at a height of a few hundred miles above the surface of the earth, we shall ignore this distance and suppose that it travels very near the surface. What is significant is that the satellite covers 25,000 miles per hour. Hence the centripetal force required to keep it in its path is considerably greater than that required to keep an object which travels 25,000 miles in 24 hours from flying off into space. We see this fact from the middle term in (28) which tells us that the centripetal force increases with the square of the velocity. Thus a great deal of the earth’s gravitational force must be expended in centripetal force. In fact, since the satellite does not fall to earth, the value of g, that is, the acceleration with which it should fall to earth, must be zero. In other words, the full gravitational force of the earth is expended in keeping the satellite on its circular path around the earth, and the satellite neither flies off into space nor falls to earth.
But the weight of any object is the product of its mass and the acceleration, g, with which gravity makes it fall to earth. Since for the satellite, g = 0, it follows that the satellite has no weight. Objects contained in the satellite would also be weightless and so would not experience any earthward pull.
In view of the importance which satellites are likely to have in future scientific investigations, it is desirable to know the velocity which a satellite must possess if it is to stay in orbit at some desired distance from the center of the earth. This velocity is readily calculated from (28). Since the satellite does not fall to earth, the value of g for it must be zero. Then
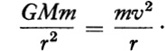
If we divide both sides by m and multiply both sides by r, we obtain
We know G and M, the mass of the earth. When r, the distance of the satellite from the center of the earth, is chosen, then we know all the quantities on the right side of (30). The quantity GM can be calculated once and for all. Thus
![]()
The value of r must be in feet. We can now calculate v.
1. Since the weight of an object is mg, how does a person’s weight change as he travels from the North Pole to the equator?
2. Suppose that a satellite stays close to the earth’s surface. How fast would it have to travel to stay on its circular path and not fall to earth? [Suggestion: Use formula (30).]
3. The moon is a satellite of the earth. Since the moon stays on its path and does not fall to the earth, we may conclude that the earth’s entire gravitational force acts as centripetal force on the moon. Using the assumption that the moon’s path is a circle and that it is 240,000 mi from the earth, calculate the velocity of the moon. [Suggestion: Use (30).]
4. Using the result of Exercise 3, calculate the time it takes the moon to make one complete revolution around the earth.
5. Calculate the speed required to maintain a satellite in an orbit 500 mi above the surface of the earth.
Thus far in this chapter we examined the evidence which convinced Newton that the law of gravitation was correct, and we have seen how it can be applied to answer a variety of questions about objects and motions on the earth and in the heavens. We should now recall that one of the major problems challenging seventeenth-century scientists was the question whether the same physical principles could account for terrestrial and celestial motions. Since the law of gravitation when applied to bodies falling near the earth’s surface reduces to fall with constant acceleration (see Section 15–8), Newton’s principles certainly encompassed earthly motions. As to heavenly motions, the three famous laws of Kepler, which he had inferred from observations, were seemingly independent of the law of gravitation. The truly great triumph of Newton was his demonstration that all three Keplerian laws were mathematical consequences of the law of gravitation and the two laws of motion.
We shall illustrate what Newton did by showing how the third Keplerian law can be deduced from the basic laws just mentioned. However, we shall simplify Newton’s work and suppose that the path of a planet around the sun is circular, whereas the true path, as Kepler proved, is an ellipse.
Let m be the mass of any planet, M the mass of the sun, and r the distance between them. Then the law of gravitation says that the force F exerted by the sun on the planet is
We also know that the sun’s force causes any planet to depart from straight-line motion and “fall” toward the sun with some acceleration. This acceleration, a, is none other than the centripetal acceleration given by formula (24), that is v2/r. The derivation of (24) dealt with the sun and earth, but it applies to any planet, provided that v is the velocity of the planet and r is its distance from the sun. We may also assert, by the second law of motion, that the centripetal force F with which the sun attracts that planet is
The velocity v of any planet is the circumference of its path divided by the time T of revolution around the sun; that is, v = 27πr/T. Hence, from (32),
Now formulas (31) and (33) yield two different expressions for the force with which the sun attracts any one planet.* Hence we may equate these two expressions and obtain
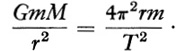
Dividing both sides of this equation by m eliminates that quantity. Multiplying both sides by T2, we obtain

If we now multiply both sides of this last equation by r2/GM, we find
The quantity 4π2/GM is the same no matter what planet is being considered, because G is a constant, M is the mass of the sun, and 4π2 is a constant. Hence formula (34) says that T2 is the product of some constant, say K, and r3; in symbols,
Thus the square of the time of revolution of any planet is a constant (i.e., the same for all planets) times the cube of that planet’s distance from the sun. Formula (35) is, then, Kepler’s third law of planetary motion. We have derived it from the two laws of motion and the law of gravitation by a purely mathematical argument.
As we remarked earlier, Newton demonstrated that all three of Kepler’s laws, which the latter had obtained only after years of observation and trial and error, were mathematical consequences of the laws of motion and gravitation. Hence the laws of planetary motion, which prior to Newton’s work seemed to have no relationship to earthly motions, were shown to follow from the same basic principles as did the laws of earthly motions. In this sense, Newton “explained” the laws of planetary motion. These facts were as much a consequence of basic physical laws as the straight-line motion of objects falling to earth from rest or of projectiles following parabolic paths. Newton’s original conjecture that the parabolic motion of projectiles should be intimately related to the elliptical motion of the planets was gloriously established. Further, since the Keplerian laws agree with observations, their derivation from the law of gravitation constituted superb evidence for the correctness of that law.
The few deductions from the laws of motion and gravitation which we have presented are just a sample of what Newton and his colleagues were able to accomplish. Newton applied the law of gravitation to explain a phenomenon which heretofore had not been understood, namely the tides in the oceans. He showed that these were due to the gravitational forces exerted by the moon and, to a lesser extent, the sun on large bodies of water. From data collected on the height of lunar tides, that is, tides due to the moon, Newton calculated the moon’s mass. Newton and Huygens calculated the bulge of the earth around the equator. Newton and others showed that the paths of comets are in conformity with the law of gravitation. Hence the comets, too, were recognized as lawful members of our solar system and ceased to be viewed as accidental occurrences or visitations from God intended to wreak destruction upon us. Newton then showed that the attraction of the moon and the sun on the earth’s equatorial bulge cause the axis of the earth to describe a cone over a period of 26,000 years instead of always pointing to the same star in the sky. This motion of the earth’s axis causes a slight change each year in the time of the spring and fall equinoxes, a fact which had been observed by Hipparchus 1800 years earlier. Thus Newton explained the precession of the equinoxes.
Finally Newton solved a number of problems involving the motion of the moon. The plane in which the moon moves is inclined somewhat to the plane in which the earth moves. He was able to show that this phenomenon follows from the interaction of the sun, earth, and moon under the law of gravitation. As the moon travels around the earth, it cuts the plane of the earth’s motion around the sun. The points in which it intersects are called the nodes. The nodes change in position, and this variation (regression of the nodes) also proved to be a consequence of the gravitational effect of the sun and earth on the moon. As the moon moves around the earth in an almost elliptical path, the point farthest from the earth, called the apogee, shifts about 2° per revolution. This effect, Newton showed, was due to the sun’s attraction. Newton and his immediate successors deduced so many and such weighty consequences about the motions of the planets, the comets, the moon, and the sea, that their accomplishments were viewed as “the explication of the System of the World.”
Today we have almost daily evidence that Newton had found sound physical principles which govern the operation of the universe. By applying just those principles man can now create satellites which circle the earth. In fact, Newton’s suggestion that projectiles shot out horizontally and with large velocities from the top of a mountain would circle the earth is, in essence, the one used to launch satellites. Strictly speaking, scientists do not operate from mountain tops because accessible peaks are not high enough to ensure that the satellite will clear other mountains, and because the air resistance at such altitudes is still considerable. Instead rockets project the satellite upward to a high altitude where the air resistance is negligible; there a mechanism turns the satellite to a horizontal direction and another rocket gives it a horizontal velocity. Then the satellite follows an elliptical path.
Newton went further in his speculations and conjectured that the planets must have been shot from the sun at some angle and, upon reaching their present distances, must have retained enough “horizontal” velocity to start moving in their elliptical paths around the sun. This conjecture is still the accepted theory of the origin of our solar system.
1. What reason would there be for calling Newton’s law of gravitation a universal law?
2. In what sense did Newton incorporate the Keplerian laws in his science of motion?
3. What support did the heliocentric theory receive from Newton’s work on gravitation?
4. What support did Newton’s principles derive from the heliocentric theory?
The work on gravitation presented mankind with a new world order, a universe controlled throughout by a few universal mathematical laws which in turn were derived from a common set of mathematically expressible physical principles. Here was a majestic scheme which embraced the fall of a stone, the tides of the oceans, the moon, the planets, the comets which seemed to sweep defiantly through the orderly system of planets, and the most distant stars. This view of the universe came to a world seeking to secure a new approach to truth and a body of sound truths which were to replace the already discredited doctrines of medieval culture. Thus it was bound to give rise to revolutionary systems of thought in almost all intellectual spheres. And it did. But, for the moment, we wish to confine ourselves to the implications and consequences of the theory of gravitation for mathematics proper.
Newton’s work followed and considerably broadened the plan laid down by Galileo, who proposed to find basic quantitative physical principles and to deduce from them the description of physical phenomena. Galileo had discovered and utilized such axioms as the first law of motion, the constant acceleration of bodies moving near the surface of the earth, and the independence of the horizontal and vertical motions of projectiles. His results were confined to terrestrial motions. Newton added to the axioms the second law of motion and replaced the principle of constant acceleration of falling bodies by the more general law of gravitation. He then found that the resulting set of principles enabled him to deduce the description of all motions of matter on earth and in the heavens. Thus the scientific method of Galileo and Newton involves mathematics not only in the expression of axioms and the laws which are deduced but also in the deductive process itself. Indeed, mathematics offered not merely the vehicle for scientific expression but the most powerful tool for the real work of science, that is the acquisition of knowledge about the physical world and the organization of that knowledge in coherent systems. From the time of Newton, these roles of mathematics have been unquestionably accepted and utilized. Hence, as the success of Newtonian mechanics spurred efforts in other physical domains, mathematics was confronted with new challenges and received new suggestions for the creation of concepts and methods which in turn gave greater power to science. This interaction of mathematics and science has grown immensely since its beginning in the seventeenth century and has become the outstanding feature of the intellectual life of our own century.
The most surprising development of the theory of gravitation and one which established a new and unanticipated role for mathematics took place after Newton had deduced a number of conclusions about our solar system. Galileo and Newton had set about finding quantitative laws that related matter, space, time, forces, and other physical properties, but had wisely decided not to look into causal relationships; that is, they had deliberately avoided such questions as why bodies fall to earth or why planets move around the sun. In other words, they had concentrated on description. Nevertheless, they did utilize the force of gravitation, a concept which had been vaguely suggested even before Galileo’s time—for example, by Copernicus and Kepler. Since the force of gravitation now assumed central importance, it was natural to ask, What is the mechanism that enables the earth to attract objects and the sun to attract planets? The heightened emphasis on this universal force could not but push such questions to the fore. The properties ascribed to the force of gravitation were indeed remarkable. It acted over distances of inches and millions of miles. It acted instantaneously and through empty space. Nor could the action of the force be suspended or blocked. Even when the moon was between the earth and the sun, the sun continued to attract the earth.
Although he tried to provide some physical explanation for the action of gravity, Newton did not succeed, and he concluded, “I have not been able to deduce from phenomena the cause of the properties of gravity and I frame no hypotheses.” In spite of his ignorance of the workings of gravitation, Newton insisted on adopting the laws of motion and gravitation. He says,
But to derive two or three general principles of motion from phenomena, and afterwards to tell us how the properties and actions of all corporeal things follow from those manifest principles, would be a very great thing though the causes of those principles were not yet discovered: and therefore I scruple not to propose the principles of motion above mentioned, they being of very general extent, and leave their causes to be found out.
Concerning his work in his Principles, he says,
But our purpose is only to trace out the quantity and properties of this force [gravitation] from the phenomena, and to apply what we discover in some simple cases as principles, by which, in a mathematical way, we may estimate the effects thereof in more involved cases; for it would be endless and impossible to bring every particular to direct and immediate observation. We said, in a mathematical way [note Newton’s emphasis on the mathematics], to avoid all questions about the nature or quality of this force, which we would not be understood to determine by any hypothesis;. . .
Newton was indeed troubled that he could give no explanation. But all he could do to justify the introduction of this force is summed up at the end of his Principles,
And to us it is enough that gravity does really exist, and act according to the laws we have explained, and abundantly serves to account for all the motions of the celestial bodies, and of our sea.
Contrary to popular belief, no one ever discovered gravitation, for the physical reality of this force has never been demonstrated. However, the mathematical deductions from the quantitative law proved so effective that the phenomenon has been accepted as an integral part of physical science. What science has done, then, in effect is to sacrifice physical intelligibility for the sake of mathematical description and mathematical prediction. This basic concept of physical science is a complete mystery, and all we know about it is a mathematical law describing the action of a force as though it were real. We see therefore that the best knowledge we have of a fundamental and universal phenomenon is a mathematical law and its consequences. And it has become more and more true since Newton’s days that our best knowledge of the physical world is mathematical knowledge.
1. Write as a decimal:
a) ![]()
b) ![]()
c) ![]()
d) ![]()
e) ![]()
2. Express each of the following numbers as a number between 1 and 10 multiplied or divided by a power of 10:
a) 58,000
b) 58,790
c) 63.4 · 103
d) 46.75
e) 0.05
f) 0.0074
3. Express each of the following quantities as a number between 1 and 10 multiplied or divided by a power of 10:
a) ![]()
b) ![]()
c) ![]()
d) ![]()
e) ![]()
f) ![]()
4. The frequency at which a frequency-modulation (FM) station broadcasts is 91 million cycles per second. Write the frequency as a number between 1 and 10 multiplied by a power of 10.
5. The mass of the earth is 13.1 · 1024 lb. A gram of mass is 0.002205 lb. Find the mass of the earth in grams.
6. The mass of the sun is 4.40 · 1030 lb. Use the data of Exercise 5 to compute the mass of the sun in grams.
In the following exercises you may use the fact that when M is the mass of the earth, GM = 32 · (4000)2(5280)2.
7. Calculate the acceleration which gravity imparts to an object
a) 2000 mi above the surface of the earth,
b) 10,000 mi above the surface of the earth.
8. Suppose a man weighs 200 lb at the surface of the earth. Calculate his weight when he is
a) 2000 mi above the surface of the earth,
b) 10,000 mi above the surface of the earth.
9. What does a man who weighs 200 lb on the earth weigh on the moon if the weight there is due only to the attraction of the moon. The acceleration which the moon imparts to objects near its surface is 5.3 ft/sec2.
1. The astronomical work of Copernicus. The books by Armitage, Dreyer, Koyre, Kuhn, Wolf, and any number of others listed in the Recommended Reading would be fine source material.
2. The astronomical work of Kepler. The books by Caspar, Dreyer, Koyre, Kuhn and Wolf listed in the Recommended Reading would be fine source material.
3. Show how the history of the heliocentric theory exemplifies the influence of mathematics on western European culture. The books by Kline in the Recommended Reading will provide material.
ARMITAGE, ANGUS: Sun, Stand Thou Still, Henry Schuman, New York, 1947. Also in paperback under the title The World of Copernicus.
ARMITAGE, ANGUS: Copernicus, W. W. Norton and Co., New York, 1938.
BAUMGARDT, CAROLA: Johannes Kepler, Life and Letters, Victor Gollancz Ltd., London, 1952.
BELL, E. T.: Men of Mathematics, Chaps. 6, 9, 10, and 11, Simon and Schuster, New York, 1937.
BONNER, FRANCIS T. and MELBA PHILLIPS: Principles of Physical Science, Chaps. 1 and 4, Addison-Wesley Publishing Co., Inc., Reading, Mass., 1957.
BURTT, E. A.: The Metaphysical Foundations of Modern Physical Science, rev. ed., Chap. 2 and pp. 202–262, Routledge and Kegan Paul Ltd., London, 1932.
BUTTERFIELD, HERBERT: The Origins of Modern Science, Chaps. 2 and 8, The Macmillan Co., New York, 1951.
CASPAR, MAX: Johannes Kepler, Abelard-Schuman, New York, 1960.
COHEN, I. BERNARD: The Birth of a New Physics, Chap. 7, Doubleday and Co., Anchor Books, New York, 1960.
DAMPIER-WHETHAM, WM. C. D.: A History of Science and Its Relations with Philosophy and Religion, pp. 160–195, Cambridge University Press, London, 1929.
DE SANTILLANA, GIORGIO: The Crime of Galileo, University of Chicago Press, Chicago, 1955.
DRAKE, STILLMAN: Discoveries and Opinions of Galileo, Doubleday & Co., Anchor Books, New York, 1957.
DREYER, J. L. E.: A History of Astronomy From Thaïes to Kepler, 2nd ed., Dover Publications, Inc., New York, 1953.
DREYER, J. L. E.: Tycho Brake, A Picture of Scientific Life and Work in the Sixteenth Century, Dover Publications, Inc., New York, 1963.
GADE, JOHN A.: The Life and Times of Tycho Brake, Princeton University Press, Princeton, 1947.
GALILEI, GALILEO: Dialogue on the Great World Systems, The University of Chicago Press, Chicago, 1953. Other editions of this work, originally published in 1632, also exist.
HALL, A. R.: The Scientific Revolution, Chap. 9, Longmans, Green and Co., Inc., New York, 1954.
HOLTON, GERALD and DUANE H. D. ROLLER: Foundations of Modern Physical Science, Chaps. 4, 5, 8 through 12, Addison-Wesley Publishing Co., Inc., Reading, Mass., 1958.
JEANS, SIR JAMES: The Growth of Physical Science, 2nd ed., Chap. 6, Cambridge University Press, London, 1951.
JONES, SIR HAROLD SPENCER: “John Couch Adams and the Discovery of Neptune,” in JAMES R. NEWMAN: The World of Mathematics, Vol. II, pp. 820–839, Simon and Schuster, Inc., New York, 1956.
KLINE, MORRIS: Mathematics: A Cultural Approach, Chapter 12, Addison-Wesley Publishing Co., Inc., Reading, Mass., 1962.
KLINE, MORRIS: Mathematics in Western Culture, Chap. 9, Oxford University Press, N.Y., 1953. Also in paperback.
KOYRE, ALEXANDRE: From the Closed World to the Infinite Universe, Chaps. 1 through 4, The Johns Hopkins Press, Baltimore, 1957.
KUHN, THOMAS S.: The Copernican Revolution, Harvard University Press, Cambridge, 1957.
MASON, S. F.: A History of the Sciences, Chaps. 17 and 25, Routledge and Kegan Paul Ltd., London, 1953.
MORE, LOUIS T.: Isaac Newton, Dover Publications, Inc., New York, 1962.
NEWMAN, JAMES R.: The World of Mathematics, Vol. I, pp. 254–285, Simon and Schuster, Inc., New York, 1956.
SMITH, PRESERVED: A History of Modern Culture, Vol I, Chap. 2 and Vol. II, Chap. 2, Holt, Rinehart and Winston, Inc., New York, 1934.
SULLIVAN, JOHN WM.N.: Isaac Newton, The Macmillan Co., New York, 1938.
TAYLOR, LLOYD WM.: Physics, The Pioneer Science, Chaps. 9, 10, and 13, Dover Publications, Inc., New York, 1959.
WIGHTMAN, WM. P. D.: The Growth of Scientific Ideas, Chaps. 8, 10, and 11, Yale University Press, New Haven, 1951.
WOLF, ABRAHAM: A History of Science, Technology and Philosophy in the Sixteenth and Seventeenth Centuries, 2nd ed., Chaps. 2, 3, 6, and 7, George Allen and Unwin Ltd., London, 1950. Also in paperback.
* The numerical values can, in principle, be obtained by measuring the accelerations with which bodies near the surface fall to earth. However, a more accurate method utilizes the formula for the period of a pendulum.
* In the light of Section 15–11 we can say that the gravitational force equals the centripetal force because the sun does not cause any planet to fall toward the sun from the circular path.