8
THE LIVES AND DEATHS
OF STARS (II)
MICHAEL A. STRAUSS
In this chapter, we’re going to explore the nature of stars in a bit more detail, building off what we learned in the last chapter. What makes an object qualify as a star? An astronomer defines a star to be a self-gravitating object that is undergoing nuclear fusion in its core. Self-gravitating means that it holds itself together by gravity. Earth also holds itself together by gravity. In fact, for an object as massive as Earth, the strength of gravity is actually much greater than the internal strength of rocks. We can see that by noticing that the shape of Earth is spherical, just like it is for stars. Gravity pulls everything together equally in all directions; the mark of an object held together by gravity is its tendency to be spherical. Smaller objects, such as asteroids, whose gravitational force is not as great, are held together by the tensile strength of their rocks or they are irregular rubble piles, often quite lumpy and elongated (figure 8.1).
But for a large massive object like the Sun, gravity is so strong relative to other forces that it compresses the mass into a spherical shape—the most compact configuration. If a large self-gravitating object is spinning rapidly, however, it will not be spherical; the spinning causes it to flatten. Isaac Newton himself understood this. Jupiter is spinning rather rapidly, and it is slightly elliptical as a result; its equatorial radius is about 7% larger than its polar radius. The most dramatic examples of flattened spinning objects are spiral galaxies, which we discuss in chapter 13.
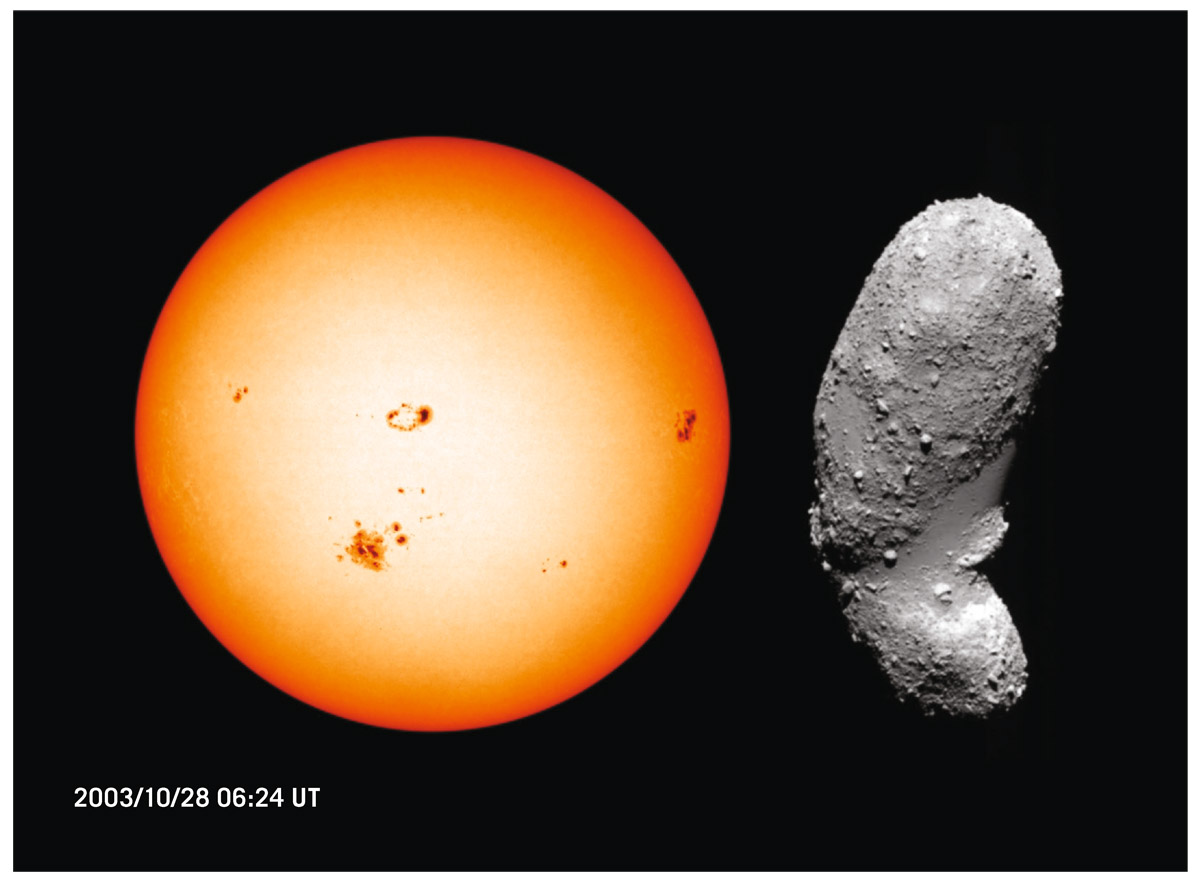
FIGURE 8.1. The Sun (left) and Asteroid 25143 Itokawa (right), not to scale, showing the different shapes of the two. The Sun, with a diameter of 1.4 million kilometers, is pulled into a spherical shape by its own gravity. Note the dramatic sunspots. The asteroid is only half a kilometer in diameter; its self-gravity is not adequate to make it spherical; it is thought to be a loose agglomeration of material that has accreted over time. The image of the Sun was taken by the Solar and Heliospheric Observatory (SOHO), a spacecraft dedicated to observing the Sun. The image of the asteroid was taken by the Hayabusa spacecraft launched by the Japan Aerospace Exploration Agency (JAXA). Photo credits: Sun: NASA, from http://sohowww.nascom.nasa.gov/gallery/images/large/mdi20031028_prev.jpg; Asteroid Itokawa: JAXA, from http://apod.nasa.gov/apod/ap051228.html
If the gas in a star is held together by gravity, what keeps all that gas from collapsing to a point? It is the internal pressure of the gas. Every parcel of gas feels gravity pulling it inward, and pressure pushing outward, with the two forces balanced in equilibrium.
An analogy is a balloon: it is not held together by gravity, but rather by the tension in the latex of the balloon. The balloon wants to shrink like a rubber band, but as in a star, the pressure of the air inside a balloon prevents it from shrinking. The air pressure and the tension are in equilibrium, and the balloon holds its spherical shape.
The gas pressure inside a star increases as you go to the center and decreases as you go out to larger radii. Gas pressure that decreases with larger radius is familiar here on Earth. Here at sea level, the atmospheric pressure is about 15 pounds per square inch; this represents the weight of the total column of air sitting above every square inch of Earth’s surface and extending to the top of the atmosphere. As you go up in Earth’s atmosphere, more and more of the atmosphere is below you, and the remaining column of atmosphere above you pressing down on you weighs less. The air pressure therefore decreases with altitude.
The pressure of the gas in a star is a reflection of its temperature and density, both of which increase dramatically as you go toward the center.
Now let’s go to the core. We can’t observe the core directly, but we can infer its properties by writing down the equations of stellar structure, which include the effects of pressure and gravity. These equations incorporate the observation that the Sun is in equilibrium, with gravity and pressure balanced throughout the star. These calculations show that in the very center of the Sun, the temperature is 15 million K, as we have discussed. This calculation also reveals the density at the center to be about 160 grams per cubic centimeter, 160 times denser than water. For comparison, the densest naturally occurring element on Earth is osmium, at 22.6 grams per cubic centimeter (about twice as dense as lead). With its enormously high temperature, the gas in the core of the Sun is ionized, meaning that the electrons are stripped from atoms, and the nuclei and electrons are zipping around at high speed—this is called a plasma. It is the pressure of these particles moving at high speed that resists gravity, keeps the Sun from collapsing, and holds it in equilibrium.
We’ve already seen that a basic property of material at a given temperature is that it emits photons. This is true for the center of the Sun as well, at 15 million K. The blackbody spectrum of an object at this temperature peaks at X-ray wavelengths. Does this mean that the Sun is shining brightly in X-rays? No. Consider an X-ray photon emitted from the center of the Sun. Can it make its way unimpeded from the center? When you go to the doctor’s office to get an X-ray; they shield those parts of your body they don’t want to X-ray with a blanket containing lead. Thus, a fraction of an inch of lead, with a density of a measly 11.34 grams per cubic centimeter, will absorb any X-rays that hit it. If that’s all it takes to absorb X-rays, you can imagine that the X-rays coming from the center of the Sun are not going to get very far. In fact, they travel only a fraction of a centimeter before they are absorbed.
The energy of an absorbed photon has to go somewhere, however. It heats up the material that absorbed it, which will then radiate blackbody radiation—more X-rays to be re-emitted. So you can think of our little photon as getting absorbed and getting reradiated over and over again. When you work through all the numbers, the time it takes for energy generated in the center in the Sun to propagate to the surface is about 170,000 years. The distance from the center to the surface is just 2.3 light-seconds, so if that photon could travel unimpeded, it would take only 2.3 seconds to go from the center of the Sun to its surface. But because the photon is getting jostled around, it makes a random, drunken walk, being absorbed and re-emitted as it slowly wanders out from the center of the Sun.
The original photon in the center is an X-ray photon, emitted by gas at 15 million K. Will it still be an X-ray photon when it reaches the surface? No; each time the energy is re-emitted, it is in the form of photons appropriate for the temperature at that position in the star. As the energy makes its way from the center to the surface, the temperature drops, and individual photons lose their identity. The energy becomes distributed among photons of lower energies, corresponding to a lower temperature. So even though X-rays are produced in the center, we don’t see X-rays from the surface. X-rays are slowly degraded down to mere visible light photons, the kind that we see coming from the surface of the Sun.
If there were no nuclear furnace in the center of the Sun to keep the center hot and the pressure up, the Sun would slowly start to shrink under the influence of gravity as it lost energy radiated from its surface. This gravitational shrinkage, as the envelope of the star falls toward the center, would generate energy, in exact analogy to the way a piece of chalk gathers speed, and thus kinetic energy, as it falls to the floor. That gravitational energy of contraction would, by itself, be enough to keep the Sun shining at its current luminosity for about 20 million years. Before Einstein, Hermann von Helmholtz (in 1856) hypothesized that this slow gravitational contraction was actually the source of energy powering the Sun. This was plausible at the time, since nuclear fusion was unknown and would not be discovered for another 82 years. This mechanism implied that the Sun had been shining as it is today for 20 million years at most. But we now know, from dating via radioactive isotopes (e.g., noting how much uranium in particular rocks has decayed into lead), that Earth is several billion years old. Moreover, fossils demonstrate that Earth’s surface temperature has remained at least approximately constant for a significant portion of that time. Thus, the Sun has been shining more or less as it does at present for much longer than 20 million years, and the gravitational contraction hypothesis for the Sun’s energy generation cannot be true.
With the understanding of the significance of E = mc2, all was answered. The Sun burns nuclear fuel in its center, providing energy. This nuclear energy generation balances the luminosity given off by the Sun and maintains its internal pressure. Thus the Sun is stable and does not contract. Nuclear fusion is so efficient in generating energy that the Sun has shone steadily for the past 4.6 billion years, giving life on Earth a long period of stable conditions over which to evolve. The Sun is now about halfway through its main sequence lifetime.
By the way, how do we measure the basic properties of the Sun: its radius, mass, and luminosity? To measure the Sun’s radius, we go through a series of steps. We have known the radius of Earth since the time of the Greek mathematician and geographer Eratosthenes, about 240 BC. Every year at noon on June 21, the Sun passed directly overhead at Syene, Egypt. Eratosthenes knew this fact. At that same moment, he measured the Sun to be 7.2° off vertical in Alexandria, which is directly north of Syene. Aristotle had argued that Earth, no matter what its orientation, always casts a circular shadow on the Moon during an eclipse of the Moon. The only object that always casts a circular shadow is a sphere; thus, Eratosthenes knew that Earth must be a sphere. He also understood that the 7.2° shift in the altitude of the Sun, as measured from the two cities at the same time, was due to the curvature of Earth’s surface, meaning that the two cities were separated by 7.2° of latitude, or about 1/50 of Earth’s entire 360° circumference. Hire someone to pace off the distance from Alexandria to Syene, multiply by 50, and you have the circumference of Earth—about 25,000 miles. Divide by 2π and you have the radius. It was easy, once someone figured out how to do it!
From different observatories widely separated on Earth’s surface, we get slightly different parallax views of Mars against the distant stars. Knowing the radius of Earth and measuring that parallax shift allows us to measure the distance to Mars. Giovanni Cassini was the first to do this. Kepler’s work allowed us to plot the orbits of planets to scale—to make a scale model of the solar system. Once you had that one Earth–Mars distance, you could deduce the size of all the orbits, including the radius of Earth’s orbit, the Astronomical Unit. Thus, Cassini in 1672 determined that the distance from Earth to the Sun was about 140 million kilometers—not far from the true value of 150 million kilometers.
We know the angular size of the Sun (about half a degree across) as seen from Earth, and knowing the distance to the Sun, we can determine the Sun’s radius. It is equal to the angular half-diameter of the Sun in degrees (1/4°) divided by 360°, multiplied by 2π times the distance to the Sun. The radius of the Sun is about 700,000 kilometers, about 109 times larger than the radius of Earth. The luminosity of the Sun is also straightforward: we can measure its brightness as seen from Earth, and now that we know its distance r, the inverse-square law allows us to determine its luminosity—about 4 × 1026 watts.
We can also determine the mass of the Sun. Newton’s laws allow us to figure out the ratio between the mass of Earth and that of the Sun. We know the acceleration Earth produces at a distance of one Earth radius (i.e., here on Earth’s surface), GMEarth/rEarth2 = 9.8 meters per second per second, which we can determine by watching apples drop. We also know the acceleration the Sun produces at a distance of 1 AU: GMSun/(1 AU)2 = 0.006 meters per second per second, which we have already calculated in chapter 3. Take the ratio of these two accelerations: 0.006 meters per second per second/9.8 meters per second per second = 0.0006 = [GMSun/(1 AU)2]/[GMEarth/rEarth2] = (MSun/MEarth)(rEarth/1 AU)2. Plugging in the known values for the radius of Earth and 1 AU, and solving, we find that the Sun has a mass of about 330,000 times that of Earth. Because the constant G factors out of the ratio, we don’t have to know it to find the ratio of the mass of the Sun to the mass of Earth.
But what is the mass of Earth in kilograms? We could solve for its mass using the equation for the acceleration of gravity at the surface of Earth, 9.8 meters per second per second = GMEarth/rEarth2, if we only knew the numerical value of Newton’s constant G. Henry Cavendish —who discovered hydrogen, the most abundant element in the universe—did a clever experiment to find the value of G. He used a torsion pendulum to measure the ratio of the force exerted on a test ball by Earth and the force exerted on it by a nearby 159 kilogram lead ball. Earth pulled the test ball downward, and the nearby heavy lead ball pulled it to the side, and he could compare the forces they exerted by measuring the angle of deflection produced in the pendulum. Knowing the distances to the nearby lead ball and to the center of Earth, and using Newton’s laws, he determined the ratio of the mass of Earth and that of the lead ball. This enabled Cavendish in 1798 to determine the value of Newton’s constant G and find the mass of Earth in kilograms. Multiply by 330,000, and you have the mass of the Sun. It turns out to be 2 × 1030 kilograms. That’s a lot!
We’ve been focusing on the Sun here, but we’d like to understand the nature of other stars as well. Just as we use the orbit of Earth around the Sun to determine the Sun’s mass using Newton’s laws, we can use observations of pairs of stars (“binary stars”) orbiting each other to determine their masses.
The lowest-mass stars on the main sequence (which are M stars) have a mass about 1/12 of that of the Sun. What about stars that are even lower in mass? With lower gravity, they will have lower temperature and lower density in the core. What happens when you get a gaseous mass held together by gravity that is simply not hot enough in its center for nuclear fusion of hydrogen to take place? Such a star we call a brown dwarf (they are not really brown, but actually appear very red and glow mainly in the infrared; sometimes astronomical nomenclature can be a bit misleading). They exist but are hard to find. Such stars are glowing feebly by the residual heat from their gravitational collapse (just as Helmholtz had imagined for the Sun); they have no significant internal nuclear furnace and are of low luminosity. They are also cool, with surface temperatures ranging from 600 K to 2,000 K, and thus their radiation is coming out mostly in the infrared rather than the visible part of the spectrum. By comparison, your oven at home gets up to 500 K. (A little known fact: 574° F is also 574 K—the crossing point of these two temperature scales.)
Most of our most powerful telescopes are sensitive to visible light, and only in the past few decades have we built telescopes that can survey the sky in infrared light (which turns out, for all kinds of technical reasons, to be quite a bit more difficult). It’s only been with the advent of powerful telescopes that are sensitive to infrared light that astronomers have even been able to find such objects.
The O, B, A, F, G, K, and M classes of stars have been around for about 100 years, but since 1999, as we have discovered brown dwarfs, we have added two new classes: L and T stars. Even more recently, an infrared satellite called the Wide-Field Infrared Survey Explorer has discovered still cooler stars, classified as Y stars, with surface temperatures as low as 400 K, just a bit above the boiling point of water. Brown dwarfs with masses between 1/80 and 1/12 of the Sun (i.e., between about 13 and 80 times the mass of Jupiter) feebly burn the trace amount of deuterium that exists in their cores. Thus, since they do have some nuclear burning in their cores, they are still are called stars. Objects of still lower mass, less than 13 times the mass of Jupiter, will have absolutely no nuclear fusion of any sort in their cores. We call such objects planets!
Let’s consider the death of stars in greater detail than we did in chapter 7. Even during its late main sequence phase, the Sun will gradually increase in luminosity, and Earth’s oceans will boil away about a billion years from now. This will represent the end of life as we know it on Earth. Roughly 5 billion years from now, when no more hydrogen remains in the Sun’s core (having all been turned into helium), the Sun’s nuclear furnace turns off, and the pressure that’s been holding up the star against gravity drops. Gravity wins, and the star will start to collapse. But recall that it takes a couple of hundred thousand years for energy generated in the core to make its way out to the surface. The inner parts of the star will start to collapse, even as energy is still flowing through the outer parts of the star, holding them up. The outer parts of the star have a couple of hundred thousand years before they get the bad news that the energy source at the center of the Sun is gone.
Consider the hydrogen shell immediately surrounding the (now pure helium) core. Outside the core, there is still plenty of hydrogen, but that region has so far been uninvolved in nuclear fusion, as its density and temperature are simply too low. As this shell of hydrogen collapses, however, it becomes hotter and denser. Very quickly, its density and temperature get high enough to trigger the fusion of hydrogen to helium in the shell. We have a new source of fuel to run the nuclear furnace: hydrogen burning in a shell.
Suddenly, the star has a new lease on life. The rate of energy production in the hydrogen-burning shell is enormous—much higher than that of the core while the star was still on the main sequence. Furthermore, the volume of the hydrogen-burning shell is much larger than that of the core.
And so, for a brief period at least, the star produces a huge luminosity, but it takes a long time for that radiation to get out, and the increased pressure starts winning the tug-of-war with gravity. As a consequence, the outer parts of the star expand (and cool somewhat), even while the inner parts contract. The Sun becomes a red giant, as discussed in chapter 7. Outside the hydrogen-burning shell, the outer parts of the star have expanded to an enormous radius, about 1 AU (or 200 times the current radius of the Sun). About 8 billion years from now, tidal interactions with the Sun during its red-giant phase will probably cause Earth to spiral into the envelope of the Sun and burn up.
While the star’s hydrogen shell is burning, its helium core has no internal energy source; gravity causes it to continue to contract and thus heat up. When the temperature reaches about 100 million K in the core of the star, the helium nuclei start fusing to make carbon and oxygen nuclei. That helium-burning phase will last for about 2 billion years for the Sun, but eventually, the helium in the core will all be depleted, and the core begins to collapse again.
For stars of the mass of the Sun, we’re near the end of our story. The outer parts of the star are far away from the core, and thus feel only a weak gravitational pull. It takes only a bit more energy to eject the outer parts of the star, which gently expand as a diffuse gaseous envelope, revealing the hot dense carbon-oxygen core of the star left behind. This ejected gas is excited by the ultraviolet light of the central star, causing it to fluoresce, and making a nebula like the Dumbbell Nebula pictured in figure 8.2. Such objects are called, confusingly, planetary nebulae, because the first astronomers to spy them through telescopes thought they looked something like planets, and the name has stuck ever since. Astronomers have a nostalgic tendency to keep names for things even when they are outmoded and misleading.
This extended envelope of material, which used to be part of the star itself, is now gently expanding outward. Stars sometimes blow off their outer layers in complex ways, giving rise to planetary nebulae with multiple shells of gas around them. Different layers are coming from different depths inside the star, which may be enriched in different elements. Rotation of the original star can cause the layers to be blown out preferentially along the spin axis, as occurs in the Dumbbell Nebula (see figure 8.2).
The now-exposed glowing core of the star is visible in the very center of the nebula. It is small (about the size of Earth) and hot enough to appear white; we thus call it a white dwarf. The white dwarf has no internal source of energy, and thus slowly cools off over billions of years. We still call a white dwarf a star, even though it is not burning nuclear fuel. (I admit it: this nomenclature is not totally consistent!)
What holds up the white dwarf against gravitational collapse? The Pauli exclusion principle, named for physicist Wolfgang Pauli, states that no two electrons can occupy the same quantum state. This is key for understanding the structure of atoms. In atoms having many electrons, the electrons must stack up into higher energy levels when the lower energy levels are filled. For white dwarfs, the Pauli exclusion principle means that the electrons don’t like to be squeezed too close together; this gives rise to a pressure that holds the white dwarf up against gravity. Our Sun will end its life as a white dwarf.
As described in chapter 7, stars more than 8 times as massive as the Sun go through a much more dramatic series of reactions. There’s enough mass in their cores for the carbon and oxygen, which would otherwise sit inertly while the star quietly becomes a white dwarf, to instead heat up enough to fuse into such elements as neon, silicon, and the others all the way up the periodic table to iron.

FIGURE 8.2. The Dumbbell Nebula. This is a red giant star that has ejected its outer layers, revealing its hot dense core. The core is a white dwarf star, seen at the center, while the outer layers are fluorescing as a planetary nebula from the ultraviolet light that the white dwarf emits. Photo credit: J. Richard Gott, Robert J. Vanderbei (Sizing Up the Universe, National Geographic, 2011)
The outer layers of these more massive stars grow appreciably larger than mere red giants. They become red supergiants, with radii of several AUs.
In the night sky, some bright stars are clearly red to the naked eye. Red stars that are on the main sequence have a low luminosity; none are visible to the naked eye. In contrast, a red giant is large, has a huge luminosity, and can be seen out to a great distance. All the bright red stars in the sky are either red giants (like Arcturus in the constellation Boötes and Aldebaran in Taurus) or red supergiants (like Betelgeuse in Orion).
Scientists overuse the prefix super-. We stick it in front of just about everything, because we keep discovering or making things that are even bigger or more spectacular than what we knew before: supernovae, supermassive black holes, and, of course, the never-completed particle accelerator known as the superconducting supercollider! The most famous red supergiant in the sky is Betelgeuse (pronounced “Beetlejuice”). It has a radius about 1,000 times as large as the Sun’s and has a mass of at least 10 solar masses. In its core, helium is burning into carbon, oxygen, and heavier elements. Outside the core is a thin shell of essentially pure helium that is not hot or dense enough to burn yet, so it’s sitting there more or less quiescent. Outside that is a shell of hydrogen burning into helium, and outside that, the vast majority of the volume of the star, is a huge extended envelope of hydrogen and helium.
This story of the evolution of stars after the main sequence was worked out in detail in the 1940s and 1950s, as people came to understand the detailed nuclear physics that takes place in the cores of stars and were able to use the first computers to solve the relevant equations of stellar structure. Much of this work was done at Princeton University, led by Professor Martin Schwarzschild. Neil, Rich, and I had the opportunity to interact with him in his later years; he was a wonderful man.
Figure 8.3 shows Schwarzschild with Lyman Spitzer and Rich Gott. When Henry Norris Russell (of HR diagram fame) retired as chair of Princeton University Observatory in 1947, he brought in two young astronomers, Martin Schwarzschild and Lyman Spitzer, both in their early thirties. Spitzer, who became chair of the department, went on to develop much of our modern understanding of the interstellar medium (the gas and dust between the stars) and founded the Princeton Plasma Physics Laboratory, where scientists are working to harness nuclear fusion as a source of energy. Spitzer will always be known to the public as the father of the Hubble Space Telescope, having developed the initial concept and working for decades to convince the astronomical community and the US Congress that it should be built. Spitzer and Schwarzschild were the core of the Princeton Astrophysics Department for the next 48 years. They passed away within 11 days of each other in 1997, quite a shock to all of us.
In the 1950s, Schwarzschild and his students worked out all the details of the story I am describing. He was one of the first people who understood the whole story of the evolution of stars. Martin’s father, Karl Schwarzschild, played a key role in the study of black holes; his name will come up again in chapter 20.
Continuing our story of stars, the pressure of electrons prevents a white dwarf from collapsing. However, if the mass of the star’s core is more than 1.4 solar masses, even this pressure is not large enough to hold it up against gravity. Compressed by gravity, the electrons and protons are pushed together to form neutrons (releasing electron neutrinos in the process). This leaves us with a neutron star—actually a giant atomic nucleus of mostly pure neutrons. The Pauli exclusion principle holds for neutrons as it does for electrons, and the resulting neutron pressure now supports the star against gravity. However, as neutrons are much more massive than electrons, the equilibrium size of a neutron star (about 25 kilometers) is much smaller than that of a white dwarf. Imagine more than a solar mass of material squeezed into a volume the size of Manhattan Island (or 100 million elephants in a thimble, from chapter 1)! Neutron-star matter is the densest material we know of. The center of a neutron star can have a density of almost 1015 gm/cm3.

FIGURE 8.3. Left to right: Lyman Spitzer, Martin Schwarzschild, and Rich Gott in the 1990s.
Photo credit: Collection of J. Richard Gott
If the core of a massive star is more massive than about 2 solar masses, the neutron star that tries to form is unstable to further collapse; the neutron pressure is inadequate to hold the star up against gravity, and a black hole forms. Whether the core collapses to a neutron star or a black hole, the infalling material compresses violently, triggering further nuclear reactions (remember that the material outside the core is still made up of elements lighter than iron). The energy that is suddenly released can eject the entire exterior of the star above the core, causing a supernova explosion. Stars with initial masses on the main sequence greater than about 8 solar masses die by exploding as supernovae, forming either neutron stars or black holes in the process. Massive exploding stars are called Type II supernovae, as there is another type of stellar explosion. Consider three stars orbiting each other, two of which are white dwarfs. Gravitational interactions between them can cause two white dwarf stars to collide. The heating due to the collision detonates their nuclear fuel and produces a supernova. Alternatively, a red giant star in a binary system can transfer mass onto a white dwarf star, pushing it over the limit of 1.4 solar masses and causing it to collapse, and a supernova ensues. Such explosions are called Type Ia supernovae, to distinguish them from the explosions of massive collapsing stars: we’ll return to them briefly in chapter 23, as they become important tools to help us measure the accelerating expansion of the universe.
In any case, in a supernova explosion, gas flies outward in all directions. This is not a gentle process like the slow wafting away of the outer parts of a planetary nebula. Rather, it is an extremely violent explosion. Most or all of the star is destroyed in the explosion, and material is sent out at speeds approaching 10% of the speed of light. Heavy elements produced in the core of the star are now returned to the interstellar medium, ready to be included in the next generation of stars and planets.
In 1054, Chinese astronomers noticed a new star in the constellation we call Taurus. The ancient Chinese were careful observers of the sky, looking for portents of future events, so they were particularly impressed by this “guest star,” which was visible for many weeks and was initially bright enough to be seen during the day. Interestingly, there are no records in any European manuscripts whatsoever that anyone living there had seen this thing, despite it being the brightest object in the sky for weeks on end. Perhaps it was cloudy for that entire period in Europe, or any written European accounts were lost, or maybe the Chinese astronomers were just paying much more attention to what was going on in the sky.
Images of the Crab Nebula in Taurus (figure 8.4) taken a few decades apart clearly show that it is expanding. Given the observed rate of expansion and its current size, we can work out when the expansion must have started; the answer is about a thousand years ago, just about the time the Chinese observed their “guest star.” Thus the Crab Nebula, located in exactly the same region of the sky that the Chinese records describe, is certainly the remnant of the supernova they discovered. In a few hundred thousand years more, this gas will have become so diffuse as to be essentially invisible, its enriched gases having mixed with the interstellar medium.

FIGURE 8.4. The Crab Nebula. This is the expanding remnant from a supernova explosion (seen on Earth in 1054 AD).
Photo credit: Hubble Space Telescope, NASA
In the center of the Crab Nebula, a rapidly spinning neutron star was discovered, rotating 30 times a second. When a star collapses, it retains its angular momentum and begins spinning more rapidly, like an ice skater pulling in her arms. Its magnetic fields become compressed and intensified as well. The magnetic field at the surface of the Crab Nebula’s neutron star is about 1012 times stronger than the magnetic field at the surface of Earth. As the neutron star rotates, its north and south magnetic poles swing around, and the neutron star emits radio waves in two beams like a lighthouse. Every time the lighthouse beam swings past Earth, we see a pulse of radio radiation. Thus, the neutron star is called a radio pulsar. The first radio pulsar was discovered by graduate student Jocelyn Bell in 1967. It had a rotation period of 1.33 seconds. Her thesis advisor Antony Hewish was awarded the Nobel Prize in Physics for the discovery. I find it outrageous that she did not share in the prize.
The Crab Nebula pulsar emits electromagnetic radiation over the full electromagnetic spectrum, from radio wavelengths all the way to gamma-ray wavelengths. The pulsar can be seen blinking rapidly 60 times a second (as each of the two lighthouse beams sweep by us) in visible light as well, but astronomers never noticed that until after its radio pulses were discovered. It just looked like a faint star at the center of the Crab Nebula. The Crab Nebula is about 6,500 light-years away, which means that the explosion really occurred about 5445 BC, but it took the light until 1054 AD to reach us.
Recall the inverse-square law. The nearest star system is Alpha Centauri, 4 light-years away. The Crab Nebula is very much farther away, yet the supernova was much brighter than any star we see in the night sky, being easily visible in the daytime. At its peak luminosity, it was about 2.5 billion times as luminous as the Sun.
Supernovae are rare. The last time a supernova is known to have gone off in the Milky Way was about 400 years ago, before Galileo first pointed a telescope at the heavens. Thus, in 1987, astronomers were particularly excited when they saw a supernova explode in the Large Magellanic Cloud, a small satellite galaxy of the Milky Way. This was the closest supernova to go off in modern history. It was bright enough to be seen with the naked eye, even though it was 150,000 light-years away. I was lucky enough to travel to Chile to use telescopes there for my PhD research in May 1987; it was very exciting (and quite easy) for me to see this “new” star in the Large Magellanic Cloud.