16

QUASARS AND SUPERMASSIVE
BLACK HOLES

MICHAEL A. STRAUSS

In the 1950s, radio astronomy, the study of electromagnetic radiation that astronomical objects emit at wavelengths longer than a centimeter or so, was still in its early days. The radio telescopes of the day were making the first maps of the sky. It was a challenge to determine which astronomical objects were responsible for the radio sources seen, because the radio telescopes did not have the resolution to pinpoint accurately the position of the radio source on the sky. That is, they could specify the position of any given source only to the nearest degree or so, and it wasn’t at all obvious which of the thousands of stars and galaxies lying in that region of sky were responsible for the radio emission.

The best radio maps of the sky at the time were made using a radio telescope in England; the Cambridge University astronomers who ran the survey published several catalogs of sources found in these maps. Our story starts with the 273rd entry in the third of the Cambridge catalogs, called, for short, 3C 273. The Moon’s path on the sky occasionally passes over 3C 273, and by timing exactly when this radio source disappeared behind the Moon, astronomers were able to pinpoint its position to much greater accuracy. Astronomers then took images of that region of the sky in visible light, to see what was responsible for the radio emission. To their surprise, 3C 273 coincided with what appeared to be a star, one too faint to be seen by the naked eye, but certainly bright enough to be easily studied with what at the time was the largest visible-light telescope in the world, the 200-inch telescope at Palomar Observatory. Maarten Schmidt, a young professor at Caltech in Pasadena, knew that to understand what sort of star it was, he needed to measure its spectrum. He obtained the spectrum with the 200-inch telescope in 1963, but when he first looked at the data, he couldn’t make sense of what he saw.

He saw a series of very broad emission lines whose wavelengths did not correspond to any atoms he had ever seen before. His first thought was that this might be some really unusual type of white dwarf star, but then he had an “aha!” moment. He realized that the emission lines were just the familiar Balmer lines of hydrogen, which form a regular pattern well known from studies of stars. However, these lines were not at their familiar wavelengths, but were all shifted systematically to the red by an astonishing 16% (figure 16.1). That is, the wavelength of each of these features in the spectrum was 16% larger than the Balmer transitions observed here in the lab on Earth.

FIGURE 16.1. The spectrum of the quasar 3C 273. The strongest emission lines present are Balmer lines of hydrogen, as marked. In each case, the arrow is drawn from the rest wavelength to the observed wavelength of the line—shifted redward in each case by 15.8%. The other emission lines apparent in the spectrum are due to oxygen, helium, iron, and other elements. Credit: Michael A. Strauss, from data taken by the New Technology Telescope at La Silla, Chile; M. Türler et al. 2006, Astronomy and Astrophysics 451: L1–L4, http://isdc.unige.ch/3c273/#emmi, http://casswww.ucsd.edu/archive/public/tutorial/images/3C273z.gif

Could this be a redshift due to the expansion of the universe? A redshift that large corresponds (using the modern value of the Hubble constant) to a distance of about 2 billion light-years. A small number of galaxies known at the time had similarly large redshifts, but they were incredibly faint, at the limit of what telescopes were capable of measuring. Yet 3C 273 was several hundred times brighter than these faint fuzzy galaxies. Moreover, it appeared starlike, a point of light, not having an extended size like a galaxy. This left two interpretations: (1) perhaps this object was much closer than 2 billion light-years—even within our own galaxy—and the redshift had nothing to do with the expansion of the universe, or (2) this star was enormously luminous. The inverse-square law tells us that for 3C 273 to be as bright as observed, if it were really at a distance of 2 billion light-years, it would have to be hundreds of times more luminous than an entire galaxy containing 10¹¹ stars!

Maarten Schmidt told his colleague Jesse Greenstein about his discovery. It turned out that Greenstein had measured the spectrum of another radio source, 3C 48; Greenstein immediately realized that this must be a similar object, at an even higher redshift of 0.37 (or 37%). Schmidt mused that many such objects must be out there to discover, and that he better get busy finding them. As he and others discovered more of these starlike radio-emitting objects with ever-larger redshifts, they needed a name for them. The first term they used was quasi-stellar radio source, but this was too much of a mouthful, and it was quickly shortened to quasar. While the first quasars were all found by their radio emissions, Allan Sandage (famous for his measurements of the Hubble constant) soon discovered similar starlike objects at high redshifts that had no associated radio emission; the majority of quasars in fact are faint in the radio part of the spectrum.

Fritz Zwicky, whom we met in chapter 12, was a colleague of Schmidt and Greenstein at Caltech. He was one of the most brilliant, and eccentric, characters in twentieth-century astronomy (figure 16.2). He made a series of discoveries so far ahead of their time that the rest of the scientific community took decades to catch up with him. We’ve already seen that in 1933, he was the first to infer the existence of dark matter from the motions of galaxies in clusters. The idea only took hold in the astronomical community in the 1970s, when Morton Roberts and Vera Rubin and her colleagues started measuring the rotation of the outer parts of galaxies, and Jeremiah P. Ostriker, Jim Peebles, and Amos Yahil began using stability arguments to infer the existence of large amounts of dark matter in galaxies. Zwicky and his colleague Walter Baade hypothesized (correctly!) in 1934 that neutron stars can form in supernova explosions, an idea that was confirmed only three decades later with the discovery of pulsars. In fact, Zwicky and Baade coined the word supernova. Zwicky also predicted correctly, decades ahead of the observations that would confirm it, that Einstein’s light-bending effect in general relativity could make distant galaxies act like gravitational lenses, magnifying even more distant galaxies behind them. And he claimed that he was the first to discover quasars.

FIGURE 16.2. Fritz Zwicky, posing with his catalogs of galaxies. Photo credit: Archives Caltech

Zwicky knew he was smart, and wasn’t shy about expressing his views when he thought others were mistaken. Denied access to the 200-inch Palomar telescope, Zwicky did most of his work with a small, 18-inch survey telescope at Palomar, using it to discover supernovae (he found more than 100 of them in his lifetime) and make catalogs of galaxies. He noticed that some of the galaxies he tabulated were quite compact, almost appearing starlike. But because he was not allowed to observe on the 200-inch, he wasn’t able to measure spectra of these galaxies and determine their physical nature. Some of the compact galaxies he had noticed turned out later to be quasars of the type that Schmidt and Sandage had subsequently discovered, and Zwicky claimed—with some justification—that he should be given credit for their discovery.

The graduate students at Caltech loved Zwicky, who shared office space with them in the sub-basement of the astronomy building on the Caltech campus. Zwicky passed away in 1974: my colleagues Jim Gunn, who was a graduate student at Caltech in the 1960s, and Rich Gott, who was a postdoc there from 1973 to 1974, remember him fondly.

Zwicky’s basic insight was correct. Some compact galaxies had an incredibly luminous unresolved pointlike source of light (the quasar) coming from the center of the galaxy, which outshone the faint parts of the galaxy surrounding it, making the galaxy itself appear almost pointlike, like a star.

This phenomenon is clearly seen in images of quasars taken with the Hubble Space Telescope: its sharp images can distinguish the light from the quasar and the faint extended light around it from the galaxy in which it sits. These images were taken by my wife Sofia Kirhakos, in collaboration with her colleagues John Bahcall and Don Schneider, so I am particularly pleased to show them in this book (figure 16.3). In the center of each image is a very bright point of light; that’s the quasar itself. It is surrounded by a galaxy (and in one case, a pair of galaxies that appear to be colliding): spiral arms are visible. Images such as these resolved the distance controversy: quasars really are at the distances their redshifts imply (they are not just a weird type of star in our own Milky Way galaxy), and thus they are incredibly luminous.

FIGURE 16.3. Quasars in their host galaxies, taken by the Hubble Space Telescope.

Photo credit: J. Bahcall and M. Disney, NASA

To understand what the quasar phenomenon is all about, let us return to the spectrum of 3C 273. The emission lines here are broad, spread over a range of wavelengths, even though we learned in chapter 6 that atomic transitions correspond to specific, precise energies and thus wavelengths. We understand this as a manifestation of the Doppler shift: within the quasar, there is gas moving at a range of speeds. The quasar overall is moving away from us at 16% of the speed of light, but relative to that overall motion, some of the gas in the quasar is moving toward us (blue shifting part of the emission line relative to the average), whereas some of the gas is moving away from us (making part of the emission line even more red shifted). This broadens or widens the emission line. Consider this emission to be from gas in orbit around a central mass: there is gas at every point along a circular orbit, and each of these points has a different component of motion along the line of sight, and thus a different Doppler shift. The broad emission line reflects this range of Doppler shifts.

We can take this one step further. The width of the emission line tells us how fast the gas is moving; a typical value for quasars is 6,000 km/sec. Something is causing the gas to move at this enormous speed. We will hypothesize that these motions are due to gravity—that this is gas moving in orbit around some central object, whose nature we would like to understand.

What is the radius of this orbit? If we can determine this, then we can use Newton’s Laws and our knowledge of the speeds to calculate how massive that central object must be. We’ve already seen that quasars appear pointlike, like a star, and therefore they are smaller than what our telescopes can resolve. A clue to their true sizes became available when people discovered that quasars are variable; their brightness changes significantly on timescales of a month or so.

Imagine that the light from a quasar was coming from a region a light-year across. The light that reaches us from the front side of the quasar (as seen by us) would arrive a year earlier than the light from the back. Even if the whole structure were somehow to double in luminosity instantaneously, the brightness we would detect would brighten gradually over the course of a year, as first the light from the front side, then eventually the back side, reached us. Thus, the fact that quasars change their brightness on timescales of a month tells us that they can’t be much bigger than a light-month in size. This size is astonishingly small: remember that stars in our Milky Way are separated from one another by several light years, and this volume a light-month across (or even smaller) is emitting as much energy as several hundred ordinary galaxies.

We now know the speed of the gas moving in the quasar, and roughly how far it is from whatever is causing it to move gravitationally. We can carry out the same calculation we did in chapter 12 when determining the mass of the Milky Way from the orbit of the Sun around it: the mass is proportional to the velocity squared times the radius. When we do this calculation for the quasar, we find a mass of an astonishing 2 ×10⁸ times the mass of the Sun.

Let us summarize: quasars are found in the centers of galaxies, they are a light-month or smaller in diameter, they have luminosities hundreds of times larger than entire galaxies, and have masses hundreds of millions times the mass of the Sun. Huge masses in a tiny volume: could this be a black hole? And yet, black holes are supposed to be black—light cannot escape from them—whereas quasars are among the most luminous objects in the universe. In addition, the only way we know how to make a black hole is to collapse a massive star. The most massive stars we know of are perhaps 100 times the mass of the Sun; we can’t make a 200-million-solar-mass black hole that way. What is going on?

Well, black holes can grow in mass. Consider gas falling toward a black hole. If it is going straight in, it will simply be swallowed by the black hole and disappear without a trace, adding to its mass but otherwise having no effect. However, it is more likely that the gas has a bit of sideways motion, or angular momentum, relative to the black hole. Because of this angular momentum, it will not fall straight in but will orbit around the black hole. In analogy to stars orbiting in the Milky Way, we think that the gas around a black hole lies in a flattened rotating disk. The gravity of a black hole is strong; the gas closest to the black hole is moving tremendously fast, at an appreciable fraction of the speed of light. The gas that is closer to the black hole will have a higher velocity and rub against the gas a little farther out. This friction can heat the gas up tremendously, to temperatures of hundreds of millions of degrees. And as we’ve seen over and over again, hot things radiate energy.

So while the black hole itself is invisible, the gas around it, before it falls all the way in, can be tremendously luminous. A quasar is a supermassive black hole, surrounded by a disk of gaseous material glowing so hot that it can outshine the entire galaxy in which it is embedded. Indeed, it is material falling in during this process that can cause a relatively small black hole, born presumably from the death of a massive star as a supernova, to grow: as material falls in, the disk material shines as a quasar and continually adds to the mass of the black hole. The quasar is powered by gravitational energy turned into kinetic energy as the gas spirals deeper and deeper into the gravitational well of the black hole. As the gas finally enters the black hole, it adds to the black hole mass. This accretion process, operating over hundreds of millions of years, can result in black holes with masses of millions, or even billions, of solar masses.

The tremendous energy associated with the disk close to the black hole causes energetic particles to be emitted. These particles are blocked by the disk itself, and thus must spurt out as a jet of material perpendicular to the disk, entrained in part by powerful magnetic fields. Such a narrow jet is seen as the faint linear feature at 5 o’clock in figure 16.4, a Hubble Space Telescope picture of 3C 273 (the sharp, straight spikes emanating from the quasar itself are artifacts of the telescope optics).

Such jets are the hallmark of black holes into which material is falling. The elliptical galaxy M87 has one of the most massive black holes in the nearby universe, 3 billion times as massive as the Sun. It is also emitting a jet, about 5,000 light-years in length.

FIGURE 16.4. Quasar 3C 273 and its jet.

Photo credit: Hubble Space Telescope, NASA

There is a popular mental image of black holes as cosmic vacuum cleaners, slurping up everything in their vicinity. However, imagine that the Sun turned magically into a black hole (of the same mass) tomorrow. This would of course be terrible news for us, because we would no longer receive heat and light from the Sun and Earth would freeze. But Earth’s orbit would remain unchanged. The angular momentum of Earth in its orbit around the Sun will keep us circling, as we have for the past 4.6 billion years. Similarly, stars in orbit around the black hole in the center of the Milky Way are not going to be swallowed up by that black hole any time soon. This black hole probably went through a quasar phase in the distant past, when it grew to its current size of 4 million solar masses. We can measure its mass today by plotting the orbits of individual stars we see orbiting around it. However, there is no material falling into it now to form a disk, so it is currently quiescent and is not shining as a quasar.

Quasars are rare in the nearby universe. Indeed, 3C 273, 2 billion light-years away, is one of the nearest luminous quasars. Quasars were much more common in the early universe; most quasars are at high redshift, and therefore at great distances. The light from these distant quasars has traveled for billions of years to reach us. We are thus seeing them at a time when the universe was significantly younger than it is today. The fact that the number of quasars in the universe has changed with time is direct evidence for an evolving universe, contradicting Hoyle’s perfect cosmological principle (see chapter 15), which endorsed an unchanging universe.

From the numbers of quasars we see in the early universe, we predict that supermassive black holes in the present-day universe must be ubiquitous. After all, black holes only grow; they don’t go away once they’ve formed. (We’ll see in chapter 20 that black holes can eventually evaporate due to quantum effects, but for supermassive black holes, this process is slow indeed, and is quite negligible over the billions of years we’re discussing here.) The fact that we don’t see these black holes shining as quasars in nearby galaxies today simply tells us that they are currently quiescent, without gas falling into them. The supermassive black hole in the center of our Milky Way, whose presence we inferred from the motions of stars in its vicinity, is just one such example.

Looking for black holes in the centers of other galaxies is a challenge. If the black hole is not being fed by gas coming in from an accretion disk, there will be no quasarlike emission for us to see. However, we can use the Doppler shifts of stars near the centers of galaxies to infer the presence of a massive gravitating object. This is done most easily for nearby galaxies, for which we can resolve the central regions, where the gravity of a black hole will dominate the motions of stars.

Astronomers have now searched in detail for black holes in about 100 galaxies. In essentially every case that they had the sensitivity to detect it, they did find evidence for a supermassive black hole in the center. As far as we can tell, essentially every large galaxy with a significant bulge (i.e., ellipticals and most spirals) hosts a black hole. Our Milky Way, with a black hole of a mere 4 million solar masses, is a relative wimp; the most massive black holes among the nearby galaxies are several billion times more massive than the Sun (as we saw for M87). Moreover, the larger the elliptical galaxy (or the bulge of the spiral galaxy), the more massive the black hole will be; the mass of the black hole is typically about 1/500 of the mass of the bulge of stars in which it sits.

The tremendous luminosities of quasars make them much brighter than galaxies. Thus a distant quasar is much brighter, and therefore easier to see, than a galaxy at the same distance. What is the most distant quasar we can see in the universe? Again, because of the finite speed of light, the light we see from such a distant quasar left it when the universe was much younger than it is today. When we look at objects at large distances in astronomy, we are looking into the past: our telescopes are time machines.

In chapter 15, I described the Sloan Digital Sky Survey, which has taken images of the sky and measured redshifts for 2 million galaxies. It has also obtained spectra of more than 400,000 quasars. From this sample, we know that quasars were most common between 2 and 3 billion years after the Big Bang; this is when the supermassive black holes found in big galaxies today are thought to have gained most of their bulk. Two billion years after the Big Bang, about 12 billion years ago, corresponds to a redshift of 3. That is, the spectral lines in the quasars appear with wavelengths 4 (i.e., the redshift + 1) times the wavelength they would have without the expansion of the universe. Redshift, in this case, is not a subtle phenomenon but a big effect!

Edwin Hubble found a linear relationship between redshift and distance for galaxies. At very large redshifts, this relationship is somewhat more complicated; it turns out that a quasar at redshift 3 is now about 20 billion light-years from Earth. How can this be, if the Universe is only 13.8 billion years old? Remember that in the time since the light left the quasar until now, the universe has expanded fourfold (again, redshift + 1), carrying the quasar farther away, and this distance of 20 billion light-years corresponds to where it is now (we call this its co-moving distance).

Figure 16.5 shows the spectrum of the most distant quasar my colleagues and I found in the Sloan Digital Sky Survey. The very strong emission line at a wavelength of 9,000 Ångstroms (0.9 microns) corresponds to the transition from the second energy level to the ground state in hydrogen—the Lyman alpha line. Blueward of this emission line (i.e., in the blue direction, at shorter wavelengths), the spectrum drops to zero; this turns out to be due to absorption from hydrogen gas distributed in the volume between the quasar and us. The spectrum shows emission at near-infrared wavelengths and essentially nothing at shorter wavelengths, making this object appear tremendously red.

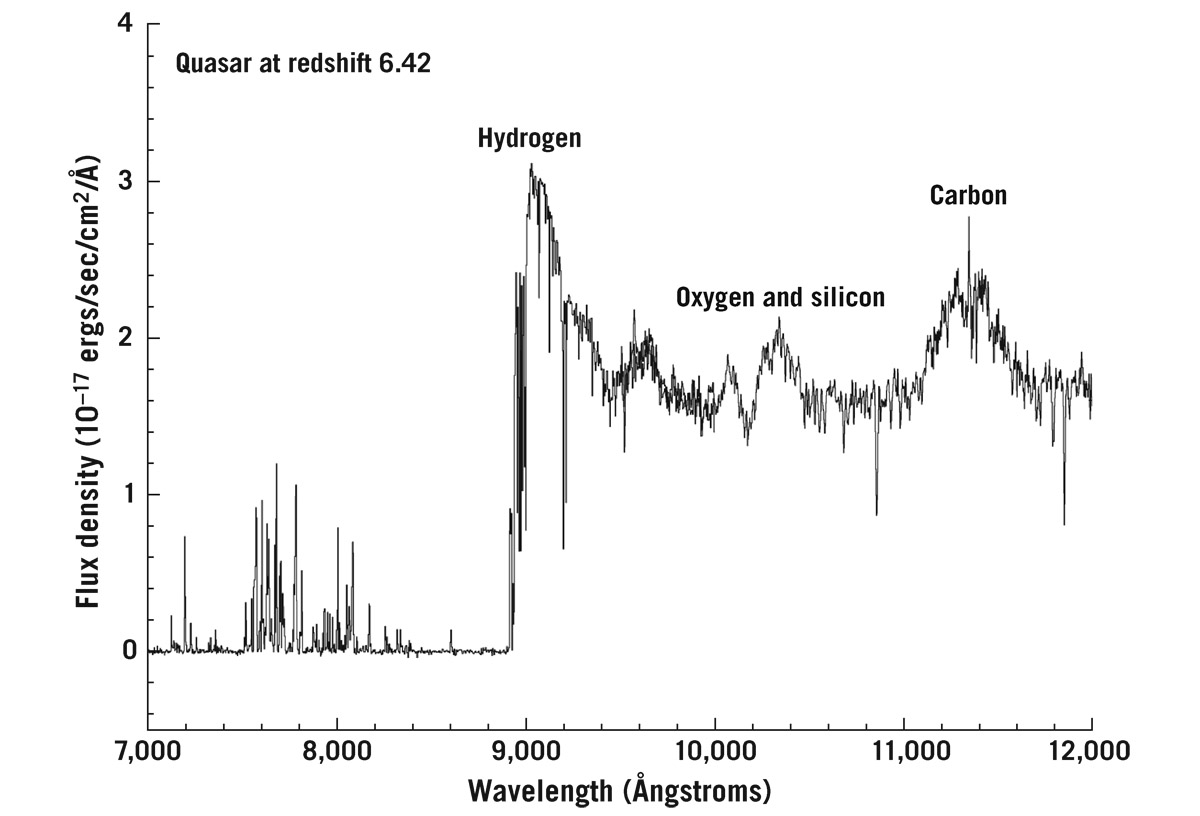
FIGURE 16.5. Spectrum of the quasar SDSS J1148+5251 at redshift 6.42. This quasar was discovered by Michael Strauss, Xiaohui Fan, and their colleagues in 2001, the highest-redshift quasar known from the time of its discovery until 2011. The light we are seeing from this quasar was emitted when the universe was less than 900 million years old. The strongest peak (emission line) in this quasar is due to emission from hydrogen atoms (the n = 2 to n = 1 transition; see figure 6.2), which has been greatly redshifted from its rest wavelength of 1,216 Ångstroms to 9,000 Ångstroms. The sharp drop in the spectrum below 9,000 Ångstroms is due to absorption from hydrogen gas between the quasar and us. Credit: Image by Michael A. Strauss using data in R. L. White, et al. 2003, Astrophysical Journal 126: 1, and A. J. Barth et al. 2003, Astrophysical Journal Letters 594: L95

Thus the job of finding the highest-redshift quasars is straightforward: look in the Sloan Digital Sky Survey images for the reddest objects you can find. This is not as easy as it sounds; the survey includes images of about half a billion objects, and we had to make sure that an apparent red color for any given object had not resulted from some sort of rare processing glitch.

There is another challenge. We know from our studies of stars that the cooler the star the redder it appears. In 1998, as the first images from the Sloan Digital Sky Survey became available, my student Xiaohui Fan and I started a program of obtaining spectra of the reddest objects we could find in the data, to confirm their quasar nature and to determine their redshifts. We used the Apache Point Telescope (at the same observatory where the Sloan Digital Sky Survey telescope is located, in Sunspot, New Mexico). The telescope is remotely operable over the internet: rather than flying across the country, we could simply eat an early dinner at home, then drive into the office, where we would carry out our observations, sending instructions for moving a telescope 2,000 miles away.

As we started measuring spectra of these very red objects, we hit pay dirt almost immediately, but in an unexpected direction. Mixed in with an assortment of high-redshift quasars, we stumbled across some of the coolest (and thus lowest-mass) stars known, right here in our Milky Way. Indeed, these are the substellar objects discussed in chapter 8, with masses too low to burn hydrogen in their cores. These stars have temperatures of 1,000 K or even lower, and their spectra were quite unfamiliar to us when we first started finding these objects. I remember scrambling to look up the few papers that had described such cool stars at 3 in the morning, as we measured the spectra and struggled to understand them. In a single night of observing, we would take spectra both of the lowest-luminosity substellar objects known, at a distance of only 30 light-years, and enormously luminous quasars close to the edge of the observable universe. This is the most extreme illustration of the fact that with an astronomical image alone, we have no depth perception. The very nearby (in astronomical terms) and extraordinarily distant objects both appeared as very faint red points in our data, requiring detailed spectra to distinguish the two.

We continued pushing to ever-redder objects, as our techniques for removing glitches in the images improved. We broke the existing quasar redshift record (4.9 at the time we started our work) multiple times. Whenever we did so, we would call our colleague Jim Gunn (the project scientist for the Sloan Survey and a pioneer in quasar studies in his own right). Waking him out of a deep sleep (it was usually 3 a.m. or so, after all!), we’d say, “Jim, we broke the record again!” “Good work, boys,” he would respond; “I always want to be woken up for this news!” And then he would go back to sleep.

The Lyman alpha hydrogen line apparent in the spectrum of our most distant object in figure 16.5 is normally found at a wavelength of 1,216 Ångstroms; here it has been redshifted all the way into the near-infrared part of the spectrum at 9,000 Ångstroms. The redshift is (9,000 Å – 1,216 Å)/1,216 Å, or 6.42, corresponding to a distance now of 28 billion light-years. This was the highest redshift quasar known when we discovered it in 2001. Perhaps even more impressive than its great distance is the fact that the light we see from this object left it about 13 billion years ago, when the universe was only about 850 million years old. If the CMB radiation comes from the universe’s infancy, we’re now probing a time when it was a toddler.

This brings up another cosmic mystery. As noted earlier, we can use a quasar’s spectrum to estimate the mass of the black hole powering it. A typical value for the most distant quasars is about 4 billion solar masses, about as massive as the largest black holes we know of in the present-day universe. But remember that the smoothness of the CMB tells us that the very early universe was almost perfectly uniform. From this almost complete absence of structure, we have to form supermassive black holes, the densest conceivable objects, in only 850 million years. To make such a black hole, the universe had to form a first generation of stars, then explode them as supernovae, leaving behind stellar-mass black holes. These black holes then had to accrete matter at a tremendous rate to acquire such an enormous mass. Theoretical models suggest that this is barely possible under ideal conditions, implying that such high-redshift quasars should be rare. Indeed they are; after more a decade of searching, we’ve found only a few dozen quasars at the very highest redshifts.

The push for the most distant quasars continues: in 2011, our record was broken in spectacular fashion with the discovery of a quasar at redshift 7.08, using a survey that was sensitive to longer wavelengths (further into the infrared) than the Sloan Survey. Since the time when this quasar emitted the light we are seeing today, the universe has expanded by a factor of 8.08. Other teams are using the Hubble Space Telescope, the Subaru Telescope in Hawaii, and other telescopes to find galaxies at higher redshifts still. It remains unclear whether models for galaxy formation and black hole growth will be able to explain these and future discoveries, if the redshift records continue to be broken. There should be interesting times ahead!

The wonderful thing about astronomy is that every time we look at the heavens in a new way, we make fundamental new and unanticipated discoveries. The Sloan Digital Sky Survey, whose discoveries have been prominent in this chapter and chapter 15, is a good example of this. I am currently involved in planning for its successor, The Large Synoptic Survey Telescope, currently under construction on a mountaintop in the Chilean Andes. It will have a much larger light-gathering power than the Sloan Telescope, and in its 10-year survey lifetime, it will study the properties of faint galaxies and quasars, map the distribution of dark matter from the gravitational lensing distortions it causes in the shapes of galaxies, and discover hundreds of thousands of supernovae and other transient phenomena. The telescope will be making a movie of a quarter of the entire sky: 860 complete frames in 10 years. This will require us to process 30 terabytes of new data every day. The survey should discover hundreds of thousands of Kuiper Belt objects, and also spot Earth-approaching asteroids. But the most exciting discoveries are likely to be those we haven’t even imagined yet, the “unknown unknowns,” in Donald Rumsfeld’s famous phrase.