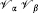Index

Page numbers in italics refer to exercises

(0, 2) compactification 324–6, 376, 472

(2, 0) (tensionless string) theory 204–5

(2, 2) compactification

Calabi–Yau 311–12, 318–19, 323–5

Gepner models 394–402

of heterotic strings 386–90

constraints on spacetime action 388–90, 473

of type II strings 379–85

orbifold 292, 297

A–D–E singularity 425

A_n−1 see SU(n)

action (D-brane) 149–50

D0-brane 161

Dp-Dp′ system (#_ND = 4) 163–4, 172

action (general)

Pontrjagin term 173, 187, 333

action (spacetime)

anomaly-cancelling terms 99–100, 130

d = 4, N = 1 supergravity 446, 474

d = 4, N = 1 supersymmetry 443, 474

d = 4, N = 4 Yang–Mills theory 443

d = 10, N = 1 Yang–Mills theory 443

eleven-dimensional supergravity 85, 453, 468, 474

F⁴ term 116, 132

and Born–Infeld action 152, 469

and duality 193–4

from (2,2) compactification 473

heterotic string 388–90

type II string 381–4

from Calabi–Yau compactification 315–20, 404–6, 472

higher corrections 321–4

from orbifold compactification 472

threshold corrections 298–300

twisted states 296–8

untwisted states 292–6

in N = 1 heterotic string vacua 359–62, 473

p-form gauge field 451–2

R⁴ term 117

R–R tadpole term 39

singularities in 411

type I supergravity 92–4

type I–heterotic relation 93, 192

type IIA supergravity 87–8

massive 89–90

type IIB supergravity 90–2

SL(2, R) symmetry 91, 181–2

action (world-sheet)

BC SCFT 4, 106

heterotic string 49

Landau–Ginzburg 270, 392

linear dilation 5

linear sigma model 398–9

N = 2 free SCFT 48

nonlinear sigma model 106–7

superfield form 105–7

superstring 2

WZNW 247–50

affine Lie algebra 66

annulus, see cylinder

anomalies 94–103, 468

anomaly polynomial 97–101, 374

cancellation for SO(32) and E₈ × E₈ 99–101

gauge 95ff

global gravitational 34

gravitational 95ff

in six dimensions 417, 420

in two dimensions 94–6, 107

mixed 95ff

see also Green–Schwarz mechanism

anti-de-Sitter space 223

antisymmetric tensor gauge field, see p-form gauge field

Atiyah–Drinfeld–Hitchin–Manin (ADHM) construction 174

auxiliary field 442–4

axion 79, 286, 315, 373–4

field (a) 293–4

and strong-CP problem 333–5

bc CFT, bosonization 15

BC SCFT 4–5

B_k see SO(2k + 1)

βγ CFT 15–20

bosonization 17–20, 118–19

BPS bound 449

D0–Dp system 168

F-string–D-string system 164, 176, 182

BPS states 449, 474

behavior at strong coupling 180–1

black p-branes 183, 220

bound states 164–75

D-branes 140–5

Kaluza–Klein states 456

macroscopic strings 81, 226, 468

stretched strings 186–7, 226

winding states 79–80

wrapped branes 404, 411–12

zero-force property 146

BRST charge 15–16, 24, 43, 50

and picture changing 118–21, 125

in topological string theory 385

BRST quantization 24–5, 43, 45, 50

beta function

and scale transformation 261, 264–6

for gauge coupling 298, 346, 367

vanishes for d = 4, N = 4 460

nonlinear sigma model 247, 321

Betti numbers 305, 308

Bianchi identity

Chern–Simons term in 86, 88, 91

for R–R vertex operators 88–9

in Calabi–Yau compactification 304, 324, 418

related to field equation by Poincaré duality 141, 451–2

black hole

entropy 219–25, 227, 470

information paradox 225–6

produced in graviton–graviton scattering 209

black p-brane 183–5, 201, 219–20, 227, 470

blow up (a fixed point) 297, 309–11, 388, 471

bosonization 11–15, 279–80, 467

and refermionization 131–2

bc CFT 15

βγ CFT 17–20, 118–19

current algebra fermions 54–5, 74–5

higher genus 13

kink operator 12

of U(1) current 68

Ramond states 13–15

twisted fermions 13–14

bound states

D0–D0 168–9, 469

and M-theory 198

and matrix theory 212–14

D0–D2 169–70

U-dual to FD-string 189

D0–D4 170–5, 176, 469–70

and instantons 172–5

U-dual to winding string 189

D0–D6 175

D1–D5 black hole 220–3

F-string–D-string 164–7, 176, 469

SL(2, Z) duality 182

U-dual to D0–D2 state 189

C invariance 330–1

C_k see Sp(k)

c-map 382

CP invariance 331, 333–5, 472

CPT invariance 331–2

c-theorem 262–3

and supersymmetry multiplets 441–2, 447

Calabi–Yau compactification 302–24, 472–4

and Gepner models 397–402

as special case of (2, 2)

compactification 311–12, 386–90

field theory approximation 315

low energy field theory 315–20

massless spectrum 312–15

of type II string 379–86

Calabi–Yau manifold 309–12

Casimir energy 145

Casimir invariant 60

central charge (conformal) 3–5, 15, 43

and BRST nilpotence 24

and density of states 222, 236

and rank of gauge group 421

and renormalization group 262–6, 271

in heterotic string 49–50

in rational CFT 258–9

in topological string theory 385

in unitary CFT 228–33

of ghosts 46–8

specific

coset models 250–4

current algebra 70–2

N = 0 minimal models 231

N = 1 minimal models 254–5

N = 2 minimal models 391

superconformal current algebra 340–2

WZNW 247–8

central charge (supersymmetry) and BPS states 449, 456

from dimensional reduction 454, 456–7, 466

in extended supersymmetry 448–9

in heterotic string 79–82

in type I/II string 144–5, 475

central extension 67

Chan–Paton degrees of freedom 30

for type I D5-brane 195–7

reinterpreted as D-brane label 141–2

Chan–Paton factor 73, 109, 468

character 235–6, 245–6

charge conjugation matrix C 434

Chern class (first) 309, 366, 402

Chern–Simons 3-form 92, 100

and modified gauge transformation 92, 107, 469

Chern–Simons terms 86, 453

and anomaly cancellation 99–100

from string perturbation theory 113–14, 127–30

in D-brane action 150

and induced R–R charge 170, 173, 203

chiral gauge couplings 282, 325, 330

constraints from 337, 340–2, 351–2, 447–8

in (2,2) models 386–8

in Calabi–Yau models 313

in orbifold models 288–9, 291

chiral multiplet 315, 441–2

chiral primary fields 378–9, 384–5

in Landau–Ginzburg models 394–5, 397

chiral ring 384–95, 473

chiral superfields 442–7

chirality

and anomalies 96–8, 101

effect of T-duality on 137–8

IIA vs IIB 27–9, 415–16, 453–4

matrix (Γ) 10, 432

of NS5-branes 204

Christoffel connection 106

Clifford (Dirac matrix) algebra 2, 193, 430

cocycle 19–20, 83

cohomology

and homology 306, 317

BRST 25, 385–6

De Rham 305

Dolbeault 307

of K3 416–17

ring 385

Coleman–Weinberg formula 31, 156

collective coordinate 139–40, 149

for eleventh dimension 202, 204

commutators and anticommutators

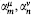[] 6

{b_m, c_n} 16

{β_r, γ_s} 16

[E_α, E_β] 61

{G_r, G_s} 7

[Hⁱ, E_α] 61

[] 66

[L_m, G_r] 7

[L_m, 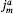] 66

[L_m, L_n] 7

{ψ_r, 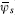} 14

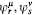{} 6

N = 2 superconformal 376

{Q_B, b_n} 24

[Q_B, β_r] 24

[T^a, T^b] 59

see also supersymmetry algebras

compactification 274

scale 344, 350

see also (0, 2), (2, 2), Calabi–Yau, Gepner, orbifold, toroidal compactifications

complex manifold 306–7

complex structure 306–7

hyper-Kähler manifold 463

large 403, 405

complex structure moduli 291, 314–15, 381

conifold singularity 409–15

effective action 319–20, 472

K3 416

moduli space 296, 383–4, 402–9

orbifold 291, 296, 299

quintic 310

conformal block 235, 272

differential equation 237–8

in rational CFT 255–7

conformal bootstrap 233–6

conformal family 229

degenerate 232, 239

conformal field theory (CFT)

c < 1 236–42

c = 1 35–7

density of states 222, 236

irrational 258–9, 471

nonunitary 232

rational 255–8, 471

unitary 45, 228–32

see also bc, βγ, ψ, coset, Landau–Ginzburg, parafermion CFTs, current algebras, minimal models, superconformal field theory

conformal gauge 20

conformal invariance

constraints on correlators 233–5

vs scale invariance 260, 262–3

conformal perturbation theory 263–6

conifold 405, 409–10, 474

massless 3-brane 411–12

singularity in CFT 410–11

transition 412–15

constraint algebra 3, 20, 24, 45–9

coset CFT 250–4, 272, 471

from gauged world-sheet symmetry 251

minimal models 250–1

N = 1 minimal models 255

parafermion CFTs 251–2

W algebras 253–4

cosmological constant 226, 372–3, 473

coupling constants

gauge 298, 361

unification 314, 345–52

relations among 107–10, 150–2, 335–6, 472

string vs M-theory 199

Yukawa 317, 331

critical dimension 4, 47–8, 198

critical phenomena 266–71, 393, 400

current algebra 66–73, 243–50, 272, 468, 471

and coset models 250–4

and unification 337–8, 351, 353–4

bosonic representation 74–5

fermionic representation 68

gauging 94–5

KZ equation 244

level 67

modular invariants 245–6, 249–50

primary fields 72–3

restriction on representations 72–3, 289, 337–8, 349

Sugawara construction 69, 82

superconformal 338–42

WZNW models 246–50

cycle 306, 319–20

collapsing 404, 410–13, 425, 427

D-brane wrapped on 404, 411–13, 425

intersection number 317–18

monodromy 410

world-sheet wrapped on 322–4, 406, 408

cylinder

D-brane interactions from 146–7, 155–60, 176, 196

in type I theory 37–9, 135

D-branes 138–77, 469–70

action 149–50

and black p-branes 219–23, 227

D1–D5 black p-brane 220–23

as BPS states 140–1, 145, 226

as R–R sources 141–2

charge 146–7

coincident, non-Abelian dynamics 149, 166, 211–23

D0-brane quantum mechanics 161–2

in matrix theory 211–14, 217–18

D0-branes as Kaluza–Klein states 198–9

D1-branes and IIB duality 180–2

D3-branes and Montonen–Olive duality 180–2

Dp–Dp′ system 152–8, 162–4, 175–6

BPS bound 168

effect of T-duality on 143–4, 429

gauge coupling 151

in type I theory 190–7

interactions between 175–6

parallel 146–7

rotated 154–8

velocity-dependent 158–61

on collapsing cycle 411–15, 425

p even, relation to M-theory 200–8

substring length scale 160–2, 412

tension 146, 150

see also bound states

D-instanton 142, 145, 334, 372, 469

D_k see SO(2k)

D-terms

four supersymmetries 364–6, 399, 443–4, 473

and orbifold blow-up modes 297

eight supersymmetries 462

in Dp-Dp′ system 163, 172, 221–2

descendants 229, 233–4, 271

diagonal modular invariant 36

current algebra 246, 249

heterotic string 55

type 0 superstring 35

differential forms 305–6, 450–2

dilaton

and string loop expansion 87–9, 360–2, 383–4

coupling to R–R fields 87–9

in chiral multiplet 294, 315

in hypermultiplet 380, 383–4, 429

in vector multiplet 428

dilaton-mediated supersymmetry breaking 370

dimensional reduction

of action 84–6, 292–5, 374

of couplings 206, 335

of d = 10 Yang–Mills 459–61

of spectrum 78–80, 84–6, 188, 453–5

of supersymmetry 79–80, 311, 454, 458, 463–4

to d = 2 392, 399, 449, 466

Dirac–Born–Infeld action 149

Dirac equation 1–2, 22, 88, 179

Dirac matrices 1–2, 8, 430

Dirac quantization condition 147–9, 183–5, 196, 247–8, 355, 366

Dirac spinor 8, 430–2

Dirichlet boundary condition 139, 142, 143, 152

discrete light-cone quantization (DLCQ) 217

discrete series, see minimal models

discrete symmetry (spacetime) 328–35, 472

Z₅ example 397–8

see also S-, T-, U-dualities

discrete torsion 58, 468

disk amplitudes 102, 110–12, 114–16, 134, 175

tadpole 38–9, 42, 147

divergences, cancellation 37–42, 102–3, 135, 142–3, 468

doubling trick 7–8, 153

dual Coxeter number 63–4, 71

duality, see Montonen–Olive, S-, string–string, T-, and U-dualities

E₆ 64–5, 82, 285ff, 312, 386–8

singlets 313, 315, 324–6, 388

see also grand unification

E₆₍₆₎ 188–9

E₇ 82

E₈ 54, 63–4, 82–3, 285, 290

and anomalies 100, 110, 134

root lattice 74–5

Eguchi–Hanson space 309–10

electroweak scale 343, 369

energy-momentum tensor 1–5, 50

in bosonization 12, 15, 18

in type I theory 37–9, 135

topological 385

enhanced gauge symmetries

at Gepner point 398

at orbifold points 309–10, 312–13

on coincident branes 149, 185–6

on singular manifolds 425, 427

on small SO(32) instantons 195–6

winding state 66, 76–7

Euler number (Calabi–Yau) 305, 309, 414–15

extended chiral algebra 253–4, 256

extended supersymmetry 359, 440, 447–9, 473–4

exterior derivative 305, 307, 450

F-terms 399, 443–5

F-theory 200–1, 474

Faddeev–Popov ghosts 15–20, 46–7

Fayet–Iliopoulos term 364–5, 399, 443, 446

Feigin–Fuchs representation 241–2, 272

fermion number

spacetime 32

world-sheet 8–9, 19, 22ff, 32–3

Feynman diagrams 102, 176, 316

fibrations 404, 428

fixed point

orbifold 275, 284, 286

blowing up 297, 309–10, 388, 471

field theory 266

nontrivial 260–1, 415, 421, 460–1

flipped SU(5) 348

flop 406–9, 474

fractional charge 352–5

fractional string theory 46, 468

free fermion models 279–80, 471–2

free parameters, absence of 369, 373

fundamental (F-) string 145, 150

fundamental region 31, 40, 284

fusion rule 238–40, 244–5, 256–8, 270–1

gauge coupling, see coupling constants, gauge

gauge-fixing 20

gauginos 30, 315

condensation 367–9

general relativity 183, 408, 429

generation (quark and lepton) 64–5, 288–92, 313–15, 324–6, 387–8, 471–2

gen.-changing transition 414–15, 474

Gepner models 394–405, 473

relation to Calabi–Yau 397–402

Gliozzi–Scherk–Olive (GSO) projection 27, 30, 33

and spin–statistics 29

and supersymmetry 50–1, 359

current algebra fermions 51–5, 82, 193, 386

diagonal 28, 35–7, 268

global symmetry 45, 94, 327–8, 334–5

goldstino 140

Goldstone boson 140

grand unification 63–5, 81, 281, 288–90, 351–6

partial 348

scale 298–9, 345–50

symmetry breaking 289, 325–6, 337–8, 349

gravitational scale 180, 344

eleven-dimensional 199, 212

gravitino 28–31, 79, 286, 315, 441

world-sheet 126

Green–Schwarz mechanism 99–101, 365, 462

Green–Schwarz superstring 29, 49, 133

H-monopole 82

Hagedorn temperature 83

Hamiltonian, D0-brane 161–2, 212

heterotic 5-brane 195

heterotic string 45–83, 468

(0,2) and (1,2) 48

amplitudes 112–13, 126–33, 134, 373–4

bosonic form 73–5

E₈ × E₈ 53–5

at strong coupling 205–8

fermionic form 49–50

nonsupersymmetric 55–9

SO(16) × SO(16) 57–9

SO(32) 51–3

at strong coupling 190–4

T-duality between SO(32) and E₈ ×E₈ 78

toroidal compactification 76–82, 422–9

hidden sector 289, 367–71

hierarchy (Higgs naturalness) problem 281–2, 350, 369

highest weight (primary) state 229ff

current algebra 72–3, 243

N = 2 chiral 378–9, 384–5

superconformal 105

Hodge numbers 309–10, 315, 402–3, 414, 416

holomorphic fields 45–6, 255

holonomy 304ff, 415–17, 463–4

homology 306, 317

hyper-Kähler manifold 417, 463–4

hypermultiplet 380–4ff, 447, 461–4

from p-p′ strings 163, 172–3, 196

index theorem 330

inheritance principle 290, 293, 314, 351

instanton (Pontrjagin) number 187, 333

instantons 334–5

D- 142, 145, 334, 372, 469

world-sheet 323–5, 383, 406, 408, 472

Yang–Mills 172–5, 194–6, 418–20, 470

intersection number 317–18

irrelevant interaction 192, 264

Ising model 266–70

tricritical 269

Jacobi’s abstruse identity 35

K3 manifold 301, 415–18

and heterotic string 418–21

and type II string 415–18, 425–8

Kac determinant 230–3, 242, 254, 271, 471

Kac–Moody algebra 66

Kähler class 308

Kähler form 307–8

Kähler manifold 307–8, 446

and supersymmetry 311, 446

Ricci-flat 308–11

see also hyper-Kähler manifold, special Kähler manifold

Kähler moduli 291, 315, 381

effective action 316–19, 323, 381–4, 472

K3 416

moduli space 296, 381–3, 402–9

Kähler cone 406–8

Kähler potential 294–8, 308, 359–62, 390, 446–7

Kähler transformation 296, 308, 446–7

Kaluza–Klein gauge symmetry 76, 79, 86, 188–9

and supersymmetry algebra 454–6

vertex operators 66

Kaluza–Klein monopole 81, 205

Kaluza–Klein states 198

Klein bottle 39–41

Klein–Gordon equation 1

Knizhnik–Zamolodchikov (KZ) equation 244, 272

Landau–Ginzburg model 270–1, 392–4, 400, 471, 473

large-N limit 223

large order behavior 145

lattice (momentum) 19, 74–8, 83

Laurent expansion, see mode expansion

length, minimum 160–2, 412

level (current algebra) 67

level-matching 25, 57, 276–9, 284–5ff, 395, 468

Lie algebra 59–65, 468

and grand unification 63–5

normalization of generators 67–8, 93, 112, 151, 336

roots and weights 61–3, 74–6

simply-laced 63

light-cone quantization 212, 216

calculations 117–18, 131–3

discrete (DLCQ) 217–18

lightlike limit 217–18

linear dilaton CFT 5, 15, 43, 230

applications 89, 185, 241–2, 272

linear multiplet 294

linear sigma model 398–402, 408

Lorentz generators 8–9, 431

Lorentz invariance 9, 331

eleven-dimensional 212

local 96

M-theory 198–205, 208–11, 426–7, 470

on S₁/Z₂ 207–8, 350, 419–20

M2-brane 201–2, 470

in matrix theory 214–16

stretched 204–5, 421

wrapped 427

M5-brane 201–5, 419–21, 470

macroscopic string 81

magnetic monopoles 81–2, 187, 205, 353, 468, 470

Dirac quantization condition 147–8, 355

Majorana spinor 433–4, 437

Majorana–Weyl spinor 433

manifold

Calabi–Yau 309–11

complex 306–7

group 246–50

hyper-Kähler 463

Kähler 307–8

quaternionic 464

real 305–6

SU(3) holonomy 308–9

special Kähler 464–5

marginal interaction 264–5

mass-shell condition 1, 20, 23

matrix theory 211–18, 226, 470

Maurer–Cartan 1-form 248

measure, supermoduli 124–6

membrane, see M2-brane

Mermin–Wagner–Coleman theorem 140

minimal models 236–42

coset construction 250–1

Feigin–Fuchs representation 241–2

fusion rule 238

Ising model 267–9

Landau–Ginzburg models 270–1

mirror symmetry 402–4, 473–4

and conifold transition 401, 414

and flop transition 406–9

and Kähler moduli space 404–9, 428–9

Möbius invariance 233

Möbius strip 39, 41–2, 135

mode (Laurent) expansion

b, c, β, γ 16

free scalar (X^µ) 6

j^a 66

N = 2 superconformal 376

p-p′ strings 153–5

ψ 6–8, 14

T_B, T_F 7

model-independent axion 333

modular invariance 33–7, 235–6, 471

A–D–E invariants 246, 250–1

and anomalies 103

current algebra 245–6, 249–50

Gepner models 395

heterotic string 52–3, 55–8, 82, 388–9

minimal model 251

orbifold 276–9

rational CFT 268

superstring 26–7, 33–5

modular transformations 34, 38, 156

modular weights 297

moduli, physical effects 291, 324–6, 331, 370–3, 445

in threshold correction 298–300, 349

moduli space

(2, 2), product form 381, 389–90

IIB theory 91–2

as spacetime 404, 417

branches 172–4, 373, 412–15, 419–21

D1–D5 system 221–2

dilaton–axion 296, 428, 457

for toroidal compactification 76–8, 81, 91–2, 427–8, 455–8

K3 417–19, 427–8, 474

metric on 362, 374

orbifold 296–7, 472

with 8 supersymmetries 462–5, 475

with 16 supersymmetries 159, 460, 475

see also complex structure, Kähler moduli

monodromy 240, 252, 410, 471

Montonen–Olive duality 186–7, 428, 470

multicritical behavior 268–70

NS algebra 7, 43

NS boundary condition 5–8, 15–16

vertex operators 10–13, 16–18

NS–NS charge 81

in supersymmetry algebra 81–2

magnetic 81–2, 145, 183–5, 189

NS–NS fields 87

background 106

tadpole 37–42

NS5-brane 470

IIA 202–4, 426

IIB 182–6, 202–4

multiple 186

heterotic 195

Narain compactification 73–9, 83, 468

naturalness problem 282, 350, 369

Neumann boundary condition 141, 143, 152

no-ghost theorem 25, 50, 232–3

no-scale models 371

noncommutative geometry 149, 161

nonlinear sigma model 106–8, 247, 311, 469, 471

nonperturbative definition (of string theory) 180–1, 208–11

nonperturbative effects 49, 134, 145, 178, 343, 366–73, 421–2, 425

see also instantons, strong coupling

nonrenormalization theorems 133–4, 300

α′ corrections 111–17, 185, 321–5, 382–3, 472

perturbative, violation by instantons 323–5, 367–8, 372, 472

string corrections 469, 473

4 supersymmetries 361–2, 364–5, 444

8 supersymmetries 381, 383–4, 410–11, 428–9

16 supersymmetries 133–4, 159, 194, 460–1

32 supersymmetries 133–4, 180

normal ordering 2, 7, 43

null state 231–3, 236–9, 243–5, 252–3

decoupling of 98, 101–2, 119, 121

old covariant quantization (OCQ) 3, 20–3, 25

one-loop string amplitudes 31–42, 126–34, 135, 146–7, 155–60, 298–300, 374, 468–9, 472

operator product expansion (OPE) 43, 82–3, 95–6

and bosonization 11–12, 17–19

B C 106, 134

βγ CFT 17–19

closure and locality 26, 52–5, 74

H H 11

in Sugawara construction 69–71

j^a j^b 66, 68

357

j_B β 24

j_B β 24

λ^Aλ^B 49

λ^aλ^b 73

N = 2 superconformal 37, 47, 375

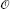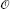_{m n} 234

ψ _ν 14

ψ_l ψ_l 252

ψ^µ ψ^ν 2, 49

ψ^µ Θ_α 112

superconformal current algebra 339

T_B 233

T_B T_B 3–4

T_B T_F 3–4

T_F 10

T_F T_F 3–4, 280

X^µ X^ν 2, 49

X^µ X^ν 106, 134

114

W W 253

orbifolds 274–301, 331ff, 471–2

asymmetric 279

fixed points 311

blowing up 296–7, 309–10, 312–13

K3 300–1, 417, 425, 427

modular invariance 276–9

non-Abelian 275

nonsingularity of CFT 275, 410, 425

T-duality 295–6

twisted sector 286–8, 296–8

twisting construction 55–9

Z₃ 283–91, 300, 309–10

Z₄ 291–2, 300

orientifold 196, 422

orientifold plane 138, 142–4, 147, 151, 175, 206–8

p-brane, see black p-, D-, M2-, M5-, and NS5-branes

p-form, see differential forms

p-form gauge field 85, 451–2

field strength 86

self-dual 91, 452

see also Chern–Simons term, R–R fields

p-p′ string 153–5, 162–4

= 4 162–4, 197

= 8 191

(p, q)-form 307–9

(p, q) string 167, 176–7, 182

P invariance, see parity

parafermion CFT 251–2, 269, 391–2, 471

parallel transport 304, 463

parity

and anomalies 95–8, 285, 304, 329–30

and T-duality 137

spacetime 27–8, 127

world-sheet 27–31, 41

partition functions 235–6, 266, 272, 471

and bosonization 13

BPS states 171, 190

GSO projected 32–7, 51–3, 55–8

with twists 33–4, 156

Pati–Salam unification 348

Pauli–Villars regulator 95, 285

Peccei–Quinn (PQ) symmetry 333–5, 360–2, 365–6, 371–2, 382–3, 473

physical states 1, 3, 20–3, 28, 45

picture changing operator (PCO) 118–21, 126, 469

pictures 108–10, 118–21, 147

Planck scale, eleven-dimensional 199, 212

Poincaré duality 86, 450–2

point group 275

primary field, see highest weight state, tensor field

projective plane 39, 42

projective space 310–11, 320, 398, 404, 465

proton decay 355–6, 472

pseudospin 67, 244

quantum mechanics 210, 225–6, 429

quaternionic manifold 464

R symmetry 393, 444

rank (algebra) 61, 77, 326, 421, 457–8

rank (form) 10, 85, 450

Ramond algebra 7, 43

Ramond (R) boundary condition 5–9, 15–16

vertex operators 13–15, 19

R–R charge

in supersymmetry algebra 144–5

of black p-brane 219

of D-brane 141–52, 425

value 147

of orientifold plane 142–3

vanishes for strings 88–9, 92, 381

R–R fields 9–10, 27, 87, 334

background 106

coupling of dilaton 87–9

effect of T-duality 137–8

tadpole 37–42

Ramond–Neveu–Schwarz (RNS) superstring 29

rational CFT 255–9, 272–3

refermionization 131–3, 156–7

relevant interaction 166, 264–6, 268–71

renormalization 393, 445

renormalization group 259–66, 346, 350, 369

Ricci flatness 308–9

Ricci form 308–9

Riemann–Roch theorem 121–3

Riemann surfaces 13, 122–4

Riemann tensor 96, 113

roots 61–3, 67–8, 425

lattice 74–5

S-duality (weak–strong) 179–82, 186–7, 190–2, 198–9, 205–7, 422–9

SL(2, R) 91–2, 182

SL(n, R) 61, 187–8

SL(2, Z) 182, 186–8, 200, 295–6, 427–8, 474

SL(3, Z) 200

SO(4) = SU(2) × SU(2) 439

[S]O(5, 5, Z) 200

SO(8) spin 22–3

SO(10) 64–5, 82, 289, 338, 346

SU(1, 1) 296, 428

SU(5) 64–5, 82, 289, 338, 345–8, 352–6

SO(n) 60, 62

traces 99

[S]O(m, n, R) 61, 76, 187–8, 417, 427–8

[S]O(m, n, Z) 76, 187–8, 428

SU(n) 60, 63

S-matrix 124

scale transformation 259–63

Scherk–Schwarz mechanism 363–4

short multiplet 170, 449

simple current 240–1, 272, 354

sin² θ_w 290, 346–54, 472

soliton 173, 182, 203–5, 426

Sp(k) 60, 62

space group 275

special Kähler geometry 319–20, 381–3, 390, 405, 464–5

spectral flow 14, 379, 387

sphere amplitudes 112–18, 134

relation to disk 116

spin 22–3

spin connection 203, 303

embedded in gauge connection 284, 291, 304, 324, 418–19

and (2, 2) supersymmetry 311–12, 376

spin field 15, 19, 131–2

current algebra 54, 66

four-dimensional 341, 356

spin–statistics relation 19, 29, 33, 35, 49, 52, 55, 82, 332

spin structure 122–4, 127, 130–2

spinor representations 430–9

products 435–7

SO(N) 437–8

under subgroups 438–9

Standard Model 178, 274, 326, 370–3, 421–2

anomalies 94, 98

CP symmetry 331–5

chirality 98, 282, 288–9, 329–31

gauge couplings 298, 314, 343–52

gauge group 64–5, 81, 289–90

gauge quantum numbers 337–8, 340–3, 352–5

generations 64–5, 288–92, 313–15, 324–6, 387–8, 471–2

hierarchy problem 281–2, 350, 369

proton stability 355–6

supersymmetric 346–8

Yukawa couplings 314, 318

state–operator isomorphism, see vertex operators

statistical mechanics 219, 224–5, 260, 266–71

Stokes’s theorem 450

string coupling, see coupling constants

string field theory 210, 259

string metric 205

string scale 343–50

string–string duality 425, 429

string tension 150, 164–7, 180

strong coupling limit

heterotic string 190–4, 205–8, 421–9

type I superstring 190–4

type IIA superstring 198–9

type IIB superstring 179–81

strong CP problem 333–5, 472

structure constants 59

Sugawara construction 69–72, 82, 243, 247, 250, 339, 468

superconformal algebra 3–4, 7, 272

as constraint 20, 45

general N 45–9

N = 2 37, 47–8, 82, 375–6

primary field 105

superconformal current algebra (SCCA) 338–42

superconformal field theory (SCFT) 467–8

(1, 1) 2–5, 254–5

(2, 2) 292, 311–12, 380–415, 473

Landau–Ginzburg models 392–4

minimal models 390–2

(4, 4) 417–18

superconformal Killing vector 123

superconformal transformation 2, 10, 43, 103–4

supercurrent 2–5, 50

superderivative 103–4

superfield 104

supergravity 468

d = 4, N = 1 293–5, 315, 359–62

eleven-dimensional 84–7, 198–9, 201, 453

long-distance 160, 176, 213–14

toroidally compactified 187–9, 454–7

type I 52–3, 92–3, 98–101

same as heterotic 93, 192

type IIA 28–9, 87–90, 96

type IIB 28–9, 90–2, 98

supermanifold, see superspace

supermembrane, see M2-brane

supermoduli space 121–6

boundary of 102–3

superpartner scale 343, 350, 369

superpotential 443–7

Calabi–Yau 315–20, 325, 390

constraints from holomorphicity 322–3, 359–62, 367–8

Landau–Ginzburg/linear sigma model 392, 398, 400

nonperturbative contributions 323–4, 371–2

orbifold 294–6

super-Riemann surface 121–4

superspace 103–8, 134

actions 105–8, 469

superstring, see types 0, I, IIA, IIB

supersymmetry (spacetime) 471

4 supersymmetries 440–7

8 supersymmetries 461–5

16 supersymmetries 457–61

32 supersymmetries 452–7

and GSO projection 29–30, 50–1, 359

and hierarchy problem 281–2

and sin² θ_w 346–8, 473

and vanishing of vacuum amplitude 35, 38, 146

as means of studying strong

coupling 178–227

conditions for unbroken

D-brane systems 152–5, 175–7

general CFT 356–9, 374, 473

orbifolds 282–3, 300

smooth manifolds 302–5

d = 2 449

d = 4, N = 1 440–7

d = 4, N > 1 448–9

in specific systems

D0–Dp system 168

F-string–D-string system 164

heterotic string 79–82

type I/II superstring 144

in statistical mechanics 269

transformations 173, 203, 302, 443

vs world-sheet 29

see also BPS bound, BPS states, nonrenormalization

supersymmetry breaking 473

at tree level 362–4

by D-branes 139–40, 152–5, 168, 175–7

dilaton-mediated 370

in loop expansion 364–6

nonperturbative 366–73

scale 369

symmetries, continuous 327–8

T-duality 452, 469

and mirror symmetry 402–4, 473

and U-duality 187–8

as a gauge symmetry 328

combined with S-duality 199, 205–7, 227, 422–7

effect on D-branes 138–45, 163–4ff, 176

effect on R–R fields 137, 149

heterotic theories 76–8

on orbifolds 295–6, 298, 300, 472

type I theory 138–43

type II theories 136–8

’t Hooft–Polyakov monopole 82, 187

tachyon 21–3, 82, 266, 341

in nonsupersymmetric D-brane systems 158, 169

removal by GSO projection 27, 31, 52, 54, 58–9

theories with 27, 55–8

tadpoles 37–42, 142–3

temperature 83, 219, 266–9

tensionless string theory 205, 260–1, 421, 474

tensor multiplet

d = 6 (1, 0) 418, 420, 461–2

d = 6 (2, 0) 204, 417, 459

tensor superfield 104

tetrad 77, 96

theta functions 33, 156, 469

Thirring model 36

threshold correction 298–300, 334, 349, 370–1, 472

topological string theory 49, 385

topology change (spacetime)

conifold 412–15, 474

flop 406–9, 474

toric geometry 404

toroidal compactification

heterotic string 76–82, 422–9, 468, 474

type I string 143–4

type II string 187–90, 454–7

torsion 106, 203, 303, 324

torus amplitudes 31–5, 51–9, 126–33, 374

transition functions 122, 125, 306

tree-level amplitudes 110–18, 135

trinification 349

twist (orbifold) construction 55–8, 251, 275–6, 279

twisted boundary conditions 13–4, 32–4, 44, 155–6ff

twisted sector 276–9, 286–8

type 0 superstring 27, 35

type I superstring 31, 37–42

amplitudes 110–16, 135

strong coupling limit 190–7

T-duality 138, 143–4

type II superstrings 26–9, 31–7

amplitudes 113–18, 134

on Calabi–Yau manifolds 379–86ff

on K3 415–18

strong coupling limits 179–87, 198–205

T-duality between 136–8, 143–4

toroidally compactified 187–90, 454–7

U-duality 187–90, 199–201, 226, 328

U(n) 30, 63, 151, 186–7

UV divergence 103

ultrashort multiplet 80, 169–71, 210, 449

unitary CFT 45–6, 228–32, 254–5, 262–3, 391, 471

vacuum amplitudes 31–42, 146–7, 155–8

vacuum selection 373

vector multiplets

d = 4, N = 1 441

d = 4, N = 2 380–4ff, 447, 463–5

d = 4, N = 4 79, 448

d = 6 (1,0) 163, 172, 461

d = 6 (1,1) 204, 459

Verlinde formula 258, 273

Verma module 232, 236

vertex operators 10–15, 108–10, 468, 471

and chiral primaries 384–9

ghosts 15–20

locality 25–6, 50–1, 256–7

gravitino 28

p-p′ strings 174, 197

pictures 108, 118–24

Ramond, branch cuts 6, 10

R–R 30, 88

Virasoro algebra 228–33ff

Virasoro master equation 258

W algebra 46, 471

W string 46, 468

WZNW model 246–50

weight (conformal) 43, 263

current algebra primary 72

unitary CFTs 231, 255, 378, 391

weight (Lie algebra) 61–3

lattice 75

weight (modular) 297

Wess–Zumino term 248

Weyl spinor 8, 432

Wilson criterion (computability) 209–11, 214

Wilson line 76–8, 83, 206, 208, 227, 429

gauge symmetry breaking 289–91, 314, 325–6

Wilsonian action 300, 361

winding string 77–81, 189–90

Yang–Mills theory, d = 4, N = 4 186–7, 428, 460–1

Zamolodchikov metric 362, 374, 389

zero modes 119

instanton 324–5

sphere 108

torus 121, 123, 127, 130, 132–3

zero-norm states, see null states

zero-point energy 7, 14, 34