CONTENTS

CHAPTER

I. PROPERTIES OF THE TRIANGLE

Methods of solution

circumcentre, incentre, orthocentre, nine-point centre, polar circle

centroid

distance between special points

errors

II. PROPERTIES OF THE QUADRILATERAL

Cyclic quadrilateral

general quadrilateral

circumscribable quadrilateral

III. EQUATIONS, SUB-MULTIPLE ANGLES, INVERSE FUNCTIONS

General solutions

sub-multiple angles

inverse functions

IV. A HYPERBOLIC FUNCTION AND LOGARITHMIC AND EXPONENTIAL FUNCTIONS

Area-function for rect. hyperbola

differentiation

addition theorem

properties of log x and e^x

useful inequalities

Euler’s constant

V. EXPANSIONS IN POWER-SERIES

Convergence

expansions of sin x and cos x

expansion of log(1 + x)

expansion of tan^–1x

evaluation of π

expansion of e^x

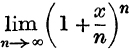
VI. THE SPECIAL HYPERBOLIC FUNCTIONS

Definitions, sh x, ch x, th x

formulae

calculus applications

sh^–1x, ch^–1x th^–1x

VII. PROJECTION AND FINITE SERIES

Projections and general angles

cos(A + B), sin(A + B)

∑ cos [α + (r – 1)/β], etc.

difference series

VIII. COMPLEX NUMBERS

Definitions

notation and manipulation

modulus and amplitude

use of Argand Diagram

products and quotients

principal values

IX. DE MOIVRE’S THEOREM AND APPLICATIONS

De Moivre’s theorem

principal values

values of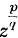

powers and roots in Argand Diagram

expansions of cosⁿθ, sinⁿθ

expansions of cos nθ, sin nθ, tan nθ

∑x^r cos rθ, etc.

cos nθ, 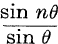as polynomials in cos θ, etc..

X. ONE-VALUED FUNCTIONS OF A COMPLEX VARIABLE

Absolute convergence

series of complex terms

exponential series and exponential function

modulus and amplitude of exp (z)

sin z, cos z, tan z

sh z, ch z, th z

XI. ROOTS OF EQUATIONS

Formation of equations

symmetric functions of the roots

essentially distinct roots

XII. FACTORS

Algebraic functions

trigonometric functions, sin nθ, etc.

x²ⁿ – 2xⁿ cos nα + 1

comparison of series and products

partial fractions

XIII. MANY-VALUED FUNCTIONS OF A COMPLEX VARIABLE

Log w

expansion of log (1 + w)

circle of convergence

z^w

binomial series

logarithms to any base

inverse functions

XIV. MISCELLANEOUS RELATIONS

General identities

conditional identities

miscellaneous transformations

elimination

inequalities

MISCELLANEOUS EXAMPLES ON CHAPTERS I-XIV

ANSWERS

INDEX

SYMBOLS